On the dimension of the divergence set of the Ostrovsky equation
2022-08-25YajuanZHAO赵雅娟
Yajuan ZHAO(赵雅娟)+
Henan Academy of Big Data,Zhengzhou University,Zhengzhou 450001,China E-mail : zhaoyj-91@zzu.edu.cn
Yongsheng LI (李用声)
School of Mathematics,South China University of Technologg,Guangzhou 510640,China E-mail : gshli@scut.edu.cn
Wei YAN(闫威)Xiangqian YAN (闫向前)
School of Mathematics and Information Science,Henan Normal University,Xinaciang 453007,China E-mail: 011133@htu.edu.cn; yan.aiangqian213@126.com
1 Introduction
The Ostrovsky equation

was proposed by Ostrovsky[12,19,26]as a model for weakly nonlinear long waves in a rotating liquid that take into account of the Coriolis force. The equation describes the propagation of surface waves in the ocean in a rotating frame of reference. Some researchers have investigated the Cauchy problem for the Ostrovsky equation;see[6,13–16,22,30,32,34]and the references therein.


2 Preliminaries
In this section, inspired by [28], we give a crucial oscillatory integrals estimate related to the free Ostrovsky equation. First of all, we recall the following well-known variant of van der Corput’s Lemma:
Lemma 2.1 ([29] P. 332-334) For a <b and I =[a,b], let Φ ∈C∞(I) be real-valued and let ψ ∈C∞(I). Then,





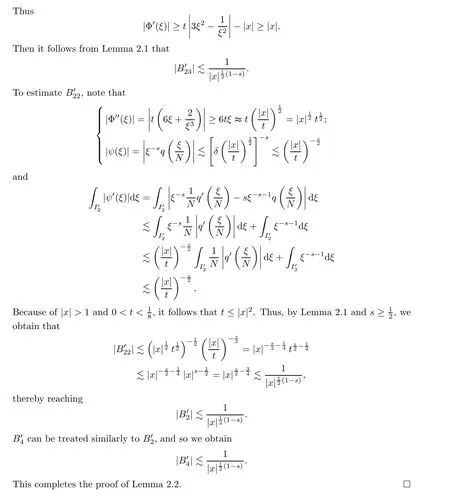
3 Demonstration of Theorem 1.1
3.1 Proof of Theorem 1.1(i)



3.2 Proof of Theorem 1.1(ii)

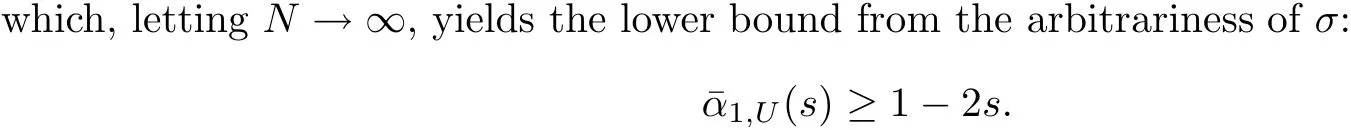
4 Appendix
In this section, we will give the proof of (1.4). First, we state and prove a Lemma which will be used to prove (1.4).


杂志排行
Acta Mathematica Scientia(English Series)的其它文章
- ITERATIVE ALGORITHMS FOR SYSTEM OF VARIATIONAL INCLUSIONS IN HADAMARD MANIFOLDS*
- Time analyticity for the heat equation on gradient shrinking Ricci solitons
- The metric generalized inverse and its single-value selection in the pricing of contingent claims in an incomplete financial market
- The global combined quasi-neutral and zero-electron-mass limit of non-isentropic Euler-Poisson systems
- Some further results for holomorphic maps on parabolic Riemann surfaces
- Global well-posedness of the 2D Boussinesq equations with partial dissipation
