Time analyticity for the heat equation on gradient shrinking Ricci solitons

.Jiayong WU (吴加勇)

Department of Mathematics，Shanghai University，Shanghai 200444，China E-mail: wujiayong@shu.edu.cn

Recall that an n-dimensional Riemannian manifold (M，g) is called a gradient shrinking Ricci soliton (M，g，f) (also called a shrinker for short) (see [10]) if there exists a smooth potential function f on M such that

where A3is some constant depending only on n and A2， and 00=1. Here µ=µ(g，1) denotes Perelman’s entropy functional.

Remark 1.2 The growth condition is necessary. As in [3]， let v(x，t) be Tychonov’s solution of the heat equation in (Rn，δij，|x|2/4)×R such that v =0 if t ≤0 and v is nontrivial for t ＞0. Then u := v(x，t+1) is a nontrivial ancient solution of the heat equation and is not analytic in time. Note that |u(x，t)| grows faster than ec|x|2for any c ＞0， but for any∈＞0， |u(x，t)| is bounded by c1ec2|x|2+∈for some constants c1and c2. This implies that growth condition (1.4) is sharp.

In general， the Cauchy problem to the backward heat equation is not solvable. However，on a complete non-compact shrinker， we can obtain a solvable result by a simple application of Theorem 1.1.

Corollary 1.3 Let(M，g，f)be an n-dimensional complete non-compact gradient shrinking Ricci soliton satisfying (1.1)， (1.2)and (1.3). For a fixed point p ∈M， the Cauchy problem for the backward heat equation

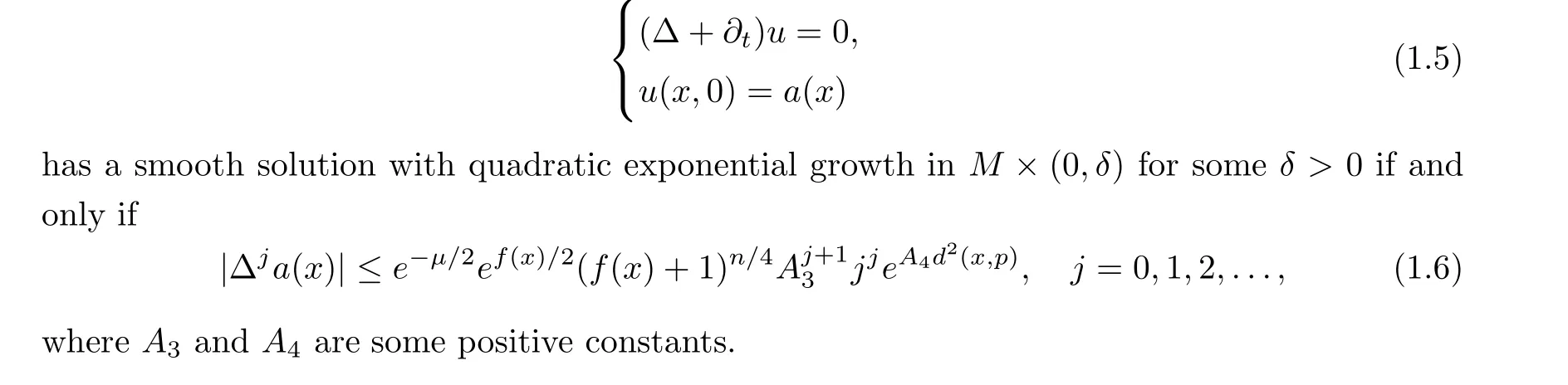
The rest of this paper is organized as follows: in Section 2， we recall some properties of gradient shrinking Ricci solitons. In particular， we give a local mean value type inequality on gradient shrinking Ricci solitons. In Section 3， adapting Dong-Zhang’s proof strategy [3]， we apply the mean value type inequality of Section 2 to prove Theorem 1.1 and Corollary 1.3.

2 Some Properties of Shrinkers

In this section， we will present some basic propositions about complete non-compact gradient shrinking Ricci solitons; these will be used in the proofs of our main results.

On an n-dimensional complete non-compact gradient shrinking Ricci soliton(M，g，f)satisfying (1.1)， (1.2)and(1.3)， from Chen’s work(Proposition 2.2 in[16])，we know that the scalar curvature is R ≥0. Moreover， by [17]， we know that the scalar curvature R must be strictly positive， unless (M，g，f) is the Gaussian shrinking Ricci soliton (Rn，δij，|x|2/4).

For any fixed point p ∈M， by Theorem 1.1 of Cao-Zhou [18] (later refined by Chow et al.[19])， we have on (M，g，f). It remains an interesting open question as to whether or not the scalar curvature R is bounded from above by a uniform constant.

By Cao-Zhou [18] and Munteanu [20]， the volume growth of a gradient shrinking Ricci soliton can be regarded as an analogue of Bishop’s theorem for manifolds with non-negative Ricci curvature (see [21]). That is， there exists a constant c(n) depending only on n such that

The point(p，s)and the radius r in Proposition 2.2 is customarily called the vertex and the size of parabolic cylinder Qr(p，s)， respectively. Compared with the classical mean value type inequality of manifolds， there seems to be a lack of a volume factor Vp(r) in (2.4). However if the factor rnis regarded as Vp(r)， this inequality is very similar to the classical case.

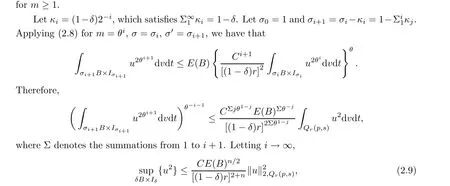
which clearly implies (2.4) when m=2， since Iδ2⊂Iδ.

The case m ＞2 then follows by the case m = 2， because if u is a nonnegative solution of(Δ-∂t)v ≥0， then um， m ≥1， is also a nonnegative solution of (Δ-∂t)v ≥0. All in all， we do， in fact， prove (2.4) when m ≥2.

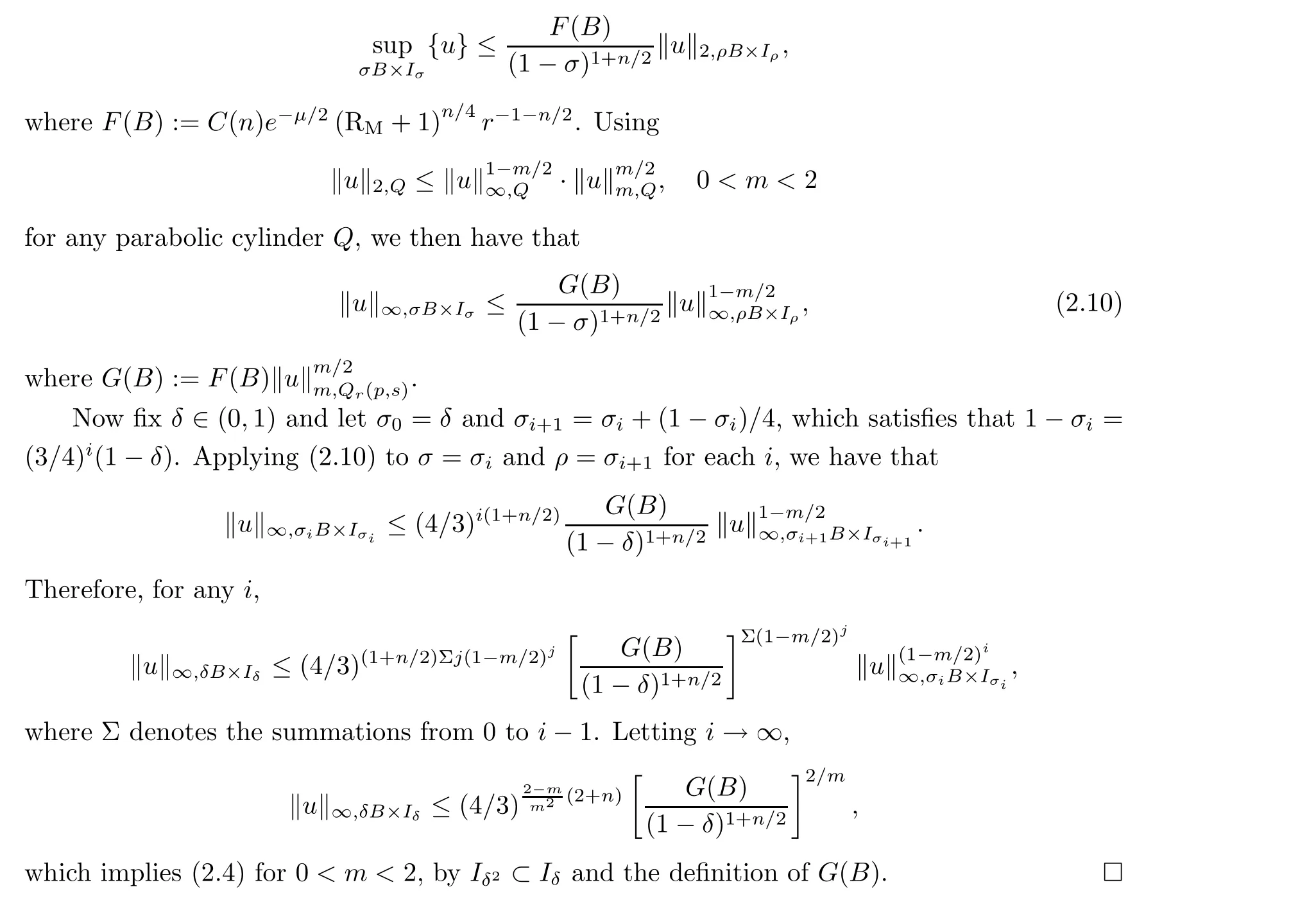
When 0 ＜m ＜2， we will apply (2.9) to prove (2.4) by a different iterative argument. Let σ ∈(0，1) and ρ=σ+(1-σ)/4. Then (2.9) implies that

3 Proof of Results

In this section， adapting the argument of Dong-Zhang [3]， we will apply the preceding propositions of shrinkers in Section 2 to prove Theorem 1.1 and Corollary 1.3. We first prove Theorem 1.1.

Proof of Theorem 1.1 Without loss of generality，we may assume that A1=1，because the heat equation is linear. To prove the theorem，it suffices to confirm the result at space-time point (x，0) for any x ∈M.

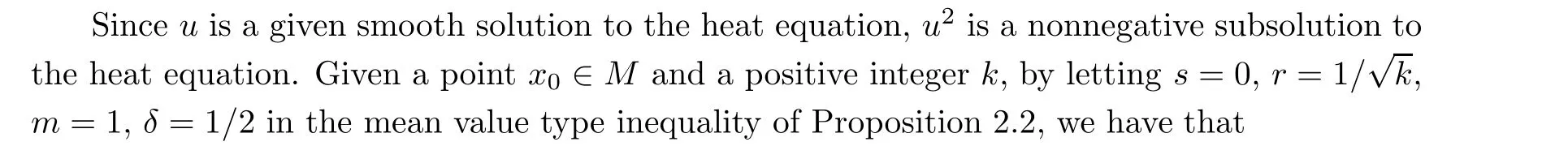
where both series converge uniformly for (x，t) ∈Bp(R)×[-δ，0] for any fixed R ＞0. Since u(x，t) is a solution of the heat equation， this implies that

where A3is a positive constant depending only on n and A2. □

In the end， we apply Theorem 1.1 to prove Corollary 1.3.

Proof of Corollary 1.3 Assume that u(x，t)is a smooth solution to the Cauchy problem of the backward heat equation (1.5) with quadratic exponential growth. Then u(x，-t) is also a smooth solution of the heat equation with quadratic exponential growth. By Theorem 1.1，we have that

Acknowledgements The author sincerely thanks Professor Qi S. Zhang for helpful discussion about the work [3].