Two dimensional subsonic and subsonic-sonic spiral flows outside a porous body

Shangkun WENG(翁上昆)Zihao ZHANG(张子豪)*

School of Mathematics and Statistics，Wuhan Universitg，Wuhan 430072，China E-mail: skweng@whu.edu.cn; zhangzihao@whu.edu.cn

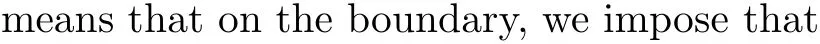
Remark 1.5 The decay rate |∇(ψ-ψ0)| = O(|x|-2) is optimal as was observed by the results in [3]， but here we remove the small perturbation conditions in [3]. We employe the Kevin’s transformation and some elliptic estimates to derive it.

Remark 1.6 We prescribe the boundary condition(1.16)on the boundary Γ，not the one that ρu·→n=(ρb(r)U1(r)er+ρb(r)U2(r)eθ)·→n. Otherwise， the solution will be the background radially symmetric solution. One may pose ρu·→n=∇⊥Ψ·→n on Γ for any given smooth function Ψ; our method still works in this case.

The research on compressible inviscid flows has a long history， and has provided many significant and challenging problems. The flow past a body， through a nozzle， and past a wall are typical flows patterns， which have physical significances and physical effects. The first theoretical result on the problem for irrotational flows past a body was obtained by Frankl and Keldysh in [14]. Important progress for two dimensional subsonic irrotational flows past a smooth body with a small free stream Mach number was made by Shiffman[21]. Later on，Bers[1] proved the existence of two dimensional subsonic irrotational flows around a profile with a sharp trailing edge， and also showed that the maximum of Mach numbers approaches one as the free stream Mach number approaches the critical value. The uniqueness and asymptotic behavior of subsonic irrotational plane flows were studied by Finn and Gilbarg in [12]. The well-posedness theory for two-dimensional subsonic flows past a wall or a symmetric body was established by Chen， Du， Xie and Xin in [2]. For the three-(or higher-) dimensional cases， the existence and uniqueness of three dimensional subsonic irrotational flows around a smooth body were proved by Finn and Gilbarg in [13]. Dong and Ou [9] extended the results of Shiffman to higher dimensions by the direct method of the calculus of variations and the standard Hilbert space method. The respective incompressible case is considered in [20]. For the subsonic flow problem in nozzles， one may refere to [8， 10， 11， 16， 19， 22， 25， 26].

On the other hand， the subsonic-sonic limit solution can be constructed by the compactness method. The first compactness framework on sonic-subsonic irrotational flows in two dimension was introduced by [4] and [25] independently. In [4]， Chen， Dafermos， Slemrod and Wang introduced a general compactness framework and proved the existence of two-dimensional subsonic-sonic irrotational flows. Xie and Xin [25] established the subsonic-sonic limit of the two-dimensional irrotational flows through infinitely long nozzles. Later on， they extended the result to the three-dimensional axisymmetric flow through an axisymmetric nozzle in[26]. Furthermore， Huang， Wang and Wang [18] established a compactness framework for the general multidimensional irrotational case. Chen， Huang and Wang [6] established the compactness framework for nonhomentropic and rotational flows and proved the existence of multidimensional subsonic-sonic full Euler flows through infinitely long nozzles. Recently，by the compactness framework in [6]， the existence of subsonic-sonic flows with general conservative forces in an exterior domain was established by Gu and Wang in [17].

The rest of this article is organized as follows: in Section 2， we introduce the subsonic truncation to reformulate the problem into a second-order uniformly elliptic equation，and then establish the existence and uniqueness of the modified spiral flow by a variational method. Then we remove the truncation and complete the proof of Theorems 1.1 and 1.3. In Section 3， the compensated compactness framework for steady irrotational flows is employed to establish the existence of weak subsonic-sonic spiral flows.

2 Subsonic Spiral Flows

This section is mainly devoted to the proof of Theorems 1.1 and 1.3. The proof can be divided into 5 subsections.

2.1 Subsonic truncation

By direct calculation， it is easy to see that the derivative of function H(s) goes to infinity as s →1. To control the ellipticity and avoid the singularity of H′， one may truncate H as

2.2 Existence of a weak solution to problem (2.2)

2.3 Regularity of weak solution

In this section， we verify that the weak solution obtained from the variation in subsection 2.2 is a classical solution.

Lemma 2.3 Suppose that ψ ∈H1loc(Ω) is a weak solution to problem (2.2). Then there is a constant α ∈(0，1) such that， for any subregion Ω1⋐(Γ ∪Ω)， it holds that

2.4 Modified spiral flows

2.5 Removal of the truncation

In this subsection， we remove the truncation and complete the proof of Theorems 1.1 and 1.3. We only need to prove Theorem 1.1， as the proof of Theorem 1.3 is similar.

3 Subsonic-Sonic Spiral Flows