Accurate analysis of limiting human dose of non-lethal laser weapons

Chen-yang Lyu, Ren-jun Zhan

College of Equipment Management and Support, Chinese People’s Armed Police Force Engineering University, No.1, Armed Police Road, Sanqiao, Weiyang District, Xi’an, 710086, Shaanxi, China

Keywords:Laser weapon Non-lethal Limit dose Tissue optics Bioheat transfer Finite element

ABSTRACT In order to maximize the lethality and reversibility of the non-lethal laser weapons (NLLW) at the same time and thus provide a theoretical basis for the R&D of laser weapons in the future,this paper accurately analyzed the limiting biological dose of irreversible damage to human skin caused by the NLLW. Firstly,based on the burn theory in medicine and the actual tactical background, this paper redefines the evaluation criteria of the limiting laser dose of NLLW to the human body. Secondly, on the basis of anatomical knowledge,a 5-layer finite element model (FEM)of superficial skin is proposed,constructed and verified, which can accurately describe the limiting reversible damage. Based on the optimized Pennes bioheat transfer equation, the diffusion approximation theory, the modified Beer-Lambert law,the Arrhenius equation, and combined with dynamic thermophysical parameters, this paper highly restored the temperature distribution and accurately solved the necrotic tissue distribution inside the human skin irradiated by 1064 nm laser. Finally, it is concluded that the maximum human dose of the 1064 nm NLLW is 8.93 J/cm2, 8.29J/cm2, and 8.17 J/cm2 when the light spots are 5 mm, 10 mm and 15 mm, respectively, and the corresponding output power of the weapon is 46.74 W,173.72 W and 384.77 W.Simultaneously,the temperature and damage distribution in the tissue at the time of ultimate damage are discussed from the axial and radial dimensions, respectively. The conclusions and analysis methods proposed in this paper are of great guiding significance for future research in military,medical and many other related fields.

1. Introduction

The NLLW in the directed energy weapon family has been booming in recent years with its unique performance [1,2]. It mainly uses the photothermal effect of laser on human skin to achieve intense thermal pain stimulation to achieve tactical purposes such as sniping, denial, or dispersal without causing permanent or irreversible damage [3]. Accurately analyzing the limiting laser biological dose that human skin can withstand can consider both lethality and reversibility to the maximum extent[4],which is the biggest focus and difficulty in the R&D of this kind of weapon. The strong scattering effect of 1064 nm laser makes it possible to maximize the activation of deep pain receptors while causing minimal damage to the surface of the skin [5], so it becomes the best choice for NLLW.In the mid-1970s,the US Air Force took the lead in studying the human dose of 1064 nm laser [6],Since then, many scholars have successively explored the interaction mechanism of 1064 nm laser-skin tissue [7-11] and damage threshold [5,12-15]. For example, the Chinese national standard GB7247.1-2012 gives a quantitative formula for the safe dose of different laser wavelengths at different irradiation time [16]. But the purpose of such standards is usually to ensure maximum safety in use, which is completely opposite to the purpose of weapon design, so the results are far from meeting the specific requirements of NLLW.Judging from the current research situation of this problem,there are mainly the following deficiencies:First,the determination of irreversible damage depth based on the outermost skin will bring greater errors to the results. Second, the current research is usually based on the assumption that the irradiation time of NLLW is 1 s, but it can not reflect the actual situation in tactical applications accurately. Third, constant photothermal parameters are usually used to describe the light distribution and heat transfer in the tissue, but the influence of larger temperature gradient under laser irradiation on the parameters should not be ignored. In general, there is currently no relevant research and clear standards that can directly guide the design of NLLW weapons, which has also become the biggest bottleneck problem that restricts its R&D.

2. Theoretical analysis

The dynamic photothermal model shown in Fig.1 describes the overall research process and the corresponding governing equations.

2.1. Limiting necrosis depth

In burn medicine theory,the thermal damage of skin is divided into three grades and four degrees. The three grades and four degrees theory can define the limiting damage depth of human skin caused by laser more accurately [17]. In this theory, I degree and superficial II degree burns are called general superficial burns;deep II degree and III degree burns are called deep burns.I degree burns are injuries to the superficial epidermis,showing local redness and swelling, slight pain, and burning sensation, which is far from meeting the lethality requirements of weapons. However, deep burns will damage below the dermal papillary layer and cause scars and local dysfunction after healing, so they do not meet the reversibility requirements.Superficial II degree burns to the dermal papillary layer are accompanied by severe pain, resulting in local redness,swelling,and blisters,but the skin can heal itself in about two weeks. The function of healed skin is perfect without scar or dysfunction, which is very consistent with the expected effect of NLLW.In human skin,the lower dermal papilla layer and the upper reticular layer are interlaced without obvious boundaries. So the critical depth of irreversible damage is redefined as the interlaced part of the upper portion of the reticular layer,which is located at a depth of about 220 μm under the skin surface. Therefore, NLLW should try to cause irreversible damage to the tissue above the critical depth to produce the strongest thermal pain stimulation effect, and the necrotic tissue will recover completely with the organism’s metabolism. Meanwhile, it is necessary to ensure the reversibility of tissue injury below the critical depth to avoid scarring or dysfunction. Therefore, on the whole, the optimal and critical biological dose of NLLW should exactly cause irreversible damage at 220 μm subcutaneously.

2.2. Limiting irradiation time

NLLW can cause intense pain like hot burning or acupuncture,so it can be considered that the maximum action time in the natural state is the time from the beginning of exposure to avoidance.This process is divided into two stages.The first stage is the time for the tissue temperature to increase to stimulate nociceptors, and the second stage is the time for avoidance due to pain. At the same time, it is considered that the skin has moved relative to the light spot at the moment of dodging,so there is no need to consider the time of dodging movement. The production of the first stage depends on the Aδ fibre and C fibre in skin tissue. The minimum temperature of thermal stimulation of the pain-related VR1 membrane protein on the peripheral cell membrane is about 316 K[18].Based on ignoring the conduction time of nerve impulses,the processing time of the first stage is 50 ms[19].The second stage of response is a complex process, which is mainly divided into three types: the first is processed by the cerebral cortex and consciousness, which usually requires more than 200 ms; the second is processed through the cerebral cortex but not consciously, usually about 100 ms;and the spinal cord completes the third without the brain, the time is about 50 ms. Pain is produced by the cerebral cortex but does not need to be processed by consciousness, so the second stage is about 100 ms. In general, the maximum laser irradiation time under the tactical background of NLLW is 150 ms.

2.3. Limiting necrosis rate

The Arrhenius equation is the most widely used method for judging the reversibility of tissue damage [20], and its expression is:

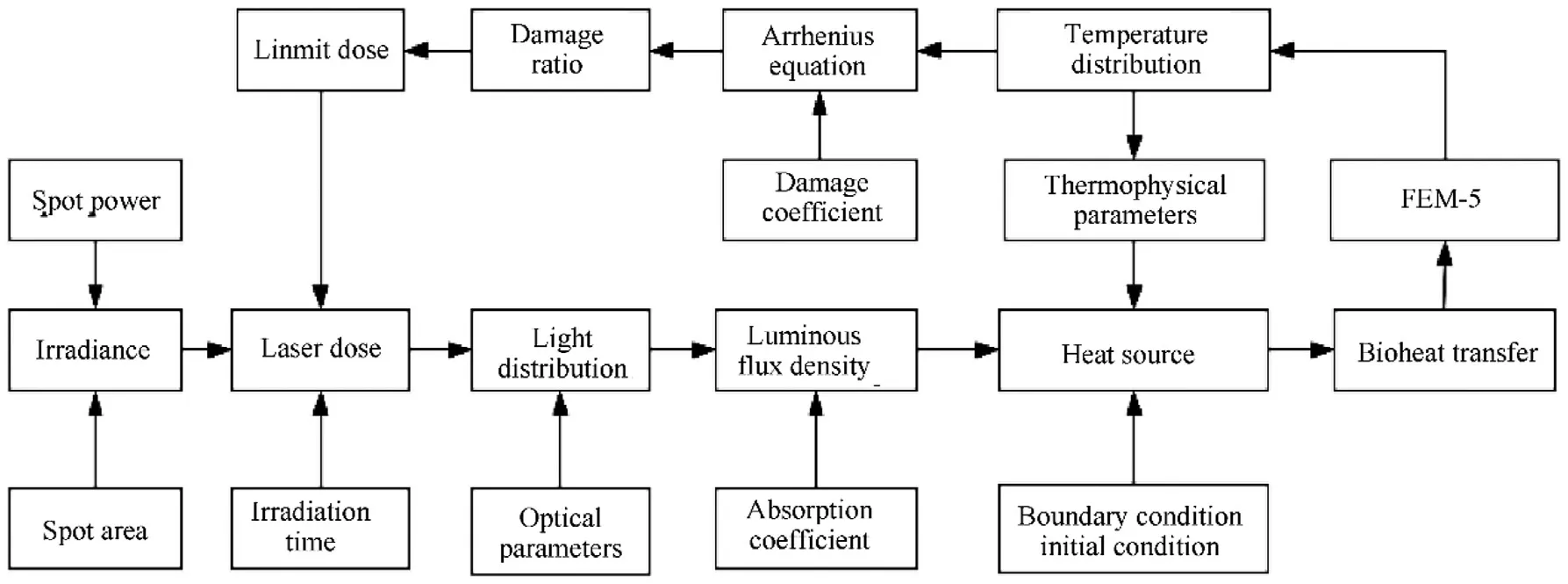
Fig.1. Dynamic photothermal model for laser-skin.

Where T(K)is the absolute tissue temperature,k=1.38×10J/K is Boltzmann’s constant,h=6.63×10is Planck’s constant,ΔS(J/K) is the increase in tissue entropy after damage. Henriques obtained the Arrhenius coefficients of the skin through experiments as [21]: A = 3.1 × 1098 s, E = 6.28 × 105 J/mol. Therefore, the thermal damage in the tissue can be described intuitively by the proportion of necrotic tissue θ, and the expression is as follows:

Ω = 1 is the critical point for judging thermal damage,Ω ＜1 is reversible, otherwise irreversible damage. Therefore, it can be concluded that the maximum value of θ at any point in the tissue is 1-1/e ≈0.63212,that is the critical tissue necrosis rate C.

3. Numerical simulation

3.1. Geometric model

In the research of skin FEM,Bromm et al.[22]first assumed it as a one-layer homogenization model in 1983, but there was a poor correlation between the calculated results and the experimental results. With the deepening of research, 2-layer, 3-layer, 5-layer,and 7-layer FEM have emerged one after another [23-26]. But in general,there is currently no FEM that can accurately describe the reversibility of tissue damage within the critical depth defined by section 2.1.Therefore,this paper proposes,constructs,and verifies a 5-layer FEM specifically for superficial skin based on anatomy to accurately analyze skin temperature and damage distribution within this depth range.

From the perspective of the anatomical structure of skin tissue,the stratum corneum is quite different from other layers and has reflections [27], so divided it independently. The papillary dermis and reticular layer are staggered with a large number of capillary plexuses densely interspersed, so the effect of capillary perfusion on heat transfer cannot be ignored.Based on these considerations,the skin is finally divided into the stratum corneum 10 μm, living epidermis layer 80 μm, papillary dermis layer 100 μm, capillary layer 20 μm,and the upper reticular layer 10 μm.In order to better observe the temperature and damage distribution of the entire skin,increase the thickness of the reticular layer to 610 μm,and add a critical calculation point C(0,600 μm)at the critical depth in FEM.It should be noted that point C is just a computational point in the geometric structure of the FEM. To further distinguish the critical values of different physical quantities, C, C, and Care used to represent the critical power,critical temperature,and critical tissue necrosis rate. Based on COMSOL Multiphysics 5.5 platform, establish a rectangle with a height of 820 μm in a two-dimensional axisymmetric space, reasonably adjust the width of the rectangle according to the size change of the light spot. When mashing the model, reasonably adjust the density of the mesh according to the thickness of each layer.The overall model and details are shown in Fig. 2.

3.2. Heat source analysis

The accurate description of the light distribution in tissue is the basis for accurate analysis of the temperature field distribution.Due to the differences between skin layers and the effects of blood perfusion rate, this paper used the Pennes biological heat transfer equation optimized by the blood perfusion term to describe the heat transfer process in tissue accurately. In addition, considering the optical and thermophysical properties difference of each skin layer and value varies with temperature, the dynamic parameters are used to solve the Pennes equation[28].Ignoring the influence of tissue metabolism under laser irradiation[29],the equation form in cylindrical coordinates is:

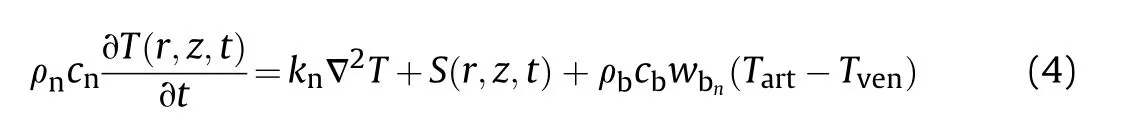
Where S(r,z,t),n,r,z,t,ρ,k,c,ware the heat source term,skin layer,distance from irradiation centre, subcutaneous depth, calculation time, tissue density, thermal conductivity, specific heat capacity,and blood perfusion rate, respectively. Tis the temperature of arterial blood and always 37C, Tis the venous blood temperature,which can be approximated to the tissue temperature T(r,z,t).

The reasonable description of light distribution in tissue is the basis of accurate calculation. This paper used the diffusion approximation theory based on the modified Beer-Lambert law(MBLL)to solve the radiative transport equation[30].This method has long been used to describe the propagation of highly scattered light in tissue[31,32],and it is still being perfected and used by now[33], and a large number of experiments have proved its accuracy.According to the beam broaden theory [31], although both absorption and scattering can make the light energy exponentially attenuate in the tissue, the scattering can only broaden the beam without the contribution of heat,and S(r,z,t)mainly comes from the heat deposition caused by the absorption of photons by the tissue.The energy within the section of the spot is Gaussian [34], so the spot radius ωat depth z can be expressed as:

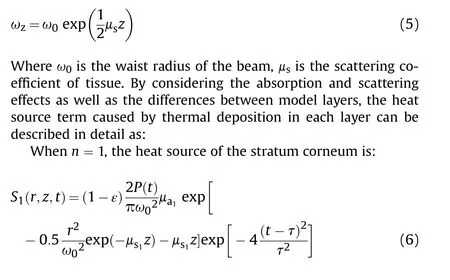
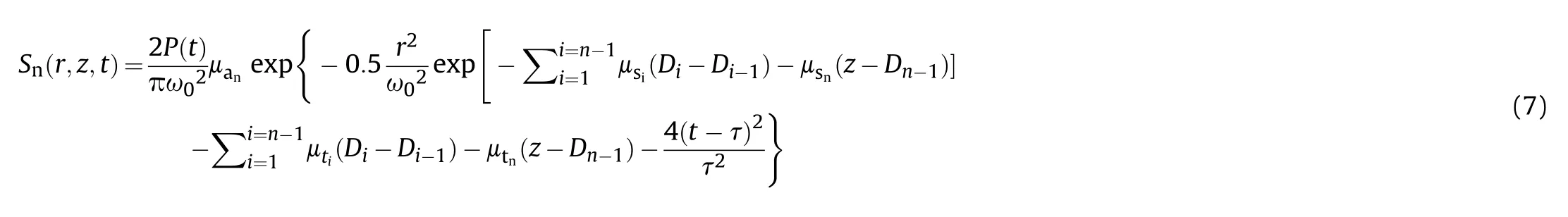
Fig. 2. 5-layer FEM of superficial skin.

When n ＞1, the heat source terms of other layers are:

Where P(t),μ,ε,and Dare the laser output power,the absorption coefficient in the skin, the surface reflectivity, and the ordinate of the end boundary of each layer, respectively.μ=（μ+μ） is the loss coefficient of light in each layer of skin, μis the reduced scattering coefficient of each layer of the skin. It combines μand anisotropy factor g to describe the scattering of photons in a random step length, so it converts the anisotropic scattering into isotropic scattering to calculate. Thus, it is suitable for the highly scattered light in the therapeutic window (λ = 600-1200 nm) of optical albedo much greater than 0.5[30].So this paper uses theμof each skin layer to calculate the effect of scattering on the heat source term,and its expression is:

3.3. Initial and boundary conditions

Arteries in the dermis are the main source of heat in skin tissue,and it transfers to each layer in the form of heat exchange, so the temperature of the dermis is slightly higher than that of the epidermis. Therefore, set the initial temperature of each layer as:

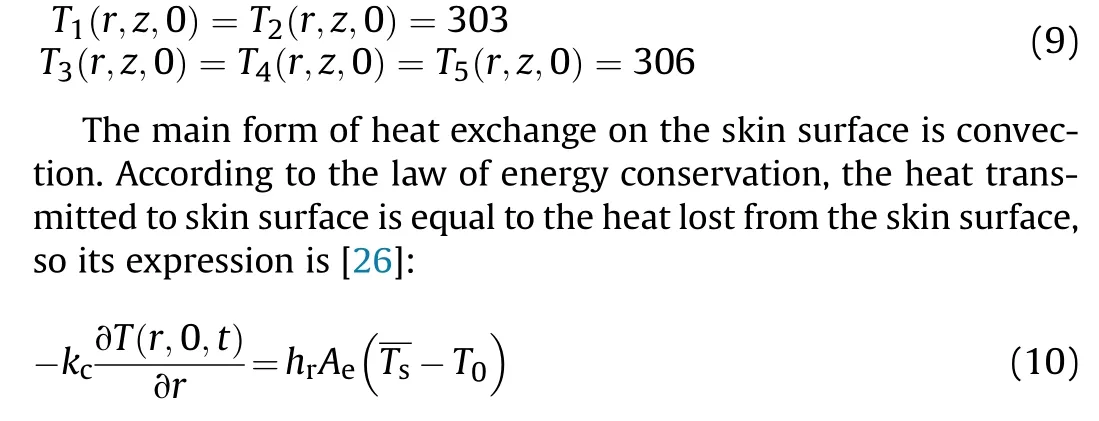
where kis thermal conductivity, Tis the ambient temperature,and his the heat exchange coefficient. hcan be taken as 51(W/m·K)under short-time laser irradiation.Ais the effective cooling area of convective heat transfer, which is the total area of skin exposed to the air.Tis the average temperature of skin surface T(r,z,0). Considering that the tissue boundary (r=R) no longer occurs heat exchange, so set it the adiabatic condition, and the temperature value is the same as the initial temperature of each layer,that is:

3.4. Photothermal parameters

3.4.1. Optical parameters

According to the research of Bolin et al.[35],the μof each skin layer can be defined as the average absorption of blood, melanin,and water, that is:

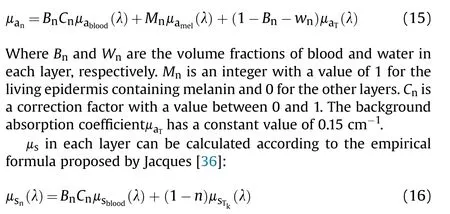
Whereμis the μof laser with different wavelengths in each skin layer without blood, and its value decreases with the increase of wavelength.The drop is very rapid within 950 nm,and very slowly when it exceeds 950 nm, so it can be expressed as [37]:

3.4.2. Dynamic thermophysical parameters

Thermophysical parameters of the skin mainly include tissue density ρ, specific heat capacity c, and thermal conductivity k,which vary according to the changes in the water content wof the skin at different layers. The huge temperature gradient caused by the laser will significantly impact these parameters, and the study of Jacques et al. [38] had also proved that the result of using static thermophysical parameters is often higher than that of using dynamic parameters. Therefore, this paper uses dynamic thermophysical parameters to describe the heat transfer process more accurately, and the expressions are:

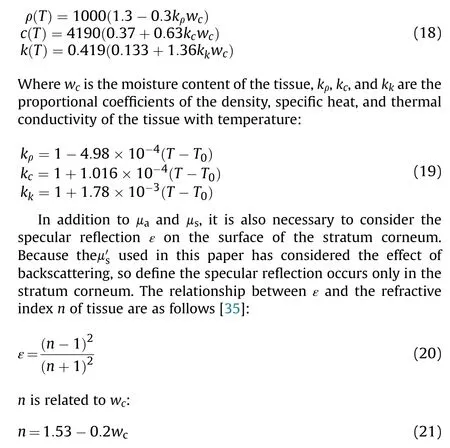
wof the stratum corneum is 15%, and the specular reflectance of the skin surface can be calculated to be 4%according to Eq.(20)and(21).Finally,based on the above analysis and related literature,the optical parameters [39-46]and thermophysical parameters[37,47,48] of 1064 nm laser in superficial 5-layer skin FEM are summarized in Table 1.

3.5. Model verification

Due to the weapon-grade human experiment violates the experimental ethics, this paper uses an indirect method to verify the constructed FEM. In 2017, Wang et al. [27] made a detailed experimental and numerical study on the thermal pain stimulation of skin caused by the laser, so their research data were used to verify the accuracy of the FEM in this paper. Keep the FEM parameter setting consistent with Wang’s experiment, that is, the spot power and radius are 110 W and 2.5 mm, respectively. The experiment time is 30 s,and the laser begins to irradiate at 10 s for 40 ms. Figs. 3-5 compares the experimental&simulation results of Wang and the simulation results of this paper.Fig.3(a)(b)compare the tissue temperature changes in depth at the end of the irradiation, and (c) (d) compare the temperature changes within the radius of the spot.

Through the above comparative analysis of the curve, it can be seen that the constructed FEM can accurately describe the tissue temperature distribution in both axial and radial directions. Fig. 4(a) (b) makes a comparative analysis of tissue temperature changes with time during the entire experimental period, which are also in good agreement.

Through the above analysis on the depth,radius,and time scale,a comprehensive verification of the tissue temperature field is achieved, proving that the model can well describe the temporal and spatial changes of tissue temperature.In order to further verify the model, Fig. 5 (a) (b) and (c) (d) compare the numerical simulation results of the temperature and necrosis ratio distribution of the spot central section at the end of irradiation, respectively.

It can be clearly seen from Fig.5 that the two sets of simulation results are highly consistent both qualitatively and quantitatively.The difference between the post-processors of MATLAB and COMSOL causes a slight difference in figures, but it does not affect the consistency of calculation results. In general, the above comprehensive comparative analysis proves that the mathematical model and the FEM constructed in this paper can restore the real physical phenomena very well, so the analysis results based on this model will have high accuracy and reliability.

4. Result

The energy of Gaussian spot is exponentially distributed in its plane,and the spot radius is defined as the distance when the edge energy density decreases to 1/e of the centre. Therefore, the damage ability of NLLW is fundamentally affected by the coupling of spot power and spot size.The light spot of a laser weapon is difficult to be as small as in an operating room.On the one hand,it is limited by the focusing ability of the optical lens; on the other hand, it is affected by many external factors such as atmospheric refraction and turbulence during long-distance transmission. Therefore, thephysical limitation of most laser weapon spot diameters is above 1 cm,and a spot larger than 3 cm will not only significantly increase the demand for output power but also cause excessive damage to the human body,which is not in line with the original intention of NLLW. Therefore, in the research process, the three cases of spot radius R of 5 mm,10 mm,and 15 mm are considered,respectively,and the corresponding limiting spot power in each case is determined.

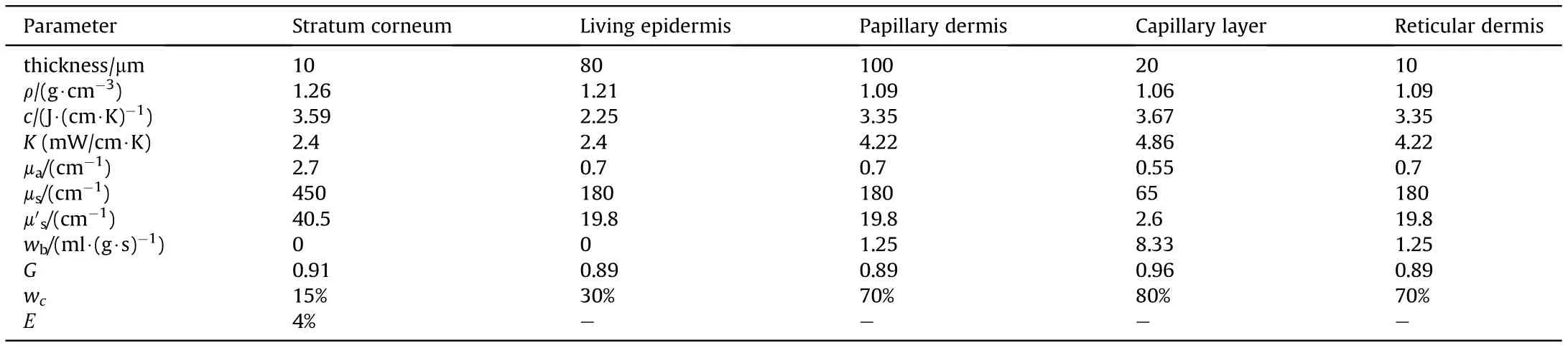
Table 1 Optical and physical parameters of 5-layer human skin (λ = 1064 nm).

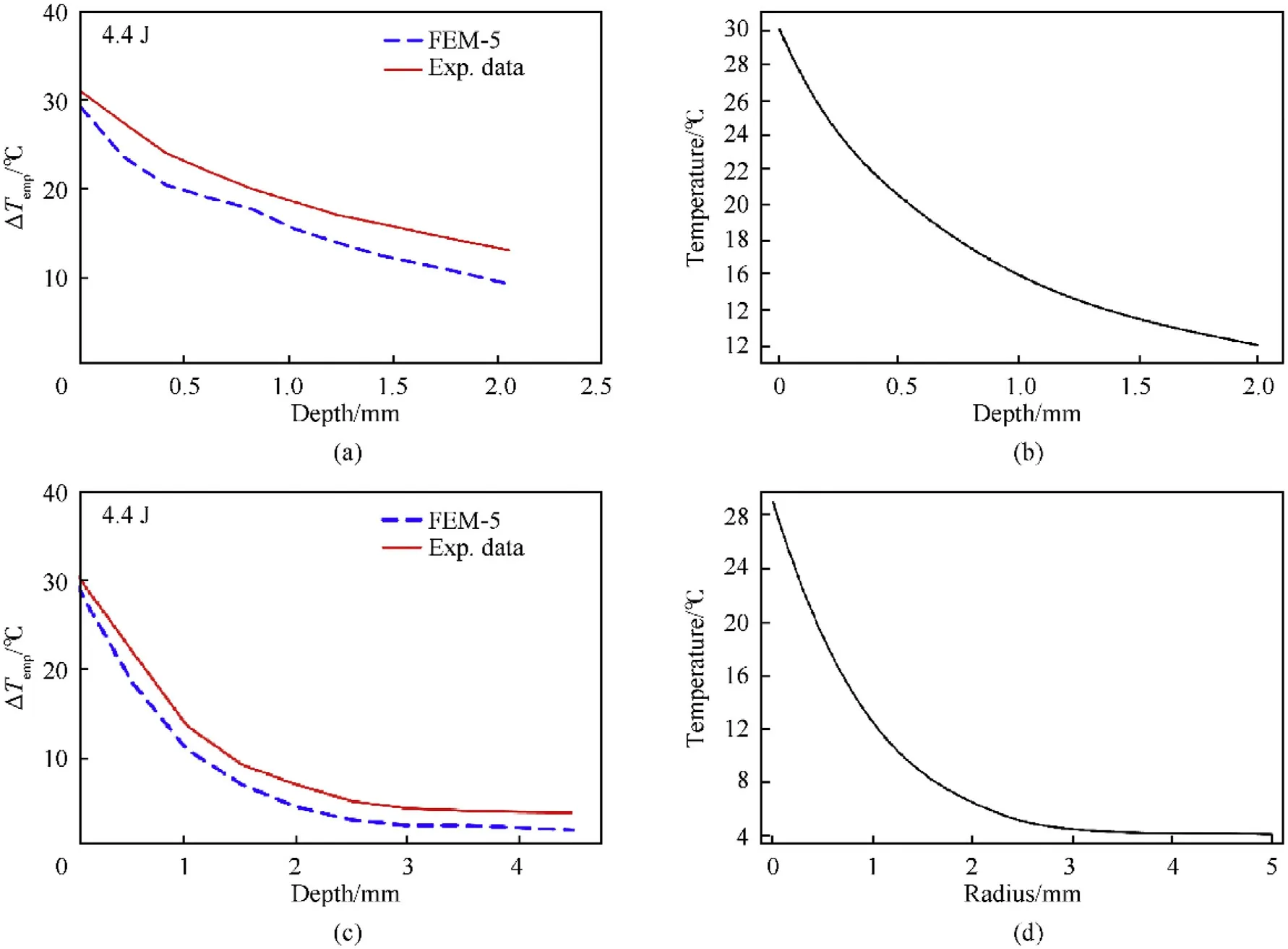
Fig. 3. Verification of axial and radial temperature distribution.

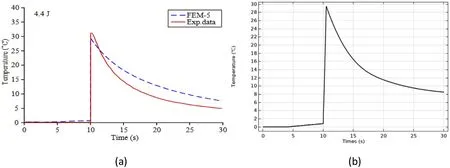
Fig. 4. Verification of temperature changes with time.

4.1. R = 5 mm

Set the irradiation time to be the human body reaction time and discretely select the spot power P within a certain range. Fit multiple sets of data composed of P and θ at the critical point C into a curve, and then the limiting power corresponding to θ = 0.63212 can be solved by analyzing the curve. The critical power Cis first limited to a smaller range by continuous differential calculation for ease of calculation, and here is 47-48 W. Then take a step size of 0.05 W in this small range, and fit a total of 20 sets of data to gets the variation of θ with P,as shown in Fig.6(a)and Figs.7-8.These figures only show a very small range near the Cto observe the precise value of P more accurately. It is evident that the Cof two significant digits when R=5 mm is 46.74 W,and the corresponding limiting laser dose is 8.93 J/cm. Fig. 6 (b) and (c) show the 3D temperature distribution and damage distribution in the tissue at this time, respectively.

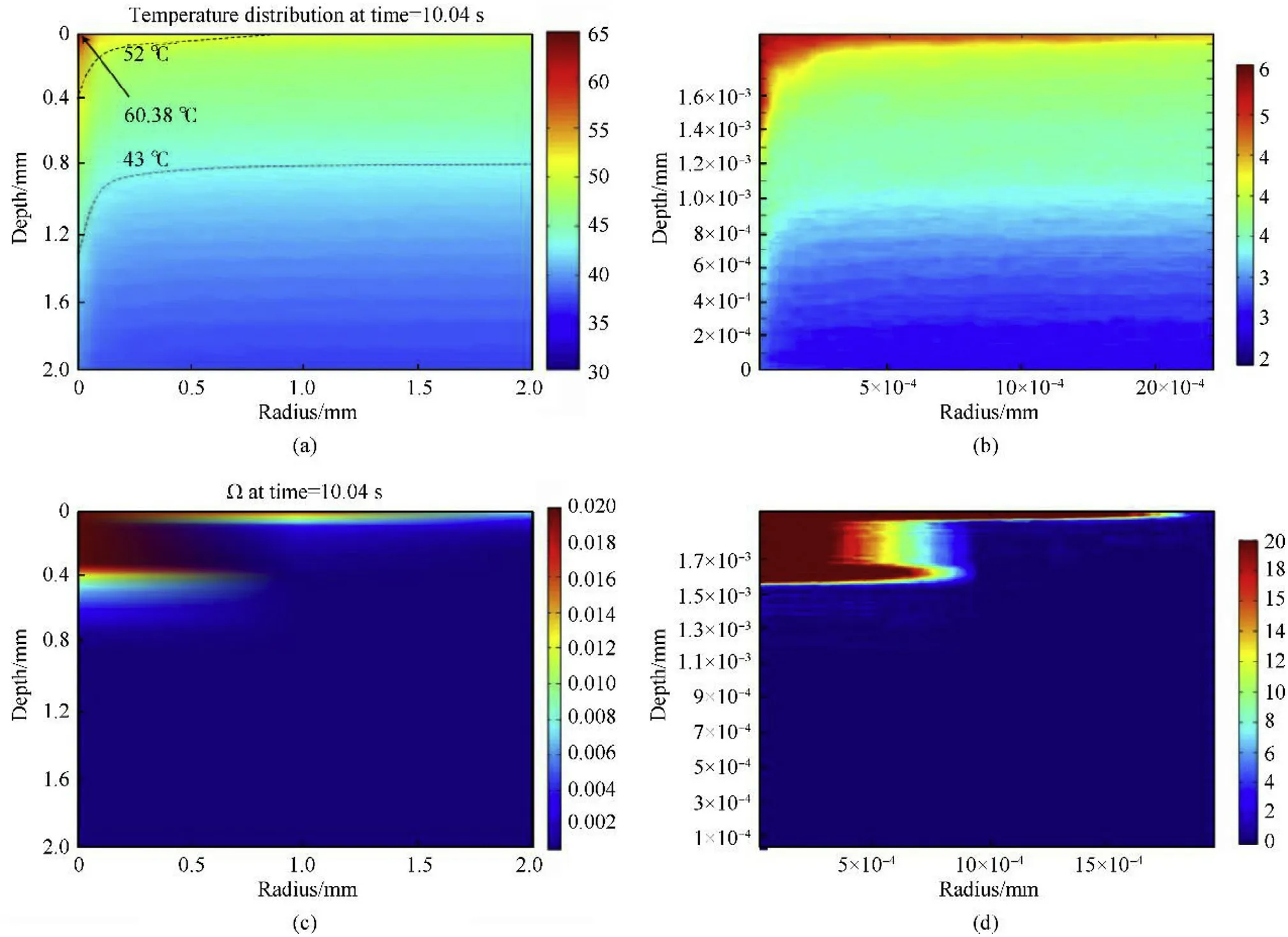
Fig. 5. Verification of FEM results for temperature and necrotic tissue distribution.

4.2. R = 10 mm

Based on the same method, it can be concluded that the Cof two significant digits is 173.7 2W when R = 10 mm, and the corresponding limiting laser dose is 8.29 J/cm. Fig. 7 shows the variation of θ with P in a very small range near the C.

4.3. R = 15 mm

Fig.8 shows the variation of θ with P in a very small range near the Cwhen R=15 mm.It can also obtain that the Cof R=15 mm is 384.77 W,and the corresponding limiting laser dose is 8.17 J/cm.

5. Discussion

The direct reason that the spot with different power and size can achieve the same damage effect is that the central axis has the same damage distribution, and the fundamental reason lies in the same temperature field distribution. Therefore, tissue temperature and damage distribution in the limiting conditions (t = 150 ms,P =173.72 W)are discussed from the axial and radial dimensions.

5.1. Temperature distribution

5.1.1. Axial distribution

Fig. 9 (a) and (b) show the 1D central axial temperature distribution of the whole model and the 2D temperature distribution on the axisymmetric plane in the limiting conditions for R = 10 mm.

Fig. 9 shows that the temperature decreases rapidly above the critical depth,and the downward trend slows down obviously after reaching the critical depth. Therefore, the temperature gradient above the critical depth is much larger than that below the critical depth. Moreover, different optical and thermophysical parameters between different layers have a certain influence on the axial temperature distribution. The maximum value of the temperature gradient appears in the living epidermis layer, and the minimum value appears in the reticular dermis layer. It can be seen that the critical temperature Cis 339.79 K.C＞316 K also proves that the Cis enough to cause intense thermal pain stimulation,which well meets the lethality requirements of NLLW. Similarly, the Cis 339.76 K and 339.78 K when R=5 mm and R=15 mm,respectively.The temperature difference between the upper and lower part of the irreversible damage is about 13-14 K when reaching the damage limitation. It can be known that the central axial temperature distribution is highly consistent under different spot sizes,which is the fundamental reason for the same damage effect in the axial direction.

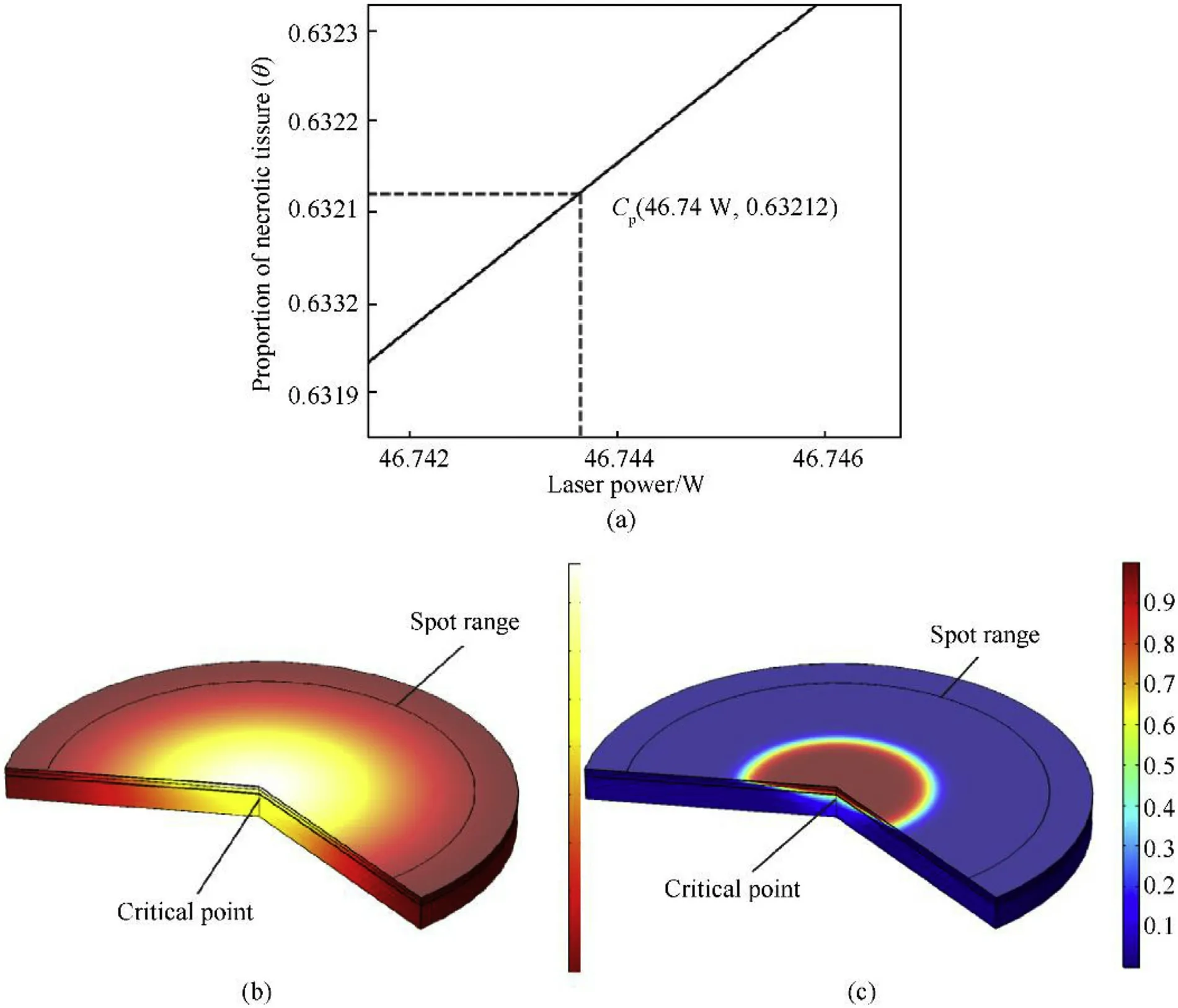
Fig. 6. Critical power, 3D temperature and damage distribution for R = 5 mm.

Fig. 7. Critical power for R = 10 mm.

5.1.2. Radial distribution

Fig. 10 shows the radial temperature distribution of the skin surface in the limiting conditions with R = 10 mm.

Fig. 8. Critical power for R = 15 mm.

Comparing Fig.10 with Fig.9,it is evident that the temperature gradient in the radial direction is much smaller than that in the axial direction and also shows a trend of increasing at first and then temperature difference caused by the uneven distribution of the Gaussian spot is between 41 and 42 K when reaching the limiting conditions.

Fig. 9. 1D and 2D axial temperature distribution.

Fig.10. Radial temperature distribution of skin surface.

5.2. Necrotic tissue distribution

5.2.1. Axial distribution

Fig.11(a)and(b)show the 1D central axial tissue necrosis rate distribution of the whole model and the 2D tissue necrosis rate distribution on the axisymmetric plane in the limiting conditions for R = 10 mm, respectively.

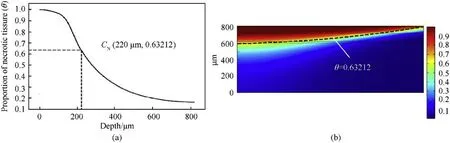
Fig.11. 1D and 2D axial tissue necrosis rate distribution.

Fig.11(a)shows that the proportion of necrotic tissue changing with depth also increases first and then slows down, which is consistent with the changing trend of temperature gradient. From section 5.1, the temperature of 339.79 K in the limiting conditions decreasing. It determines that the radial temperature change is more gentle than the axial direction, and the descending speed from the spot centre to the edge first increases and then decreases,which is consistent with the Gaussian distribution of the spot energy.The calculation shows that the spot edge temperature in three spot radius is 312.75 K,311.56 K,and 311.49 K,respectively,and the temperature difference between the spot edge and centre is 41.33 K, 41.66 K, and 41.53 K. It can be seen that the radial

will cause the maximum reversible damage in the reticular dermis layer, and tissue above critical tissue necrosis rate Cwill be irreversibly damaged due to excessive temperature. Partial condensation will occur when the tissue temperature is between 333 and 373 K [49], and the calculation in section 4 shows that the instantaneous temperature of the stratum corneum surface center is 354.08 K, 353.22 K, and 353.02 K. Therefore, the tissue above the critical depth will be necrotic due to condensation,but there will be no vaporization, carbonization, or even melting. This is highly consistent with the description of shallow II degree in burn theory.

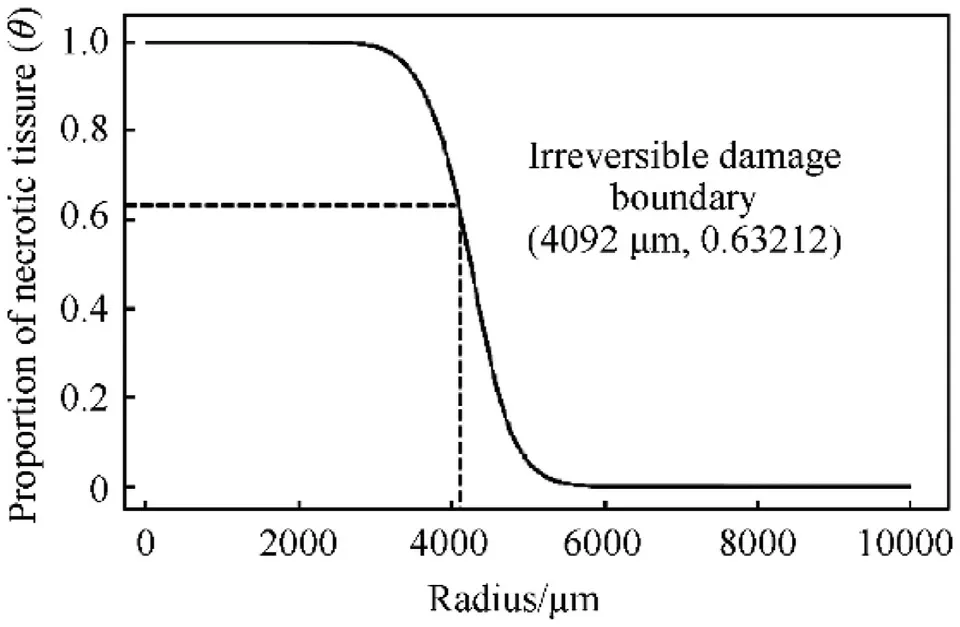
Fig.12. Radial tissue necrosis rate distribution of skin surface.

5.2.2. Radial distribution

Fig.12 shows the radial tissue necrosis rate distribution on the stratum corneum surface in the limiting conditions when R = 10 mm.

In radial direction, θ rapidly decreases from 1 to 0 within the range of about 2 mm,and the range of 1 to Cis less than 1 mm.It indicates that the damage profile caused by light spot is obvious,which is consistent with the 3D damage distribution in Fig.6(c).θ at the spot edge is only about 10,no longer has attack effect.After further calculation, the radial damage boundary from z-axis of three radii are 2.42 mm, 4.09 mm, and 6.06 mm, respectively. The ratio of necrotic radius to spot radius is 0.484, 0.409, and 0.404.Therefore,it can be concluded that the necrotic tissue area accounts for only 16-23% of the entire spot area and shows a decreasing trend with the light spot increases.

6. Conclusion

This paper creatively combines the burn theory and anatomy knowledge in medicine with the actual tactical background.On the one hand,it redefined the evaluation criteria of limiting laser dose to human skin for NLLW. On the other hand, it proposed, constructed, and verified a 5-layer FEM of superficial skin, which can restore the reality well and describe the temperature and necrotic tissue distribution accurately. Based on the optimized Pennes bioheat transfer equation, the diffusion approximation theory, the modified Beer-Lambert law,the Arrhenius equation,and combined with dynamic thermophysical parameters, the limiting human dose of NLLW was obtained as 8.93 J/cm,8.29J/cm,and 8.17J/cmwhen the light spots are 5 mm,10 mm, and 15 mm, respectively,and the corresponding output power of the weapon is 46.74 W,173.72 W, and 384.77 W. In general, the limiting human dose of NLLW between 8 and 9 J/cmcan maximize both lethality and reversibility,and the laser dose should be slightly reduced with the spot radius increasing. The conclusions and analysis methods proposed in this paper are of great guiding significance for future research in military, medical, and many other related fields.

The authors declare that there is no Conflict of Interest in this paper.