Local Unstable Entropy and Local Unstable Pressure for Partially Hyperbolic Endomorphisms∗

Xinsheng WANG

1School of Mathematical Sciences,Xiamen University,Xiamen 361005,China;School of Mathematical Sciences,Hebei Normal University,Shijiazhuang 050024,China.E-mail:xswang@xmu.edu.cn

Abstract In this paper,local unstable metric entropy,local unstable topological entropy and local unstable pressure for partially hyperbolic endomorphisms are introduced and investigated.Specially,two variational principles concerning relationships among the above mentioned numbers are formulated.

Keywords Partially hyperbolic endomorphism,Local unstable metric entropy,Local unstable topological entropy,Local unstable pressure,Variational principle

1 Introduction

Entropy including metric entropy and topological entropy plays an important role in dynamical systems,which describes the complexity of a given dynamical system from different points of view.It is well known that there is a variational principle connecting metric entropy and topological entropy,which says that for a given system(X,T),whereXis a compact topological space andT:X→Xis a surjective and continuous map,its topological entropy is equal to the supremum of all metric entropies over all invariant probability measures with respect toT.As a generalization of entropy,pressure with respect to a potential function is introduced,and a similar variational principle can also be established.The reader can refer to[7]for more details concerning entropy theory.

In order to obtain more information from a dynamical system,various versions of entropy are introduced,among which local entropy including local metric entropy and local topological entropy is an important one.Correspondingly,related variational principle is formulated.In[14],given aT-invariant measureµ,for a given open cover U ofX,Romagnoli introduced two types of metric entropies with respect to U:hµ(T,U)andwithand gave the following local variational principle:

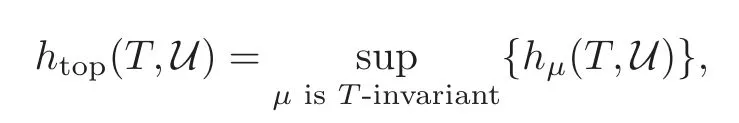
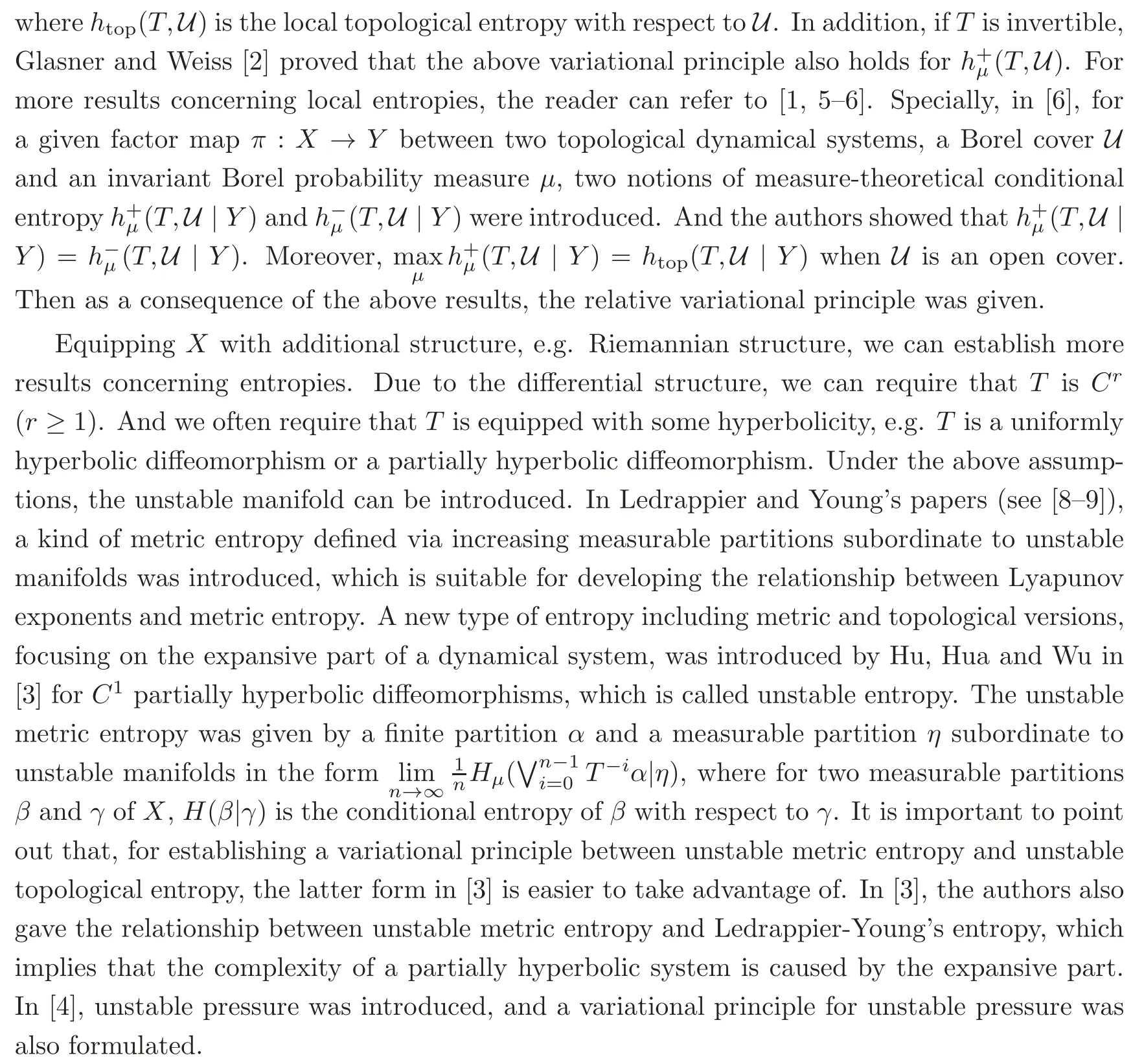
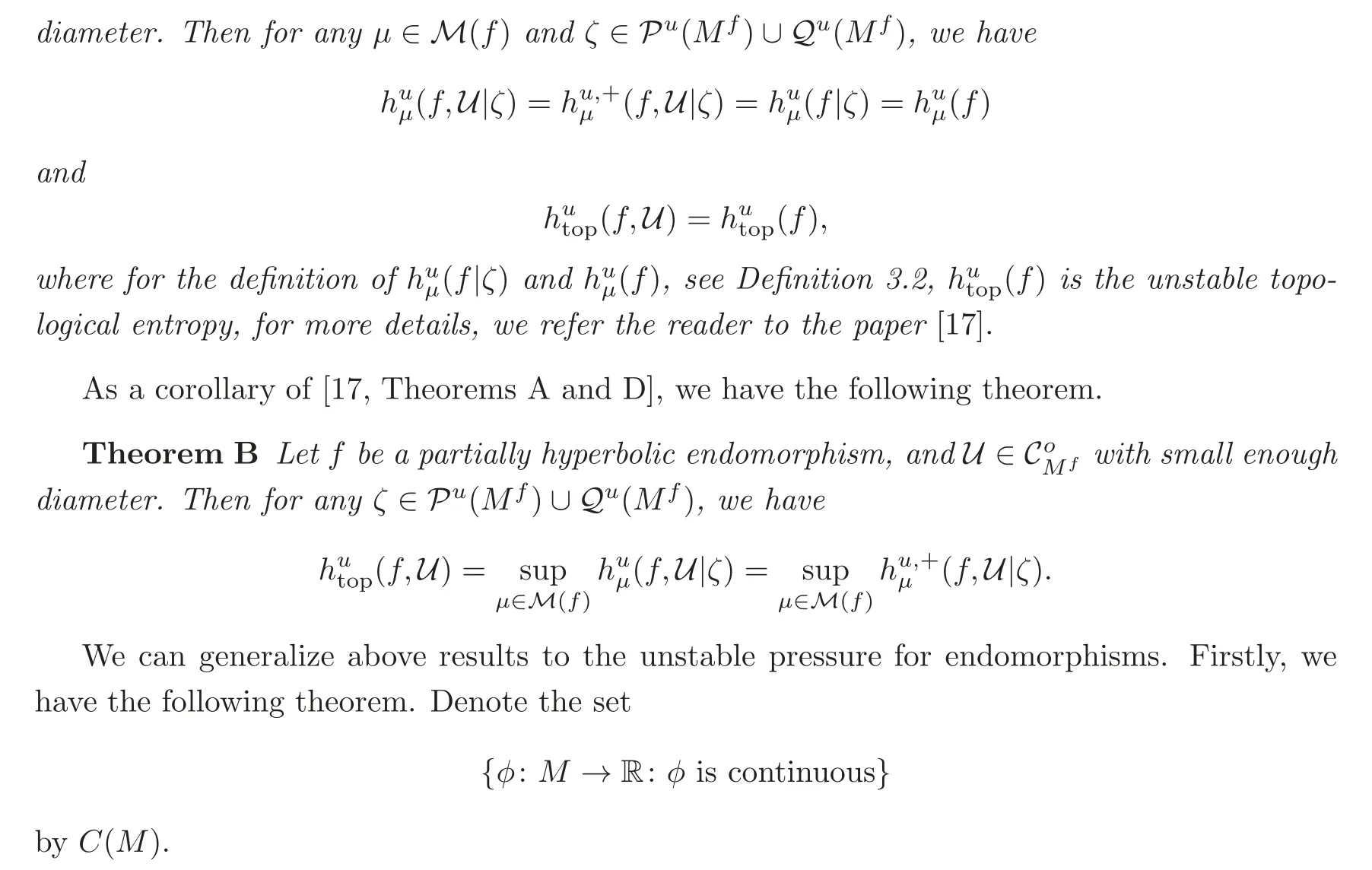
In[19],the concepts of unstable entropies and unstable pressure were generalized to local case for partially hyperbolic diffeomorphisms,which bring us a new point of view to investigate the complexity of a partially hyperbolic dynamical system.Variational principles for local unstable topological entropy and local unstable pressure were obtained respectively.Note that in order to give the above variational principles,unstable topological conditional entropy and unstable tail entropy were introduced,which play crucial roles in the proofs.

Noticing that plenty of physical processes are irreversible,in addition,the evolution law dependents on time,some counterparts of the above objects are considered for noninvertible map via preimage structure(see[20])and some of the above results are generalized to random case(see[16,18]).It is interesting to investigate corresponding results as in[3–4,19].In[17],the authors introduced unstable entropies and unstable pressure for partially hyperbolic endomorphisms,and obtained a corresponding variational principle.The main purpose of this paper is to introduce local unstable entropies and local unstable pressure for partially hyperbolic endomorphisms.However,for endomorphisms,there are some difficulties to establish similar results.Due to non-invertibility,the notion of unstable manifolds is not well defined,in order to overcome this difficulty,in[22],Zhu introduced the inverse limit space(see Section 2,for details),which makes it possible to define the unstable manifolds and borrow some ideas from the smooth ergodic theory of random dynamical systems.Moreover,some techniques and results in[17]can be applied.

This paper is organized as follows.In Section 2,we give some basic knowledge necessary for our goal and state our main results.In Section 3,we give the definitions of two kinds of local unstable metric entropies,and some properties of these two local entropies and relations between them are also obtained.In Section 4,we give the definition of local unstable topological entropy with some important properties of them.In Section 5,we give the definitions of unstable topological conditional entropy and unstable tail entropy and their relations with local unstable entropies and unstable entropies,which are crucial to the proofs of our variational principles.In Section 6,we give the proofs of the variational principles for both local entropies and local pressure.

2 Preliminaries and Main Results

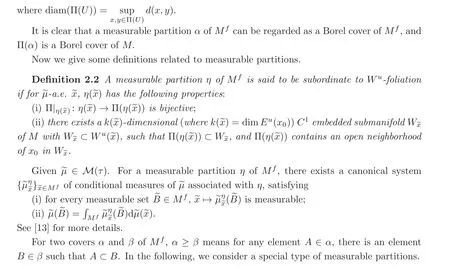
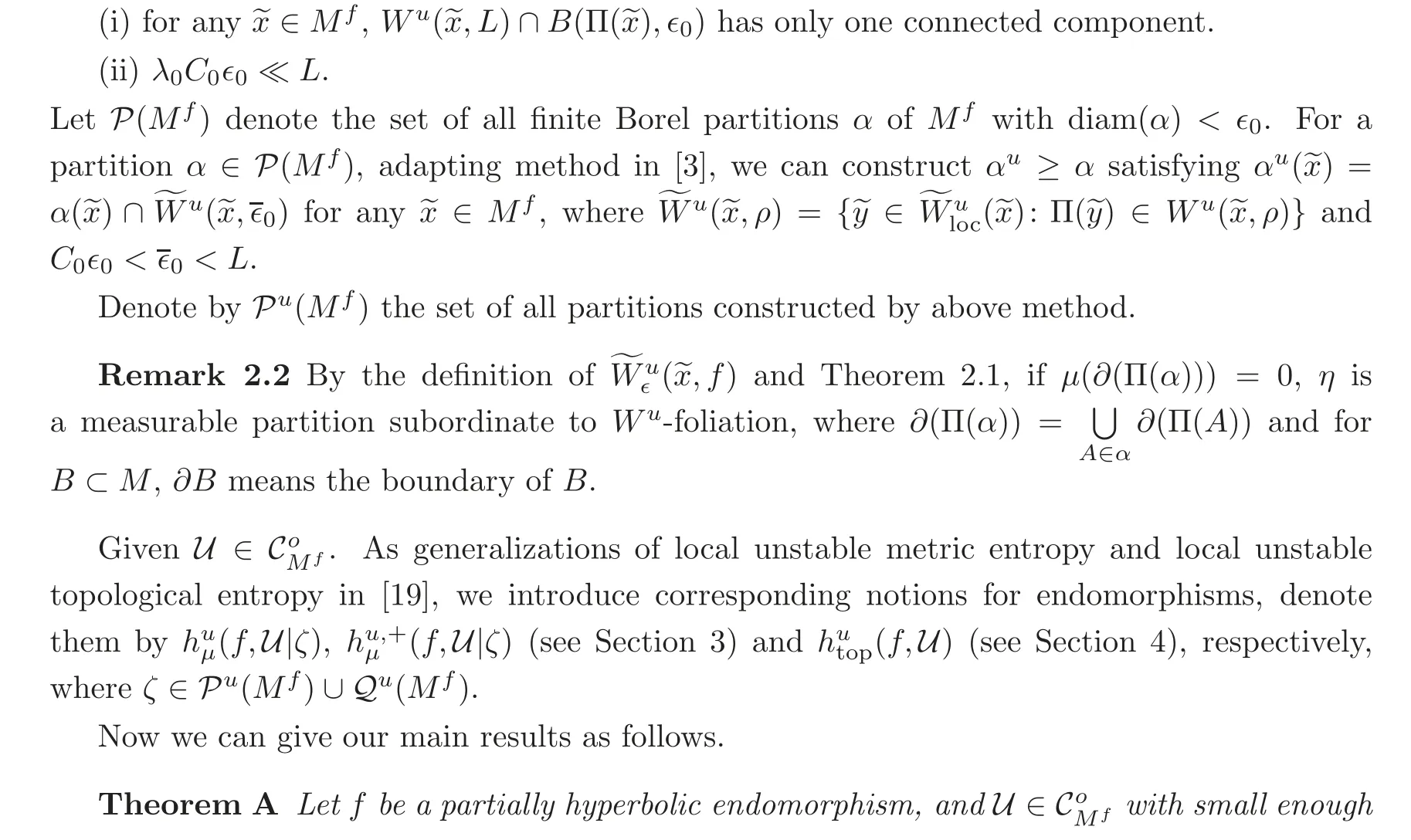
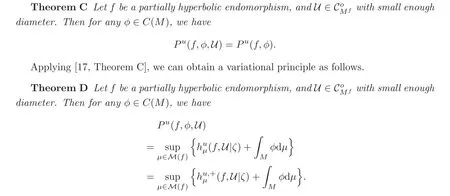
3 Local Unstable Metric Entropy

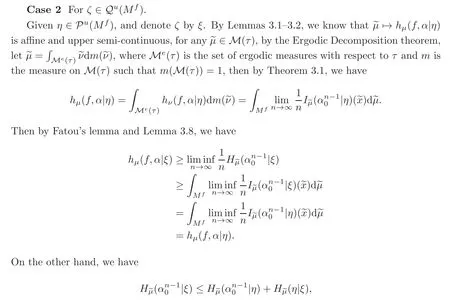
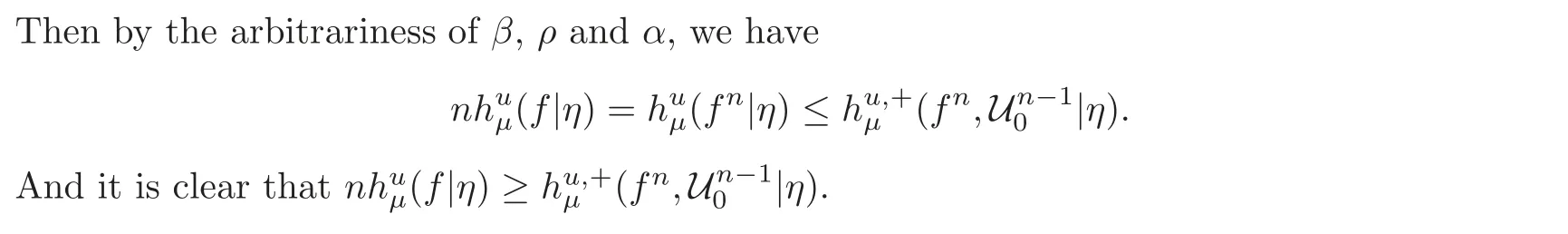
4 Local Unstable Topological Entropy and Pressure

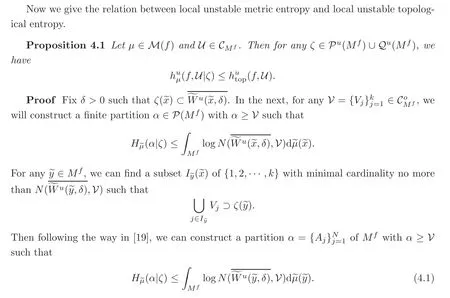
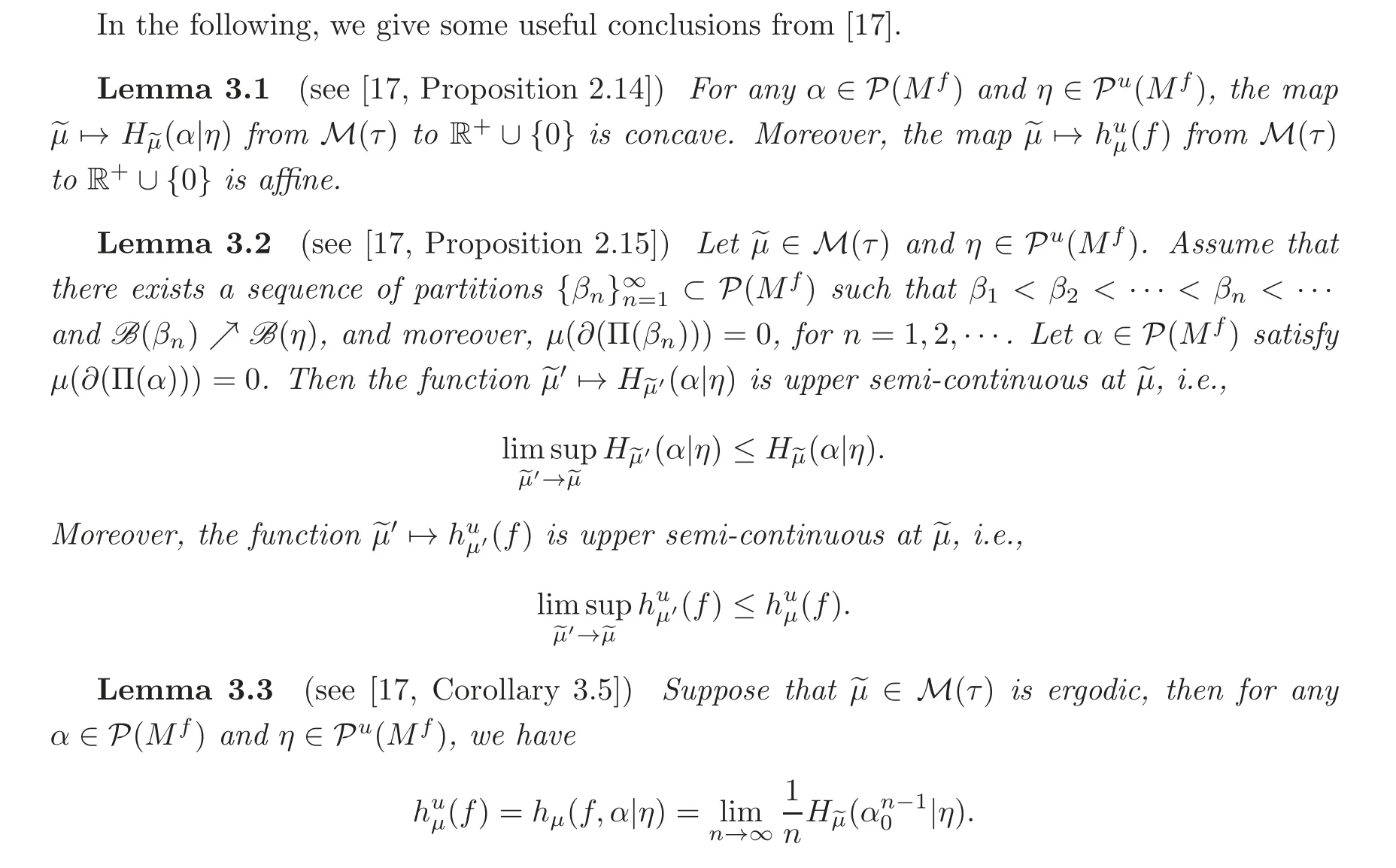
5 Unstable Topological Conditional Entropy and Unstable Tail Entropy

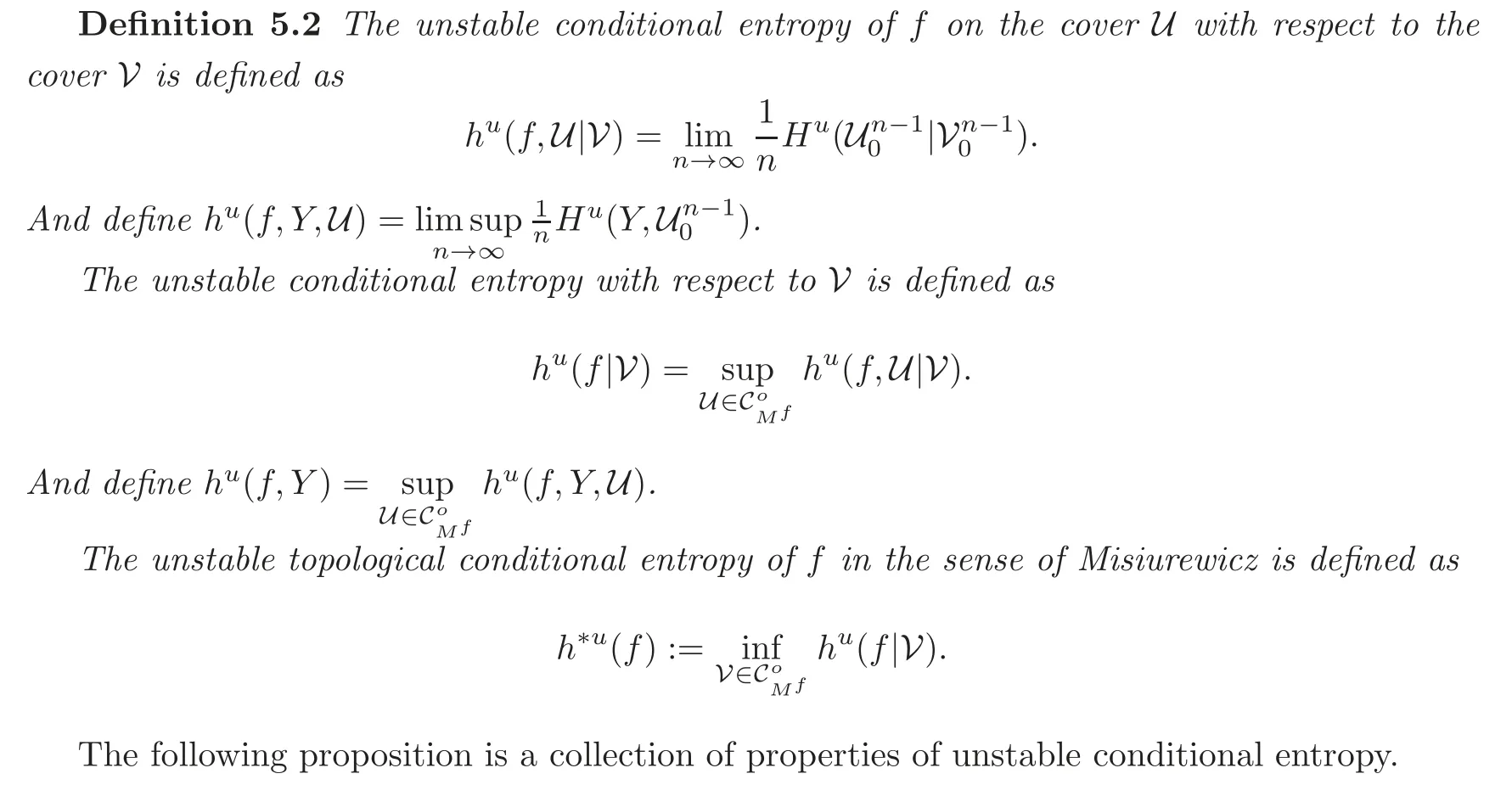
6 Variational Principles for Local Unstable Entropy and Unstable Pressure

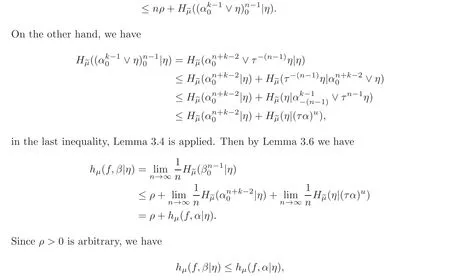
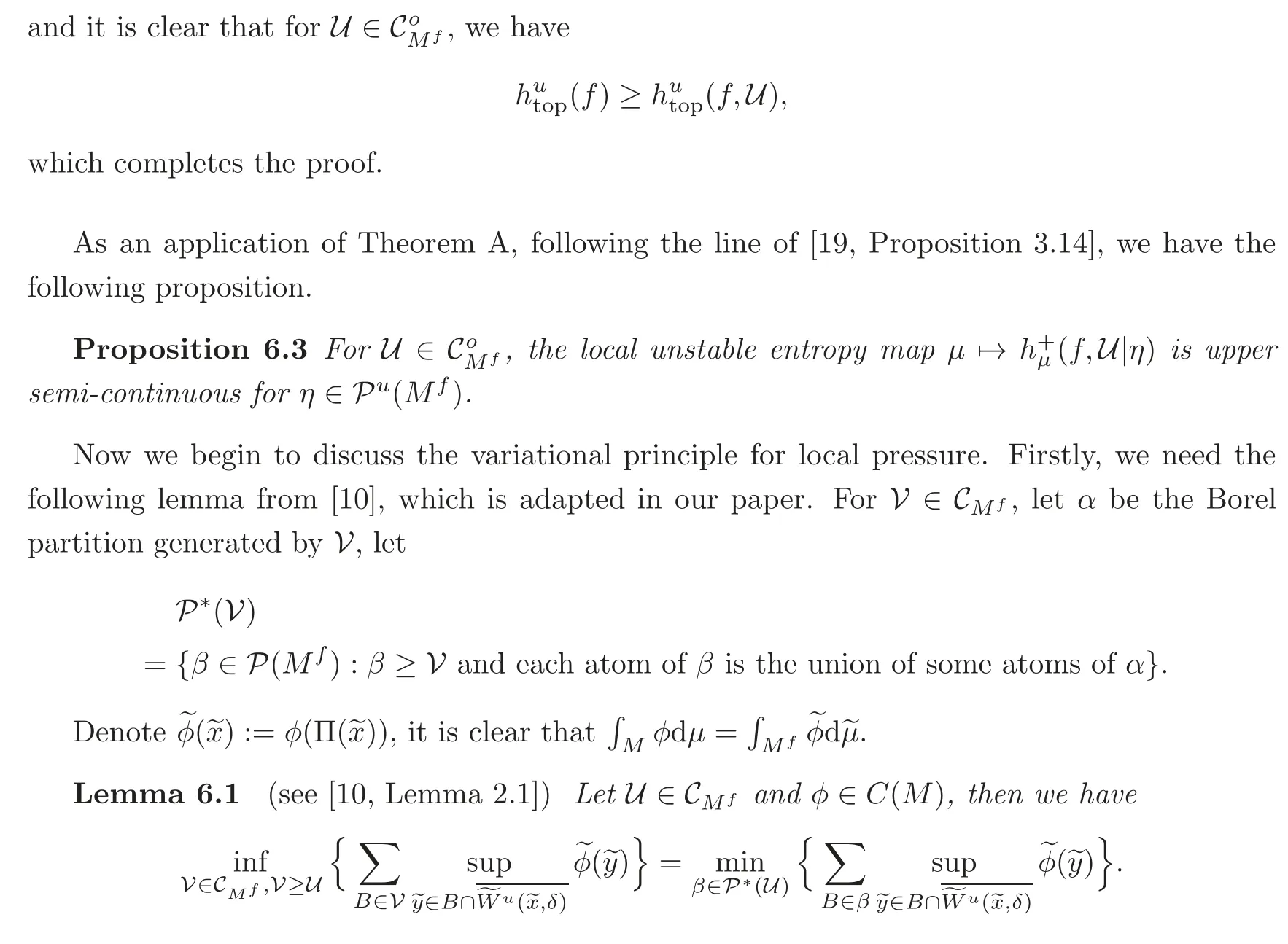
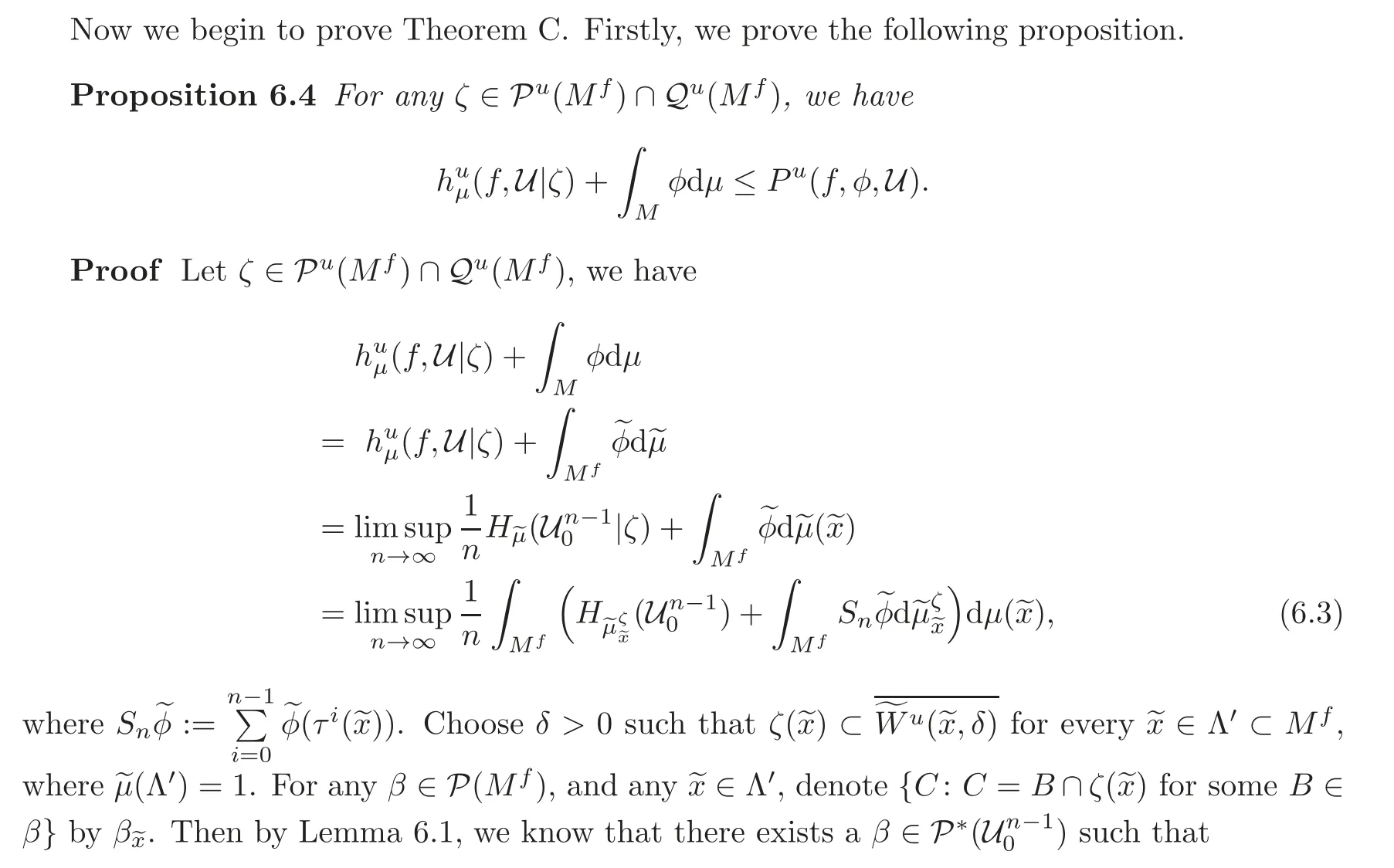
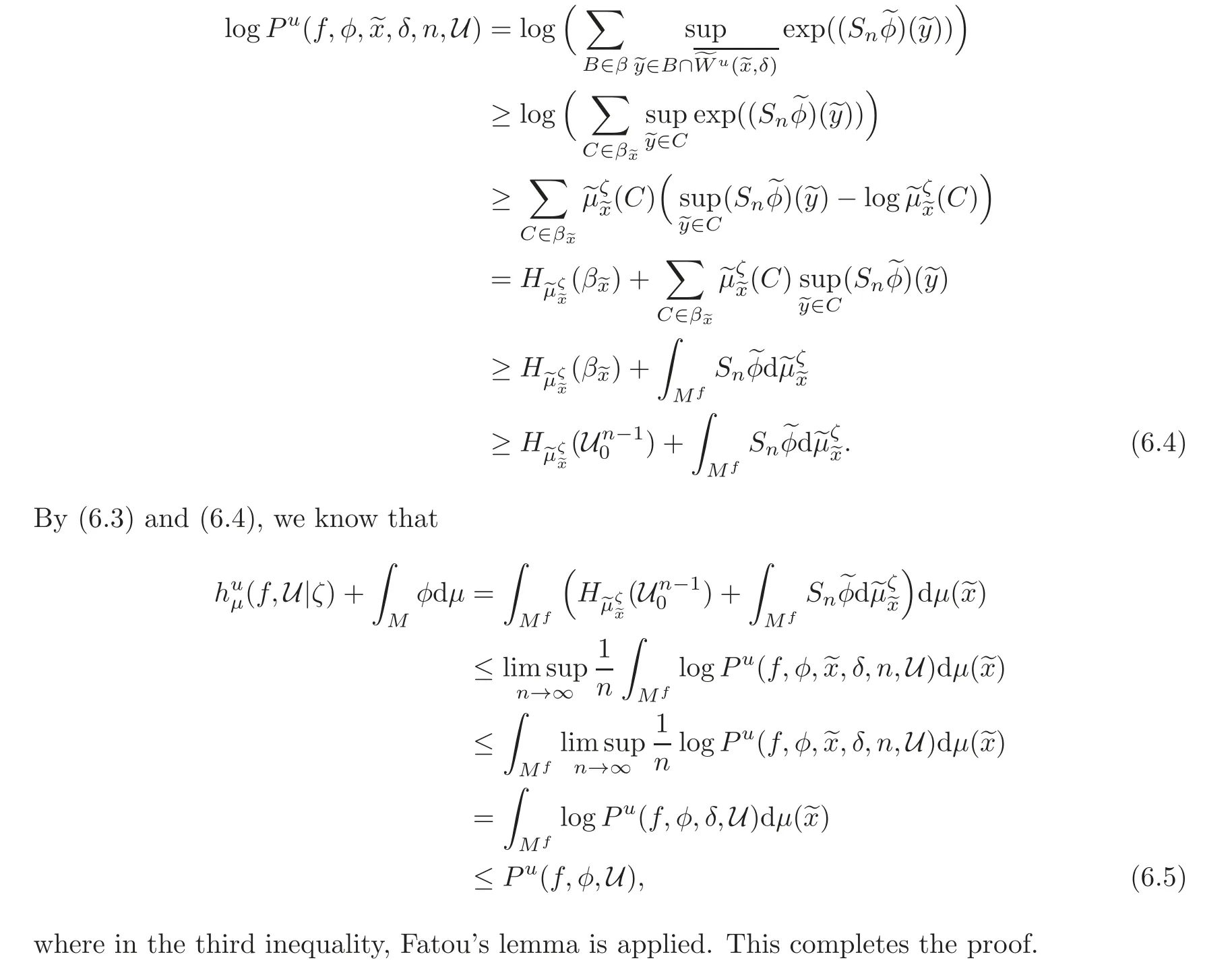
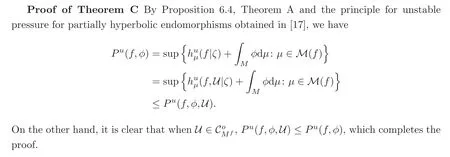
In this section,we prove Theorem A,then variational principles for local unstable entropy and unstable pressure are obtained.First of all,we give two propositions as follows.

AcknowledgementThe author wishes to express his sincere thanks to Professor Yujun Zhu and Professor Weisheng Wu for many useful discussions and constructive suggestions.The author also would like to thank the referees for the detailed review and very valuable suggestions,which led to improvements of the paper.