OPERATOR NORM AND LOWER BOUND OF FOUR-DIMENSIONAL GENERALIZED HAUSDORFF MATRICES*
2022-03-12GholamrezaTALEBI
Gholamreza TALEBI
Department of Mathematical sciences,Vali-e-Asr University of Rafsanjan,Rafsanjan,Islamic Republic of Iran E-mail:Gh.talebi@vru.ac.ir
Abstract The problem addressed is the exact determination of the operator norm and lower bound of four-dimensional generalized Hausdorffmatrices on the double sequence spaces Lp.A Hardy type formulae is found as an exact value for their operator norm and a Copson type formulae is established as a lower estimate for their lower bound.Further,exact values are found for the operator norm and lower bound of the transpose of generalized Hausdorffmatrices.
Key words four-dimensional Hausdorffmatrices
1 Introduction
For p>0,let Lpdenotes the space of all double real or complex sequences x=such that‖x‖p:=<∞,[3].Let V and W be two double sequence spaces and H=(hnmjk) be a four-dimensional in finite matrix of real or complex numbers.Then,we say that H defines a matrix mapping from V into W,and we denote it by writing H:V→W,if for every double sequence x=(xn,m)∈V,the double sequence Hx={(Hx)n,m},the H-transform of x,is in W,where

The operator norm and lower bound of a non-negative four-dimensional matrix H on the double sequence space Lp,are respectively defined by

Obviously Lp(H)≤‖H‖p.To give full knowledge on the boundedness problem of four-dimensional matrices on double sequence spaces,we refer the readers to the recent papers[10-15]and to the monograph[2]for the four-dimensional triangle matrices,spaces of double sequences and related topics.
Let dμ and dλ be two Borel probability measures on[0,1].Then the four-dimensional generalized Hausdorffmatrixis defined by
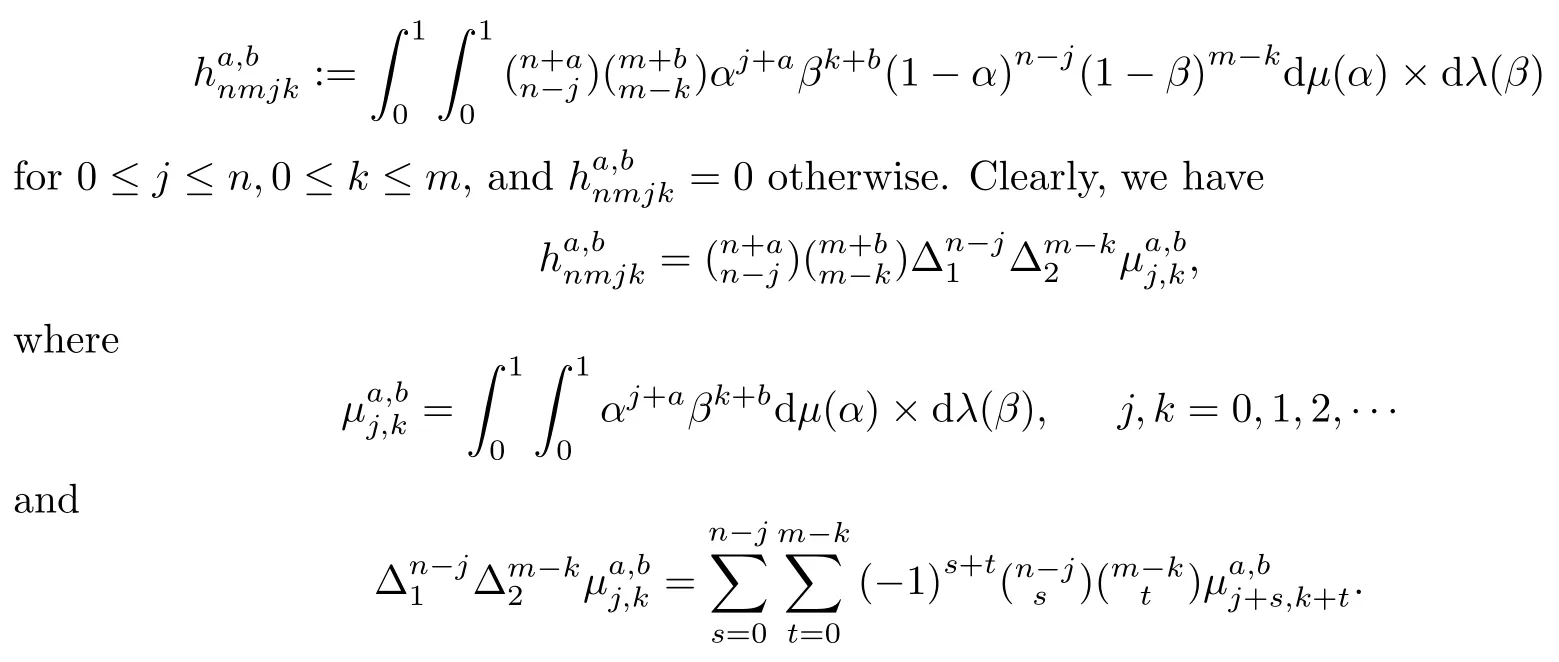
Here a,b≥0,and the cases a=b=0 correspond to the ordinary four-dimensional Hausdorffmatrices[9].
In this paper,we are going to consider the boundedness problem of four-dimensional generalized Hausdorffmatrices and their transpose on the double sequence spaces.In our results,as we will see later,the two values

have essential roles.However,in the first integral for 0<p<1,and in the second integral for 1<p<∞,there are some singularity at the origin.For this reason,we have to restrict ourselves to those Borel probability measures dμ and dλ for which the above integrals exist.So,according to the subject under discussion,we may consider only the four-dimensional generalized Hausdorffmatrices associated with such measures.
2 Computation of the Operator Norm
In Theorem 3.1 of[10],we found the exact value of.In the following,we extend it to the casewith a,b>0.
Theorem 2.1Let 1<p<∞.Then the four-dimensional generalized Hausdorffmatrix is a bounded operator on Lpwith the operator norm

ProofLet x=(xn,m) be a non-negative double sequence in Lp.Clearly,we have


This implies by Theorem 3.3 of[14],that
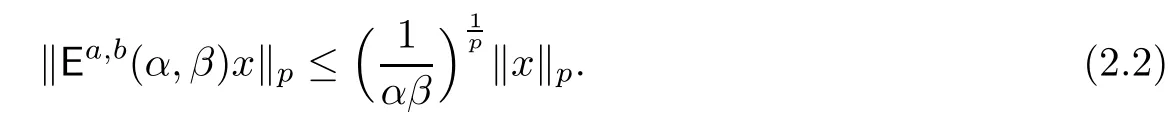
Applying Minkowski’s inequality together with (2.1) and (2.2),we deduce that

Then we have x=(xn,m)∈Lp,and


Since∈is arbitrary,this completes the proof. □
We remark here that Theorem 2.1 can be proved without the use of the Hardy’s method.It suffices to apply Theorem 2.2 of[13]to Corollary 1 of[1].
The generalized four-dimensional Euler and Cesàro matrices are the special cases of the generalized Hausdorffmatrices[14].Applying Theorems 2.1 to them,we have the following results.
Example 2.2Let 1<p<∞.Then the generalized four-dimensional Euler matrix Ea,b(α,β),is a bounded operator on Lpwith the norm

Example 2.3Let 1<p<∞.Then the generalized four-dimensional Cesàro matrix Ca,b(α,β),is a bounded operator on Lpwith the norm

For the operator norm of the transpose matrix,we have the following result as an extension of Theorem 4.1 in[13].Its proof is a consequence of Theorem 2.1.
Corollary 2.4Let 1<p<∞.Then the transpose of the generalized four-dimensional Hausdorffmatrix is a bounded operator on Lpwith the norm

Example 2.5Let 1<p<∞.Then the transpose of generalized four-dimensional Euler matrix is a bounded operator on Lpwith the norm

Example 2.6Let 1<p<∞.Then the transpose of generalized four-dimensional Cesàro matrix is a bounded operator on Lpwith the norm

Remark 2.7For the case 0<p≤1,the problem of finding the exact value ofis still open.
3 Computation of Lower Bound
For the ordinary four-dimensional Hasudorffmatrix,recently in Theorem 2.3 of[12],we have found a suitable formulae for.In the following,we extend it to the casewith a,b>0.
Theorem 3.1Let a,b>0 and 0<p≤1.Then

ProofFor a non-negative sequence x in Lp,we can easily obtain
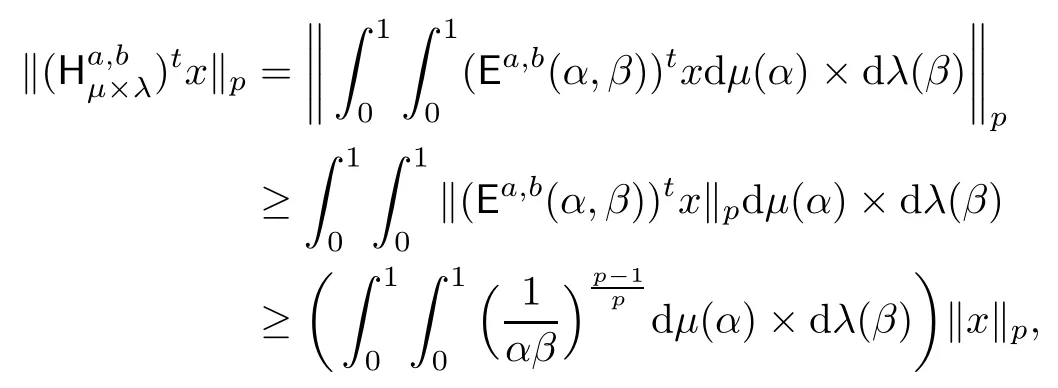
which implies

To show that the constant in (3.1) is the best,inspired by Bennett’s proof ([4],p.33),suppose that ρ>1/p and that n0,m0are fixed integers with n0,m0≥ρ.Consider the double sequence (zj,k)=,where

Then z=(zj,k)∈Lp,and we have
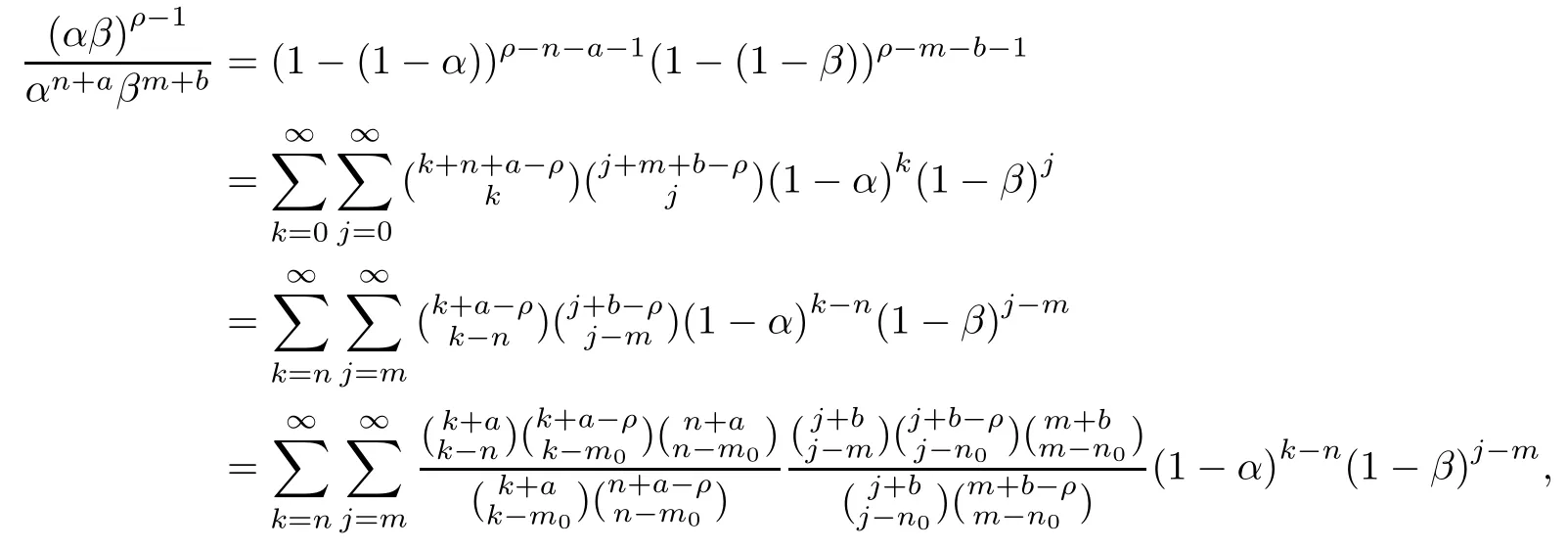
which implies that

By integrating against dμ(α)×dλ(β),we get
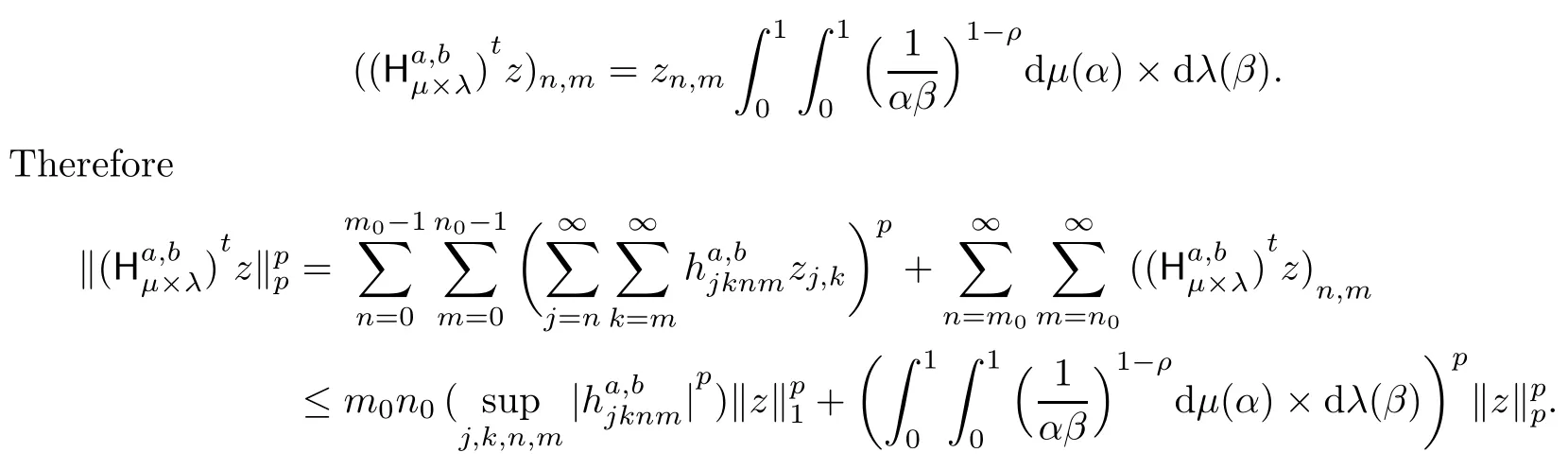
Now if ρ→1/p,then‖z‖p→∞,which gives us

This shows that the constant in (3.1) is optimal and completes the proof. □
Example 3.2Let a,b>0 and 0<p≤1.Then for all α,β∈(0,1),we have

Example 3.3Let a,b>0 and 0<p≤1.Then for all α,β>0,we have

We observe that the inequality
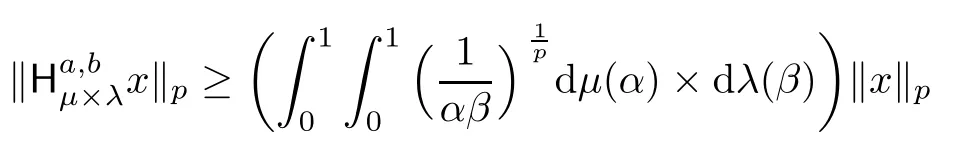
can be obtained in a similar way as in the proof of the proceeding theorem,and enables us to obtain the following generalization of Theorem 2.7 in[12].
Theorem 3.4Let a,b≥0,0<p≤1.Then
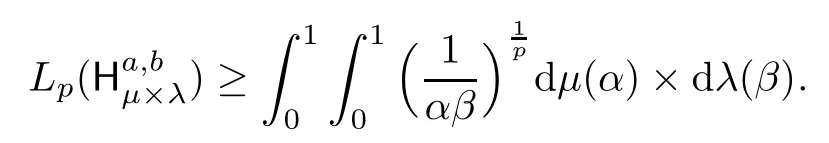
Example 3.5Let 0<p≤1 and α,β∈(0,1).Then

where α,β>0.Then the associated generalized four-dimensional Hausdorffmatrix is called the generalized four-dimensional Gamma matrix Γa,b(α,β),for which we have the following results.
Example 3.6Let a,b>0 and 0<p≤1.Then

Example 3.7Let 1<p<∞.Then the generalized four-dimensional Gamma matrix is a bounded operator on Lpwith the norm
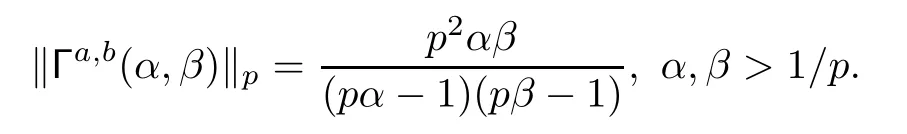
We note that if α or β is less than or equal to,the singularity of the integral (1.1) at the origin is non-integrable.So,in both Examples 3.6 and 3.7,we only consider the cases α,β>1/p.
Example 3.8Let a,b>0 and 0<p≤1.Then

Example 3.9Let 1<p<∞.Then the transpose of the generalized four-dimensional Gamma matrix is a bounded operator on Lpwith the norm

Again we note that if α or β is less than or equal to (p-1)/p,then the singularity of (1.2) at the origin is non-integrable.Therefore,in Example 3.9,we only consider the cases α,β>(p-1)/p.
Remark 3.10For the four-dimensional Cesàro matrix C0,0(1,1),since the singularity of (1.1) is non-integrable for 0<p≤1,the estimate in Theorem 3.4 gives us somewhat strange.The constant in this estimate is in finite and writing the below estimate with in finite constant seems to be formally incorrect.
Remark 3.11For the case 1<p<∞,recently in Theorem 4.3 of[13],we have obtaind the following results for The lower bound of the ordinary four-dimensional Hasudorffmatrix and its transpose,respectively;

For the four-dimensional generalized Hausdorffmatrix and its transpose,using Theorem 6.1(ii) and Theorem 6.2(iii) of[5]together with Theorem 2.1 of[13],we have the following results,respectively;

AcknowledgementsWe would like to thank Professor Graham Jameson and Professor Billy E.Rhoades for their technical assistance during the preparation of the present paper.
杂志排行
Acta Mathematica Scientia(English Series)的其它文章
- UNBOUNDED COMPLEX SYMMETRIC TOEPLITZ OPERATORS*
- HYBRID REGULARIZED CONE-BEAM RECONSTRUCTION FOR AXIALLY SYMMETRIC OBJECT TOMOGRAPHY*
- A SPECTRAL METHOD FOR A WEAKLY SINGULAR VOLTERRA INTEGRO-DIFFERENTIAL EQUATION WITH PANTOGRAPH DELAY*
- TOEPLITZ OPERATORS FROM HARDY SPACES TO WEIGHTED BERGMAN SPACES IN THE UNIT BALL OF Cn*
- THE EXPONENTIAL PROPERTY OF SOLUTIONS BOUNDED FROM BELOW TO DEGENERATE EQUATIONS IN UNBOUNDED DOMAINS*
- ANISOTROPIC (p,q)-EQUATIONS WITH COMPETITION PHENOMENA*
