Effect of atmospheric turbulence on orbital angular momentum entangled state
2022-03-12XiangYanPengFeiZhangChengYuFanandJingHuiZhang
Xiang Yan, Peng-Fei Zhang, Cheng-Yu Fan and Jing-Hui Zhang,*
1 School of Physics and Electronic Information Engineering, Hubei Engineering University, Xiaogan,432000, China
2 Key Laboratory of Atmospheric Composition and Optical Radiation, Anhui Institute of Optics and Fine Mechanics, Chinese Academy of Sciences, Hefei 230031, China
Abstract The entangled orbital angular momentum(OAM)photons propagating across a weakly turbulent atmosphere are investigated.Here, the paper uses the single-phase screen model based on the Kolmogorov theory of turbulence, and focuses on the influence of the backward scattering on OAM evolution.The results indicate that backward scattering plays an important role in the analysis of OAM entanglement evolution in the turbulent atmosphere.It cannot be negligible especially for higher-order OAM mode.Moreover,when OAM mode is greater than 4,entangled photon pairs composed of higher OAM modes are not more robust in turbulence within the weak scintillation regime.These results will be useful in future investigations of OAM-based optical wave propagation through turbulent atmosphere.
Keywords: turbulent atmosphere, backward scattering, orbital angular momentum
1.Introduction
The orbital angular momentum (OAM) photon is currently the focus of intense research within the quantum information community, because in principle it has an infinite number of eigenstates,which can store and process quantum states in a highdimensional Hilbert space.Such a high-dimensional Hilbert space provides better security and more information capacity[1-5].As such, the use of OAM modes of light to encode quantum information could play an important role in long-range quantum communication and higher dimensional quantum key distribution.
Quantum entanglement is an important resource for quantum information processing and quantum communication.Unfortunately, when entangled photons are sent through the turbulent medium, beam wandering, wavefront distortions and scintillation induced by fluctuations of the atmospheric optical refractive index destroy the OAM entanglement.Up to now,various aspects of OAM entanglement decay in atmospheric turbulence have been considered theoretically and experimentally[6-16].A significant number of these studies have focused on two-level (qubit) case, which only projects the multilevel state of each photon onto a two-level encoding subspace[8-16]and calculates the evolution of a truncated density matrix.In recent years, the effects of atmospheric turbulence on high dimensional OAM entanglement have received more and more attention[15,17,18].The case where the output after turbulence is projected into a three-dimensional (qutrit) basis composed of specific Laguerre-Gaussian modes has been mentioned.To our knowledge, there have been rarely reports that discuss the influence of the backward scattering from outside of the encoding subspace to density matrix elements inside of the subspace on the evolution of truncate density matrix yet.The backward scattering is usually expected to be negligible and of minor importance to the evolution of the truncated density matrix [17].However, it is known that the coupling between adjacent OAM modes (those with the smallest difference in OAM) is stronger than the coupling between OAM modes that are further apart[17,19].The density matrix truncation leads to a loss of norm and can also affect the OAM entanglement[20].Furthermore,a beam with larger OAM mode experiences more significant crosstalk than one with smaller OAM mode.Higherorder OAM modes have a larger radius and more rapidlyvarying phase on propagation,leading them more susceptible to turbulence[21].Thus,for higher-order OAM modes,it is more necessary to analyze the impact of the backward scattering upon OAM entanglement.
In the present paper,the decay of OAM entanglement of photon pairs propagation in a turbulent atmosphere modeled with a single-phase screen has been numerically studied.We particularly focus on the effect of the backward scattering on OAM evolution of two-dimensional state.After the photons propagate through the turbulent media, OAM entanglement evolution of each photon which is projected onto the multilevel state is investigated for calculating the effect of the backward scattering on entanglement evolution.Additionally,the photons are assumed to be monochromatic, uniformly polarized and they propagate paraxially through turbulent media.OAM entanglement is quantified in terms of Wootter’s concurrence [22].The paper is organized as follows: the numerical procedure is introduced in section 2.In section 3,the numerical results and analysis are presented.The conclusion is presented in section 4.
2.Numerical procedure
The quantum optical system is shown in figure 1.It is assumed that a source field produces a pair of photons,which are initially in Bell state encoded by LG modes with the opposite azimuthal quantum number

Figure 1.Diagram showing how the OAM entangled biphoton propagates through weakly turbulent atmosphere, modeled as random phase screens, toward the two detectors.

where the subscripts A and B are labeled as the two different paths and the parameterℓis the azimuthal mode order.The LG mode can be described in cylindrical coordinates by [19]

When two photons propagate through independent turbulence, each phase screens introduce the phase aberrations that cause the OAM modes of each photon scattered into neighboring OAM modes.To describe the entanglement evolution,it is useful to recall the density matrix of two-photon output stateρoutthat is related to the density matrix of the initial stateρin= ∣ψ〉in〈ψ∣,by the formula [11, 15]

where Λi(i= 1, 2)is the linear map which represents the action of an ensemble averaged phase screen on each photon.It is customary to assume the parameters in each turbulence are the same,which allows us to set Λ1= Λ2= Λ.The density matrix element at the output of the turbulence, is given by

where the basis element denoted by∣ℓm〉(∣ℓm′〉 )and∣ℓn〉(∣ℓn′〉 )can be explained as the states at the measurement stage.The state∣Iψ〉is the initial state∣ψ〉inafter propagating through turbulence.The turbulence medium is assumed to be modeled with a single-phase screen, where the cumulative effect of the turbulence is reduced to phase errors in the beam’s transverse profile[19].This allows us to express the state∣Iψ〉in cylindrical coordinates as

wherejφ(j=A,B) is the random phase perturbation of each turbulence.
Because the OAM mode coupling is strongest for neighboring modes, here we keep track of the OAM information contained in the modes where±ℓ±1 and±ℓ.It should be mentioned that previous studies of the effects of atmospheric turbulence on the OAM modes only obtain the OAM information that is contained in OAM modes either±ℓ[10-16] or±ℓand 0 [17, 18].One finds that if the initial azimuthal mode order ofℓ=1 is given, the output density matrix is a 25 × 25 matrix.Otherwise, for∣ℓ∣>1,it is a 36 × 36 matrix (see appendix).Generally, in a practical quantum information system the information would be encoded in a finite-dimensional subspace.For this reason,one only needs extract the output density matrix elements in subspace∣ℓ,ℓ〉, ∣ℓ, -ℓ〉 , ∣ -ℓ,ℓ〉 , ∣-ℓ, -ℓ〉.Further, the backward scattering from outside the subspace to elements in the subspace is considered.With the above analysis, the resulting density matrix can now be written as

For initial azimuthal quantum number∣ℓ∣=1, the matrix elements read


On the other hand,the resulting density matrix elements lead to

and three distinct nonzero matrix parameters are recognized as the survival amplitudes (a) described the survival probability of the initially populated OAM modes onto themselves(Δℓ= 0) [11], crosstalk amplitudes (b) indicated the crosstalk probability of the initially populated modes±ℓonto OAM modes∓ℓ(∣ Δℓ∣ = 2ℓ) [11], and crosstalk amplitudes(cj(j= 1, 2, 3)) shown the crosstalk probability of OAM modes onto the nearest neighbor modes(∣ Δℓ∣ = 1), respectively, described by


whereis called the atmospheric refractive index structure constant which describes the strength of turbulence along the path,zis the propagation distance, andλis the wavelength.The state∣ψℓ〉(∣ψ-ℓ〉) and∣ψ±ℓ±1〉(∣ψ±ℓ∓1〉) are output state of single photon state ∣ℓ〉(∣-ℓ〉) and ∣±ℓ±1 〉(∣±ℓ∓1〉),respectively.Whenc1=c2=c3= 0can be satisfied,equation (7) reduces to the case of projecting each photon state onto two-dimensional OAM space, which ignore the backward scattering from outside the encoding subspace to elements inside the encoding subspace

In the last step, the quantum entanglement of a bipartite qubit state is quantified in terms of Wootter’s concurrence[22], which is used as a measure of entanglement, given by
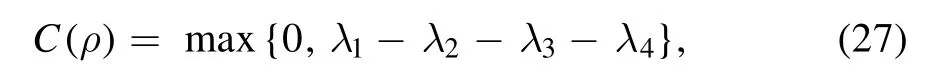
whereλiare the eigenvalues, in decreasing order, of the Hermitian matrixξ

with the asterisk representing the complex conjugate andyσbeing the Pauliymatrix

Note that, if equation (7) is normalized by its trace, using equations (26)-(29), an analytical expression for the output entanglement can be obtained

which is the same with the result in [11].
3.Numerical results and analysis
Based on the derived analytical results in the above section,the entanglement evolution of OAM photons propagating in atmosphere turbulence is investigated.First, our numerical results are compared with the result in [11].It can be seen in figure 2 that the numerical result agrees well with the result in[11] when∣ℓ∣≤3.However, the numerical results diverge from the result in[11]for higher values of∣ℓ∣ .This difference comes from the effect of the backward scattering.Therefore,the backward scattering plays an important role in the analysis of OAM entanglement evolution in the turbulent atmosphere.It can not be negligible especially for higher values of∣ℓ∣ .
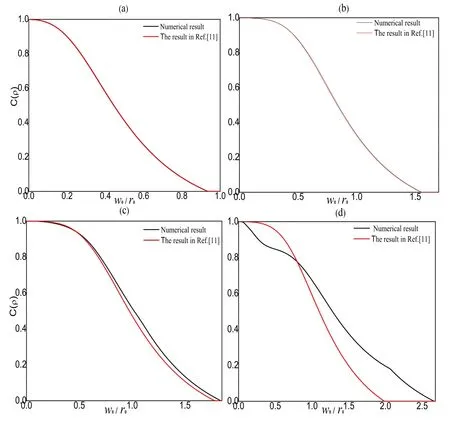
Figure 2.Concurrence as a function of ratio for different values of the azimuthal modes.In (a) ℓ = 1,in (b) ℓ = 3.In (c)ℓ = 4,in (d) ℓ =5.
This is more clearly seen in figure 3 where the numerical results are plotted against a function of ratioon a logarithmic scale.From the resulting curves in figure 3, one can see that the concurrence survives long for higher values of∣ℓ∣ .This is because with the azimuthal mode increasing, the OAM beam widens, and its phase front oscillates more rapidly,which implies that its spatial phase structure gets finer[11, 14].Moreover, at a scale< 1,it is found forℓ= 1, 3, 4entangled photon pairs composed of higher OAM modes are more against atmospheric decoherence, as was observed previously [7, 9, 12-14].However, when OAM modeℓ=5 is considered, the concurrence decay more quickly, which means the entangled OAM photons with larger values of the azimuthal mode order are not more robust in weak scintillation when OAM mode is greater than 4.This deviates from the original trend that was found that the entanglement to be more robust in weak scintillation for higher OAM values.This can be understood as follows.The neighboring modes in the OAM coupling each other strongly when separated by∣ Δℓ∣ = 1and much weaker when separated by∣ Δℓ∣ = 2ℓ[24].As a result the coupling betweenℓ=1andℓ= - 1(∣ Δℓ∣= 2) is much stronger than∣ Δℓ∣ = 6, 8, 10.This, of cause, means that the backward scattering affectsℓ= 5more greatly thanℓ= 1, 3, 4,as shown in figure 2.Due to the stronger couplings predicts a much quicker decay rate for the concurrence, thusℓ= 3andℓ= 4are better thanℓ= 1.Additionally, a beam with larger value of∣ℓ∣experiences significant crosstalk than one with smaller value of∣ℓ∣[21].The coupling betweenℓ= 5andℓ= - 5becomes much smaller, and the backward scattering has a great influence onℓ= 5,leading toℓ= 5worse thanℓ= 3andℓ=4.
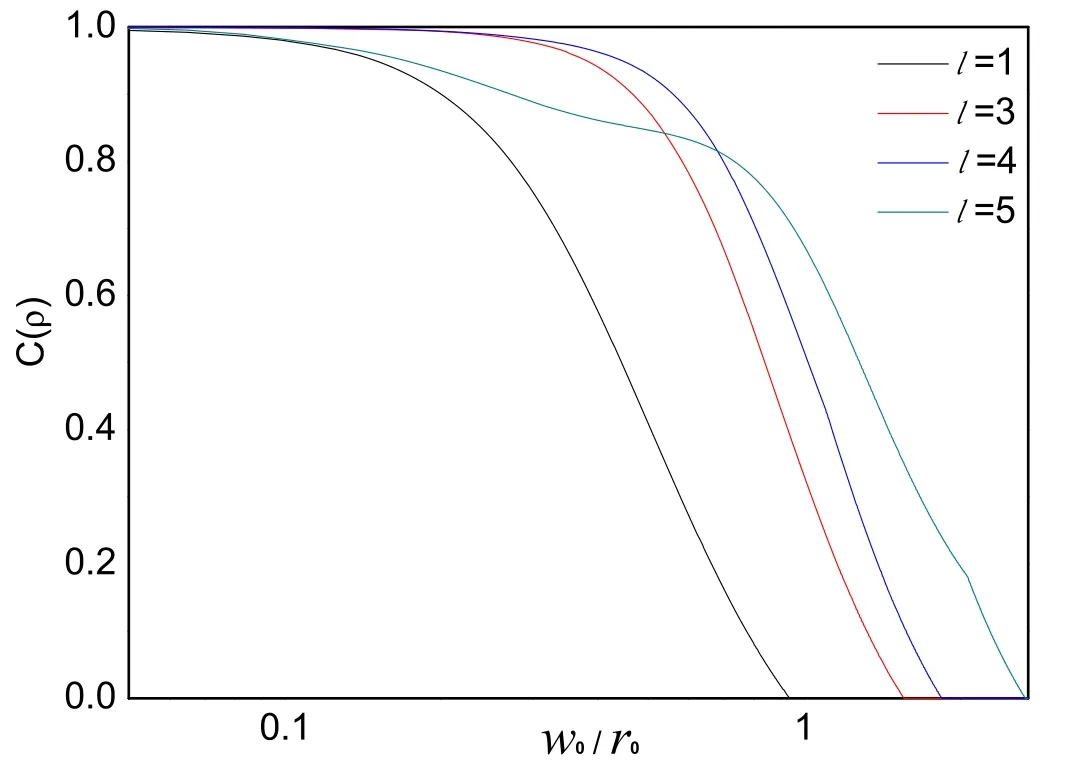
Figure 3.Concurrence as a function of ratio for different values of the azimuthal modes.
It should be noted that the original trend applied Bell states within the weak scintillation regime [9, 12-14].It has already been shown that this trend does not apply in strong scintillation and high-dimensional states in weak scintillation[13, 18].Here, it is shown that the trend also does not apply for Bell state of two-dimensional bipartite systems in weak scintillation when the effect of backward scattering on OAM entanglement is considered.
4.Conclusion
In summary,the effect of backward scattering on entanglement evolution of entangled OAM two qubit state in weak scintillation was numerically investigated.The case in which both photons passed through turbulence was considered and our results were compared with the result in[11].The results show that the numerical result agrees well with the result in [11]when∣ℓ∣≤3.However,the numerical results diverge from the result in[11]for higher values of∣ℓ∣ .Therefore,The backward scattering plays an important role in the analysis of OAM entanglement evolution in turbulent atmosphere and can not be negligible especially for higher values of∣ℓ∣ .In addition, our results reveal that OAM entangled state with larger OAM values survives for longer distances,suggesting that states with larger OAM values are more suitable for long distances freespace quantum communication.Moreover, at a scale< 1,it is found forℓ= 1, 3, 4entangled photon pairs composed of higher OAM modes are more robust in turbulence, as was observed previously.However, when OAM modeℓ=5 is considered,the evolution of OAM entanglement decays more quickly.This deviates from the original trend that was found for Bell states that the entangled OAM photons with larger values of the azimuthal mode order are more robust in weak scintillation.It is believed that these findings would play an important role in the design of a free-space QKD system.
Acknowledgments
Project supported by the Major Research Plan of the National Natural Science Foundation of China (Grant No.11847118).
Appendix
If∣ℓ∣=1 is given, we keep track of the OAM information contained in the modes:

By virtue of equations (4)-(6), the output density matrix is obtained
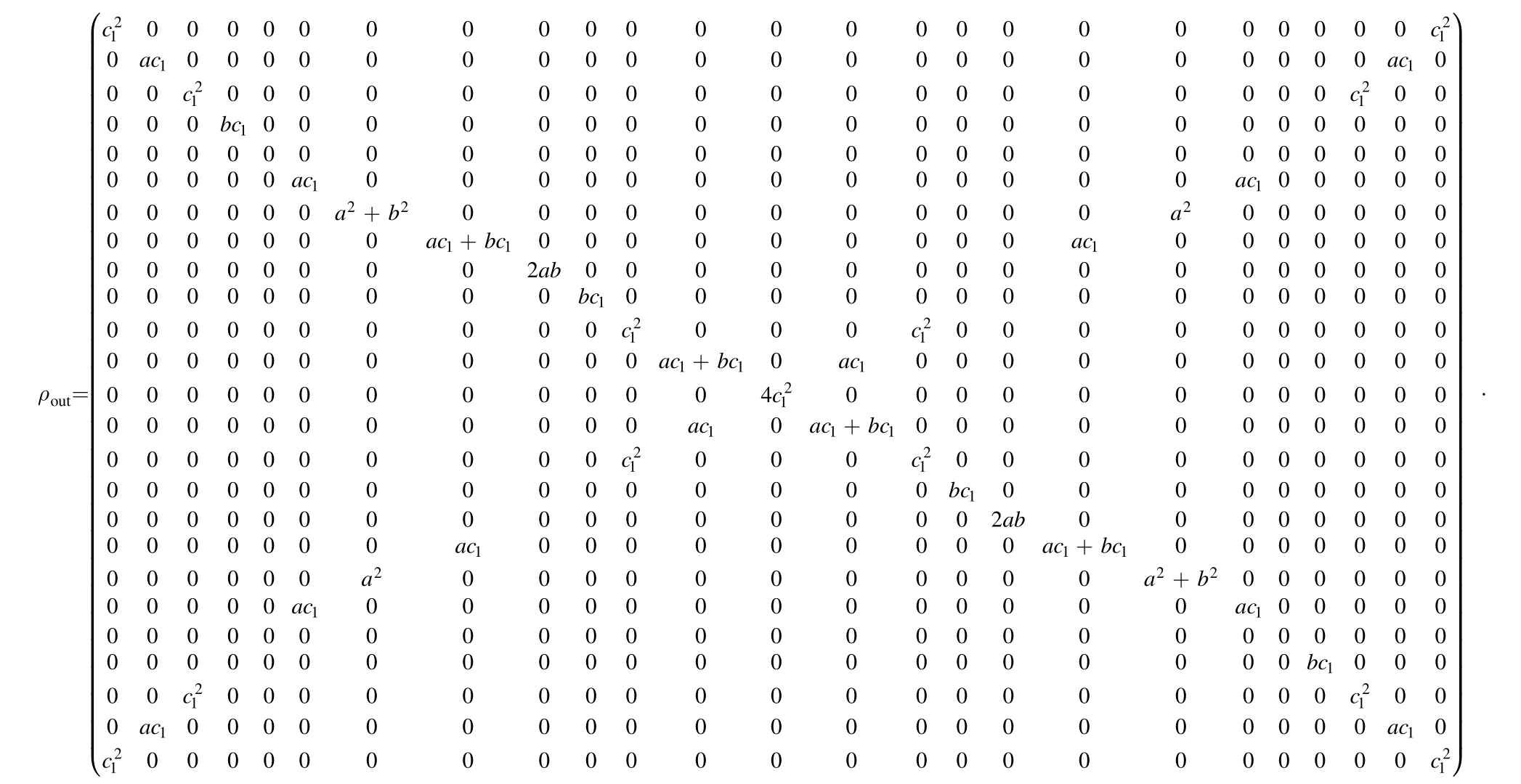
Because Wootter’s concurrence can be used as a measure of entanglement for 4 × 4 density matrix system, the paper only extracts the information in OAM subspace∣ 1 , 1〉, ∣ 1, - 1〉 , ∣- 1 , 1〉 , ∣- 1, - 1〉.Note thatc3= ∣〈ψ0∣ ± 1〉 ∣2= 0.Using steps similar to those that produced the output density matrix, the backward scattering from outside the subspace to output density matrix elements inside subspace can be obtained.The resulting matrix elements are:


Otherwise, for∣ℓ∣>1, the OAM information is restricted in the modes:
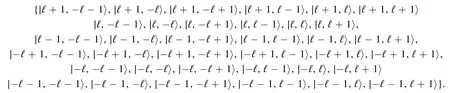
The output density matrixρoutcan be expressed as:

The resulting density matrix elements:
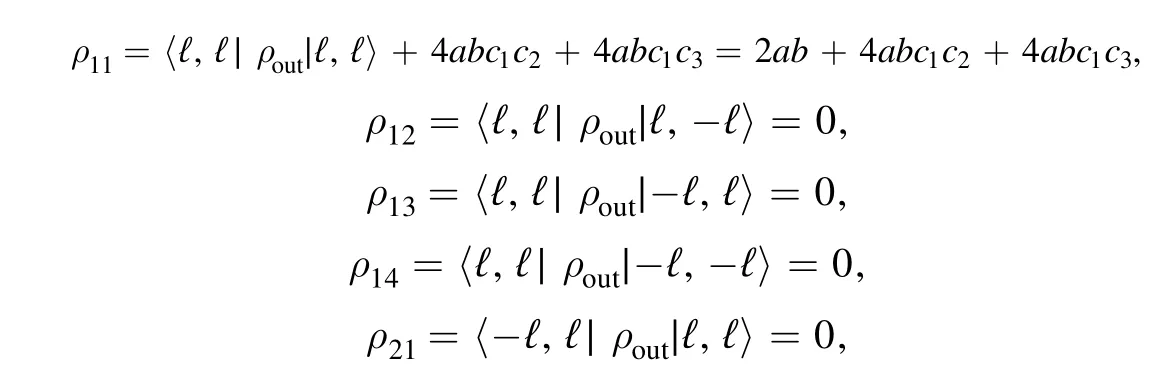
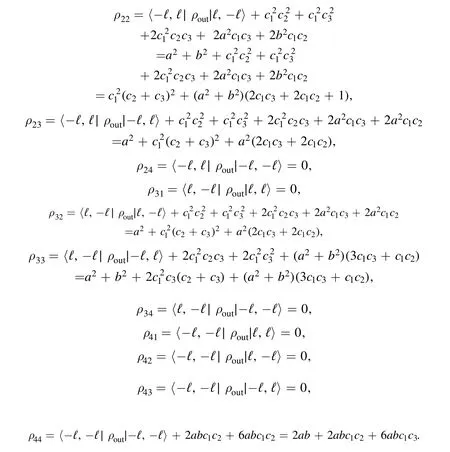
杂志排行
Communications in Theoretical Physics的其它文章
- The Sharma-Tasso-Olver-Burgers equation: its conservation laws and kink solitons
- Magnetically propelled Carreau fluid flow over penetrable sensor surface influenced by thermal radiation, Joule heating and heat generation
- Stable high-dimensional solitons in nonlocal competing cubic-quintic nonlinear media
- Few-photon routing via chiral light-matter couplings
- Persistent and enhanced spin-nematic squeezing in a spinor Bose-Einstein condensate
- Block-coherence measures and coherence measures based on positive-operator-valued measures
