Few-photon routing via chiral light-matter couplings
2022-03-12YaYang杨亚JingLu卢竞andLanZhou周兰
Ya Yang (杨亚), Jing Lu (卢竞) and Lan Zhou (周兰)
Synergetic Innovation Center for Quantum Effects and Applications, Key Laboratory for Matter Microstructure and Function of Hunan Province,Key Laboratory of Low-Dimensional Quantum Structures and Quantum Control of Ministry of Education, School of Physics and Electronics, Hunan Normal University, Changsha 410081, China
Abstract A quantum router is one of the essential elements in the quantum network.Conventional routers only direct a single photon from one quantum channel into another.Here, we propose a fewphoton router.The active element of the router is a single qubit chirally coupled to two independent waveguides simultaneously, where each waveguide mode provides a quantum channel.By introducing the operators of the scatter-free space and the controllable space, the output state of the one-photon and two-photon scattering are derived analytically.It is found that the qubit can direct one and two photons from one port of the incident waveguide to an arbitrarily selected port of the other waveguide with unity, respectively.However, two photons cannot be simultaneously routed to the same port due to the anti-bunch effect.
Keywords: few-photon routing, nonreciprocity, anti-bunching, chiral waveguides
1.Introduction
Quantum channels, which serve mainly to distribute quantum information, are building blocks of scalable quantum information processing and quantum communication.In a quantum channel, the information is usually carried by single photon,which is an ideal carrier of a flying qubit for long-distance communication due to the fact that photons have vanishingly small cross sections for direct coupling.However,digital signal processing requires photon-photon interactions on the level of a single quantum.Since the nonlinearities in nonlinear crystals are tiny at the single photon level, it would be fulfilled by the mediation of material systems.Although a single atom is able to have an appreciable influence on the light field,a strong matterlight interaction is desirable for not being masked by the dissipative coupling to the environment.The attainment of strong coupling was pioneered by putting an atom in tiny high-Q cavities [1-4], where the electric field enclosed in three dimensions, and antibunching has been observed [5-7].As the technology proceeds to being smaller on chip structures, strong coupling is also achieved between a trapped atom and the singlephoton flying through the waveguide.Then the coherent of single photons controlled by interaction with an atom inside a waveguide have been a subject of considerable interest in recent years [8-21], since a waveguide confining photons to a onedimensional (1D) geometry increases the atom-photon interaction and the field remains uniform in the longitudinal direction of propagation.With waveguides becoming basic elements for complex quantum networks, quantum devices operating on the level of a single quantum are necessary for signal processing in waveguides.Quantum router is one of the essential elements in the quantum network, which directs single photons from a coherent input to a separate output.Single-photon router is proposed both theoretically and experimentally by exploiting the strong coupling between an atom and the confined field[22-34].The chiral atom-photon couplings has even been used to direct a single photon from the input port to an arbitrarily selected output port deterministically.However, few attentions have been paid on the routing of two photons from the input port to anarbitrarily selected output port.Here, we proposed a one-and two-photon router.The active element of the router is a single qubit chirally coupled to two independent waveguides simultaneously.The study of the scattering of few photons by the qubit shows that we can route one and two photons from an input port of the incident waveguide to an arbitrarily selected port of the other waveguide with a ratio of unity, and the routed photons are anti-bunched.
This paper is organized as follows: in section 2, we present the theoretical model for a qubit coupled to two identical 1D waveguides.The scatter-free subspace and the controllable subspace has been introduced.In section 3, we study the scattering process of one photon in the real-space and give the expressions for scattering coefficients, the properties of the nonreciprocity are discussed.In section 4,we study the scattering process in the double excitation subspace in the real space.After obtain the output wavefunction for the photon-pair incident from one port of a waveguide,the anti-bunch and bunch effect are discussed for photon-pair appearing to the same port,and the conditions of incident photons routed to desired ports are studied.Finally,we conclude with a brief summary of the results.
2.Model for a qubit coupling to two 1D waveguides
The system we consider is depicted in figure 1: a pointlike scatterer e.g.a two-level quantum emitter or qubit at coordinate x=0, is coupled to two identical 1D waveguides,which we label a and b respectively, We neglect thermal fluctuations and losses.The qubit absorbs and emits photons with rates γpland γprinto the left- and right-going modes along waveguide p=a, b.The emission of the qubit into guided modes of waveguide p is depicted by γp=γpr+γpl.The Hamiltonian of the total system consists of three parts

The qubit Hamiltonian is given by

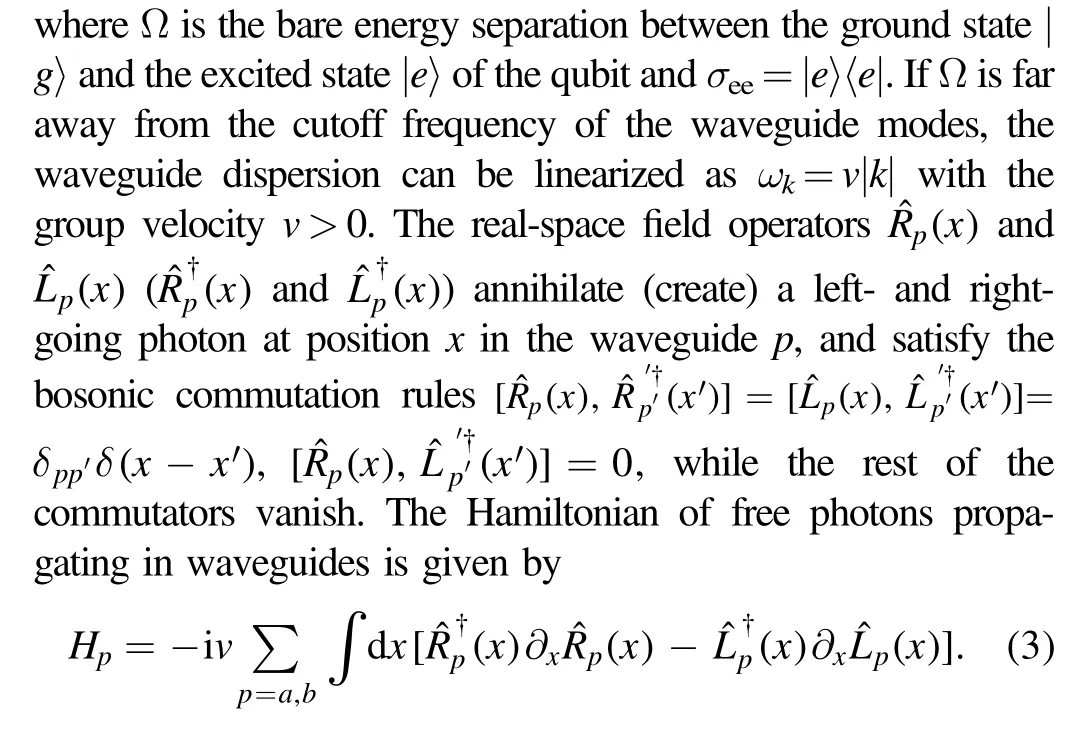
The variable x takes values in the continuum-∞<x <+∞.The dipole Hamiltonian under the rotating-wave approximation


Figure 1.(a) Schematic of a few-photon router with four ports: a two-level system interacts with two independent waveguides labeled by a and b.The blue (green) line represents the chiral coupling between the two-level atom and the left and right going photons of the bottom waveguide a (b), and the coupling rates are γar (γbr) and γal (γbl), respectively.(b) An equivalent few-photon router by introducing the scatter-free channels and the controllable channel:two-level system only interacts with the lower C mode with strength determined γ.
is adopted to describe the coupling between the qubit and the field as long as the coupling strengthsare much smaller than the excitation energy Ω.σ-=|g〉〈e|is the lowering operator of the qubit.The direct coupling between waveguides is neglected for simplify.This simplification can be realized as long as the distance between the two waveguides is much larger than the characteristic length of a two-level quantum emitter.
We further introduce the following transformation

where γp=γpl+γpr,the free Hamiltonian for the waveguides is transformed into

and the interaction Hamiltonian between the qubit and photons reads
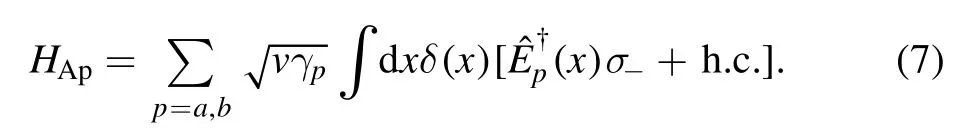
By defining the creationCˆ†andDˆ†as a linear combination of the real-space field operators of two waveguides

the Hamiltonian of the total system is the sum of the Hamiltonian Hcof the controllable space and Hdoof the scatter-free space, i.e.H=Hc+Hdowhere

Here, the qubit couples to the C mode of the field with an effective coupling constant characterized by the total decay of the qubit γ=γa+γb.It can be found that the number of excitations in this system is conserved.
3.Transport property of one photon
As the photons in the O and D spaces propagate freely, the scattering process mainly occurs in the C space.Since a photon lying at x=0 can be absorbed by the qubit, the eigenstate of Hctakes the form
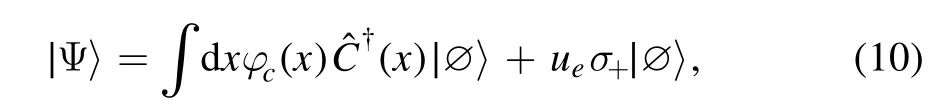


The photon coming from one side of the qubit in one waveguide can be scattered into four different ports,resulting in a reflected, transmitted and transferred waves.We aim to determine the scattering coefficients for an incident monochromatic photon.Photons incident from the left side of the qubit in waveguide p are described by the state
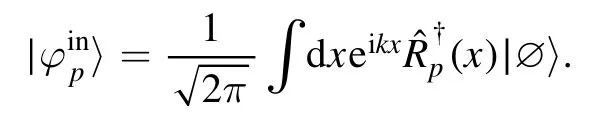

For photons incident from the right side of the qubit in waveguide p
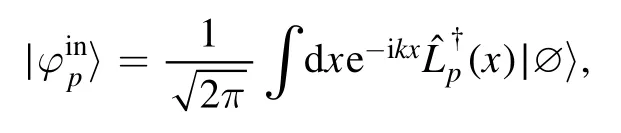
one can also obtain the four scattering amplitudes in a similar way
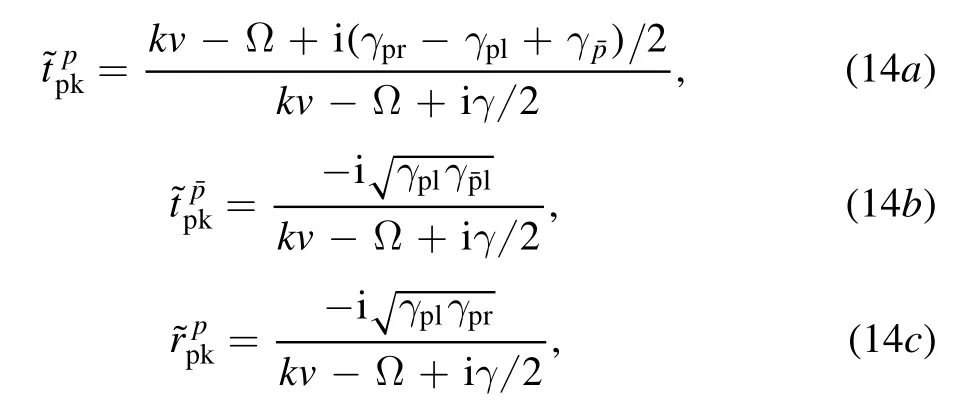
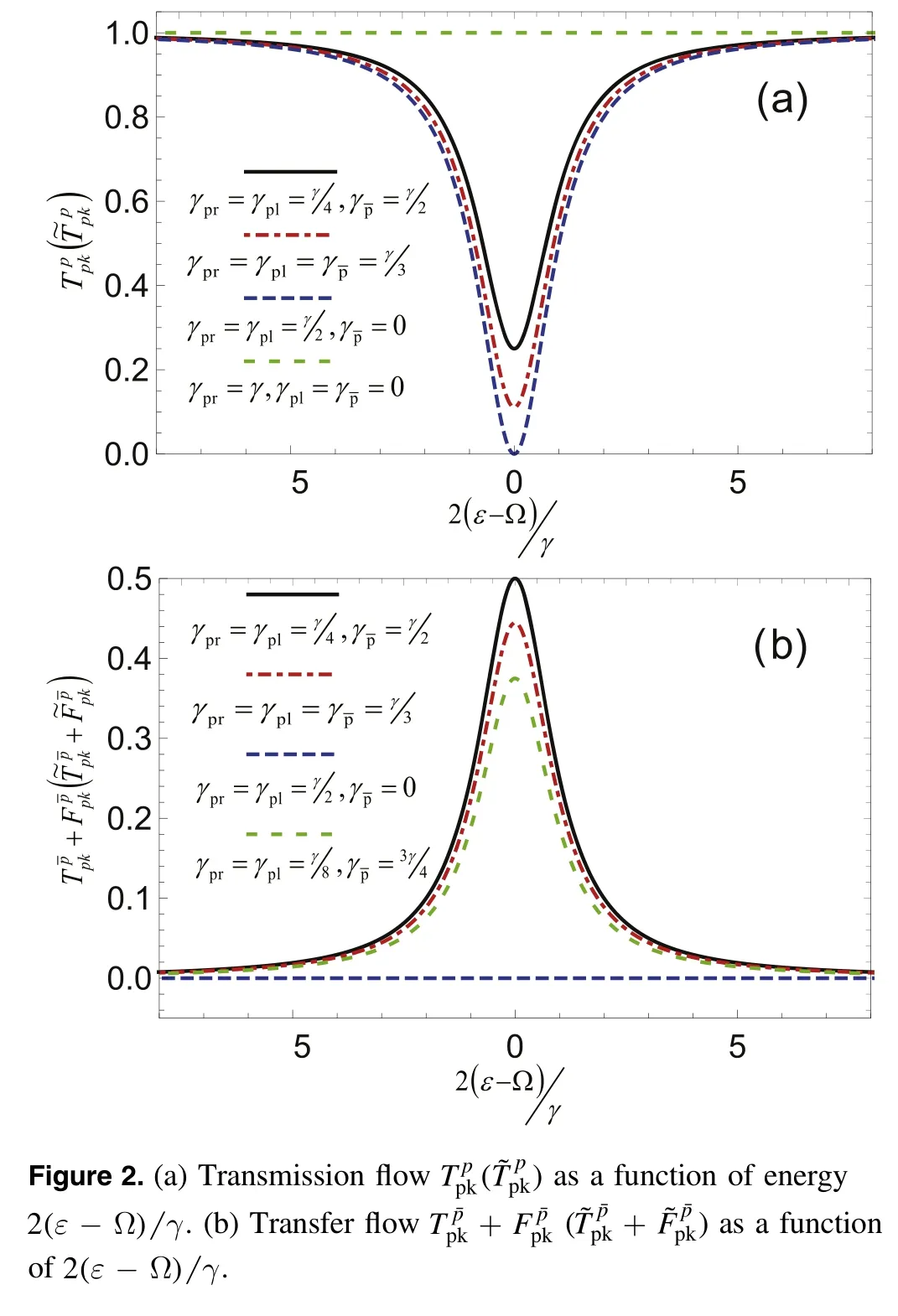




4.Transport property of two photons
In this section, we consider the scattering process in the double-excitation subspace.Since any photons incident far away from the qubit in waveguides can be decomposed to the Oa,Ob,D and C modes,photons in the Oa,Ob,and D modes propagate freely,so we need to find the two-photon scattering state in the C space.An eigenstate for Hamiltonian Hcis a superposition of two-excitation states:


The boson statistics allowed us to confine the solution on x1<x2and the solution for x1>x2can be obtained by interchanging x1⇐x2.At the region that all photons are away from the qubit,there are two kinds of solutions[8,10]:plane waves with wave vectors k andk′ and energyE=v(k+k′)
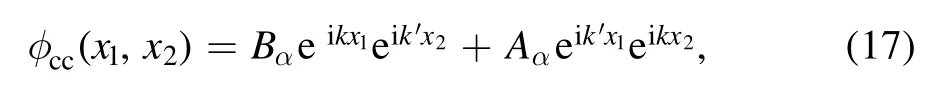
and two-photon bound state

where regions 0 <x1<x2, x1<0 <x2, and x1<x2<0 are denoted by α=1, 2, 3 respectively.The interaction between the qubit and field gives the boundary conditions [8]

for waves at the position x2>x1=0, and
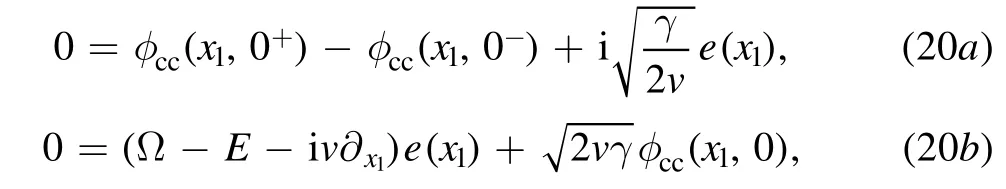
for waves at the position x2=0 >x1as well as the matching condition

The conditions help us to find

for the plane waves in equation (17) and β=γ/2,
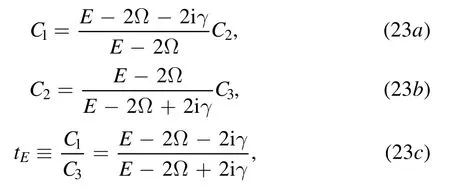
for bound state in equation (18).
The solution of the eigenstate in the real space allows us to consider the two-photon scattering by the qubit.A twophoton state incoming from the left in waveguide a is given by

The amplitude with the center-of-mass coordinatexc=and the relative coordinate x=x1-x2reads

where the total energy of the two photonsE= (k+k′ )vand the energy difference between two photonsFor later convenience, we introduce
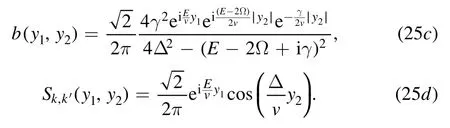
Each of photons impinges on the qubit, being eventually scattered off.Each one might be reflected to the left side of the qubit (denoted as port 1) and transmitted to the right side of the qubit(denoted as port 2)in the same waveguide,or be directed to the left side of the qubit (denoted as port 3) and transmitted to the right side of the qubit (denoted as port 4)instead of continuing in the same waveguide.Then the output state contains ten terms: Both photons are reflected to port 1 or 3 with amplitudesρ11(x1,x2)orρ33(x1,x2);


Figure 4.(a)-(d) The probability as a function of the scaled relative coordinate γx.When vk =vk ′= Ω for (a)-(c) and E-2Ω=0,Δ=γ/2 for (d), where the damping rates are setting as follow: (a)γar=γal=γ/2,γbr=γbl=0; (b)γar=γbr=γ/2,γal=γbl=0; (c)γar=γ/2, γal=0, γbr =γ/6, γbl=γ/3, (d) γar=γ/2, γal=0,γbr=γ/6, γbl=γ/3.The condition of the vanishing probability for two photons transmitted to port 2 at x=0 when (e) γar=γ/2 and(f) E-2Ω=0.
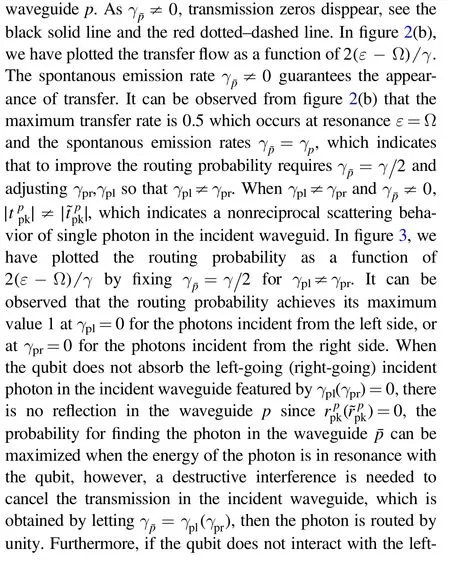
It can be found from equations (26), (27), (28a) and(29b) that the probabilities for two photons appearing to the same port (denoted byand the same side of the qubit are independent of the centerof-mass coordinate.However, the probabilities for two photons traveling to different sides of the qubit are independent of relative coordinate, which can be seen from equation (28b),(29a), (30) and (31).The spatial features of the output photons are characterized by the interference between the plane wave and the wave of the bound state.When the energies of the two incident photons are equal and on resonance with the atom (i.e.vk=vk′= Ω), only anti-bunching can be found for output photons which change the initial propagating direction to the same port,the direction change of photons are caused by the absorption and emission of photons by the qubit, but qubit can only absorb one photon and emit it later once.And the bunched output photons can only be observed when they keep the initial propagating direction.In figures 4(a)-(d),we have plotted the probability as a function of the scaled relative coordinate γx.Whenvk=vk′= Ω,T22(green short dashed line in figures 4(a)-(c)) decays exponentially as |x| increases, and thus they are in a bound state.When the total energy of the photon-pair is kept on resonance with the qubit while the energy difference between the photons increases (E-2Ω=0, Δ=γ/2), the peak at x=0 reduces zero, then the photon-pair transmitted to port 2 change from bunching to antibunching.However,the photonpair traveling away from their incident direction are always antibunching.In figure 4(a),R33and T44is always zero in the absence of the other waveguide.In figure 4(b), R11and R33vanish due to no coupling between the qubit and the leftgoing modes of both waveguides.However,when the qubit is allowed to emit a photon to the left-going modes of the waveguide b, T44decreases.To understand how the change from bunching to antibunching depend on the parameters,we have plotted T22for γar=γ/2 in figure 4(e) and for a fixed total energy E=2Ω in figure 4(f).The solid lines in figures 4(e)-(f)present the condition for T22(0)=0.It can be found that at the condition of γar=γ/2, the photon-pair exhibit a bunching effect as long as|Δ|<γ/2 no matter what the value of total energy E is,and at the condition of E=2Ω,bunching effect appears to the photon-pair as long as γar<γ/4.
To discuss the routing of the photon-pair from the incident waveguide into the other waveguide, we introduce the definition of the detection probabilities as

which denote the photons detected at ports n and m.The number of the probabilities is ten, and the ten probabilities add up to unity.The total probability for the photon-pair to be routed is depicted by P33+P44+P34≡Pb.As the integral over coordinates ranges from negative infinite to positive infinite,the contribution of the bound state is negligible,thus the single-photon scattering coefficients are the key to maximize the routing probability.When the energy of each incident photon is equal to the bare energy separation of the qubit(vk=vk′= Ω), the detection probability has a very simple expression
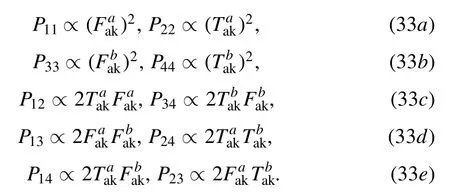
From the discussion in section 2, the routing probability Pb=1 can be achieved if a single photon is fully routed which is achieved at γb=γar=γ/2.Furthermore,the photonpair is directed to port 3(4)with unity when γbr(γbl)=0 since only left-going (right-going) modes interact with the qubit.
5.Discussion and conclusion
We have studied the coherent scattering process of few photons in two waveguide modes chirally coupled to a qubit.The output states of the photons are derived analytically in realspace for an incident one-and two-photon state after scattering off the qubit by introducing the scatter-free channel and the controllable channel.(1)In the one-photon case,the scattering coefficients are obtained.The properties of nonreciprocity are discussed.It is found that the maximum probability for the photon to be routed is 50%in the nonreciprocal case,however,the photon can be directed from the incident waveguide to an arbitrarily selected port of the other waveguide with unity in the reciprocal case.(2) In the two-photon case, the wavefunctions to each port are obtained.By notice that the probabilities for two photons appearing to the same port and the same side of the qubit are determined only by the relative coordinate of the two photons, and the probabilities for two photons traveling to different sides of the qubit are determined only by the center-of-mass coordinate, we found that photons which changing the initial propagating direction to the same port only display anti-bunching effect, the photon-pair transmitted in the incident waveguide can change from bunching to antibunching.Although two photons cannot be simultaneously routed to the same port since the qubit can only absorb one photon and emit it later once,the qubit can direct two photons from one port of the incident waveguide to an arbitrarily selected port of the other waveguide with unity,which means a single photon source can be achieved in the other waveguide.
Acknowledgments
This work was supported by NSFC Grants No.11 975 095,No.12 075 082, No.11935006, and the science and technology innovation Program of Hunan Province (Grant No.2020RC4047).
杂志排行
Communications in Theoretical Physics的其它文章
- The Sharma-Tasso-Olver-Burgers equation: its conservation laws and kink solitons
- Magnetically propelled Carreau fluid flow over penetrable sensor surface influenced by thermal radiation, Joule heating and heat generation
- Stable high-dimensional solitons in nonlocal competing cubic-quintic nonlinear media
- Effect of atmospheric turbulence on orbital angular momentum entangled state
- Persistent and enhanced spin-nematic squeezing in a spinor Bose-Einstein condensate
- Block-coherence measures and coherence measures based on positive-operator-valued measures
