Generalized boundary dilatation flux on a flexible wall

Tao Chen, Tianshu Liu

a Department of Mechanics and Aerospace Engineering, Southern University of Science and Technology, Shenzhen 518055, China

b Department of Mechanical and Aerospace Engineering, Western Michigan University, Kalamazoo, Michigan, 49008, USA

Keywords:Boundary dilatation flux Surface deformation Boundary vorticity dynamics Near-wall flow

ABSTRACT In this paper, by applying theoretical method to the governing equations of compressible viscous flow,we derive the theoretical formula of the boundary dilatation flux (BDF) on a flexible wall, which generalizes the most recent work of Mao et al. (Acta Mechanica Sinica 38 (2022) 321583) for a stationary wall.Different boundary sources of dilatation are explicitly identified, revealing not only the boundary generation mechanisms of vortex sound and entropy sound, but also some additional sources due to the surface vorticity, surface angular velocity, surface acceleration and surface curvature. In particular, the generation mechanism of dilatation at boundary due to the coupled divergence terms is highlighted, namely, the product of the surface velocity divergenceand the vorticity-induced skin friction divergenceThe former is attributed to the surface flexibility while the latter characterizes the footprints of near-wall coherent structures. Therefore, by properly designing the surface velocity distribution, the dilatation generation at the boundary could be controlled for practical purpose in near-wall compressible viscous flows.

Compressible viscous flow has drawn extensive attention in fluid-mechanic community due to the non-linear coupling between the transverse and longitudinal processes [1]. The former falls in the scope of vorticity and vortex dynamics, which is featured by the vorticityω≡∇×uwithubeing the velocity. The latter is characterized by the dilatationϑ≡∇·u, which is of critical importance to the understanding of aeroacoustics and gas dynamics.In the presence of the solid boundary, the viscous coupling among the two processes and the wall will greatly increase the complexity of the problem. The source of transverse field at solid boundary was already studied through the boundary vorticity dynamics for both the incompressible and compressible flows [1–12]. Compared to the role of the boundary vorticity flux (BVF) [2–4,11] or the boundary enstrophy flux (BEF) [1,5–7,9,13] in the dynamics of transverse field, the longitudinal-field dynamics is much more complicated. The source of longitudinal field at solid boundary was only studied by Mao et al. [14] in a recent paper, which was shown to be caused by the on-wall kinematic and dynamic coupling of longitudinal and transverse processes. The main theoretical progresses are briefly reviewed as follows.

Mao et al. [14] pointed out that the boundary dynamics of longitudinal field had a two-level structure. The first level concentrates on the boundary dilatation and the fluxes of thermodynamic variables, which can be obtained by applying the continuity equation and the momentum equation to the boundary. It represents the boundary coupling of longitudinal and transverse processes from the longitudinal side. The second level focuses on the boundary dilatation flux (BDF), which can be evaluated by taking the wall-normal derivative of the continuity equation and applying the results to the wall. It is found that the BDF neatly represents the production of vortex sound and entropy sound at the boundary,which demonstrates its critical role in longitudinal-field dynamics.Interestingly, they found that the mutual generation mechanism of the longitudinal and transverse fields on the boundary did not occur symmetrically at the same level, but appeared along a zigzag route. Specifically, the surface pressure gradient generates vorticity unidirectionally at the first level; while at the second level, it is the vorticity that generates dilatation unidirectionally.

However, the theoretical results derived by Mao et al. [14] are restricted to a stationary wall, where the movement and deformation of the boundary are not involved. Motivated by their work,the present paper attempts to generalize the BDF to a flexible wall(arbitrarily moving and deforming) by using theoretical approach.

whereμis the dynamic viscosity,μbis the bulk viscosity andκis the thermal conductivity coefficient.μϑ=μb+4μ/3 is the longitudinal viscosity.Φ≡σ:S=μω2+μϑϑ2−2μ∇·(B·u)is the viscous dissipation rate, whereB≡ϑI−∇uTis the surface rate-ofdeformation tensor [15,16]. The corresponding kinematic viscosities areν=μ/ρ,νb=μb/ρandνϑ=μϑ/ρ, respectively. All the viscosities mentioned and the thermal conductivityκare reasonably assumed as constants under the linear diffusion approximation.

By using the fundamental differential equation of thermodynamics for the entropys(Tds=de+pd(1/ρ)), one can obtain the entropy equation as

Equation (4) implies two sources to generate entropy. The first source is the viscous dissipation rateΦ, which irreversibly transfers the kinetic energy to the internal energy. The second source is the heat conduction as described by the divergence of the heat flux field (namely, the term −∇·q). Interestingly, the reversible pressure-dilatation coupling termpϑis responsible for the exchange between the kinetic energy (ρu2/2) and the internal energy(ρe), which is absent in the entropy equation.

The no-slip and no-penetration boundary conditions are considered on an arbitrarily deformable wall∂B, that is,

whereUis the known velocity of the solid boundary andr∂Bis the spatial position vector of the point on the boundary. The unit normal vector of the wall∂Bis denoted byn(without specific definition off the wall), which orients from the wall to the fluid. A physical quantity with the subscript∂Bdenotes its value at the wall. Usually, two types of temperature boundary conditions can be considered for temperature: [∂T/∂n]∂B=0 (adiabatic wall) and∇∂BT∂B=0 (isothermal wall).

The tangential surface gradient operator is defined as ∇∂B≡P·∇, whereP≡I−nnis the tangential projection tensor,Iis the unit tensor and ∇is three-dimensional spatial gradient operator.The surface Laplacian operator is defined as ∇2∂B≡∇∂B·∇∂B. The surface curvature tensor is denoted asK=−∇∂Bn, whose tracetr(K)is twice the mean surface curvatureH, namely,tr(K)=−∇∂B·n=2H=κ1+κ2, whereκ1andκ2represent the two principal curvatures of the surface. Obviously,Kvanishes for a flat surface. In addition, through orthogonal projection with respect to the tangent plane of the surface∂B, any vectorξcan be split asξ=ξπ+ξnn, whereξπandξnstand for its tangential and wallnormal components, respectively.

As a generalization of Caswell’s work for a stationary wall [17],Wu et al. [16] proposed an intrinsic decomposition of strain rate tensor on an arbitrarily moving and deforming wall as

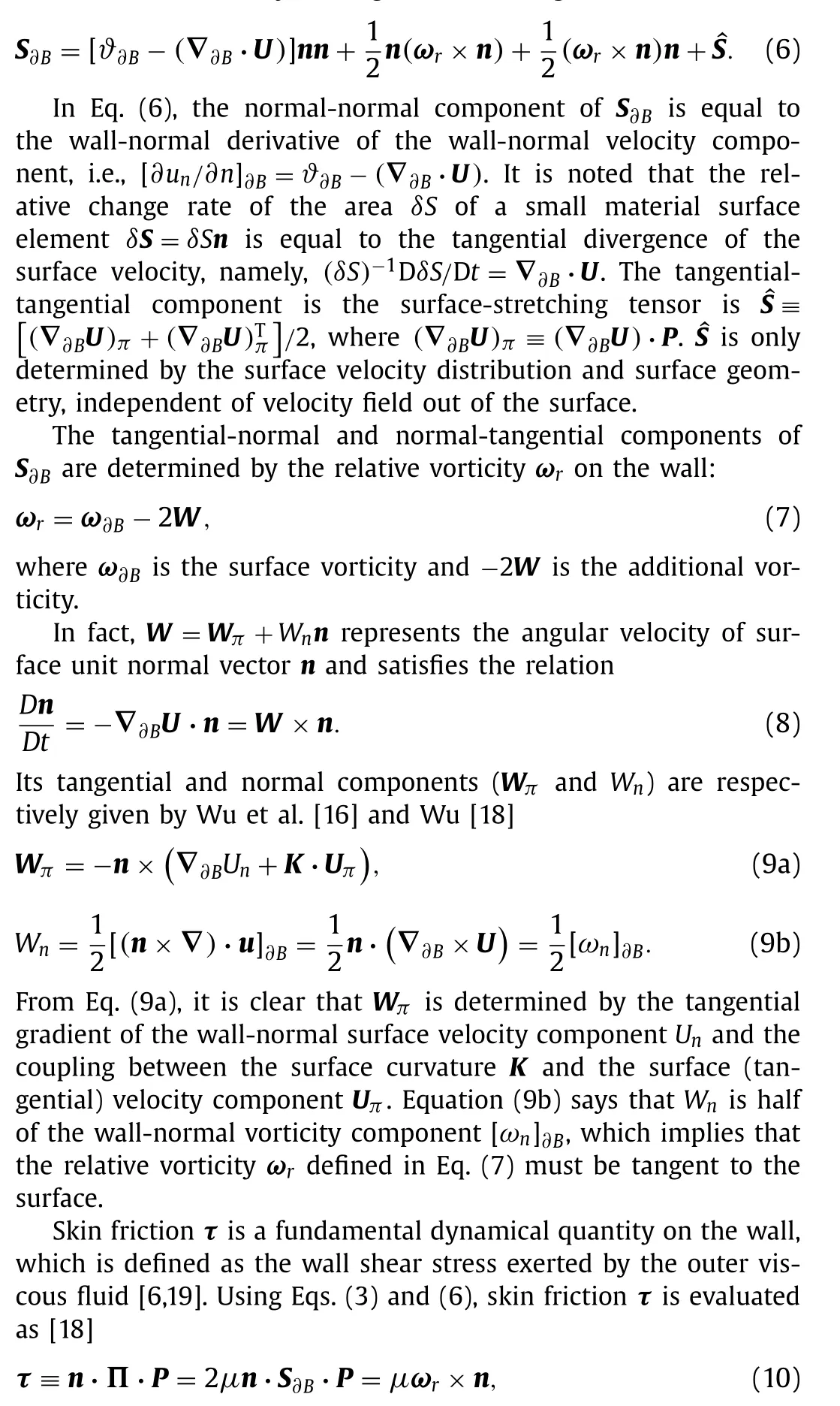
which indicates thatτis determined by the relative vorticityωrcoupled with the dynamic viscosityμ. It is noted that skin frictionτand surface relative vorticityωr(rather thanω∂B) form an orthogonal pair on a deformable wall.

For the sake of the following discussion, we prefer to rewrite Eq. (10) as

whereτωis the vorticity-induced skin friction.τWis the additional skin friction due to the local surface angular velocity, which originates from the tangential component of the surface deformation stressts=−2μn·B∂B=2μ(n×∇∂B)×U.tsis interpreted as a viscous resistance to the variation of the direction and area of the surface elementδS[16,18].

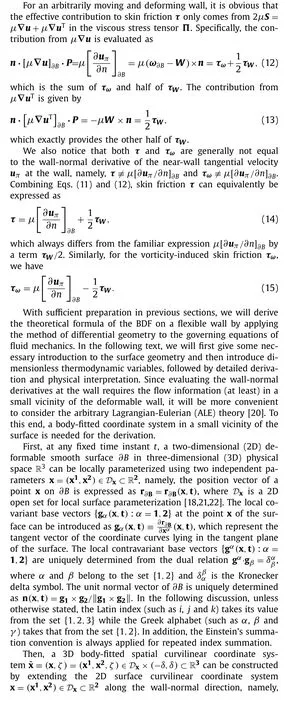
The choice of two independent thermodynamic variables were discussed by Mao et al. [14] in detail, followed by some estimations on the entropy variation for the shear layer and shock wave at high Reynolds number. For unbounded compressible flow,Pands*are chosen as the two independent thermodynamic variables, sinces*is a by-product of viscous heat-conducting flow (see Eq. (20c)) and its variation is small at large Reynolds number. For wall-bounded compressible flow, the entropy variation may not be small and the thermal boundary conditions are usually specified through the temperatureT. Therefore,PandTare more appropriate for wallbounded cases.

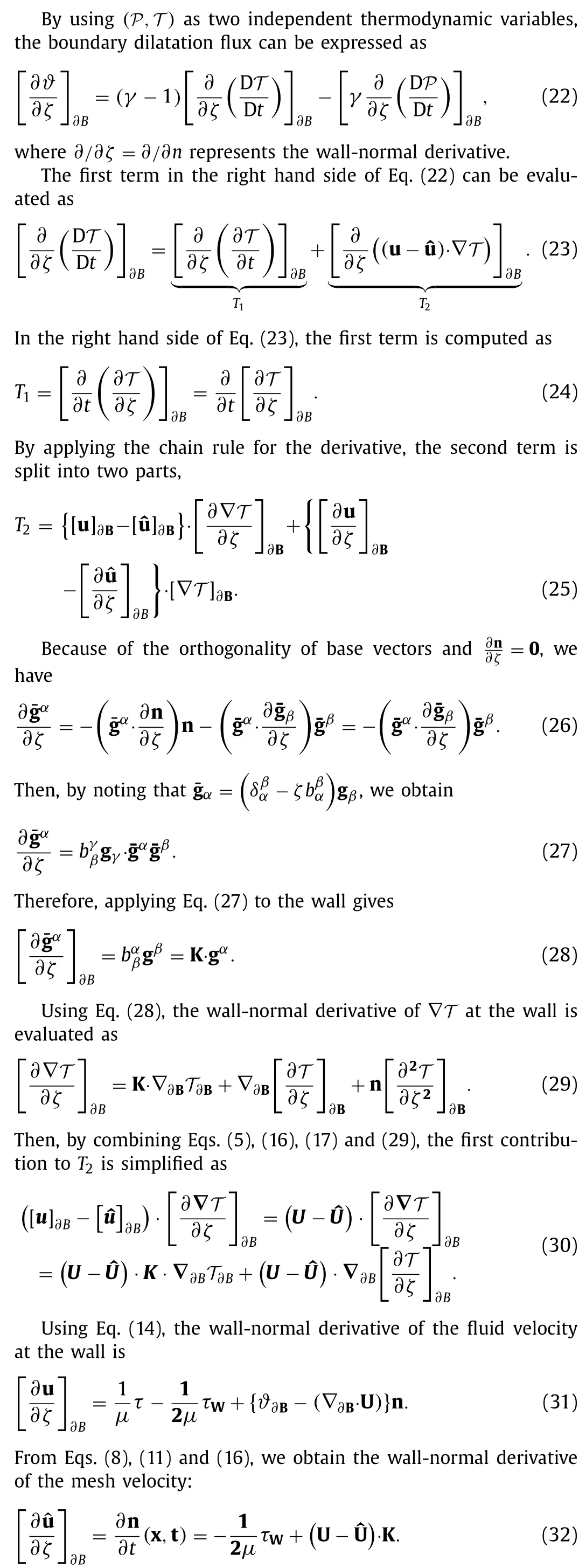
In other words, the restriction of the material derivative of Q at the wall∂Bis equal to the material derivative of the restricted field Q∂B (namely, the surface material derivative for short). Particularly,if Q is specified as the fluid velocity u, then the continuity of the fluid acceleration at the solid boundary readily follows as a corollary. It should be claimed that the spatial variable of the temporal partial derivative is the body-fitted coordinate x=(x1,x2)rather than the fixed Eulerian coordinate r=(X,Y,Z).

By combining Eqs. (24), (34) and (35), we arrive at

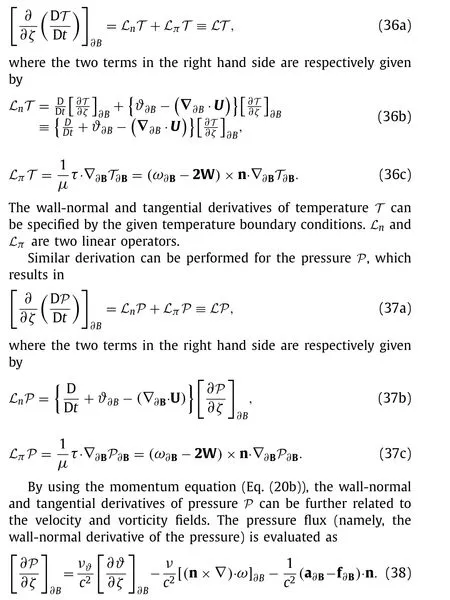
Then, by using the relation ∇∂B×n=0, the second term in the right hand side of Eq. (38) is simplified as

Recall that according to Lighthill-Panton-Wu’s definition [2,4,25], the boundary vorticity flux (BVF) is defined asσ≡ν[∂ω/∂ζ]∂B. A general expression for the BVF is obtained by Wu and Wu [4] as

respectively. Here,σ≡ν[∂ω/∂ζ]∂B andfΩ≡ν[∂Ω/∂ζ]∂B=ω∂B·σare respectively the boundary vorticity flux (BVF) and the boundary enstrophy flux (BEF) in transverse boundary dynamics.

For Eq. (45e), the first term represents the variation of the boundary dilatation along the skin friction line. The second term denotes the boundary production of the vortex sound by the BEFfΩ. Interestingly, the vortex sound can also be generated via the viscous coupling between the surface angular velocity W and the BVFσon a flexible wall. Other generation mechanisms of dilatation at boundary can be attributed to the non-vanishing wall-normal vorticity componentωnand its tangential gradient,quadratic coupling between the surface vorticityω∂B(or the angular velocityW) and the curvature tensorK, and the coupling between skin frictionτand surface accelerationa∂B(or body forcef∂B).

BDF is the core of the boundary dynamics of longitudinal field at the second level, whose sources at the boundary are caused by the on-wall viscous coupling of longitudinal and transverse processes. Generalized formula of the BDF [∂ϑ/∂ζ]∂Bon an arbitrarily moving and deforming wall is derived by applying the theoretical method to the governing equations of compressible viscous flow,which generalizes the study of Mao et al. [14] for a stationary wall.Different generation mechanisms (or sources) of dilatation at solid boundary are explicitly elucidated with special attention on physical effects caused by the surface flexibility. It is worth pointing out that from the perspective of flow control, the generation of dilatation at solid boundary can be influenced and controlled through the design of the motion of the wall.

Declaration of Competing Interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.

Acknowledgments

This research did not receive any specific grant from funding agencies in the public, commercial, or not-for-profit sectors. The authors thank Prof. Jie-Zhi Wu from Peking University for sharing the preprint of their accepted manuscript (Ref. [14]) on the boundary dilatation flux, which motivates the writing of the present paper.

Appendix A. Simplified boundary dilatation flux for a stationary wall

For a stationary wall without body force and acceleration, by choosing the coordinate system fixed on the wall, the source terms of dilatation in Eqs. (45b)– (45e) reduce to

Equations (A1a) – (A1d) are essentially the same as those obtained by Mao et al. [14] except that some terms are directly expressed in terms of skin frictionτ(a dynamical quantity) instead of vorticityω∂B(a kinematic quantity).