RESEARCH ANNOUNCEMENTS ON“MODERATE DEVIATIONS FOR GRENANDER ESTIMATOR NEARBOUNDARIES OF THE SUPPORT”
2022-02-11GAOFuqingJIANGHuiZHAOXingqiu
GAO Fu-qing,JIANG Hui,ZHAO Xing-qiu
(1.School of Mathematics and Statistics,Wuhan University,Wuhan 430072,China)
(2.School of Mathematics,Nanjing University of Aeronautics and Astronautics,Nanjing 210016,China)
(3.Department of Applied Mathematics,Hong Kong Polytechnic University,Hong Kong 999077,China)
1 Introduction and Main Results
Let f be a decreasing density with support[0,∞).Denote by Fnthe empirical distribution function of a sample X1,...,Xnfrom f.Letbe the concave majorant of Fnon[0,∞),i.e.the smallest concave function such that

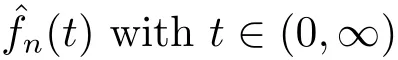


It should be noted that the Grenander estimatoris not consistent at boundaries([1],[10]).This phenomenon has great influences on the global measures of deviation,such as the Lk-distances with k>1([2],[7])and L∞-distance([3]),because the inconsistency at the boundaries will dominate the convergence.

where c,α>0,0<α<1.To be explicit,for the left boundary zero,suppose the following conditions hold:
(C1)0 < f(0)=limt↓0f(t)< ∞;
(C2)there exists some positive constant ε0such that f has k-th order continuous derivative in(0,ε0]and f(ε0)/=0.Moreover 0 < |f(k)(0)| ≤ supt≥0|f(k)(t)| < ∞,with f(k)(0)=limt↓0f(k)(t)and f(i)(0)=0 for 1 ≤ i≤ k-1.

Moreover,in the case of 1/(2k+1)<α<1,
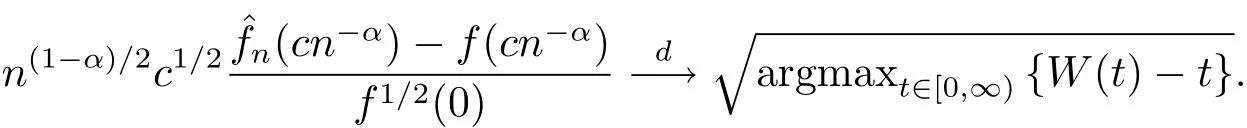
In this paper,the moderate deviations ofˆfn(cn-α)-f(cn-α)in the above two cases(0<α<1/(2k+1)and 1/(2k+1)<α<1)will be considered.By using strong approximation technique and comparison method,we obtain the following main results.
Theorem 1.1When 0<α<1/(2k+1),let‘nsatisfy as n→∞
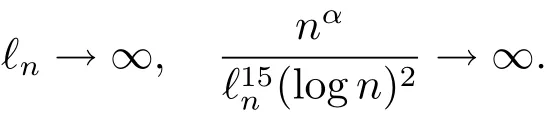
Then,under conditions(C1)and(C2),the sequence

satisfies the moderate deviations inwith speedand rate function,that is,for any open subset G of,

and for any closed subset F of,
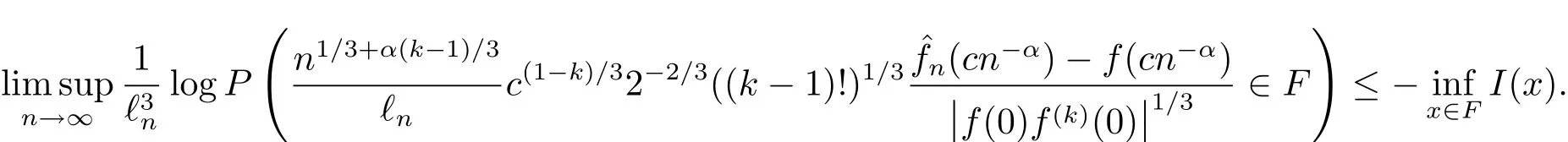
Remark 1For any x>0,by Theorem 1.1,we have

Theorem 1.2When 1/(2k+1)< α < 1,let{λn}satisfy that

Then,under conditions(C1)and(C2),the sequence
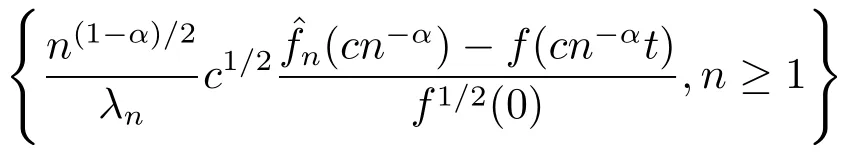
satisfies the moderate deviations inwith speedand rate function
Remark 2For any x>0,by Theorem 1.2,we have

Remark 3If f has compact support,without loss of generality,assume it is the interval[0,1].The moderate deviations ofnear the right boundary 1(similar to Theorem 1.1 and Theorem 1.2)can also be obtained,and the details are omitted here.
For a detail study of the moderate deviations for Grenander estimator near boundaries,please refer to[5].
杂志排行
数学杂志的其它文章
- ON REFINED YOUNG’S REVERSE INEQUALITIES FOR POSITIVE LINEAR OPERATORS
- SYMPLECTIC CRITICAL SURFACES WITH CIRCULAR ELLIPSE OF CURVATURE IN TWO-DIMENSIONAL COMPLEX SPACE FORMS
- STUDY ON 2-DIMENSIONAL SUBMANIFOLDS WITH CONSTANT DETERMINANT OF BLASCHKE TENSOR
- THE SPREADING SPEED FOR A NONLOCAL DIFFUSIVE PREDATOR-PREY MODEL WITH ONE PREDATOR AND TWO PREYS
- ON SPLIT REGULAR BIHOM-LIE COLOR ALGEBRAS
- RESEARCH ANNOUNCEMENTS ON“UPPER BOUND ESTIMATES OF EIGENVALUES FOR HRMANDER OPERATORS ON NON-EQUIREGULAR SUB-RIEMANNIAN MANIFOLDS”
