Research on phase shift characteristics of electromagnetic wave in plasma
2021-07-07ZhaoyingWANG王召迎LixinGUO郭立新andJiangtingLI李江挺
Zhaoying WANG(王召迎),Lixin GUO(郭立新)and Jiangting LI(李江挺)
School of Physics and Optoelectronic Engineering,Xidian University,Xi’an 710071,People’s Republic of China
Abstract The phase shift characteristics reflect the state change of electromagnetic wave in plasma sheath and can be used to reveal deeply the action mechanism between electromagnetic wave and plasma sheath.In this paper,the phase shift characteristics of electromagnetic wave propagation in plasma were investigated.Firstly,the impact factors of phase shift including electron density,collision frequency and incident frequency were discussed.Then,the plasma with different electron density distribution profiles were employed to investigate the influence on the phase shift characteristics.In a real case,the plasma sheath around the hypersonic vehicle will affect and even break down the communication.Based on the hypersonic vehicle model,we studied the electromagnetic wave phase shift under different flight altitude,speed,and attack angle.The results indicate that the phase shift is inversely proportional to the flight altitude and positively proportional to the flight speed and attack angle.Our work provides a theoretical guidance for the further research of phase shift characteristics and parameters inversion in plasma.
Keywords:electromagnetic propagation,electron density,phase shift,plasma sheath,propagation characteristic
1.Introduction
The re-entry of a high-speed vehicle will lead to extreme friction between the body and dense air,which will form a high-temperature and high-pressure plasma sheath.The plasma sheath wrapped around the aircraft contains many free electrons,ions,neutral molecules,as well as ablation particles[1–4].This shell will result in the so called ‘black barrier’phenomenon,which affects the radar detection and even breaks down the communications between aircraft and grounding monitoring stations[5].In order to solve the above problems effectively,the mechanism of interaction between plasma and electromagnetic(EM)wave is very needed to be investigated,and it has become a hot research topic in recent years.
At present,most of the works focus on the propagation and the scattering characteristics of EM wave in plasma.Jiaet al[6]derived the dielectric relationships and numerically calculated the propagation properties of the EM wave in fully ionized dusty plasma based on the Boltzmann distribution law.Liet al[7]proposed a technique of shift operator discontinuous Galerkin time-domain to analyze the EM wave propagation characteristics of metallic blunt cone aircraft coated plasma sheath.Yuanet al[8]applied a finitedifference time-domain(FDTD)method to model the terahertz wave propagation in a high-temperature unmagnetized plasma.Liet al[9]studied the effect of charged dust particle and their size distribution on the propagation of the EM wave in dusty plasma.Zhanget al[10]used a two-dimensional FDTD model to simulate EM wave propagation properties in the plasma sheath.Wanget al[11]modified the dielectric constant model of weakly ionized dust plasma and analyzed the influence of dust parameters on EM wave propagation by the scattering matrix method(SMM).Zhanget al[12]analyzed the absorption,transmittance,and reflectance of terahertz waves in magnetized plasma by using the layered SMM.Songet al[13]introduced alternating direction implicit technology into the high order auxiliary differential equation(ADE)FDTD method for unmagnetized plasma and analyzed the propagation and scattering characteristics of EM wave in the plasma sheath.Liuet al[14]deduced the Boltzmann FDTD iteration formula for EM wave obliquely incident into plasma under a one-dimensional case and calculated the reflection and transmission coefficients of the plasma with different incident angles.By using the ADEFDTD method,Guoet al[15]investigated the scattering effect of EM waves in dusty plasma sheath and analyzed backward radar cross-sectional values of various parameters in time and space inhomogeneous plasma sheath.Liuet al[16]studied the scattering characteristics of a blunt cone with electrically large size covered by inhomogeneous plasma sheath by using physical optics method.Brittet al[17]used the finite-difference techniques in conjunction with pulse response techniques to calculate the pulse response and scattering cross section as a function of frequency for various objects.Chenet al[18]used the FDTD algorithm to investigate the effects of EM wave scattering in three-dimensional time-varying and spatially nonuniform plasma.
In addition,there are also many researchers interested in the parameter inversion of plasma.Donatoet al[19]proposed first-order linearized approaches to solve the one-dimensional single-view multifrequency EM inverse scattering problem through the sparse recovery optimization provided by the prominent compressive sensing theory.Joneset al[20]estimated electron densities in a plasma column from measurements of phase shift in the radiation scattered at various angles.Fenget al[21]researched the inversion of the resonant frequency of cold unmagnetized plasma by combining the current density Laplace transform FDTD method with the particle swarm optimization algorithm based on Fourier series expansion.The purpose of these studies is aimed to understand the interaction between plasma and EM wave,and determine the range and correlation of characteristic parameters in plasma,and finally provide a reliable basis for theoretical and ground experimental research.
In the study of electron density inversion,microwave interferometer has a wide range of applications,because it is simple to operate and easy to realize,and is popular[22,23].Its application principle is to measure the phase of EM wave passing through the plasma,and to obtain the phase shift compared with the phase without plasma.Through the relationship between phase shift and electron density,the value of electron density can be obtained indirectly.However,the phase shift characteristics of EM wave in plasma are not the focus and have not been deeply studied[24].Thus,the correlation between phase shift and EM wave parameters should be investigated in detail.This can provide a comprehensive understanding about the action mechanism of plasma on EM wave,and can also provide a useful guidance for the inversion of parameters.
In this paper,we mainly analyzed the influence of plasma and EM parameters on phase shift characteristics.Section 2 describes the basis theory of EM wave propagation in plasma.In section 3,the influences of EM wave frequency,electron density and collision frequency on phase shift were discussed firstly.Then,the effect of different electron density distribution types on EM wave phase shift was investigated.Finally,the phase shift of EM wave was analyzed in detail under different flight conditions(flight altitude,speed,and attack angle).Section 4 is a brief summary of this study.
2.The foundamental theory
The dielectric parameter in the plasma can be described as[25]
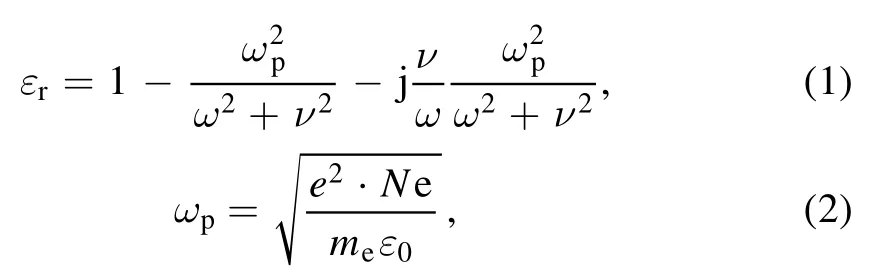
where ωp,ν and ω are the plasma,collision and incident frequency,respectively.eandmeare the charge and mass of an electron.Ne denotes electron density.ε0is the permittivity of vacuum.
The attenuation constant α[26]and phase constant β[27]of EM wave propagating in plasma are as follows:

Therefore,the phase shift(Δφ)of EM passing through the plasma and vacuum can be expressed as:
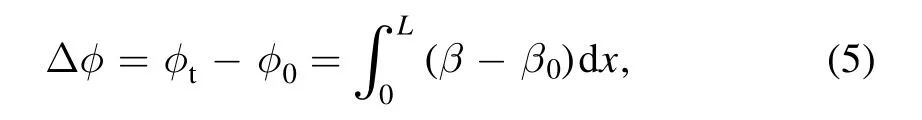
whereφtis the phase of the electromagnetic wave passing through the plasma with thicknessL,φ0is the phase of the electromagnetic wave generated through a vacuum with the same thickness,β0=ω/cis the phase of electromagnetic waves in vacuum andcis the speed of light in vacuum.
3.Results and discussion
3.1.Relationship among phase shift,incident frequency,collision frequency and plasma frequency
Figure 1(a)shows the relationship among phase shift,incident wave frequency and collision frequency.It is found that the phase shift of EM wave increases first and then decreases with the increase of collision frequency in the low frequency domain,and increases gradually with the increase of collision frequency in high frequency domain,and then the variation range is smaller and smaller,even insensitive to the change of collision frequency.For the change of incident EM wave frequency,the phase shift decreases first and then increases with the increase of EM wave frequency.Meanwhile,the phase shift curve appears valley value,and the phase shift is the negative maximum value,which indicates that the phase lag of EM wave passing through plasma is the most serious at this position.The simulation results show that the incident wave frequency corresponding to the phase shift valley value is about 28 GHz,which is approximate to the plasma frequency.To a certain extent,it is shown that the electron density of plasma plays an important role in the process of phase shift produced by EM wave through plasma.In order to further illustrate the relationship among the plasma frequency,the incident frequency and the phase shift,the following simulation analysis is carried out.
Figure 1(b)shows the relationship among the phase shift of EM wave through plasma,the frequency of incident wave and plasma frequency.The phase shift decreases at first and then increases with the increase of plasma frequency for a given frequency.The valley of phase shift curve gradually shifts to the higher plasma frequency region with increasing of incident wave frequency,and the minimum phase shift is getting smaller and smaller.In addition,we find that the minimum phase shift occurs when the incident wave frequency is equal to the plasma frequency,and the change of plasma frequency leads to the position where the minimum phase shift occurs.Combined with figure 1,we find that when the electron density changes,the phase shift valley value will shift to the corresponding frequency,which proves the correctness of the above conclusion again.
Figure 1(c)shows the variation of the phase shift as a function of the plasma frequency and the collision frequency for a fixed incident frequency.The overall phase shift increases with the increasing of collision frequency for a given plasma frequency.The change of phase shift with the increase of collision frequency is greater for the increasing plasma frequency(that is,the steeper the change trend of phase shift curve).The phase shift decreases first and then increases with the increasing of plasma frequency for a given collision frequency.The phase shift increases gradually with the increasing of collision frequency.It is interesting that the corresponding plasma frequency of valley value in phase shift does not change with the increase of collision frequency.To further illustrate this relationship,we add the following two graphs to explain.

Figure 1.The phase shift(Δφ)as a function of(a)incident frequency(f)and collision frequency(ν),(b)incident frequency(f)and plasma frequency(ωp),and(c)collision frequency(ν)and plasma frequency(ωp).
As can be seen from figure 2(a),for a given incident frequency,the corresponding frequency of the valley of the phase shift curve does not change regardless of the collision frequency.The phase shift increases with the increase of the collision frequency.The calculated influence of different incident frequencies on phase shift as function of plasma frequency was shown in figure 2(b).The results indicate that the phase shift first decreases and then increases with increasing of plasma frequency for a given incident frequency.When the incident wave frequency is changed,the valley position is shifted to the larger plasma frequency,and the higher the incident wave frequency,the more obvious the change of phase shift with the plasma frequency is.As a result,the phase of the EM wave transited from advance to lag after passing through the plasma.
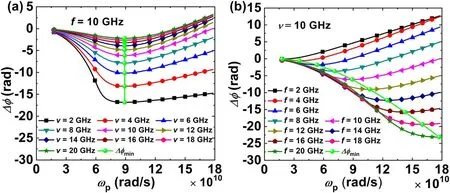
Figure 2.The influence of different parameters on phase shift:(a)different collision frequency(ν)and(b)different incident frequency(f)as a function of plasma frequency(ωp).
3.2.Influence of different electron density distributions on phase shift
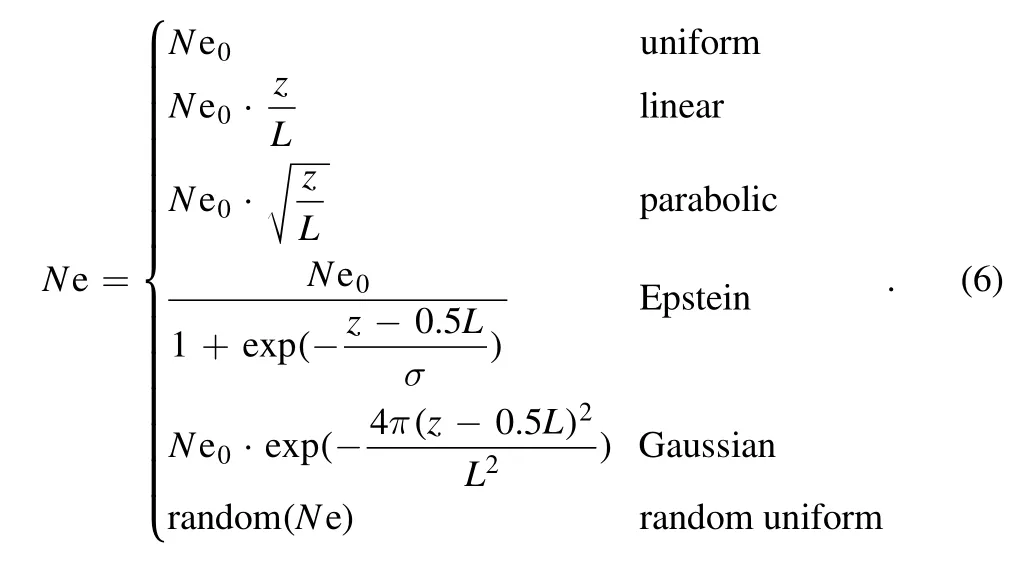
The commonly used spatial distribution functions of electron density are shown in equation(6).Ne0=1.0×1019m-3.zis the spatial position along the plasma thickness.Lis the thickness of plasma.Figure 3(a)shows six different types of electron density distributions considered in our simulations,including uniform distribution,linear distribution,parabolic distribution,Epstein distribution,Gaussian distribution,and random uniform distribution.Figure 3(b)shows the phase shift of EM passing through plasma within different electron density spatial distributions.It can be observed from figure 3(b)that the phase shift of uniform distribution is larger than that in parabolic distribution,while the linear,Epstein,Gaussian,and random uniform distributions are very close to each other.This is because among the six different electron density distributions,the uniformly distributed electron density is always in themaximum state,and it has the significant influence on the phase of EM,results in the larger phase shift.The mean values of the electron density were obtained for the other five distributions,the parabolic distribution results in a larger mean value than the remaining four types of distributions,which are close especially in the higher frequency,as shown in figure 3(b).Thus,the phase shift is related to the mean electron density of different electron density distributions.
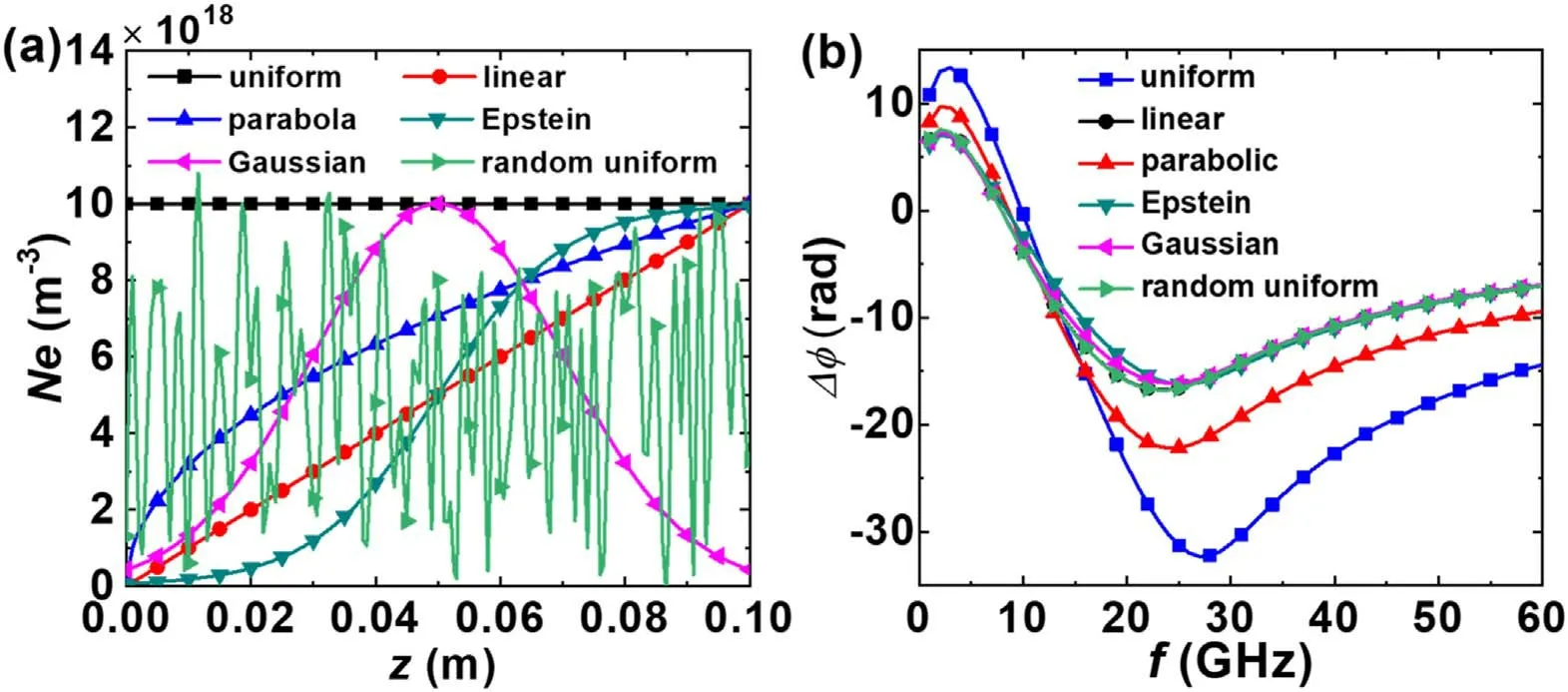
Figure 3.(a)The spatial distributions of electron density(Ne)with different distribution types and different positions(z)of plasma sheath.(b)Effect of different electron density distributions on phase shift(Δφ)as a function of incident frequency(f).
3.3.The phase shift of EM wave under different flight conditions
Due to the change of flight altitude,flight speed and attack angle in the process of re-entering the atmosphere,it is inevitable to discuss the influence of plasma on the phase shift of EM wave under different flight conditions.Schematic diagram of the hypersonic vehicle model is presented in figure 4(a).The total length,the head radius,and the tail height of the model are 2.0 m,0.0225 m,and 0.3 m,respectively.The flow fields around hypersonic vehicle model under different flight conditions are shown in figures 4(b)–(h).Assuming that the antenna is located on the upper surface of the hypersonic vehicle,x=1,the electron density at this position under different flight conditions was extracted and substituted into equation(2)to obtain the plasma frequency.Then substituting the obtained plasma frequency into equation(4),the electromagnetic wave phase coefficient in plasma can be acquired.Finally,the phase shift of electromagnetic wave through plasma can be obtained by using equation(5).The considered conditions for the simulations are shown in table 1.

Figure 4.(a)Schematic diagram of the hypersonic vehicle model.(b)–(h)Flow fields of plasma sheath under different flight conditions.
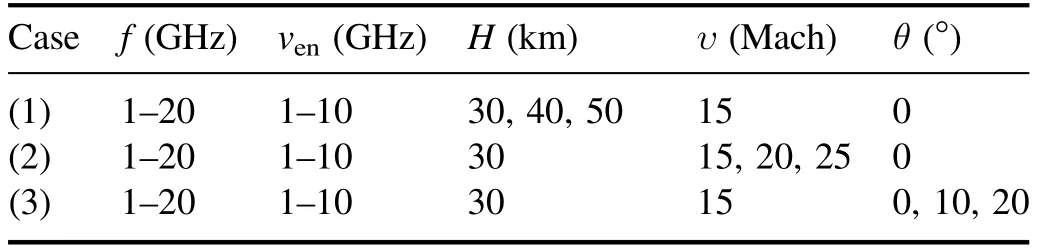
Table 1.The considered flight conditions in our calculations,including plasma frequency,collision frequency,flight altitude,speed,and attack angle.
3.3.1.The phase shift of EM wave under different flight altitudes.Figure 5 shows the phase shift of EM wave passing through plasma under different flight altitudes.The change of phase shift decreases gradually with increasing of the flight altitude.This is because with the increase of flight altitude,the air becomes thinner and thinner.Then the reduction of the air which can be ionized results in the decrease of electron density in plasma.The low electron density at higher flight altitude weakened the interaction between EM wave and plasma,results in the decrease of phase shift.In addition,the corresponding frequency of the maximum phase shift change is close to the collision frequency(~5 GHz),which indicates that when the incident frequency of EM wave is gradually close to the collision frequency,it will lead to a significant effect on the change of phase,especially around the collision frequency,as shown in figure 5(d).

Figure 5.The phase shift as functions of incident and collision frequency under different flight altitudes.(a)30 km,(b)40 km and(c)50 km,(d)comparison of the phase shifts as a function of incident frequency with different flight altitudes and constant collision frequency.
3.3.2.The phase shift of EM wave under different flight speeds.Figure 6 shows the phase shift of EM wave passing through plasma within different flight speeds.The phase shift increases gradually with the increase of the flight speed,which results from the higher speed of flight and the stronger friction around the flight surface.The surrounding air heats up and the air ionization degree increases,which leads to the sharp increase of electron density in the plasma.Therefore,the interaction between EM wave and plasma enhanced,resulting in an increase in the phase shift of the EM wave,as shown in figure 6(c).
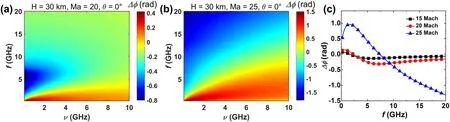
Figure 6.The phase shift as functions of incident and collision frequency under different flight speeds.(a)20 Mach,(b)25 Mach and(c)comparison of the phase shift as a function of incident frequency with different speeds and constant collision frequency.
3.3.3.The phase shift of EM wave under different attack angles.Figure 7 shows the phase shift of EM wave passing through plasma within different attack angles.The phase shift increases gradually with the increasing of attack angle.Due to the increase of the attack angle and the specific aerodynamic shape of the aircraft,the thickness of the plasma layer formed on the upper surface of aircraft increased.At this time,the EM wave is incident into the plasma,the interaction time between the two is increased,and the phase shift of the EM wave is further affected,so that the phase shift change of the EM wave is increased,as shown in figure 7(c).
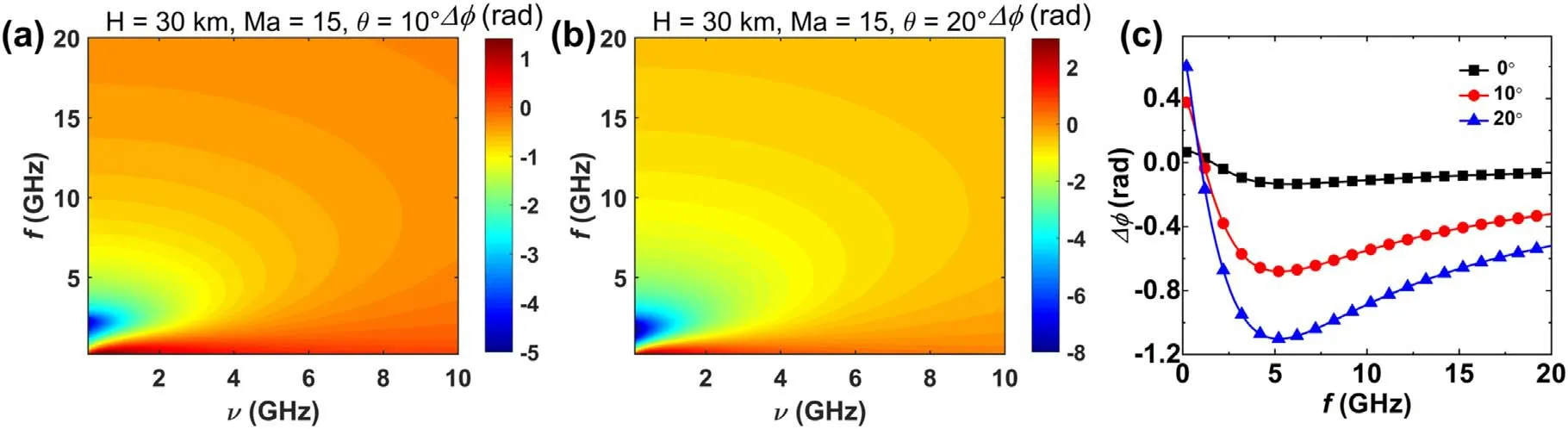
Figure 7.The phase shift as functions of incident and collision frequency within different attack angles.(a)10°,(b)20°,and(c)comparison of the phase shift as a function of incident frequency with different attack angles and constant collision frequency.
4.Conclusions
The phase is a very important parameter of the EM wave,which can reflect the state of the EM wave at any time.When the EM wave passes through the medium,it will have a certain influence on the phase.Thus,the study of the phase shift characteristics of EM wave after passing through the plasma is helpful to understand the action mechanism between plasma and EM wave.In this work,we first studied the relationship between the phase shift characteristics of EM waves in plasma and electron density,collision frequency and incident frequency,which reveals the internal relationship between phase shift and plasma parameters.Then we explored the influence of different electron density distribution on the phase shift of EM wave,and found that the phase shift of the EM wave is independent of the electron density distribution but associate to the average electron density.Finally,the phase shift characteristics of EM wave in the high-speed target plasma sheath were studied.The phase shift characteristics were investigated from three aspects:different flight altitudes,flight speeds and attack angles.The results showed that the phase shift is inversely proportional to the flight altitude and positively proportional to the flight speed and attack angle,which is related to the electron density distribution and thickness of the plasma.This research provides a useful theoretical support for the further study of the phase shift characteristics of EM waves and parameter inversion in plasma.
Acknowledgments
The authors appreciate the Aircraft Technology Research Center of Zhejiang University for the flow field data.Project supported by National Natural Science Foundation of China(Nos.U20B2059,62071353,61627901 and 62071348),the Foundation for Innovative Research Groups of the National Natural Science Foundation of China(No.61621005),and the Key Laboratory Foundation(No.6142502190203).
杂志排行
Plasma Science and Technology的其它文章
- Rice plant growth and yield:foliar application of plasma activated water
- Three-dimensional non-equilibrium modeling of a DC multi-cathode arc plasma torch
- Magnetic field induction and magnetic force distribution profiles in plasma focus discharge device
- Comparison of sample temperature effect on femtosecond and nanosecond laser-induced breakdown spectroscopy
- An active tunable Fano switch in a plasmafilled superlattice array
- Enhanced removal of ultrafine particles from kerosene combustion using a dielectric barrier discharge reactor packed with porous alumina balls
