非线性三阶常微分方程的多点边值正解问题探索

何林海



非线性三阶常微分方程的多点边值正解问题探索

何林海

湘潭医卫职业技术学院, 湖南 湘潭 411102

针对非线性三阶常微分方程多点边值正解问题研究较少的现状，本文以锥上不动点定理为基础，构建相应的等价方程，证明非线性三阶常微分方程存在正解的可能性。计算结果表明：在Banach空间的锥中，当条件（）成立，若（1）成立，则至少存在3个正解；若条件（2）成立，则至少存在2个正解；若条件（3）、（4）成立，则存在至少1个正解。相对于已有文献的研究结果，本文的解法有一定的创新价值。

三阶常微分方程; 非线性; 多点边值; 正解

三阶常微分方程的多点边值问题在天文学、流体力学等领域广泛出现[1]。学者们对于该问题的正解研究进行了多方尝试，试图证明三阶常微分方程存在1个或多个正解，并取得丰硕的成果。根据文献[2]提出的三阶常微分方程三点边值问题，证明了至少存在3个正解[2]。文献[3]同样证明了三阶常微分方程三点边值至少存在3个正解[3]。文献[4]则通过锥上不动点定理，证明三阶常微分方程三点边值存在正解的可能性[4]。但在以上文献中，关于非线性三阶常微分方程的多点边值问题讨论较少。本文基于锥上不动点定理，建立非线性三阶常微分方程等价方程，通过计算证明非线性三阶常微分方程有1个或多个正解，得到与当前文献不同的结果，具备一定的创新价值。

1 非线性三阶常微分方程的相关定义

定义1：设为Banach实空间，的锥为Ì，称之为非空闭凸集。该定义要满足以下条件：∈，若∈，则≥0；，-∈，=0。

定义2：对于[0,1]，函数为凸泛函，满足条件如下：(1+(1-)2)≥(1)+(1-)(2),,1,2∈[0,1]

（1）若ǁAǁ≤ǁǁ，∈∂D，则i(,D)=1；

（2）若有∈{0}，令¹A+，∈∂D，>0，则i(,D)=0；

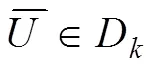
2 计算结果与证明

首先对非线性三阶常微分方程的边值问题进行考虑，有：ʹʹʹ()=()，∈[0,1] (1)

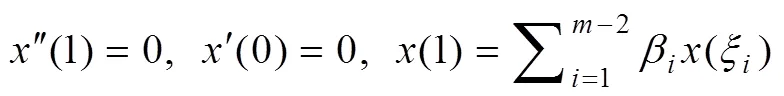
引理2：令0=0，-1=1，0=β-1=0，()∈[0,1]，式（1）（2）与下面等价：

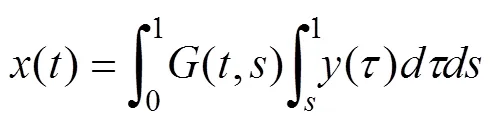
设(,)为边值的问题，则下式成立：-ʹʹ()=0 (6)

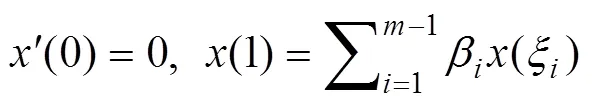
根据Green函数的定义与性质，对式（7）的边值条件进行结合，得出下式：

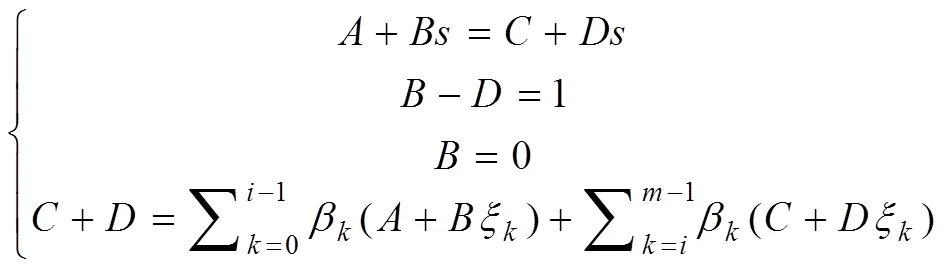
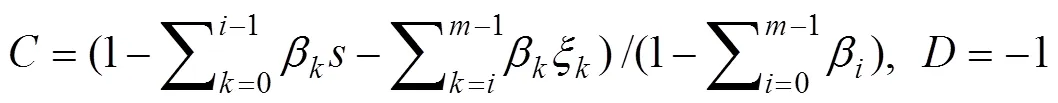
引理3：(,)满足以下条件(,)≥0,,∈[0,1]。

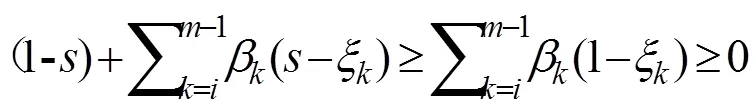
对于-1≤≤，=1,2,…,-1,≥，下式成立：

综合以上证明，(,)≥0,,∈[0,1]。

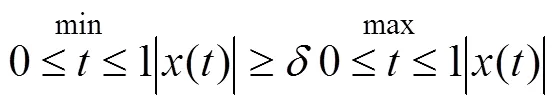
()具有凸性：((1)-(0))≤()-(0)。

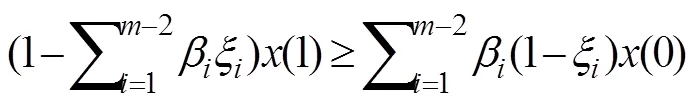
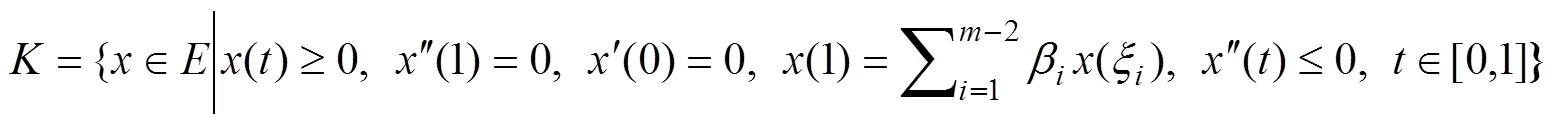
进一步计算：()=min{,1-},∈[0,1] (19)

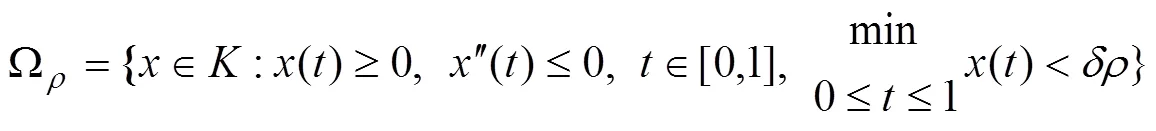
则非线性三阶常微分方程的多点边值问题在里面至少存在2个正解。

证明4：假设条件（1）成立，对辅助函数*(,)∈((0,1)×(0,∞),(0∞))进行计算：

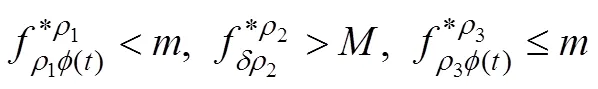
说明ǁ()ǁ≤ǁǁ，∈∂。设()≡1，∈[0,1]，则∈1。以下必成立：¹+，∈∂Ω2，≥0。否则必有：0∈∂Ω2，0≥0，得出0=0+0。但：

这样就容易验证（*）在[1(),∞]里面的正解，说明非线性三阶常微分方程的多点边值问题至少会存在3个正解。设条件（2）成立，存在的正解同样可以证明，与定理1完全相似，因此得到以下结论：

若条件（4）也成立，则（4）中有正常数1,2∈(0,∞)，并满足以下条件：1<2，令：

则说明非线性三阶常微分方程的多点边值问题至少会存在1个正解。

3 讨论

三阶常微分方程组的类型对于解法有较大的影响，而且需要掌握相关的定理。最近几年，关于非线性三阶常微分方程的边值正解问题，逐渐引起学者们的关注，但研究依然较少。Kreutz等通过莱格特·威廉姆斯定理，证明非线性三阶常微分方程的两点边值问题，结果表明存在3个正解[5]。Mukhigulashvili研究了三阶常微分方程的非线性特征值，在对该类型方程有界集的非线性特点考察的基础上，构建问题正解的存在性以及多解性[6]。Bhaumik等探究了非线性三阶常微分方程的正解，认为非线性项在有界集上的高度函数积分若恰当，则非线性三阶常微分方程至少存在1~3个正解[7]。

4 结论

常微分方程是代数领域最简单也最重要的方程组之一，三阶常微分方程被人们广泛用于解决日常问题，包括电子通信、化学反应、自动化、航空航天等领域的计算。本文在前人研究的基础上，通过锥上不动点定理的运用，对非线性三阶常微分方程的多点边值正解问题进行了探索，主要得出以下结论：在Banach空间的锥中，当条件（）成立，若（1）成立，则至少存在3个正解；若条件（2）成立，则至少存在2个正解；若条件（3）、（4）成立，则存在至少1个正解。

[1] Yebdri M. On Weak Solutions of a Functional Differential Equation[J]. International Journal of Applied Mathematics & Statistics, 2018,57(2):83-88

[2] Bhaumik P, Ghosal S. Bayesian inference for higher-order ordinary differential equation models[J]. Journal of Multivariate Analysis, 2017,157(3):103-114

[3] Haug EJ. An Ordinary Differential Equation Formulation for Multibody Dynamics: Nonholonomic Constraints[J]. Journal of Computing and Information Science in Engineering, 2016,17(1):1-13

[4] Dallas S, Machairas K, Papadopoulos E. A Comparison of Ordinary Differential Equation Solvers for Dynamical Systems With Impacts[J]. Journal of Computational and Nonlinear Dynamics, 2017,12(6):37-48

[5] Hass H, Kreutz, C, Timmer J,. Fast integration-based prediction bands for ordinary differential equation models[J]. Bioinformatics, 2016,32(8):1204-1210

[6] Mukhigulashvili S. The mixed BVP for second order nonlinear ordinary differential equation at resonance[J]. Mathematische Nachrichten, 2017,290(2):393-400

[7] Bhaumik P, Ghosal S. Efficient Bayesian estimation and uncertainty quantification in ordinary differential equation models[J]. Bernoulli: official journal of the Bernoulli Society for Mathematical Statistics and Probability, 2017,23(4):3537-3570

Exploration for Multipoint Boundary Value Positive Solutions of Nonlinear Third Order Ordinary Differential Equations

HE Lin-hai

411102,

In view of the current situation that there are few studies on positive solutions of multi-point boundary value of third-order non-linear ordinary differential equations, this paper constructs corresponding equivalent equations on the basis of fixed point theorem on cone, and proves the possibility of existence of positive solutions of third-order non-linear ordinary differential equations. The results show that:in the coneof Banach space, if condition () holds and (1) holds, there are at least three positive solutions;If condition (2) holds, there are at least two positive solutions, and if condition (3) and (4) holds, there is at least one positive solution. Compared with the research results in the existing literature, the solution in this paper has some innovative value.

Third order ordinary differential equations; nonlinear; multipoint boundary values; positive solutions

O175.14

A

1000-2324(2019)02-0346-04

10.3969/j.issn.1000-2324.2019.02.036

2018-07-10

2018-09-20

何林海(1973-),男,本科,讲师,研究方向:应用数学. E-mail:helinhai88@126.com