Impact of social relationship on firms’ sharing reward program

Wei Wei Mei Shu’e Zhong Weijun

(School of Economics and Management, Southeast University, Nanjing 211189, China)

Abstract：In order to make strategic decision on firms’ sharing reward program (SRP), a nested Stackelberg game is developed. The sharing behavior among users and the rewarding strategy of firms are modeled. The optimal sharing bonus is worked out and the impact of social relationships among customers is discussed. The results show that the higher the bonus, the more efforts the inductor is willing to make to persuade the inductee into buying. In addition, the firms should take the social relationship into consideration when setting the optimal sharing bonus. If the social relationship is weak, there is no need to adopt the SRP. Otherwise, there are two ways to reward the inductors. Also, the stronger the social relationship, the fewer the sharing bonuses that should be offered to the inductors, and the higher the expected profits. As a result, it is reasonable for the firms to implement SRPs on the social media where users are familiar with each other.

Key words：social relationship; sharing reward program; incentive strategy; social commerce

W ith the proliferation of social media such as Facebook, WeChat and Weibo, it becomes much easier for users to communicate with each other[1]. Also, firms’ social media initiatives have strengthened firm-customer interaction[2]. According to the report of iResearch, almost 79.3% of users followed business accounts on WeChat and over 88.9% of users were willing to share information with friends (http://www.iresearch.com.cn/report/2393.html). Consequently, more and more enterprises have launched sharing reward programs (SRPs) to boost sales. For instance, many vendors in Taobao.com have launched sharing reward programs to encourage customers (inductors) to share business information with friends (inductees) through social media, such as Wechat, Weibo, QQ, etc. If the inductees successfully buy the products which the inductors recommended, the inductors will receive bonuses paid by the vendors. In addition, firms like Didi, Mobike, ele.me in China also offer users Hongbao to encourage them to share business information in their friends’ circles of WeChat. Although SRPs have been adopted as an effective tool to acquire potential customers in many industries, they can also cost the firms much money since the bonuses are highly dependent on the successful purchases of the inductors[3]. Thus, it is of great significance to make managerial decisions on the optimal design of SRPs.

Our study is related to two streams of research. In the first stream, many researchers have explored the factors which may influence the users’ sharing willingness. Wirtz and Chew[4]showed that the price and the customers’ satisfaction had great impact on their sharing willingness. However, Ahrens et al.[5]proposed that the bonus difference between inductors and inductees also influenced the inductors when sharing information. Wentzel et al.[6]noted if the information contained more social emotions instead of business advertisements, users were more willing to share. Some researchers also found that users’ sharing behavior had an impact on firms’ performance. On the one hand, Armelini et al.[7]proposed that referral behavior reduced the users’ perceived risk, which improved firms’ profits. On the other hand, Tuk et al.[8]considered that sharing business information showed a lack of sincerity, which reduced the customers’ intensions.

The literature in the second stream focuses on the design of the referral reward program. Biyalogorsky et al.[9]proposed that the basis of offering a bonus was the successful purchase in referral reward programs, which might avoid inductors’ free riding. Also, the referral reward program is more effective than the low price strategy. Kornish et al.[10]considered the inductors’ satisfaction degree and provided a strategy to design reward programs. On the basis of Kornish’s study, Xiao et al.[3]took price into consideration and discussed the optimal design of reward programs. Mirzaei et al.[11]showed that compared with offering bonuses, the users on social media were more inclined to share their favorite firms’ information for free. Arbatskaya and Konishi[12]proposed that when adopting referral reward program, firms can decrease the advertisement cost.

Although there is much similarity with each other, the sharing reward program differs from the referral reward program in many aspects. For example, the SRPs are often implemented on an e-commerce platform and on social media, and are highly dependent on a successful purchase. However, as far as we have seen, little research has focused on sharing reward programs and none of them has taken both the users’ shopping behavior and social elements into consideration. By integrating the two streams’ research, we develop a nested game model based on Ref.[3] and concentrate on the scenario where only the inductors are rewarded. Our main aim is to figure out the best rewarding bonus and provide some guidance for firms when implementing SRPs on social media. Different from the previous research, we consider social media users’ social relationships when studying the SRP. Moreover, the impact of social relationships on reward strategy is discussed.

1 Sharing Reward Program Model

Consider that the market consists of two types of customers: loyal customers and potential customers. The aim of the SRP is to utilize the loyal customers to acquire potential customers. Throughout the paper, we shall use “the inductor” and “the inductee” to refer to the loyal customer and the potential customer, respectively. In addition, there is one firm in the market selling products with pricepto customers. For each customer, the evaluation of the product isV, whereV～U[0,1]. Whether the potential customers will purchase the products is dependent on product pricepand trust costh. Trust costhrepresents the time and energy customers spend in trusting the vendors because of unfamiliarity with the products[13]. WhenV≥p+h, they will make the purchase. In our model, pricepis set to be an exogenous variable for two reasons. On the one hand, firms such as Taobao will not use discriminatory pricing strategy when adopting SRPs. On the other hand, if the firms lower their regular price, the loyal customers will not have the motivation to share information for bonuses.

Meanwhile, the firm will offer the loyal customers sharing bonus to encourage them to share business information with their friends, which will enhance the purchasing possibility. In order to obtain sufficient income to support SRP, we have to assumep≥1-h. The constraint guarantees the existence of the optimal solution of the reward strategy. It also means that the firm cannot recoup the bonus cost by selling products when setting a low price. Thus, the following analysis is based on the previous assumption.

In the SRP, the firm will offer bonuses to encourage inductors to share business information and to persuade their friends to buy products. When the products are successfully purchased, the inductors will obtain the sharing bonus. Consequently, the SRP can be modeled as a nested Stackelberg game, as shown in Fig.1. The inner game is between the inductor and the inductee. For the given bonusr, the inductor who is the leader first decides whether to make an efforteto share information. Then, the inductee decides whether to purchase the products. The outer game is between the firm, the inductor and the inductee, where the firm acts as the game leader. Once the inner game reaches equilibrium, the firm can determine the optimal sharing bonusr*.

Fig.1 The structure of sharing reward game

2 Equilibrium between the Inductor and Inductee

As illustrated before, each customer will purchase the products ifV≥p+h. In the SRP, consumers’ trust costhcan be reduced by an inductor’s efforts, such as explanation and persuasion. As a result, a consumer’ trust costhcan be written as the linear function of the inductor’s efforts, which ish(e)=h0-θe. Parameterh0shows a consumer’s base trust cost andeis an inductor’s effort. Parameterθdenotes the social relationship between inductors and inductees. It is clear that the closer the inductors and inductees are, the more likely the purchasing decision will be influenced[14]. Consequently, the inductees’ purchasing possibility can be described as

P=Pr{V≥p+(h0-θe)}=(1-p-h0+θe)

(1)

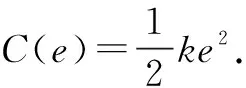
(2)

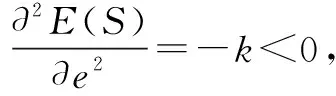
Lemma1Assuming that the sharing bonus is given, the inductor’s optimal efforts aree*=rθ/k, and the inductor’s optimal expected income isE(S*)=r(rθ2-2h0k-2kp+2k)/2k.

ProofTake the first-order derivatives ofE(S) with respect toeand we obtain ∂E(S)/∂e=rθ-ke. Let it be zero and solve the equation, then we can calculate the optimal bonuse*=rθ/k. Substitutinge*into Eq.(2), the optimal expected income can be obtained.

3 Equilibrium between the Firm and Inductor

When the inner game reaches equilibrium, we can obtain the response of the inductor and inductee to bonusr. In the outer game, the firm, who is the leader, will set the optimal bonusrbased on the inductee’s purchase possibility and the inductor’s optimal efforts. Assume that the firm makes the bonus decision at one stage, and the inductor only shares the information with one inductee. The revenue comes from the inductees’ successful purchase and the cost is the reward offered to the inductors. Then, we can obtain the expected profit function of the firm as

(3)

Meanwhile, the inductor will have the motivation to share the business information only because of his/her expected incomeE(S*)≥0. So, finding optimalrto maximize the expected profitsE[π(r)] is equivalent to solving

(4)

As it turns out,the optimal sharing bonus is dependent on the strength of the social relationship between the inductor and the inductee, which is shown in Proposition 1 and Proposition 2.

Proposition1Ifθ2<(2h0k+2kp-2k)/p, there is no need for the firm to adopt the SRP. If (2h0k+2kp-2k)/p≤θ2<(3h0k+3kp-3k)/p, the optimal bonus isr*=2k(h0+p-1)/θ2, and the optimal expected profits areE[π*(r*)]=L(pθ2-2h0k-2kp+2k)(h0+p-1)/θ2.

Proposition2Ifθ2≥(3h0k+3kp-3k)/p, the optimal bonus isr*=(pθ2+h0k+kp-k)/(2θ2), and the optimal expected profits areE[π*(r*)]=(L(pθ2-h0k-kp+k)2/(4θ2k).

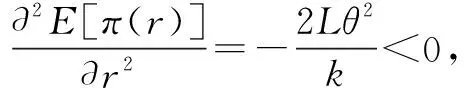
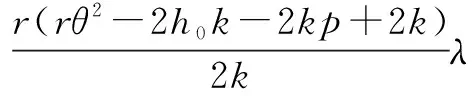
(5)

Since the expected profits function is concave, the necessary and sufficient conditions for optimality are as follows:

The first-order conditions of the Lagrangean are

(6)

(7)

In order to solve the problem, we have to discuss the value of parameterλ.

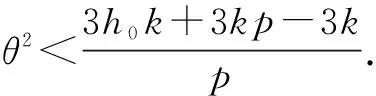
From Proposition 1 and Proposition 2, we can find out whether to implement the SRP on social media and how much reward should be offered to the inductors are dependent on users’ social relationships. If the social relationship is weak (θ2<(2h0k+2kp-2k)/p), there is no need to adopt the SRP on social media. However, there are two strategies for offering a reward when the users’ social relationship is strong enough . If the social relationshipθ2<(3h0k+3kp-3k)/p, the firm will set the optimal sharing-reward with 2k(h0+p-1)/θ2; otherwise, the inductors will be offered a reward of (pθ2+h0k+kp-k)/(2θ2).

In practical terms,there are many types of social media in the market, such as WeChat, Weibo, QQ, BBS, etc. The social relationship among users on different platforms also varies significantly. For example, the users’ social relationship on BBS or forums is usually weak[16], since the users have not met in real life and cannot establish strong trust with each other. As a result, it seldom occurs to firms to implement SRPs on social media. We find that neither Mobike’s nor Didi’s SRPs have the sharing option for the “weak ties” social media.

However, the social network on Wechat mainly reflects real relationships in everyday life, which results in a relatively stronger relationship among users. Many firms’ SRPs allow users to share information through Wechat. It suggests that when making the optimal reward strategy, the firm needs to take the type of social media and users’ social relationship into consideration.

Meanwhile, we differentiater*andπ*(r*) with respect to social relationshipθand obtain the following proposition.

Proposition3The optimal sharing bonus is negatively correlated with the social relationship, and the firm’s expected profits are positively correlated with the social relationship.

Proposition 3 shows that the stronger the social relationship between the inductor and the inductee, the fewer bonuses the firm will offer to the inductor. In the SRPs, each inductor becomes a temporary salesman and is delegated to persuading his or her friends to buy the goods. It can be understood that the closer the inductor and the inductee, the stronger the persuasion effect of bonus per unit. Moreover, the stronger the social relationship, the great the purchasing possibility will be, which will bring more profits to the firm. As a result, the firm has no need to pay a high bonus to the inductor if the SRP is implemented on the social media where the users’ relationship is very strong. The firm may also obtain more profits at the same time.

Proposition 3 provides the implication for managers that the SRPs should be implemented on the social media where users are familiar with each other. In China, WeChat and Weibo are the best choices. Also, it indicates that the loyal customers’ social network can be viewed as a kind of social capital. This is the reason why Alibaba invests much money on e-commerce socializing. For example, at the recent China Spring Festival Gala, Alipay developed the “Lucky Card Collection” campaign to encourage the users on Taobao to make friends. Then, social capital can be converted into economic capital. So, the firms should take full advantage of social media when doing promotions.

4 Conclusion

With the popularity of social media, more and more firms have adopted sharing reward programs to enhance sales. The social relationship among users may have an impact on the SRPs. In this paper, we use the nested Stackelberg game model to find the optimal sharing bonus. Some interesting observations are given. First, the higher the bonus, the more effort the inductor will make to persuade his friends. Secondly, the optimal sharing bonus depends on the social relationship between the inductor and the inductee. Moreover, the stronger the social relationship, the smaller the sharing bonus should be offered to the inductor, and the higher the expected profits will be.

In future research directions, two issues are worth considering. First, when adopting SRPs, many firms also use mass advertisement to attract potential customers. So, a comparison between the mass advertisement strategy and the SRP can be made in future study. In addition, sharing business information also makes friends feel distrust. Consequently, the negative impact of sharing behavior should also be taken into consideration.