行为AANA阵列的收敛性

王宽程, 杨英钟

(闽南理工学院 信息管理学院, 福建 泉州 362700)

AANA序列是一类广泛的负相依序列,文献[1]介绍了AANA序列的定义并指出NA(相关定义可见文献[2])序列是AANA序列,其混合系数满足(q(n)=0,n≥1),文中还举出了一些例子.因此研究AANA阵列的收敛性质是有意义的.文献[3]研究AANA序列的Hajek-Renyi型极大值不等式及其应用,文献[4]建立了AANA序列的Rosenthal型不等式;文献[5]研究了AANA序列极大不等式和强大数定律.有关更多AANA序列的研究成果,可以参见文献[6-9].本文利用截尾方法和矩不等式,研究AANA阵列在h-可积下的Lp收敛性和完全收敛性.由于AANA列比NA列更弱,因此本文所得的结论对NA随机变量序列仍然成立.

由于r阶Cesaro一致可积严格弱于r阶一致可积.而h-可积是比Cesaro一致可积更弱的条件[10]]. 本文研究了AANA阵列在h-可积条件下Lr收敛性和完全收敛性 ,因此本文所得结论推广了Cesaro一致可积条件下的相应结论.

1 相关定义

定义1[1]称{Xn;n∈N}为渐近几乎负相依(简称AANA)随机变量序列,如果存在非负序列q(n)→0(n→0),对任意的n,k≥1都有

Cov(f(Xn),g(Xn+1,…,Xn+k))≤q(n)(Var(f(Xn))Var(g(Xn+1,…,Xn+k)))1/2.

式中:f和g是任何两个使上述方差存在且对每个变元均为非降的连续函数.称{q(n);n∈N}为该序列的混合系数.

称随机阵列{Xnk;1≤k≤n,n∈N}是行为AANA阵列,固定n,假设每一行内的随机变量列{Xnk}是AANA的.

定义2[10]称阵列{Xnk;1≤k≤n,n∈N}关于常数阵列{ank;1≤k≤n,n∈N}h-可积的,若满足下列条件:

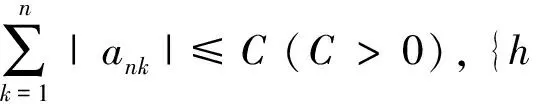
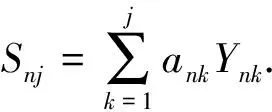
2 主要结论

引理1[6]设{Xn;n∈N}为AANA序列,并且混合系数是{q(n);n∈N},若{fn;n∈N}皆是单调非降(或者单调非增)连续函数,那么{fn(xn);n∈N}仍然是AANA序列,其混合系数仍然是{q(n);n∈N}.

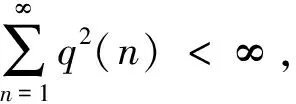
定理1 设{Xnk,1≤k≤n,n≥1}是行为两两AANA阵列,{ank,1≤k≤n,n≥1}是常数阵列,{h(n),n≥1}是单调不减序列,且h(n)→∞(n→∞),1≤p<2,若满足以下条件:

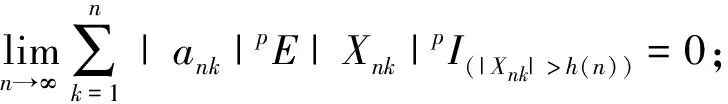
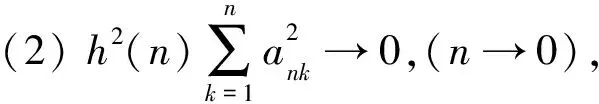
证明 对每个1≤k≤n(n≥1)令

由引理1可知Ynk及Znk仍为AANA阵列,且有|Ynk|≤h(n)和|Znk|≤|Xnk|I(|Xnk|>h(n)).由引理2及Jessen不等式、Cr不等式可知

由条件(1),(2)可得

定理1证毕.

定理2 设{Xnk;1≤k≤n,n≥1}为零均值且E|Xnk|<∞的行为AANA的阵列,{ank}是常数阵列,{h(n)}是单调不减序列,且h(n)→∞(n→∞).设α>0,αp>1,0<δ<1,q>0,t>0为实数,满足:αp-α-q<0及α-q+1<0.如果下面3个条件成立:

(ⅰ) {Xnk}是关于常数阵列{ank}的h-可积,且max|ank|=O(n-q);

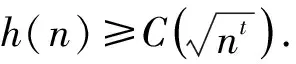
∀ε>0,有

(1)

证明 对每个1≤k≤n(n≥1),令

Ynk=XnkI(|Xnk|≤

h(n))-h(n)I(Xnk<-h(n))+

h(n)I(Xnk>h(n)),

由引理1可知{Ynk;1≤k≤n,n≥1}仍为AANA阵列.

先证

因为∀ω∈Dn,有

且∀1≤i-h(n)或Xnj(ω)>-h(n).

若记a=#{i:1≤i≤n,Xni(ω)>h(n)},b=#{i:1≤i≤n,Xni(ω)<-h(n)}.

则易知a≤1,b≤1.

当a=0,b=0时,有∀j(1≤j≤n),|anjXnj(ω)|≤|anj|h(n),所以|anjXnj(ω)|=|anjYnj(ω)|,从而

当a=1,b=0时,仅有某个i0,使得Xni0(ω)>h(n),但仍有|ani0Xni0(ω)|<εnα,而其余的i,都有aniXni(ω)=aniYni(ω).若1≤k≤i0-1,则Sk(ω)=Uk(ω);若i0≤k≤n,则

Yi0(ω)=h(n)

从而

同理可证当a=0,b=1时及当a=1,b=1时的情况,故式(2)成立.因此,有

所以要证式(1),只需证明:

先证式(3),

再证式(4),

要证式(5)成立,只需证

由引理2及条件(ⅱ)可得

定理2证毕.

[ 1 ] CHANDRA T K,GHOSAL S. Extensions of the strong law of large numbers of Marcinkiewicz and Zygmund for dependent variables[J]. Acta Mathematica Hungarica, 1996,71(4):327-336.

[ 2 ] JOAG-DEV K,PROSCHAN F. Negative association of random variables with applications[J]. Annals of Statistics, 1983,11(1):286-295.

[ 3 ] KO M H,KIM T S,LIN Z. The Hjek-Rényi inequality for the AANA random variables and its applications[J]. Taiwanese Journal of Mathematics, 2005,9(1):111-122.

[ 4 ] YUAN D M,AN J. Rosenthal type inequalities for asymptotically almost negatively associated random variables and applications[J]. Science in China, 2009,52(9):1887-1904.

[ 5 ] WANG X,HU S,LI X,et al. Maximal inequalities and strong law of large numbers for AANA sequences[J]. Communications of the Korean Mathematical Society, 2011(26):151-161.

[ 6 ] CHANDRA T K,GHOSAL S. The strong law of large numbers for weighted averages under dependence assumptions[J]. Journal of Theoretical Probability, 1996,9(3):797-809.

[ 7 ] ZHANG L,WANG X. Convergence rates in the strong laws of asymptotically negatively associated randomfields[J]. Applied Mathematics: A Journal of Chinese Universities, 1999,14(4):406-416.

[ 8 ] WANG X,HU S,YANG W. Convergence properties for asymptotically almost negatively associated sequence[J]. Discrete Dynamics in Nature and Society, 2010(1):179-186.

[ 9 ] WANG X,HU S,YANG W. Complete convergence for arrays of row wise asymptotically almost negatively associated random variables[J]. Discrete Dynamics in Nature and Society, 2011(1):309-323.

[10] CABRERA M,VOLODIN A. Mean convergence theorems and weak laws of large numbers for weighted sums of random variables under a condition of weighted integrability[J]. Journal of Mathematical Analysis & Applications, 2005,305(2):644-658.