非线性能量阱对飞轮扰振特性的抑制
2017-06-15刘海平王耀兵史文华
刘海平,王耀兵,史文华
(1. 北京空间飞行器总体设计部,北京100094;2. 空间智能机器人系统技术与应用北京市重点实验室,北京100094)
非线性能量阱对飞轮扰振特性的抑制
刘海平1,2,王耀兵1,2,史文华1
(1. 北京空间飞行器总体设计部,北京100094;2. 空间智能机器人系统技术与应用北京市重点实验室,北京100094)
以星载飞轮稳定运行和姿态调整过程中瞬态输出扰振抑制为目标,利用欧拉屈曲梁并联线性弹簧构建一种非线性能量阱(NES)。利用所建耦合多自由度系统动力学模型,采用龙格-库塔法得到各部分时程响应曲线,并转化为能量形式对比研究在瞬态载荷和稳态激励下,外部输入能量在飞轮-非线性能量阱-支承结构之间的传递特征,进而判断非线性能量阱发生靶能量传递的初始条件。研究表明:只有在中等强度激励能量作用下,才能使非线性能量阱产生高效的靶能量传递;当能量由支承结构和飞轮组成的线性系统传递到非线性能量阱发生靶能量传递时,将产生覆盖较宽频带的显著振动响应;以实测飞轮系统稳态输出扰动作为输入载荷,安装非线性能量阱可有效抑制飞轮输出扰动的影响。
非线性能量阱;欧拉屈曲梁;飞轮;振动抑制
0 引 言
在轨航天器微振动源主要包括:姿态控制执行机构和驱动机构。其中,飞轮和控制力矩陀螺工作时产生的微振动是影响有效载荷工作性能的主要干扰源之一[1-4]。为便于指导飞轮微振动抑制系统的设计,众多学者研究了飞轮微振动产生机理和机制。针对单个飞轮、飞轮和安装结构、多个飞轮等不同研究对象开展了理论分析和实验研究[5-9]。
目前,已有不少针对星载飞轮的振动抑制研究,徐超等[10]提出将阻尼层嵌入飞轮复合材料支架内提高系统阻尼比,进而实现飞轮扰振抑制;张庆君等[11]系统全面的梳理了光学遥感卫星微振动抑制设计方法和关键技术,并提出一种同时满足主动段和在轨段扰振抑制的隔振器设计方案;关新等[12]为了兼顾高频和低频减隔振要求,提出采用被动元件实现类似主动隔振的控制规律,在保证隔振装置高频段隔振性能的同时,有效抑制共振峰。
由于飞轮旋转速度和考虑陀螺效应转子固有频率吻合时的共振放大作用引起的低频线谱干扰,虽然已采取隔振措施,但是由于低频干扰频率位于隔振系统的转折频率附近[13],仅通过阻尼抑振或者线性被动隔振其效果均有限。
相比于线性吸振器,非线性吸振器具有工作频带宽、吸振效率高、鲁棒性强、可靠性高、不需要提供额外能源、附加质量小等优点[14-15]。由于卫星等航天器对搭载质量和能耗有严格要求,并且在发射段和在轨运行阶段需要经历和承受复杂多变的空间环境,因此导致其对振动控制装置要求能够进行较宽频段的振动抑制。
近年,基于靶能量传递的非线性吸振器研究成为热点。靶能量传递是一种能量在离散非线性系统中的传递机制,其特点是精确定量的能量可由振源向强非线性耦合的受体单向不可逆的完全传递。用来实现靶能量传递的非线性吸振器称之为非线性能量阱(Nonlinear energy sink, NES)[14]。
针对星载飞轮在轨扰振特性,本文利用欧拉屈曲梁并联线性弹簧构建一种非线性能量阱,并结合实际工况建立飞轮-非线性能量阱-支承结构耦合离散多自由度系统动力学模型。飞轮工作过程中分别在稳定运行和姿态调整过程中产生低频线谱扰动和瞬态脉冲激励。根据其载荷特征,利用所建系统动力学模型采用龙格-库塔法计算得到系统各部分的时程响应曲线,并转化为能量形式对比研究在瞬态载荷和稳态激励下,外部输入能量在飞轮-非线性能量阱-支承结构之间的传递特征,进而判断非线性能量阱发生靶能量传递的初始条件。研究表明:当输入线谱能量大于一定阈值可使非线性能量阱发生靶能量传递,并使其在一定时段内产生宽频大幅振动响应。
1 动力学建模
1.1 非线性能量阱模型
利用欧拉屈曲梁并联线性弹簧构建的非线性能量阱如图1所示[16-17]。
假设在初始状态下,单根欧拉屈曲梁中间初始挠度q0,在垂向载荷P的作用下,其末端垂向位移为y,中间挠度为q见图2。
轴向载荷P和末端垂向位移y之间的关系:
P=
(1)
式中:Pe=EI(π/L)2,L为欧拉屈曲梁未变形时长度,E为材料弹性模量,I为曲梁截面惯性矩。
假设质量块的平衡位置为欧拉屈曲梁水平时的位置,得到非线性能量阱ya方向的弹性恢复力为:
(2)
式中:F为弹性恢复力,kL为线性弹簧刚度,h为曲梁截面厚度,w为曲梁截面宽度。
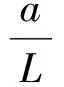

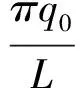
1.2 安装非线性能量阱系统动力学模型
图3给出飞轮-非线性能量阱-支承结构系统动力学模型。由图3可知,Mm为主系统质量,km为主系统弹性系数,cm为主系统阻尼系数;ma为非线性能量阱质量,ca为非线性能量阱阻尼系数;F(z)为欧拉屈曲梁输出弹性恢复力,ft为飞轮输出扰振。
在实际中,飞轮安装在铝蜂窝夹层结构板上。假设,飞轮简化为等效质量Mt,等效刚度kt和等效阻尼ct;铝蜂窝夹层结构板质量mm已知的情况下,根据结构板四边固支约束对应第1阶模态固有频率计算得到相应的等效刚度km;支承结构自身阻尼为cm。
由图3所示模型建立系统动力学方程:
(3)
暂不考虑系统中阻尼对输入能量的耗散,得到系统总能量:
E(t)=E1(t)+E2(t)+E3(t)
(4)
E1(t)为支承结构储存能量:
(5)
E2(t)为非线性能量阱储存能量:
(6)
E3(t)为飞轮储存能量:
(7)
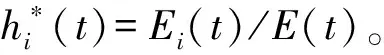
2 数值仿真及结果分析
2.1 瞬态激励响应
飞轮-非线性能量阱-支承结构耦合的离散多自由度系统动力学模型中各部分设计参数如表1所示。
在初始瞬态载荷作用下系统各部分的动力学响应分为三个区域(如图4所示)。根据系统各部分能量比,在t<0.5s时间范围内输入能量由支承结构和飞轮迅速转移到非线性能量阱。之后,在0.5s
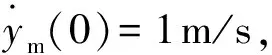
显然,随着输入能量量级变化系统各部分的响应发生显著变化。在低量级条件下,系统并未发生振动能量由线性振动系统向非线性能量阱转移的靶能量传递特征,振动能量局限于由支承结构和飞轮组成的线性系统参见图5(a)、(b)。当输入能量增加到中等量级时,非线性能量阱的靶能量传递特性被激发参见图5(c)、(d)。随着输入能量进一步增加,仍然能激发出非线性能量阱的靶能量传递特性;但是,其有效作用范围显著减小参见图5(e)、(f)。根据以上计算结果发现,飞轮-非线性能量阱-支承结构系统在中等量级输入能量激励下可以实现非线性能量阱最佳的靶能量传递。

表1 计算参数初值Table 1 Initial parameter values
通过对比系统各部分结构在不同输入能量激励下的瞬时能量比可以更清晰的观察到以上结论。在低输入量级区域振动能量局限于由支承结构和飞轮组成的线性系统中,并未激发非线性能量阱的靶能量传递参见图6(a)。随着初始输入能量增加,总能量中的相当一部分传递到非线性能量阱,由此实现对线性系统振动响应的有效衰减如图6(b)所示。当初始输入能量进一步增大,尽管激发了靶能量传递特征,但是仅有一部分能量传递到了非线性能量阱(如图6(c)所示)。因此,最有效的靶能量传递出现在输入能量大部分传递给非线性能量阱的工况下。
2.2 受迫振动响应
根据文献[18]某飞轮系统实测输出扰动,采用径向输出扰动力作为本文所述飞轮-非线性能量阱-支承结构系统的输入载荷,具体表达式为:
ft(t)= 0.4cos(120πt)+11.4cos(200πt)+
1.3cos(240πt)+0.33cos(280πt) +
2.1 cos(400πt) +1.5cos(600πt)
(8)
由于输入能量变化,为保证有效激发非线性能量阱的靶能量传递特征,选择欧拉屈曲梁设计参数:未变形长度L=0.05m,中间初始挠度q0=5×10-3m,梁截面厚度h=1×10-3m,其他参数与表1相同。
将安装非线性能量阱前后飞轮和支承结构位移响应时程曲线采用傅里叶变换转化到频率域如图7所示。由图7可知,在分析频段0Hz~300Hz范围内,支承结构和飞轮的响应得到了有效抑制,证明本文提出的非线性能量阱有效。
4 结 论
以星载飞轮稳定运行和姿态调整过程中输出扰动抑制为目标,本文利用欧拉屈曲梁并联线性弹簧构建一种非线性能量阱。通过数值分析计算,得到如下结论:
1)不同输入激励能量在支承结构、非线性能量阱和飞轮之间的传递特性差别较大;只有在中等强度激励能量下,才能使非线性能量阱产生高效的靶能量传递;
2)以实测飞轮系统稳态线谱输出扰动作为输入载荷,对比结构位移响应表明非线性能量阱有效;且非线性能量阱可有效抑制飞轮的扰动影响。
为验证本文所建理论模型和分析结果的有效性,正在开展地面验证试验,相关结果将另文详述。
[1] 孟光, 周徐斌. 卫星微振动及控制技术进展[J]. 航空学报, 2015, 36(8): 36-40. [Meng Guang, Zhou Xu-bin. Progress review of satellite micro-vibration and control [J]. Acta Aeronautica et Astronautica Sinica, 2015, 36(8): 36-40.]
[2] 邵骁麟. 航天器典型部件微振动隔离技术研究[D]. 上海: 上海交通大学, 2013. [Shao Xiao-lin. Investigation on micro-vibration isolation technology for typical units of spacecraft [D]. Shanghai: Shanghai Jiao Tong University, 2013.]
[3] Liu K C, Maghami P, Blaurock C. Reaction wheel disturbance modeling, jitter analysis, and validation tests for solar dynamics observatory [C]. AIAA Guidance, Navigation and Control Conference and Exhibit, Honolulu, USA, 2008.
[4] Kim D K. Micro-vibration model and parameter estimation method of a reaction wheel assemble [J]. Journal of Sound and Vibration, 2014, 333: 4214-4231.
[5] 邓瑞清, 赵岩, 房建成, 等. 磁悬浮飞轮与机械飞轮干扰特性的对比分析 [J]. 宇航学报, 2016, 37(8): 917-923. [Deng Rui-qing, Zhao Yan, Fang Jian-cheng, et al. Disturbance characteristics analysis of magnetically suspended and mechanical flywheels [J]. Journal of Astronautics, 2016, 37(8): 917-923.]
[6] Luo Q, Li D X, Zhou W Y, et. al. Dynamic modelling and observation of micro-vibrations generated by a single gimbal control moment gyro [J], Journal of Sound and Vibration, 2013, 332: 4496-4516.
[7] Zhou W Y, Li D X, Luo Q, et al. Analysis and Testing of Microvibrations Produced by Momentum Wheel Assemblies [J], Chinese Journal of Aeronautics, 2012, 25(4): 640-649.
[8] Firth J, Black J. Vibration interaction in a multiple flywheel system [J]. Journal of Sound and Vibration, 2012, 331: 1701-1714.
[9] Narayan S S, Nair P S, Ghosal A. Dynamic interaction of rotating momentum wheels with spacecraft elements [J]. Journal of Sound and Vibration, 2008, 315(4-5): 970-984.
[10] 徐超, 李瑞杰, 游少雄. 卫星飞轮支架的共固化阻尼减振设计[J]. 宇航学报, 2010, 31(3): 907-911. [Xu Chao, Li Rui-jie, You Shao-xiong. Passive vibration control design with co-curing damping composite for a satellite flywheel bracket [J]. Journal of Astronautics, 2010, 31(3): 907-911.]
[11] 张庆君, 王光远, 郑钢铁. 光学遥感卫星微振动抑制方法及关键技术[J], 宇航学报, 2015, 36(2): 125-132. [Zhang Qing-jun, Wang Guang-yuan, Zheng Gang-tie. Micro-vibration attenuation methods and key techniques for optical remote sensing satellite [J]. Journal of Astronautics, 2015, 36(2): 125-132.]
[12] 关新, 王全武, 郑钢铁. 飞轮拟主动隔振方法[J]. 宇航学报, 2010, 31(7): 1870-1876. [Guan Xin, Wang Quan-wu, Zheng Gang-tie. A pseudo-active vibration isolation method for reaction wheels [J]. Journal of Astronautics, 2010, 31(7): 1870-1876.]
[13] Zhou W Y, Li D X, Luo Q, Jiang J P. Design and test of a soft suspension system for cantilevered momentum wheel assembly [J]. Proceedings of the Institution of Mechanical Engineers, Part G: Journal of Aerospace Engineering. 2012, 227(7):1144-1160.
[14] Kopidakis G, Aubry S, Tsironis G P. Targeted energy transfer through discrete breathers in nonlinear systems [J]. Physical Review Letters, 2001, 87(16): 1-4.
[15] Gendelman O, Manevitch L I, Vakakis A F, Closkey R M. Energy pumping in coupled mechanical oscillators, Part I: dynamics of the underlying Hamiltonian systems [J]. Journal of Applied Mechanics, 2001, 68: 34-41.
[16] 刘海平, 杨建中, 罗文波, 等. 新型欧拉屈曲梁非线性动力吸振器的实现及抑振特性研究[J]. 振动与冲击, 2016, 35(11): 155-160. [Liu Hai-ping, Yang Jian-zhong, Luo Wen-bo, et al. Realization and vibration suppression ability of a new novel Euler buckled beam nonlinear vibration absorber [J]. Journal of Vibration and Shock, 2016, 35(11): 155-160.]
[17] Liu H P, Shi W H, Vibration suppression of a flywheel system using a novel nonlinear vibration absorber with an Euler buckled beam [J]. Journal of Physics: Conference Series 744, 2016:1-12.
[18] 葛东明, 邹元杰, 张志娟, 等. 基于全柔性卫星模型的控制闭环微振动建模与仿真[J]. 航天器工程, 2012, 21(5): 58-63. [Ge Dong-ming, Zou Yuan-jie, Zhang Zhi-juan, et al. Control closed-loop micro-vibration modeling and simulation based on flexible satellite model [J]. Spacecraft Engineering, 2012, 21(5): 58-63.]
通信地址:北京海淀区友谊路104号(100094)
电话:(010)68113137
E-mail:lhpvibration@163.com
(编辑:牛苗苗)
Vibration Suppression for a Flywheel Based on Nonlinear Energy Sink
LIU Hai-ping1,2, WANG Yao-bing1,2, SHI Wen-hua1
(1. Beijing Institute of Spacecraft System Engineering, Beijing 100094, China; 2. Beijing Key Laboratory of Intelligent Space Robotic Systems Technology and Applications, Beijing 100094, China)
In order to absorb the vibration from the flywheel, a nonlinear energy sink (NES) is presented in this paper. The NES is composed of a linear spring and the parallel Euler buckled beams. A discrete multi-degree-of-freedom dynamic model, including the flywheel, the NES and the supporting structure is built. The systematic dynamic equations under the transient and steady loadings, respectively, are solved by fourth-order Runge-Kutta method. Based on these calculating results, it is shown that the NES exhibits the efficiency of energy transfer from the linear system, which includes the supporting structure and the flywheel, to the NES. Meanwhile, the targeted energy transfer has been excited. Then, a detailed computational research of the different types of nonlinear energy transitions that occur under the three-level inputting energy is provided. As a result of these studies, the medium-level exciting energy leads to the effective targeted energy transfer from the linear system to the NES. Under this condition, it exhibits that apparent responses of the NES in a wider frequency range. According to the calculating results under the experimental data from the flywheel, it can be concluded that the NES has suppressed the influences from the disturbance of the flywheel effectively.
Nonlinear energy sink; Euler buckled beam; Flywheel; Vibration suppression
2017-01-10;
2017-03-28
国家自然科学基金(51405014)
O322; TH113.1; X966
A
1000-1328(2017)05-0490-07
10.3873/j.issn.1000-1328.2017.05.007
刘海平(1982-),男,博士,高级工程师,主要从事空间结构动力学及振动噪声控制。
