第二类完全椭圆积分的平均值不等式

袁 琴， 俞芳婷， 王淼坤

(湖州师范学院 理学院， 浙江 湖州 313000)

第二类完全椭圆积分的平均值不等式

袁 琴， 俞芳婷， 王淼坤

(湖州师范学院 理学院， 浙江 湖州 313000)

研究一个与第二类完全椭圆积分相关的平均值,证得它关于调和平均、反调和平均的算术凸组合与几何凸组合的两个最佳双边不等式,进而得到第二类完全椭圆积分在某种形式下的两个最优不等式.

调和平均； 反调和平均； 完全椭圆积分； 不等式

MSC 2010:33E05; 26E60

0 引 言

对t∈[0,1],第一类完全椭圆积分K(t)和第二类完全椭圆积分E(t)分别定义如下[1]：

(1)

(2)

自上世纪90年代以来,完全椭圆积分被广泛研究,并应用于拟共形映射偏差定理的估计.关于完全椭圆积分的基本性质及其应用见文献[1-6].

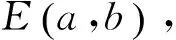
(3)

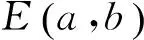
定理1 当a,b>0且a≠b时,不等式

(4)

成立当且仅当α≤α0=4/π2及β≥β0=7/16.

定理2 当a,b>0且a≠b时,不等式

(5)

若在定理1和定理2中令a=1,b=t′2,便得第二类完全椭圆积分的不等式.这里及下面均记：

(0,1)).

成立当且仅当α≤α0=4/π2及β≥β0=7/16.

1 主要结果的证明

定理1的证明 通过不等式变形,定理1可改写为:当a,b>0且a≠b时,

(6)

当且仅当α≤4/π2及β≥7/16.

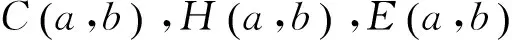
则b∈(0,1)且

(7)

令p∈(0,1),则

(8)

记

(9)

计算得:

(10)

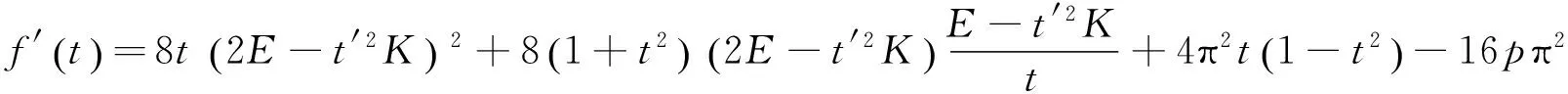
(11)

再令

(12)

则

(13)

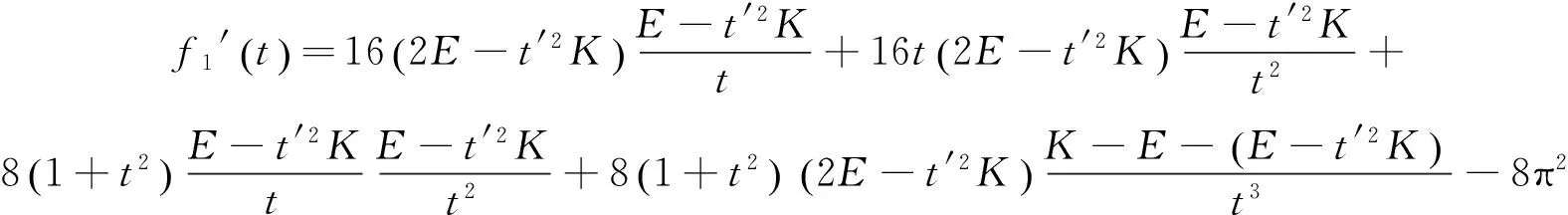
(14)

令

(15)

则

(16)

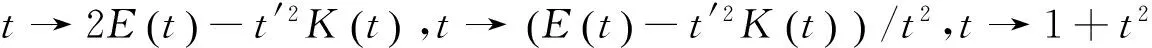
下面分两种情况进行讨论：

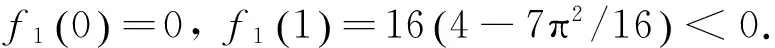
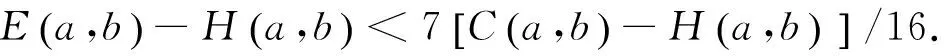
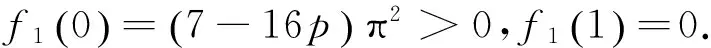
最后证明α0和β0是使得双边不等式(4)成立的最佳参数.

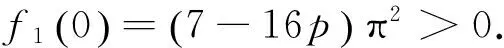
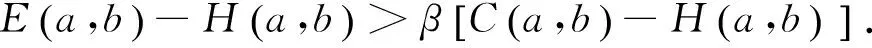
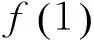
定理2的证明 通过对不等式两边同时取对数变形后，定理2可改写为：当a,b>0且a≠b时,

(17)

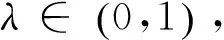
(18)

令

(19)

则

(20)

(21)

其中：

λ(4t2+12)(2E-t′2K)(1-t4)+4(1-λ)(2E-t′2K)(1+t2)(t4+6t2+1)；

(22)

(23)

下面分两种情形进行讨论：

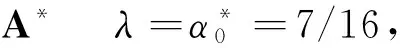
(24)

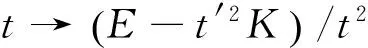
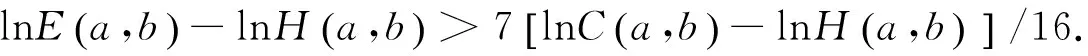
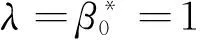
[1]ANDERSON G D,VAMANAMURTHY M K,VUORINEN M.Conformal Invariants,Inequalities,and Quasiconformal Maps[M].New York:John Wiley & Sons,1997.

[2]ABRAMOWITZ M,STEGUN I A.Handbook of Mathematical Functions,with Formulas,Graphs,and Mathematical Tables[M].New York:Dover Publications,1965.

[3]ANDERSON G D,VAMANAMURTHY M K,VUORINEN M.Functional inequalities for complete elliptic integrals and their ratios[J].SIAM J Math Anal,1990,21(2):536-549.

[4]ALZER H,QIU S L.Monotonicity theorems and inequalities for the complete elliptic integrals[J].J Comput Appl Math,2004,172(2):289-312.

[5]ANDERSON G D,QIU S L,VAMANAMURTHY M K.Elliptic integral inequalities,with applications[J].Constr Approx,1998,14(2):195-207.

[6]ALZER H,RICHARDS K C.A note on a function involving complete elliptic integrals:monotonicity,convexity,inequalities[J].Anal Math,2015,41(3):133-139.

[7]WANG M K,QIU S L,CHU Y M,et al.Generalized Hersch-Pfluger distortion function and complete elliptic integrals[J].J Math Anal Appl,2012,385(1):221-229.

[8]TOADER G H.Some mean values related to the arithmetic-geometric mean[J].J Math Anal Appl,1998,218(2):358-368.

[9]BULLEN P S,MITRINOVIC D S,VASIC P M.Means and Their Inequalities[M].Dordrecht:D Reidel Publishing Co,1988.

[10]NEUMAN E.On some means derived from the Schwab-Borchardt mean[J].J Math Inequal,2014,8(1):171-183.

[12]WANG M K,QIU Y F,CHU Y M.Sharp bounds for Seiffert means in terms of Lehmer means[J].J Math Inequal,2010,4(4):581-586.

MSC 2010:33E05; 26E60

[责任编辑 高俊娥]

Inequalities for the Complete Elliptic Integrals of the Second Kind in Terms of Means

YUAN Qin, YU Fangting, WANG Miaokun

(School of Science, Huzhou University, Huzhou 313000, China)

A mean value related to the complete elliptic integrals of the second kind is investigated, and some optimal double inequalities in terms of harmonic mean and contra-harmonic mean are proved. These results lead to two best possible inequalities for the complete elliptic integrals of the second kind in some form.

harmonic mean; contra-harmonic mean; complete elliptic integrals; inequality

2016-12-23

浙江省教育厅科研计划项目(Y201635325);湖州师范学院“大学生创新训练计划”项目(2016-100).

王淼坤，博士，讲师，研究方向：特殊函数、拟共形映射.E-mail:wangmiaokun@zjhu.edu.cn

O172

A

1009-1734(2017)02-0012-05