一类广义Emden-Fowler阻尼方程的振动准则

惠远先，王俊杰

(普洱学院数学系， 云南　普洱　665000)



一类广义Emden-Fowler阻尼方程的振动准则

惠远先，王俊杰

(普洱学院数学系， 云南普洱665000)

文章研究一类广义Emden-Fowler阻尼方程的振动性质，利用广义Riccati变换和积分平均技巧，建立了保证方程一切解振动或者收敛到零的若干新的充分条件.所得结论推广和改进了最近文献中的若干结果.

广义Emden-Fowler阻尼方程；广义Riccati变换；振动准则

微分方程解的振动性是微分方程解的重要性态之一，它在很多领域具有广泛的应用.本文考虑一类广义Emden-Fowler方程：

(E)

其中 ：

Z(t)=x(t)+p(t)x(τ(t)),r(t)∈C1([t0,∞),R),p(t),τ(t),g(t)∈C[t0,∞)，α,β

是两个常数.本文假设下面条件成立.

(H1)α>0,β>0；

(H2)0≤p(t)≤1,q(t)≥0,g(t)≥0；

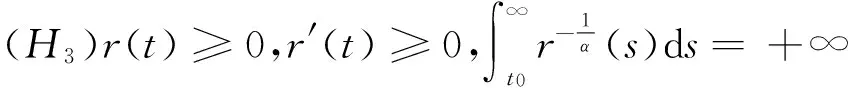
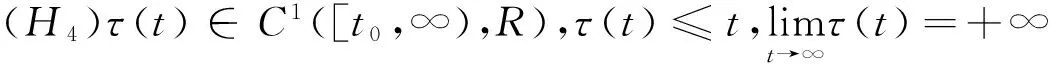
方程(E)的一个非平凡解是振动的，如果它有任意大的零点.否则为非振动的.如果方程(E)的一切解都是振动的则称该方程是振动的.近年来，关于动力学方程的振动性与非振动性的研究引起了学者们的广泛兴趣，出现了大量优秀研究成果[1-9].2006年，MENG[3]给出了方程

的振动结果.2012年,LIU[9]给出了方程

(E1)

在α≥β>0情形下的振动准则.

由于方程(E1)没有阻尼项，且受条件α≥β>0限制，该文所得的振动结果具有很大局限性.本文利用广义Riccati变换和积分平均技巧，将文献[9]的相关结论由非阻尼方程(E1)推广到阻尼方程(E)的情形，而且将条件α≥β>0推广到α>0，β>0的一般情形.

1　引理

引理1设x(t) 是广义的Emden-Fowler阻尼方程(E)的最终正解，则相应Z(t)只有下面两种情况：

Z′(t)]′≤0；

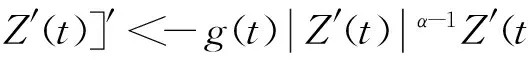
证明设x(t) 是广义的Emden-Fowler阻尼方程(E)的最终正解，由Z(t)的表达式得到Z(t)≥x(t)>0.由方程的(E)得到两种可能：Z′(t)>0 或Z′(t)<0.

(Ⅰ)设Z′(t)>0，由方程(E)可以得到：

(Ⅱ)设Z′(t)<0，由方程(E)可以得到：

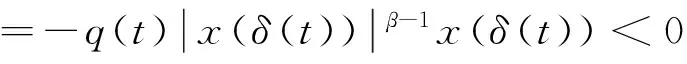
由条件(H2)和Z′(t)<0可以得到两种可能：

对上式从t1到t积分，可以得到：

让t→∞，利用(H3)得到Z(t)→-∞.这与Z(t)>0矛盾.

引理2[4]设存在两个函数A(θ)>0,B(θ)>0 且θ>0，则

(1)

引理3设x(t)是广义的Emden-Fowler阻尼方程(E)的最终正解，相应的Z(t)满足(Ⅰ),则

[r(t)Z′(t)α]′+g(t)Z′(t)α+Q1(t)Zβ(δ(t))≤0

(2)

其中，Q1(t)=[q(t)(1-p(δ(t)))]β.

证明由Z(t)=x(t)+p(t)x(l(t))可得x(t)=Z(t)-p(t)x(l(t))，利用(H4)和Z′(t)>0，可得：

x(t)=Z(t)-p(t)x(l(t))≥Z(t)(1-p(t))，

x(δ(t))β≥Z(δ(t))β(1-p(δ(t)))β，

再由方程(E)，可得：

Q1(t)Zβ(δ(t))≤0.

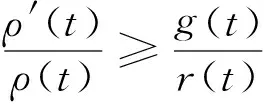
ϖ′(t)≤-ρ(t)Q1(t)+Q2(t)ϖ(t)-

其中：

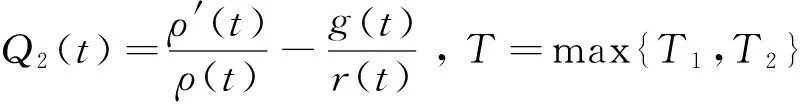
证明由ϖ(t)的定义及引理3得：

≤-ρ(t)Q1(t)+Q2(t)ϖ(t)-

(i)β>α>0时，

由引理3知，[r(t)Z′(t)α]′≤-g(t)(Z′(t))α-Q1(t)Zβ(δ(t))≤-Q1(t)Zβ(δ(t))≤0.所以r(t)Z′(t)α单调递减，r(t)(Z′(t))α≤r(δ(t))(Z′(δ(t)))α.由(H3)知，

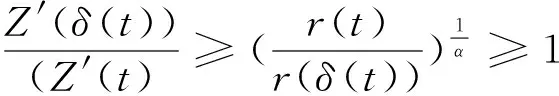
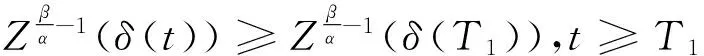
从而

当β>α>0时，

ϖ′(t)≤-ρ(t)Q1(t)+Q2(t)ϖ(t)-

(3)

(ii)α≥β>0时，

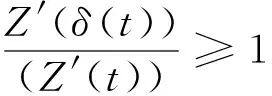
ϖ′(t)≤-ρ(t)Q1(t)+Q2(t)ϖ(t)-

(4)

现记T=max{T1,T2}，λ=min{α,β}，

综合(3)式和(4)式可得α>0,β>0时的广义黎卡提不等式为：

ϖ′(t)≤-ρ(t)Q1(t)+Q2(t)ϖ(t)-

(5)

2　主要结果

定理1假定引理1—引理4 成立，x(t)是广义的Emden-Fowler阻尼方程(E)的最终正解，且相应的Z(t)满足(Ⅰ).若

(6)

则x(t)振动.

证明假设x(t)不振动，不失一般性，令x(t)是广义的Emden-Fowler阻尼方程(E)的最终正解.由引理4可得：

ϖ′(t)≤-ρ(t)Q1(t)+Q2(t)ϖ(t)-

由引理2可得：

对上面方程从T到t积分可得：

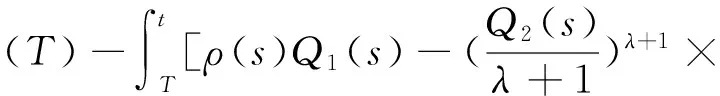
当t→+∞时，由(6)式可得ϖ(t)→-∞.矛盾，所求得证.假定ρ(t)=δn(t)，可得以下推论：

推论1假定引理1—引理4 成立，x(t)是广义的Emden-Fowler阻尼方程(E)的最终正解，且相应的Z(t)满足(Ⅰ).若

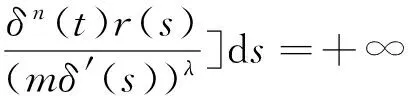
则x(t)振动.

定理2假定引理1—引理4 成立，x(t)是广义的Emden-Fowler阻尼方程(E)的最终正解，且相应的Z(t)满足(Ⅰ).若

(7)

则x(t)振动.

证明假设x(t)不振动，不失一般性，令x(t)是广义的Emden-Fowler阻尼方程(E)的最终正解，由引理4可得：

ϖ′(t)≤-ρ(t)Q1(t)+Q2(t)ϖ(t)-

利用引理2可得：

两边同时乘以(t-s)n，再从T到两边积分(t>T)可得：

≤(t-T)nϖ(T).

两边同除以tn可得：

(8)

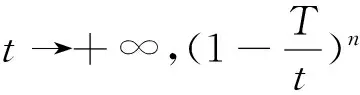
(9)

这与(7)式矛盾，假设不成立，所求得证.假定ρ(t)=δn(t)，由定理2可得以下推论：

推论2假定引理1至引理4 成立，x(t)是广义的Emden-Fowler阻尼方程(E)的最终正解，且相应的Z(t)满足(Ⅰ).若

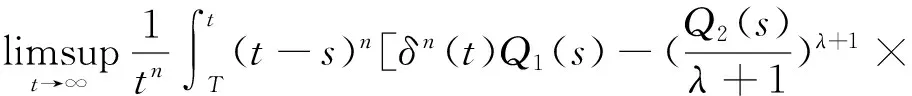
以下利用Philos型积分平均条件，给出广义的Emden-Fowler方程(E)的振动准则.为此令

D0={(t,s)：t>s≥t0}

D={(t,s)：t≥s≥t0}

我们称函数H(t,s)∈C1(D,R)为属于F类，记作H(t,s)∈F，如果满足

(Ⅰ)H(t,t)=0，t≥t0，H(t,t)>0；

(Ⅱ)存在ρ(t)∈C1([t0,∞),R+)，h∈C(D0,R)，使得

(10)

定理3假定引理1—引理4 成立，x(t)是广义的Emden-Fowler阻尼方程(E)的最终正解，且相应的Z(t)满足(Ⅰ).若

(11)

则x(t)振动.

证明假设x(t)不振动，不失一般性，设x(t) 是广义的Emden-Fowler方程(E)的最终正解，利用引理4可得：

ϖ′(t)≤-ρ(t)Q1(t)+Q2(t)ϖ(t)-

两边同乘以H(t,s)，并从T到t(t>T)两边积分，再由引理2及方程(10)得：

(12)

由方程(12)可得：

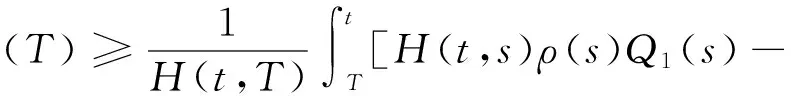
(13)

这与(11)式矛盾，原假设不成立，所求得证.若取H(t,s)=(t-s)n，则定理3可简化为Kamenev型结果如下：

推论3假定引理1至引理4 成立，x(t)是广义的Emden-Fowler阻尼方程(E)的最终正解，且相应的Z(t)满足(Ⅰ).若

则x(t)振动.

推论4假定引理1—引理4 成立，x(t)是广义的Emden-Fowler阻尼方程(E)的最终正解，且相应的Z(t)满足(Ⅰ).若

则x(t)振动.

[1]PHILOSCG.Oscillationtheoremsforlineardifferentialequaionsofsecondorder[J].Arch.Math,1989,53(5):482-492.

[2]YANJ.Oscillationtheoremsforsecondorderlineardifferentialequationswithdamping[J].AmericanMathematicalSociety,1986,98(2):276-282.

[3]SUNYG,MENGFW.Noteonthepaperofdzurinaandstavroulakis[J].AppliedMathematicsandComputation,2006,174(2):1634-1641.

[4]XUR,MENGFW.Somenewoscillationcriteriaforsecondorderquasi-linearneutraldelaydifferetialequations[J].AppliedMathematicsandComputation, 2006,182(1): 797-803.

[5]XUR,MENGFW.Oscillationcriteriaforsecond-orderquasi-linearneutraldelaydifferentialequation[J].AppliedMathematicsandComputation,2007,192(1):216-222.

[6]LIULH,BAIYZ.Newoscillationcriteriaforsecondordernonlinearneutraldelaydifferentialequation[J].JournalofComputationalandAppliedMathematics，2009, 231(2):657-663.

[7]WongJSW.AnonoscillationtheoremforsublinearEmdenFowlerequations[J].AnalysisandApplications2011,1(1):71-79.

[8]LITX,HANZL,ZHANGCH.Ontheoscillationofsecond-orderemdenfowlerneutraldifferentialequations[J].JournalofAppliedMathematicsandComputation,2011,37(1):601-610.

[9]LIUHD,MENGFW,LIUPC.OscillationandasymptoticanalysisonanewgeneralizedEmden-Fowlerequation[J].AppliedMathematicsandComputation,2012,219(5):2729-2748.

(责任编辑穆刚)

Some oscillation results of a generalized Emden-Fowler equation

HUI Yuanxian, WANG Junjie

(Mathematics Department,Puer University, Puer Yunnan 665000, China)

The purpose of this paper is to study the oscillation properties of a generalized Emden-Fowler equation with damping. Using a generalized Riccati transformation and integral averaging technique, some new sufficient criteria were established to insure that any solution of this equation oscillates or converges to zero. The results extend and improve the ones in recent literature.

a generalized Emden-Fowler equation with damping; generalized Riccati transformation; oscillation criteria

2016-03-29

云南省教育厅基金项目(2015Y490)；普洱学院校级科研创新团队项目(2015CXTD003)；普洱学院课题(2015xjkt20).

惠远先(1983—)，男，河南南阳人，讲师，硕士，主要从事微分方程方面的研究.

O175.25

A

1673-8004(2016)05-0019-05