一类扩散型捕食—食饵模型非常值正稳态解的不存在性
2016-09-27周文书王书臣
周文书,王书臣,王 倩
(大连民族大学 理学院,辽宁 大连116605)
一类扩散型捕食—食饵模型非常值正稳态解的不存在性
周文书,王书臣,王倩
(大连民族大学 理学院,辽宁 大连116605)
研究了一类扩散型捕食-食饵模型非常值正稳态解的不存在性问题。 该模型能够用来描述处于异构环境中的两个种群的生存状态。利用极值原理和迭代技巧,给出了该模型不存在非常值正稳态解的一个充分条件。这个结果是对该模型理论研究的一个补充。
捕食-食饵模型;稳态解;迭代技巧
1 问题背景与主要结果
Courchamp和 Sugihara[1]构建了如下异构环境中的一类捕食-食饵模型:

式中,B为食饵密度,C为捕食者密度,K为异构环境的搬运容量,rb和rc分别为食饵和捕食者的增长率,μ为捕食者的捕食率。该模型能够用来描述在异构环境中的两个种群的生存状态。
当考虑到种群在空间Ω中的扩散影响时,上述ODE模型转化为如下反应-扩散模型:
(1)
式中, Ω⊂RN为有界光滑区域,ν为∂Ω上单位外法向量, db和dc分别表示两个物种的扩散率,亦称扩散系数。目前,已有很多关于扩散型捕食-食饵模型的理论研究结果,见参考文献[2-9]。
模型(1)的稳态解满足如下奇异椭圆方程组:
为方便讨论,不妨设扩散系数都等于1,则上述方程组转化为
(2)
Du[9]等人研究了一个与模型(1)类似的模型:

模型(1)和(3)的本质区别是后者的解不会发生爆破现象,这说明了模型(1)的复杂性。
Gaucel和Langlais[7]研究了模型(1)解的存在性、大时间行为以及解的爆破现象等。 然而,至今未见有关椭圆方程问题(2)的研究结果。本文的主要目的是研究问题(2)非常值正解的不存在性。主要结果如下:
定理1设rc>rb>1,rc>2,则问题(2)没有非常值正解。
2 主要结果的证明
为证明定理1,不加证明地引用如下引理:

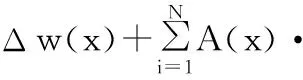
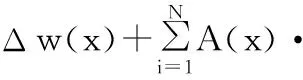
定理1的证明
假设(B,C)是如下问题的一个正解:
(4)
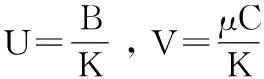
(5)

-ΔU=rb(1-U)U-φU=U(rb-rbU-φ),
-φΔU-UΔφ-2▽U▽φ=rc(1-φ)φU
即
=rc(1-φ)φ-[rb(1-U)φ-φ2]
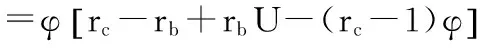
令u=rbU,则(φ,u)满足
-Δu=u(rb-u-φ),
(6)

(7)
由式(6)得

注意到, rc>rb>1,rc>2,则由引理1得

(8)
将式(8)代入式(6)得
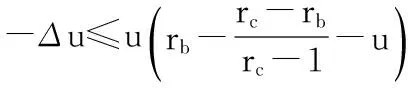
由引理1得
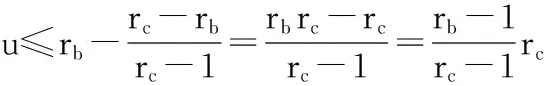
(9)

(10)
将式(10)代入式(6)得
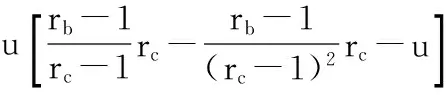
由引理1得
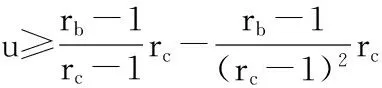
(11)
将式(11)代入式(7)得

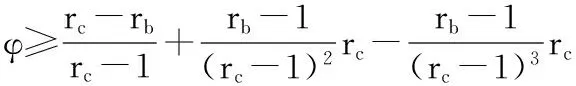
(12)
将式(12)代入式(6)得
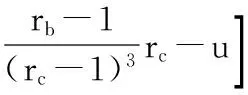
由引理1得
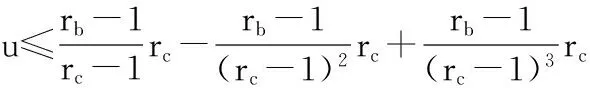
(13)
将式(13)代入式(7)得
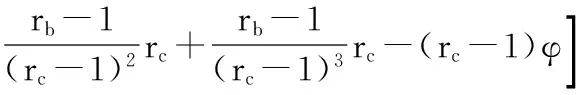
由引理1得
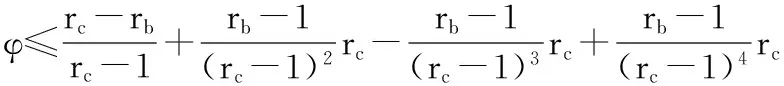
通过归纳,知对任意自然数n成立如下关系:
以及
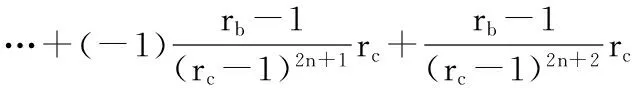
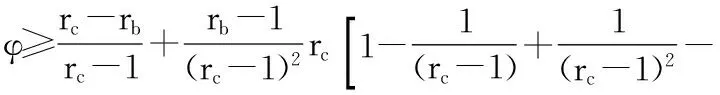
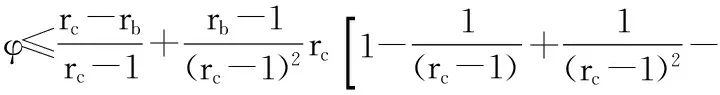
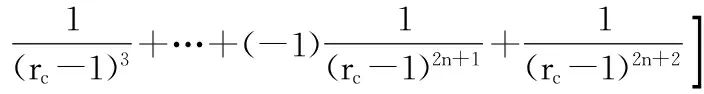
令n→+∞,得
以及
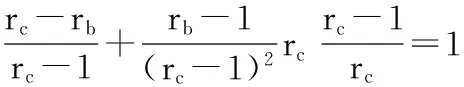
故φ=1,即 V=U。代入V的方程知
-ΔU=0,
这表明U是常数,于是
rb(1-U)=1,
即
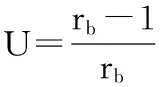
从而
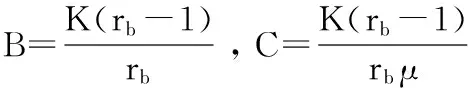
所以,模型(1)只有正常值解。
证毕。
[1] COURCHAMP F, SUGIHARA G. Modelling the biological control of an alien predator to protect island species from extinction[J]. Ecological Applications, 1999,9: 112-123.
[2] MURRAY J D. Mathematical Biology[M]. 3rd ed. Berlin:Biomathematics Series, Springer Verlag, 2002.
[3] WANG M X. Spreading and vanishing in the diffusive prey-predator model with a free boundary[J]. Communications in Nonlinear Science and Numerical Simulation, 2015, 23(1): 311-327.
[4] WANG M X, ZhAO Y G. A semilinear parabolic system with a free boundary[J]. Zeitschrift für angewandte Mathematik und Physik, 2015, 66(6): 3309-3332.
[5] GAUCEL S, PONTIER D. How predator food preference can change the destiny of native preys in predator-prey systems[J]. Biological Invasion, 2005, 7: 795-806.
[6] CHEN W, WANG M.Qualitative analysis of predator-prey models with Beddington-DeAngelis functional response and diffusion[J].Math Computer Modelling, 2005, 42: 31-44.
[7] GAUCEL S, LANGLASI M PONTIER D. Invading introduced species in insular hetero -geneous environments[J]. Ecological Modelling, 2005, 18: 62-75.
[8] GAUCEL S. Some remarks on a singular reaction-diffusion system aring in predator-prey modeling[J]. Discrete and Continuous Dynamical System, Series B, 2007, 8(1): 61-72.
[9] DU Y H, HSU S B, A diffusive predator-prey model in heterogeneous Environment [J].J Differential Equations, 2004, 203: 331-364.
[10]LOU Y, NI W M. Diffusion, self-diffusion and cross-diffusion[J]. Journal of Differential Equations, 1996, 131: 79-131.
(责任编辑邹永红)
Nonexistence of Non-constant Positive Stationary Solution for a Diffusive Prey-predator Model
ZHOU Wen-shu, WANG Shu-chen, WANG Qian
(School of Science, Dalian Minzu University, Dalian Liaoning 116605, China)
The nonexistence of positive stationary solution for a prey-predator model with diffusion is studied. The model can be used to describe the survival state of two species in heterogeneous environments. By means of the maximum principle and iterative technique, a sufficient condition for the nonexistence is given. This result complements some previous results on the model.
prey-predator model; stationary solutions; iterative technique
2096-1383(2016)05-0492-04
2016-05-25
国家自然科学基金项目(11571062); 辽宁省高等学校杰出青年学者成长计划(LJQ2013124); 中央高校基本科研业务费专项资金资助项目(DC201502050202)。
周文书(1974-),男,黑龙江齐齐哈尔人,教授,博士,博士生导师,主要从事非线性偏微分方程研究。
O175.2
A
