基于脉冲和时滞效应的拟线性双曲系统的(强)振动性分析*

罗李平， 罗振国， 曾云辉

(衡阳师范学院数学与统计学院，湖南 衡阳 421002)



基于脉冲和时滞效应的拟线性双曲系统的(强)振动性分析*

罗李平， 罗振国， 曾云辉

(衡阳师范学院数学与统计学院，湖南 衡阳 421002)

摘要：脉冲偏微分系统能够成功地应用于力学、理论物理、化学及人口动力学、生物工程、最优控制和经济学等方面的数学模拟。振动性理论作为脉冲偏微分系统定性理论的重要分支之一，对其进行研究具有极大的理论意义与实用价值。本文考虑一类基于脉冲和时滞效应的拟线性双曲系统的(强)振动性问题，直接从(强)振动的定义出发，借助新的处理拟线性扩散项的技巧、Green散度定理和第二类边界条件把这类系统解的振动问题转化为脉冲时滞微分不等式不存在最终正解的问题，并利用二阶脉冲时滞微分不等式的某些结果，建立了该类系统在第二类边界条件下所有有界解(强)振动的若干充分判据，结论充分地表明系统振动是由脉冲量和时滞量引起的，“强振动性”的判别条件比“振动性”的判别条件更强些。

关键词：双曲系统；(强)振动性；拟线性扩散项；脉冲；时滞

引用格式：罗李平， 罗振国， 曾云辉. 基于脉冲和时滞效应的拟线性双曲系统的(强)振动性分析[J]. 中国海洋大学学报(自然科学版)， 2016, 46(4)： 149-152.

LUO Li-Ping, LUO Zhen-Guo, ZENG Yun-Hui. (Strong) Oscillation analysis of quasilinear hyperbolic systems based on effect of impulse and delay[J]. Periodical of Ocean University of China, 2016, 46(4): 149-152.

脉冲现象作为一种瞬时突变现象，在现代科技各领域的实际问题中普遍存在，且往往对实际问题的规律产生本质的影响。因此，在建立数学模型对这些实际问题进行研究时，必须充分考虑脉冲现象的作用，这类数学模型往往可归结为脉冲偏微分系统。它比通常连续微分系统所表达的模型研究起来要困难得多，除了一般的理论困难外，可能还出现在扰动时刻不固定时滞系统常常产生的不断脉冲打击现象，这使得系统中原来保持的一些好的性质被脉冲的干扰而破坏。尽管如此，但脉冲偏微分系统表达了许多连续微分系统中无法表达的具有实际背景的数学模型，在物理学、电磁学、生态学及人口动力学以至于经济学中的许多数学模型都需要它来表达。因此，研究脉冲偏微分系统在理论和应用两方面都有重要意义。近年来对脉冲偏微分系统振动性理论的研究引起了人们的极大关注，已陆续有一些很好的研究工作发表[1-6]。但相对而言，关于脉冲偏微分系统的强振动的研究还不多见[7-9]。本文的目的是研究如下一类特殊的基于脉冲和时滞效应的拟线性双曲系统(1)在第二类边界条件(2)下有界解的(强)振动性，利用新的处理拟线性扩散项的技巧及二阶脉冲时滞微分不等式的某些结果，获得了判别该类系统所有有界解(强)振动的若干充分条件，所得结果充分表明系统振动是由脉冲和时滞效应引起的。

(1)

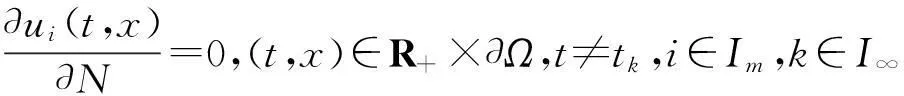
(2)

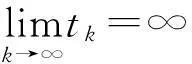
在本文中，对系统(1)总假定下列条件成立：

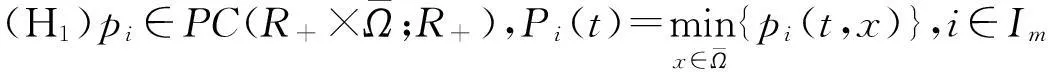
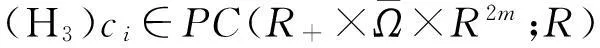
ci(t,x,ξ1,…,ξi,…,ξm,η1,…,ηi,…,ηm)=

ci(t,x,ξ1,…,-ξi,…,ξm,η1,…,-ηi,…,ηm)=

-ci(t,x,ξ1,…,ξi,…,ξm,η1,…,ηi,…,ηm)，i∈Im。

1有关定义、引理及记号

定义1称向量函数u(t,x)=(u1(t,x),u2(t,x),…,um(t,x))T为边值问题(1)～(2)的解，若对i∈Im，ui(t,x)满足：

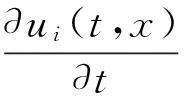
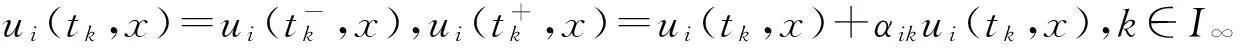
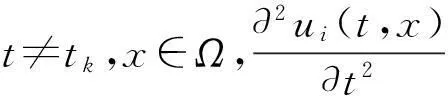
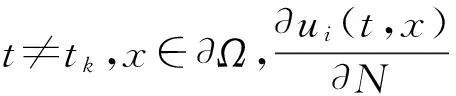
定义2称数值函数ν(t,x)：G→R为非振动的，若它最终为正或最终为负；反之，称ν(t,x)为振动的。称向量函数u(t,x)：G→Rm为非振动的，若它的每一分量都是非振动的；称向量函数u(t,x)：G→Rm为振动的，若它至少有一分量作为数值函数是振动的。称向量函数u(t,x)：G→Rm为强振动的，若它的每一个分量作为数值函数都是振动的。

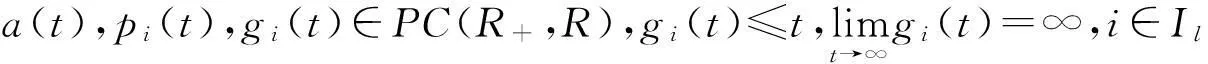
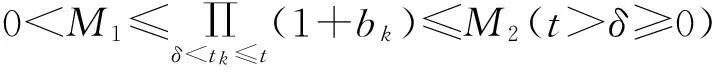
且有

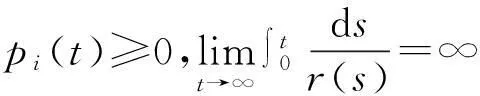
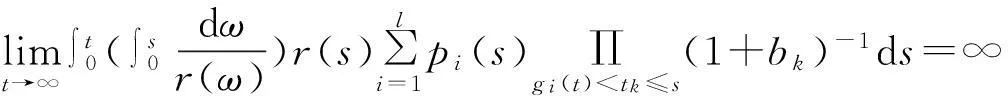
则脉冲时滞微分不等式

为叙述方便，引入记号：Ui(t)=∫Ωui(t,x)dx，t≥0，i∈Im。

2主要结果及其证明

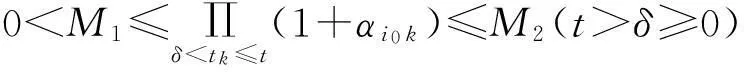
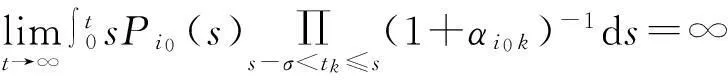
(3)

则系统(1)～(2)的所有非零有界解在区域G内振动。

证明(用反证法)假设系统(1)～(2)有一个非振动的有界解u(t,x)=(u1(t,x),u2(t,x),…,um(t,x))T，则∀i∈Im，u(t,x)在G内非振动，当然也有ui0(t,x)在G内非振动。不失一般性，设ui0(t,x)最终为正，于是存在T>0，使得∀(t,x)∈[T,∞)×Ω，有ui0(t,x)>0，ui0(t-τ,x)>0，ui0(t-σ,x)>0，ui0(t-ρ,x)>0。

考虑下面的方程：

(4)

(Ⅰ)当t≥T，t≠tk，k∈I∞时，对(4)式的第一式两边关于x在Ω上积分，有

∫Ωpi0(t,x)ui0(t-σ,x)dx-

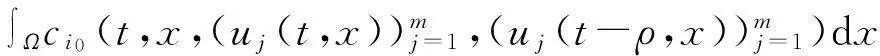
(5)

由Green公式，边值条件(2)及条件(H2)有

∫Ωai0i0(t,ui0(t,x))Δui0(t,x)dx=

∫Ωai0j(t,uj(t,x))Δuj(t,x)dx=

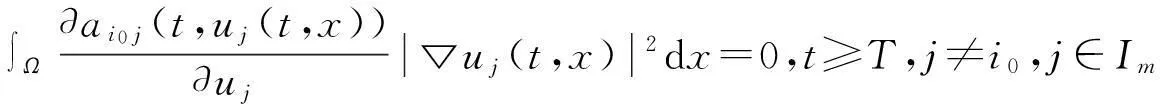
其中dS是∂Ω上的面积元素，

即

t≥T，j∈Im。

(6)

同理

∫Ωbi0j(t,uj(t-τ,x))Δuj(t-τ,x)dx=

(7)

由条件(H3)易知

(8)

因此由(5)～(8)式及条件(H1)可得

U″i0(t)+Pi0(t)Ui0(t-σ)≤0，t≥T。

(9)

(Ⅱ)当t≥T，t=tk，k∈I∞时，由(4)式中的脉冲条件，结合条件(H4)及定义1.1中的条件①可得

∫Ωui0(tk,x)dx=αi0k∫Ωui0(tk,x)dx=αi0kUi0(tk)，

即

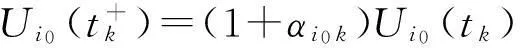
(10)

即

(11)

从而可知脉冲时滞微分不等式(9)～(11)有最终有界正解Ui0(t)。另一方面，结合条件(4)，由引理1知，脉冲时滞微分不等式(9)～(11)无最终有界正解，矛盾。

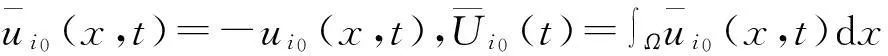
利用上面的结论，很容易得到下面的关于系统(1)～(2)强振动的结论。限于篇幅，其证明在此略去。

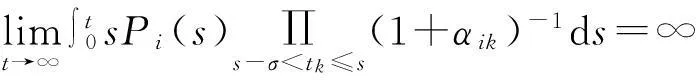
则系统(1)～(2)的所有非零有界解在区域G内强振动。

3结语

本文讨论了一类拟线性脉冲时滞双曲系统(1)～(2)非零有界解的(强)振动性问题，所用方法异于参考文献[1-6]中所用的通常的垂直相加法，但仍然建立了判别这类系统每个非零有界解(强)振动的显式充分性条件，结果充分反映了脉冲量和时滞量在系统(强)振动中的决定性作用，这是一个重要的结论。由本文结果易知，若系统(1)～(2)强振动，则系统(1)～(2)必振动。“强振动性”的判别条件比“振动性”的判别条件更强些。本文结果可为解决生物学、物理学、工程学、气象学、医学、化学和控制理论等学科领域中的一些实际问题提供数学理论依据和科学基础。

参考文献：

[1]罗李平, 罗振国, 曾云辉. 基于脉冲控制的非线性时滞双曲系统的振动分析[J]. 系统科学与数学, 2013, 33(9): 1024-1032.

LuoLP,LuoZG,ZengYH.OscillationAnalysisofnonlineardelayhyperbolicsystemsbasedonimpulsivecontrol[J].JournalofSystemandMathematicalScience, 2013, 33(9): 1024-1032.

[2]LuoLP,LiaoJD,GaoZH.Oscillationofsystemsofimpulsivedelayhyperbolicequations[J].InternationalJournalofAppliedMathematicsandApplications, 2008, 1(2): 147-154.

[3]罗李平. 具非线性扩散系数的脉冲时滞双曲型方程组的振动性[J]. 自然科学进展, 2008, 18(3): 341-344.

LuoLP.Oscillationofsystemsofimpulsivedelayhyperbolicequationswithnonlineardiffusioncoefficient[J].ProgressofNaturalScience, 2008, 18(3): 341-344.

[4]罗李平, 欧阳自根. 脉冲中立型时滞抛物偏微分方程组的振动准则[J]. 应用数学学报, 2007, 30(5): 822-830.

LuoLP,OuyangZG.Oscillationcriteriaofsystemsofimpulsiveneutraldelayparabolicpartialdifferentialequations[J].ActaMathematicaeApplicataeSinica, 2007, 30(5): 822-830.

[5]LuoLP,PengBY,YangL.Oscillationofsystemsofimpulsivedelayparabolicequationsaboutboundaryvalueproblems[J].AnnofDiffEqs, 2007, 23(4): 470-476.

[6]LuoLP.Oscillationtheoremofsystemsofquasilinearimpulsivedelayhyperbolicequations[J].NortheastMathJ, 2007, 23(3): 255-262.

[7]罗李平, 俞元洪. 具拟线性扩散系数的脉冲中立型抛物系统的(强)振动性[J]. 振动与冲击, 2011, 30(8): 183-186.

LuoLP,YuYH. (Strong)oscillationforsystemsofimpulsiveneutralparabolicequationswithquasilineardiffusioncoefficient[J].JournalofVibrationandShock, 2011, 30(8): 193-196.

[8]ZhangYT,LuoQ.Ontheforcedoscillationofsolutionsforsystemsofimpulsiveneutralparabolicdifferentialequationswithseveraldelays[J].JofMath. (PRC), 2006, 26(3): 272-276.

[9]LiWN.Ontheforcedoscillationofsolutionsforsystemsofimpulsiveparabolicdifferentialequationswithseveraldelays[J].JComputApplMath, 2005, 181(1): 46-57.

[10]YanJR.Oscillationpropertiesofasecond-orderimpulsivedelaydifferentialequation[J].ComputersMathApplic, 2004, 47: 253-258.

AMS Subject Classifications：35B05,35R12,35L99

责任编辑陈呈超

(Strong) Oscillation Analysis of Quasilinear Hyperbolic Systems Based on Effect of Impulse and Delay

LUO Li-Ping, LUO Zhen-Guo, ZENG Yun-Hui

(College of Mathematics and Statistics, Hengyang Normal University, Hengyang 421002, China)

Abstract：Impulsive partial differential systems can be successfully used for mathematical simulation in mechanics, theory physics, chemistry, population dynamics, biotechnology, optimal control and economics, etc. The oscillation theory is the one of the important branches of qualitative theory of impulsive partial differential systems. Therefore, it is of great theoretical and practical value to research the oscillation of partial functional differential equations. In this paper, the (strong) oscillation problems for a class of quasilinear hyperbolic systems based on the effect of impulse and delay are considered. Starting from the definition of oscillation directly, the oscillatory problems to the systems are reduced to the problem of which impulsive delay differential inequality hasn’t eventually position solution by employing a new technique of treating quasilinear diffusion term and Green’s divergence theorem and second boundary condition, and thereby some sufficient criteria are obtained for the (strong ) oscillation of all bounded solutions of such systems under second boundary condition via some results of second order impulsive delay differential inequality. The conclusions fully indicate that the system oscillation are caused by impulse and delay, and the criterion of strong oscillation is more sharp than the one of oscillation.

Key words：hyperbolic system; (strong) oscillation; quasilinear diffusion term; impulse; delay

DOI：10.16441/j.cnki.hdxb.20140067

中图法分类号：O175.27

文献标志码：A

文章编号：1672-5174(2016)04-149-04

作者简介：罗李平(1964-)，男，教授，研究方向：(脉冲)偏微分系统解的性态。E-mail:luolp3456034@163.com

收稿日期：2014-03-03；

修订日期：2015-12-10

*基金项目：湖南省“十二五”重点建设学科项目(湘教发[2011]76号)；湖南省普通高校青年骨干教师培养对象项目(湘教通[2015]361号)；湖南省自然科学基金面上项目(2016JJ2008)资助

Supported by the Construct Program of the Key Discipline in Hunan Province([2011]76)， the Program of Hunan Province Ordinary University Youth Backbone Teacher Training Object([2015]361) ; The Program of Hunan Province Natural Science Foundation(2016JJ2008)