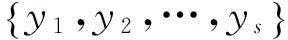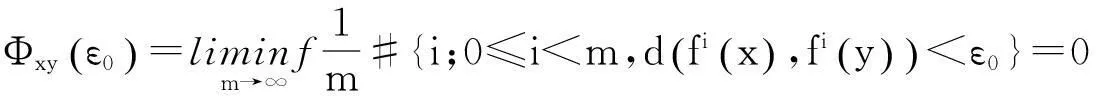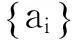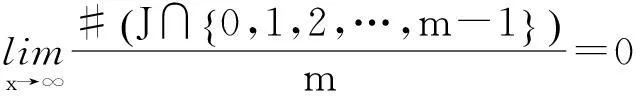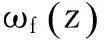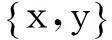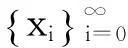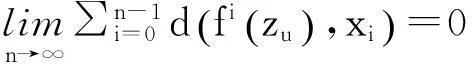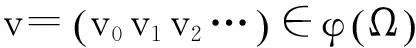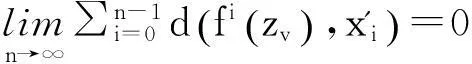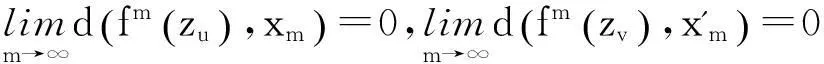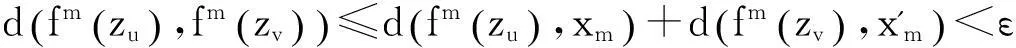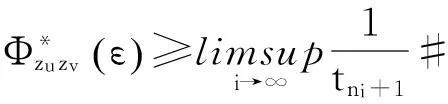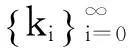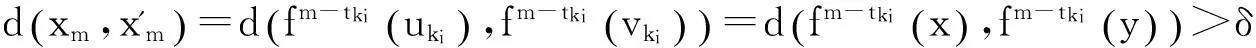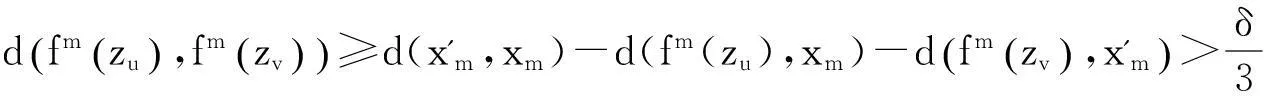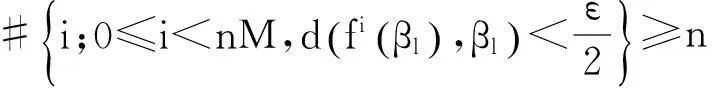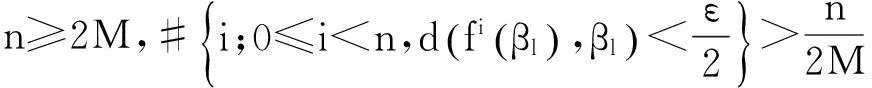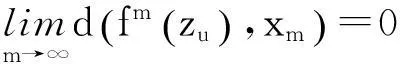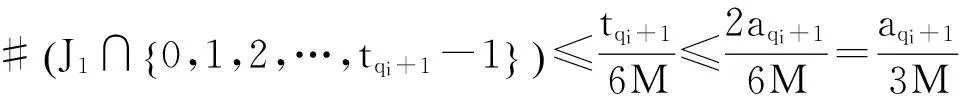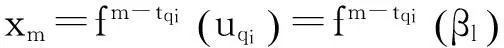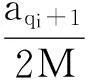渐近平均伪轨跟踪性质、弱specification性质和分布混沌

王立冬，王　翔，刘　恒

(1.大连民族大学 a理学院；b环境与资源学院，辽宁 大连116605；

2.大连理工大学 数学科学学院，辽宁 大连 116024)



渐近平均伪轨跟踪性质、弱specification性质和分布混沌

王立冬1a,2，王翔1b,2，刘恒1a,2

(1.大连民族大学 a理学院；b环境与资源学院，辽宁 大连116605；

2.大连理工大学 数学科学学院，辽宁 大连 116024)

摘要：证明了有渐近平均伪轨跟踪性质的非平凡紧致动力系统具有一致分布混沌或者按序列分布混沌。此外，在具有渐近平均伪轨跟踪性质系统中的分布混沌在测度中心是一致和稠密的，即有一个不可数的一致分布混沌集是由这样的点组成，它们的轨道闭包包含测度中心。作为一个推论，具有弱specification性质的系统也有类似的结果。

关键词：渐近平均伪轨跟踪；弱specification性质；分布混沌；按序列分布混沌

最近，在具有specification性系统中的分布混沌已被深入研究[1-5]。最新的一个成果是，具有Specification性的系统一定具有一致稠密的分布混沌，且若它有一个不动点，可表现出稠密不变的一致分布混沌[5]。

既然弱specification性和AASP都比specification性质弱，那么就有这样一个问题：它们中任何一个是否具有分布混沌？尽管文献[6]中证明了具有AASP和两个周期点的系统一定具有李约克混沌，但距离这个问题的答案还很远。

本文对这个问题做出了正面的解答，带有AASP(或者弱specification性质)的非平凡系统如果有两个弱周期点，则具有一致分布混沌。

1预备知识

设(X,d)是一个至少有两个点的紧致度量空间。f:X→X是连续映射。

f的测度中心记为M(f), 是所有不变测度的所有支撑集并的闭包。易知，X-M(f)对任何不变测度都是零测度集。如果对于任意ε>0 存在正整数M使得对所有n≥1,#{i;0≤i

0=j1≤k1

存在点z∈X 使得下列条件成立：

(1)d(fi(z),fi(ym))<δ 对于所有m≤s以及i,(jm≤i≤km) 成立；

(2)fn(z)=z, n=ks+Nδ;

就称f有specification性。

定义2[8-9]如果S⊂X 包含至少两个点，对于任意x,y∈S 且x≠y 时满足：

就称S 是一个分布混沌集。如果f有一个不可数分布混沌集，称f分布混沌。

2渐近平均伪轨跟踪的非平凡系统

本节证明如果一个具有AASP的非平凡系统(或者弱specification性)有至少两个几乎周期点，那么它具有一致分布混沌，否则它具有按序列的分布混沌。

由引理1， 存在稠密度为零的集合J1,J2⊂+满足当m∉J1时并且当m∉J2时。

令J=J1∪J2，则J也是稠密度为零的集合，这样对于m∉J都有

对所有满足tni≤m

=1。

假设i足够大且满足tki≥K则对于满足tki≤m

因此

对所有满足tki≤m

=0。

因此，对所有

推论1假设f有弱specification性并且有一个具有全支撑集的不变测度，那么f具有传递一致分布混沌。

参考文献：

[1]DOLEŽELOVáJ.Distributionallyscrambledinvariantsetsinacompactmetricspace[J].NonlinearAnalysis:Theory,Methods&Applications,2013,79:80-84.

[2]OPROCHAP.Specificationpropertiesanddensedistributionalchaos[J].DiscreteandContinuousDynamicalSystems,2007,17: 821-833.

[3]OPROCHAP,TEFáNKOVáM.Specificationpropertyanddistributionalchaosalmosteverywhere[J].ProceedingsoftheAmericanMathematicalSociety, 2008, 136: 3931-3940.

[4]SKLARA,SMíTALJ.Distributionalchaosoncompactmetricspacesviaspecificationproperties[J].Journalofmathematicalanalysisandapplications, 2000, 241: 181-188.

[5]WANGH,WANGLD.Theweakspecificationpropertyanddistributionalchaos[J].NonlinearAnalysis:Theory,Methods&Applications, 2013,91: 46-50.

[6]NIUYX,SUSB.Onstrongergodicityandchaoticityofsystemswiththeasymptoticaverageshadowingproperty[J].Chaos,Solitons&Fractals， 2011, 44: 429-432.

[7]ZHOUZL.Weaklyalmostperiodicpointandmeasurecenter[J].ScienceinChinaSeriesA,1993, 36 : 142-153.

[8]SCHWEITZERB,SMíTALJ.Measuresofchaosandspectraldecompositionofdynamicalsystemsoftheinterval[J].TransAmerMathSoc, 1994,344:737-754.

[9]OPROCHAP.Distributionalchaosrevisited[J].TransAmerMathSoc, 2009,361: 4901-4925.

[10]WANGLD,HUANGGF,HUANSM.Distributionalchaosinasequence[J].NonlinearAnalysis:Theory,Methods&Applications, 2007,67: 2131-2136.

[11]WALTERSP.AnIntroductiontoErgodicTheory[M].NewYork:Springer-verlag, 2000: 43.

(责任编辑邹永红)

Asymptotic average shadowing property, almost

specification property and distributional chaos

WANG Li-dong1,2,WANG Xiang2,3,LIU Heng1,2

(1.School of Science, Dalian Minzu University, Dalian Liaoning 116605, China;

2.School of Mathematical Science, Dalian University of Technology, Dalian Liaoning 116024, China;

3. School of Environment and Resource, Dalian Minzu University, Dalian Liaoning 116605, China;)

Abstract:It is proved that a non-trivial compact dynamical system with asymptotic average shadowing property displays uniformly distributional chaos or distributional chaos in a sequence. Moreover, distributional chaos in a system with asymptotic average shadowing property can be uniform and dense in the measure center, that is, there is an uncountable uniformly distributionally scrambled set consisting of such points that the orbit closure of every point contains the measure center. As a corollary, the similar results hold for the system with almost specification property.

Key words:asymptotic average shadowing property; almost specification property; distributional chaos; distributional chaos in a sequence

中图分类号：O189

文献标志码：A

文章编号：2096-1383(2016)01-0043-04

作者简介：王立冬(1955-)，男，吉林德惠人，教授，博士，学校特聘教授，博士生导师，主要从事拓扑动力系统研究。

基金项目：国家自然科学 (11271061)；中央高校基本科研业务费专项资金资助项目(DC201502050201)。

收稿日期：2015-11-19；最后修回日期：2015-11-27