结构方阵秩亏为1的可信性验证
2013-12-03李喆,周蕊
李 喆,周 蕊
(长春理工大学 理学院,长春 130022)

定理1[10]设A∈n×n.若对某个b∈n,c∈n,(n+1)×(n+1)矩阵非奇异,则rankA=n-1当且仅当线性方程组的解满足条件f=0.
定理2[11]输入一区间矩阵A∈In×n及区间右端列向量b∈In,若Verifylss函数[12]成功输出区间向量X⊂In,则X满足条件对某个⊆X.
利用区间牛顿迭代法可以验证非线性方程的解.
定理3[11]令f:为可微函数,X=(x1,x2)∈I且给定假设0∉f′(X),利用区间运算,定义若⊂X,则X内包含f的唯一解.若Ø,则对所有的x∈X,f(x)≠0.

定义边界矩阵
(1)
其中b,c∈n.则当时,矩阵非奇异,且在向量附近,线性方程组
(2)
本文利用隐行列式方法[13]计算梯度f(ε),其理论基于数值二分法[8,10].对线性方程组(2)关于每个变量εi求导,得
(3)
因此可以通过求解k个具有相同系数矩阵,但不同右端列向量的线性方程组得到f(ε).
下面基于文献[14]的结果通过将非线性方程f(ε)=0的某些变元做特定化方法,将验证f(ε)=0的解转化为验证具有一个变元非线性方程的解.设
(4)
定义
(5)


1) 选择i0满足式(4).

3) 若步骤2)不收敛,则输出算法失败.
引入参数向量ε=(ε1,ε2,ε3,ε4,ε5,ε6),定义参数区间对称矩阵
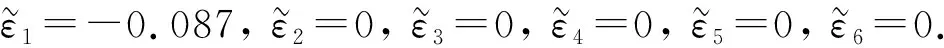
1) 确定i0=1;
2) 令E1=(-0.088,-0.086);
3) 计算参数向量U=(0.077 4,0.077 5);

[1] Rump S M.Verified Bounds for Singular Values,in Particular for the Spectral Norm of a Matrix and Its Inverse [J].BIT Numerical Mathematics,2011,51(2):367-384.
[2] Nishi T,Rump S M,Oishi S.On the Generation of Very Ill-Conditioned Integer Matrices [J].Nonlinear Theory and Its Applications,2011,2(2):226-245.
[3] Rump S M.Verified Bounds for Least Squares Problems and Underdetermined Linear Systems [J].SAIM J Matrix Anal Appl,2012,33(1):130-148.
[4] Rump S M.Accurate Solution of Dense Linear Systems,Part Ⅰ:Algorithms in Rounding to Nearest [J].Journal of Computational and Applied Mathematics,2013,242:157-184.
[5] Rump S M.Accurate Solution of Dense Linear Systems,Part Ⅱ:Algorithms Using Directed Rounding [J].Journal of Computational and Applied Mathematics,2013,242:185-212.
[6] Govaerts W.Bordered Matrices and Singularities of Large Nonlinear Systems [J].Internat J Bifurcation Chaos,1995,5(1):243-250.
[7] Griewank A,Reddien G W.Characterisation and Computation of Generalised Turning Points [J].SIAM J Numer Anal,1984,21(1):176-185.
[8] Griewank A,Reddien G W.The Approximate Solution of Defining Equations for Generalized Turning Points [J].SIAM J Numer Anal,1996,33(5):1912-1920.
[9] Rabier P J,Reddien G W.Characterization and Computation of Singular Points with Maximum Rank Deficiency [J].SIAM J Numer Anal,1986,23(5):1040-1051.
[10] Griewank A,Reddien G W.The Approximation of Generalized Turning Points by Projection Methods with Superconvergence to the Critical Parameter [J].Numer Math,1986,48(5):591-606.
[11] Rump S M.Verification Methods:Rigorous Results Using Floating-Point Arithmetic [J].Acta Numerica,2010,19:287-449.
[12] Rump S M.Kleine Fehlerschranken Bei Matrixproblemen [D].Karisruhe:Karlsruhe University,1980.
[13] Spence A,Poulton C.Photonic Band Structure Calculations Using Nonlinear Eigenvalue Techniques [J].J Comput Phy,2005,204(1):65-81.
[14] CHEN Xiao-jun,Womersley R S.Existence of Solutions to Systems of Underdetermined Equations and Spherical Designs [J].SIAM J Numer Anal,2006,44(6):2326-2341.
(责任编辑:赵立芹)
研究简报
