三阶常系数拟线性泛函微分方程的周期解

2013-07-05田德生

纯粹数学与应用数学 2013年3期

关键词：三阶范数常数

田德生

(湖北工业大学理学院,湖北武汉 430068)

三阶常系数拟线性泛函微分方程的周期解

田德生

(湖北工业大学理学院,湖北武汉 430068)

研究了一个三阶泛函微分方程周期解的存在唯一性和全局吸引性:

这是一个常系数拟线性泛函微分方程.通过将这个方程转变为三维的拟线性微分方程(组),得到了这个方程存在唯一周期解的充分条件;通过选取适当的李雅普诺夫函数,推导了这个方程解的全局吸引性;进一步,得到了此方程周期解的全局吸引性.最后,举出了两个应用实例.

泛函微分方程;周期解;唯一性;全局吸引性

1 引言

考虑三阶泛函微分方程:

其中a,b,c,τ∈R(τ＞0)为常数;p(t)∈C(R,R)具有周期为T-函数(T为正常数); g∈C(R2,R)关于第一个变量为T-周期函数.本文研究此方程周期解的存在唯一性及全局吸引性.

近年来,三阶微分方程周期解的问题得到了广泛研究[19],所应用的研究方法也是多种多样.文献[1-3]对如下微分方程周期解的问题做了许多研究工作:

得到了方程在多种情况下周期解的存在性;文献[4-6]应用各种不动点定理,研究了三阶微分方程周期解的存在性问题;文献[7]应用Leray-Schauder非线性选择性原理的方法,研究了多种形式三阶微分方程的周期解问题;文献[8-9]应用重合度理论的延拓定理,分别研究了下面两个形式的三阶微分方程周期解的存在性问题:

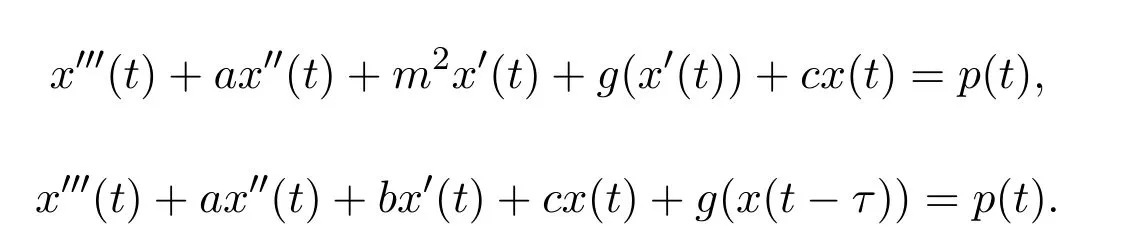
随着人们利用计算机对微分方程解数值计算的应用和普及,越来越迫切地要求人们研究微分方程解的存在唯一性和吸引性.而且,就现有的文献看,对三阶微分方程周期解的存在唯一性和全局吸引性问题的研究成果尚不多见.因此,本文对方程(1)周期解问题的研究是十分有意义的.

目前,许多数学工作者研究了生物模型周期解的全局吸引性问题[10-12],他们的研究方法主要是选择适当的李雅普诺夫函数,计算右上狄尼导数.本文将采用计算左上狄尼导数的方法,研究方程周期解的全局吸引性,这与他们的研究方法是不同的.一般地,计算右上导数的方法,需要估计李雅普诺夫函数的右上导数在无限区间[0,+∞)上的符号;而计算左上导数的方法,只需估计左上导数在某个有限区间[0,Ti]上的符号(详见定理3.1的证明).因此,本文采用的计算左上导数的方法更为简洁.

2 周期解的存在唯一性

在这一节,讨论方程(1)周期解的存在唯一性.首先,介绍本文所用到的引理,它是文献[13]中的定理3.考虑拟线性方程:

其中X∈C(R,Rn),t∈R为变量,A∈Rn×n为常数矩阵,F∈C(R×Rn,Rn)关于第一个变量为T-周期函数,τ∈R为常数.

引理2.1设T‖A‖＜2π,detA/=0,又设存在一个常数L≥0,它满足

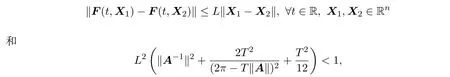
则方程(2)有唯一的T-周期解.这里,矩阵范数‖A‖是由向量范数‖X‖引导的,A∈Rn×n, X∈Rn.

在下面的讨论中,约定如下的向量范数和矩阵范数:

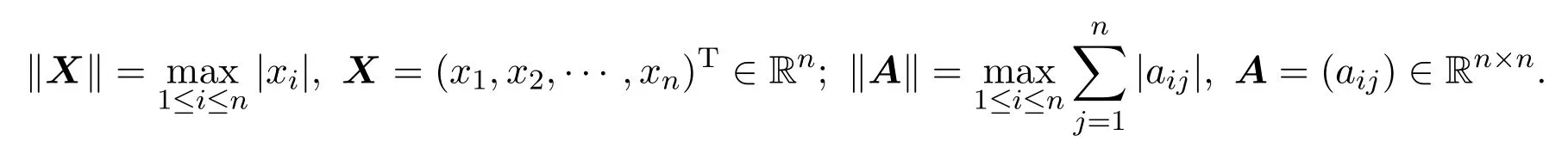
定理2.1设

(H1)存在常数L≥0,满足

那么方程(1)有唯一的T-周期解.

证明类似于定理2.1的证明,此处略去.

3 全局吸引性

在这一节,研究方程(1)的解和周期解的全局吸引性.在这里指出,说方程(1)的一个解x∗(t)是全局吸引的,如果方程(1)的其它任意解x(t)与x∗(t)满足如下的关系:

4 应用实例

最后提出:满足定理2.1条件的周期解是否也具有全局吸引性?这将是下一步的研究工作.

致谢作者对审稿专家提出的宝贵修改意见表示真诚的感谢!

[1]Ezeilo J O C.On the existence of periodic solution of a certain third-order differential equation[J].Pro. Cambridge Philos.Soc.,1960,56:381-389.

[2]Sedziwy S.On periodic solution of a certain third-order nonlinear differential equation[J].Ann.Polon Math., 1965,17:147-154.

[3]Afuwape A U,Omari P,Zanolin F.Nonlinear perturbation of differential operators with nontrivial kernel and application to third-order periodic boundary value problems[J].J.Math.Anal.Appl.,1989,143:35-56.

[4]Chu J,Zhou Z.Positive solutions for singular non-linear third-order periodic boundary value problems[J]. Nonlinear Analysis,2006,64:1528-1542.

[5]Feng Y.On the existence and multiplicity of positive periodic solutions of a nonlinear third-order equation[J]. Appl.Math.Lett.,2009,22:1220-1224.

[6]Prasad K R,Murali P.Multiple positive solutions for nonlinear third order general three-point boundary value problems[J].Differential Equations and Dynamical Systems,2008,16:63-75.

[7]John R G,Chattanooga.Positive solutions for third order multi-point singular boundary value problems[J]. Czechoslovak Math.J.,2010,60:173-182.

[8]Zhang Z,Wang Z,Yu J.On the existence of periodic solutions of third order functional differential equations[J].Funkcialaj Ekvacioj,2000,43:461-469.

[9]Amster P,P De N´apoli,Mariani M C.Periodic solutions of a resonant third-order equation[J].Nonlinear Analysis,2005,60:399-410.

[10]Wang Q,Jizhou.Existence and attractivity of a periodic solution for a ratio-dependent Leslie system with feedback controls[J].Nonlinear Analysis:RWA,2011,12:24-33.

[11]Zhang Z,Wang H.Existence and global attractivity of positive periodic solutions for a generalized predatorprey system with time delay[J].Math.and Computer Modelling,2006,44:188-203.

[12]Li Zhong,Chen F,He M.Permanence and global attractivity of a periodic predator-prey system with mutual interference and impulses[J].Nonlinear Sci.Numer.Simulat.,2012,17:444-453.

[13]马世旺,庾建设,王志成.拟线性泛函微分方程周期解的存在唯一性[J].数学年刊:A辑,2001,22(1):105-110.

Periodic solution of a third-order quasilinear functional differential equation with constant coefficients

Tian Desheng

(College of Sciences,Hubei University of Technology,Wuhan 430068,China)

This paper considers the existence,uniqueness and global attractivity of a periodic solution for a third-order functional differential equation:

which is a third-order quasilinear functional differential equation with constant coeffcients.By converting this equation into a three-dimensional quasilinear one,the sufficient conditions for the existence of exactly one periodic solution of this equation are established.By constructing suitable Lyapunov functionals,the global attractivity of a solution for the above equation is established;Moreover,the global attractivity of a periodic solution is established.In the last section,two examples will be provided to illustrate the applications of the results.

functional differential equation,periodic solution,uniqueness,global attractivity