一类基于比率的且具有收获率和时滞的捕食系统的周期解

王晖

(内蒙古民族大学数学学院,内蒙古通辽 028000)

一类基于比率的且具有收获率和时滞的捕食系统的周期解

王晖

(内蒙古民族大学数学学院,内蒙古通辽 028000)

研究一类基于比率且具有收获和时滞的捕食系统.证明了系统正周期解的存在性,并通过构造适当的Lyapunov泛函,给出了正周期解全局稳定的充分条件.

时滞;收获率;比率依赖;周期解;Lyapunov泛函

DO I：10.3969/j.issn.1008-5513.2013.05.012

1 正周期解的存在性

生物种群的持续生存是数学生态学中捕食理论及其有关课题的一个重要方面.近年来,越来越多的生物学和生理学证据表明,在许多情形下,特别是当捕食者不得不搜寻食物(因此不得不分享或竞争食物)时,更切合实际且更一般的捕食-被捕食模型应基于比率依赖理论.很多学者在这方面做出了贡献[15].具有收获率的系统,由于存在开发项,往往表现出复杂的动力学行为.目前已有很多学者研究了此类模型[46].本文考虑了基于比率的HollingⅢ型功能反应的且同时具有时滞和收获率的捕食模型:

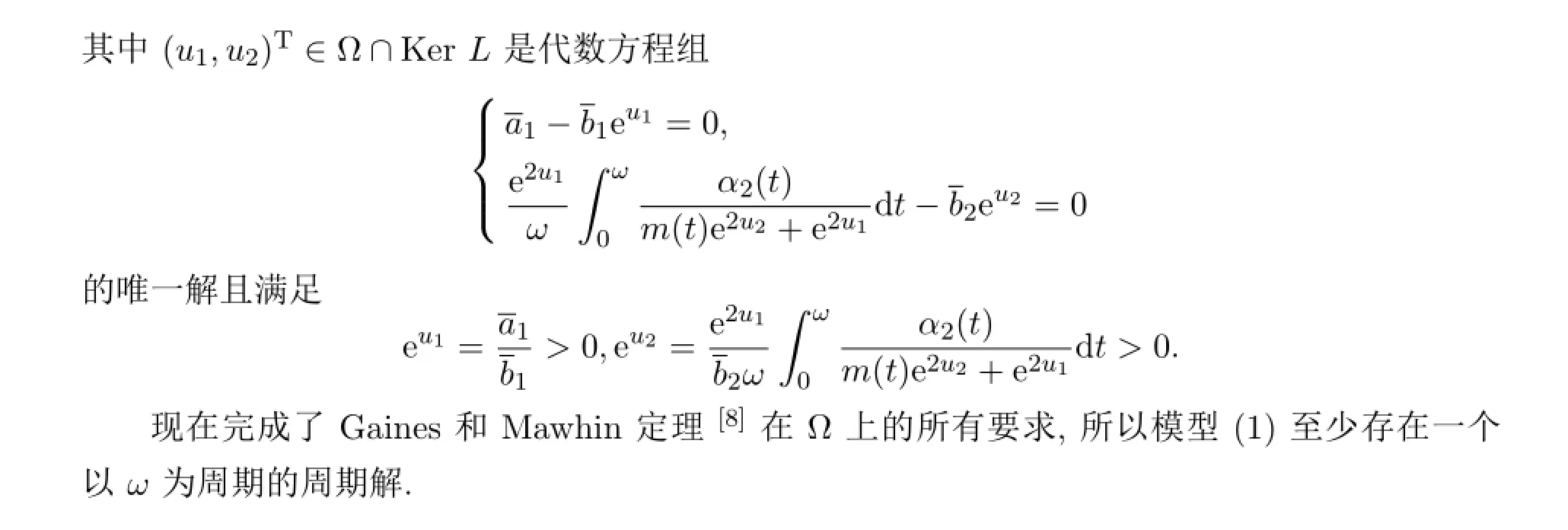
2 正周期解的唯一性及全局稳定性

参考文献

[1]Edoardo Beretta.G lobal analyses in som e delayed ratio-dependent predator-prey system s[J].Non linear analysis Theory M ath App lic.,1997,32(3):381-408.

[2]Tang S Y,Chen L S.G lobal qualitative analysis for a ratio-dependent predator-prey modelw ith delay[J]. M ath.Anal.App l.,2002,266:401-419.

[3]吴亭.基于比率依赖Bedd ington-DeAngelis功能反应的离散n-种群竞争-捕食系统的持久性[J].纯粹数学与应用数学,2010,27(4):437-441.

[4]Zhang R Y,Wang Z C,Chen Y,et al.Periodic solution of a single species discrete popu lation modelwith periodic harvest/stock[J].Com puters and Mathematicsw ith App lications,2000,39:77-90.

[5]Zhang Z Q,Tang H S.Fou r positive period ic solutions for the f rst order d if erential system[J].J.M ath. Anal.App l.,2007,332:123-136.

[6]秦发金,姚晓洁,黄燕革.一类具有收获率的时滞阶段结构捕食系统的多重正周期解[J].数学的实践与认识, 2010,40(4):134-143.

[7]许天周.应用泛函分析[M].北京:科学出版社,2002.

[8]Ganes R E,Mawhin J L.Coincidence Degree and Non linear Dif erential Equation[M].Berlin:Sp ringer Verlag,1997.

[9]Barbalat I.System s d′equations d if erentielle d′oscillations non lin raires[J].Rev.Roum aine M ath.Pu res. App l.,1959,4:267-270.

The period ic solu tions of ratio-dependen t p redator-p rey m odel w ith harvesting and tim e delay

Wang Hui

(Mathematics College,Inner Mongolia University for the Nationalities,TongLiao 028000,China)

In this paper,we consider the Ratio-dependent predator-prey system w ith harvesting and time delay.The existence of positive period ic solu tion is proved,and by constructing app rop riate Lyapunov function, suf cient conditions are obtained to ensure the global stability of positive periodic solution.

time delay,harvesting,ratio-depedent,positive periodic solution,Lyapunov function

O175.13

A

1008-5513(2013)05-0520-09

2013-07-10.

王晖(1982-),硕士,讲师,研究方向：生物数学.

2010 MSC:34D 05