具有脉冲毒素投放和营养再生的恒化器模型

傅晓钒,张树文

(集美大学理学院,福建 厦门 361021)

具有脉冲毒素投放和营养再生的恒化器模型

傅晓钒,张树文

(集美大学理学院,福建 厦门 361021)

研究具有脉冲毒素投放和营养再生的恒化器模型.利用脉冲微分方程的比较定理和小扰动方法得到了边界周期解全局渐近稳定的充分条件,进而得到了系统持续生存的充分条件.结果表明毒素环境将会导致微生物种群的灭绝.

脉冲毒素投放;营养再生;恒化器;持续生存

1 引言

恒化器是进行微生物连续培养的一种实验装置,用于研究微生物在一个连续培养环境中的生长状况.恒化器模型的设计和研究对于食品加工,污水处理,转基因微生物的培养等都具有积极作用.近年来,已经有许多学者利用脉冲微分方程理论[12]解决各类生态学问题,如捕食-食饵系统[34],并且对各类条件下的恒化器模型进行了大量研究[57].文献[5]研究了具有阶段结构的单种群恒化器模型,文献[6]研究了具有脉冲输入和营养回收的单种群恒化器模型.文献[7]研究了具有脉冲扩散的污染环境下的恒化器模型.在自然环境中,潮水的冲刷既给微生物带来营养物,也带来了环境毒素.因此,本文建立了具有脉冲毒素投放的恒化器模型,用定期脉冲投放来模拟自然因素对微生物的影响,并且对死去微生物考虑营养回收的作用.

其中F(t)=a e-at,a＞0,且满足0≤b≤1,1δ≥1.S(t)表示t时刻营养物质的浓度,x(t)表示t时刻微生物的浓度,co(t)表示微生物体内毒素的浓度,ce(t)表示环境毒素的浓度,fce(t)表示微生物从环境中吸收毒素的浓度,-gco(t),-m co(t)分别表示微生物消除和净化毒素的浓度,-hce(t)表示流失的环境毒素的浓度,T表示脉冲周期,D表示冲洗速率,p表示投放的营养物质的浓度,q表示投放的环境毒素的浓度,b表示死去的微生物被重新转化为营养的部分,δ表示微生物的产生和消耗的营养物的比,r表示微生物的死亡率.

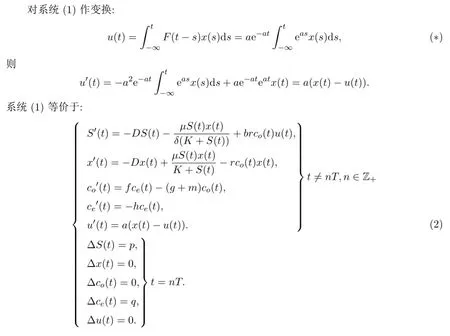
2 预备引理

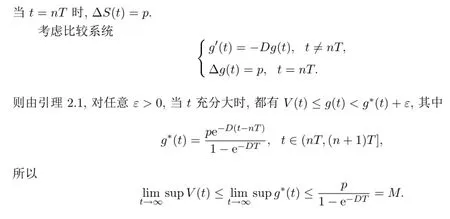
3 灭绝和持续生存

4 结论

通过上述讨论,得到了系统的一个临界值:

当Δ＜0时,系统(2)的边界周期解(S*(t),0,c*o(t),c*e(t),0)是全局渐近稳定的,这意味着微生物将趋于灭绝.当Δ＞0时,系统(2)是持续生存的.

[1] Laksmikantham V, Bainov D D. Theory of Impulsive Differential Equation[M]. Singapore: World Science, 1989.

[2] Bainov D D, Simeonov P S. Impulsive Differential Equations: Periodic Solutions and Applications[M]. London: Longman, 1993.

[3] 张树文,张耘嘉,谭德君.具脉冲效应和Beddington-DeAnglis功能反应时滞周期捕食系统[J].纯粹数学与应用数学,2010,26(4):534-540.

[4] Zhang Shuwen, Tan Dejun. Permanence in a food chain system with impulsive perturbations[J]. Chaos, Solitions and Fractals, 2009,40:392-406.

[5] Li Shuping, Pei Yongzhen. Stability analysis for a single-species chemostat model with age structure and contribution of population to resource[J]. Math. Chem., 2010,47:111-122.

[6] Teng Zhidong, Gao Rong. Global behaviors of Monod type chemostat model with nutrient recycling and impulsive input[J]. Math. Chem., 2010,47:276-294.

[7] Jiao Jianjun, Ye Kaili, Chen Lansun. Dynamical analysis of a five-dimensioned chemostat model withimpulsive diffusion and pulse input environmental toxicant[J]. Chaos, Solitions and Fractals, 2011,44:17-27.

A chemostat model with impulsive toxicant input and nutrient recycling

Fu Xiaofan,Zhang Shuwen

(School of Sciences, Jimei University, Xiamen 361021, China)

In this paper, we consider a chemostat model with impulsive toxicant input and nutrient recycling. By comparison theory and small amplitude perturbation method, we obtain the su±cient condition for the global asymptotic stability of boundary periodic solution. Furthermore, the su±cient condition for the permanence of the system is obtained. The results show that poisonous environment will lead the microorganism species to be extinct.

impulsive toxicant input, nutrient recycling, chemostat, permanence

O175.12

A

1008-5513(2012)02-0262-07

2011-12-09.

福建省自然科学基金(2008J0199).

傅晓钒(1987-),硕士生,研究方向：生物数学.

2010 MSC:34D05,34D20