循环矩阵反问题的最小二乘解
2011-12-08张湘林李云翔
张湘林,李云翔
(湖南城市学院数学与计算科学学院,中国益阳 413000)
循环矩阵反问题的最小二乘解
张湘林*,李云翔
(湖南城市学院数学与计算科学学院,中国益阳 413000)
讨论了一类循环矩阵反问题的最小二乘解,给出了解的存在定理和解的一般表达式.考虑了给定矩阵的最佳逼近问题,证明了问题存在唯一解,给出了唯一解的表达式,最后给出了两个数值算例.
循环矩阵;矩阵反问题;矩阵范数;最小二乘解

本文第2节首先分析了循环矩阵的结构特性,然后给出了问题Ⅰ和问题Ⅱ的解的表达式,第3节给出了求解问题Ⅱ的2个数值例子.
1 问题Ⅰ和问题Ⅱ的解


3 数值算法与实例
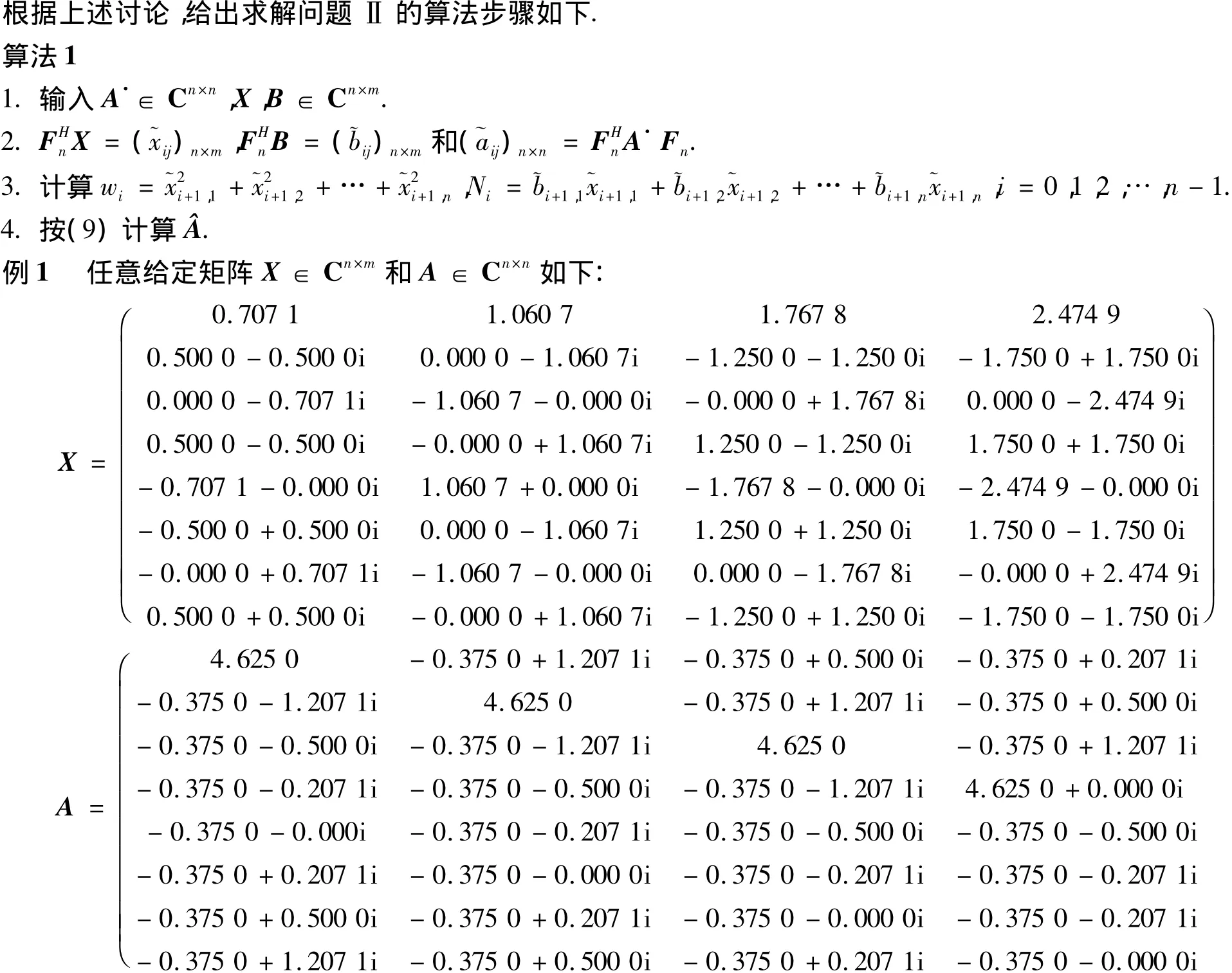
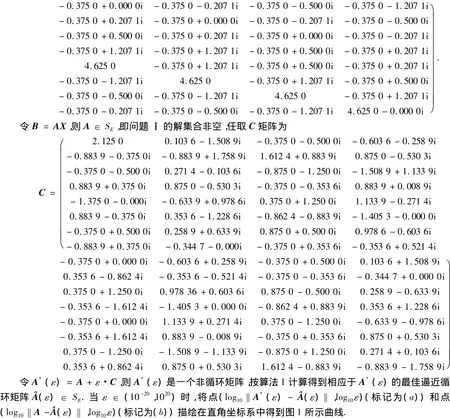

图1相应于A*(ε)的最佳逼近循环矩阵图示

表1 ‖-A*‖与‖-A0‖的结果比较
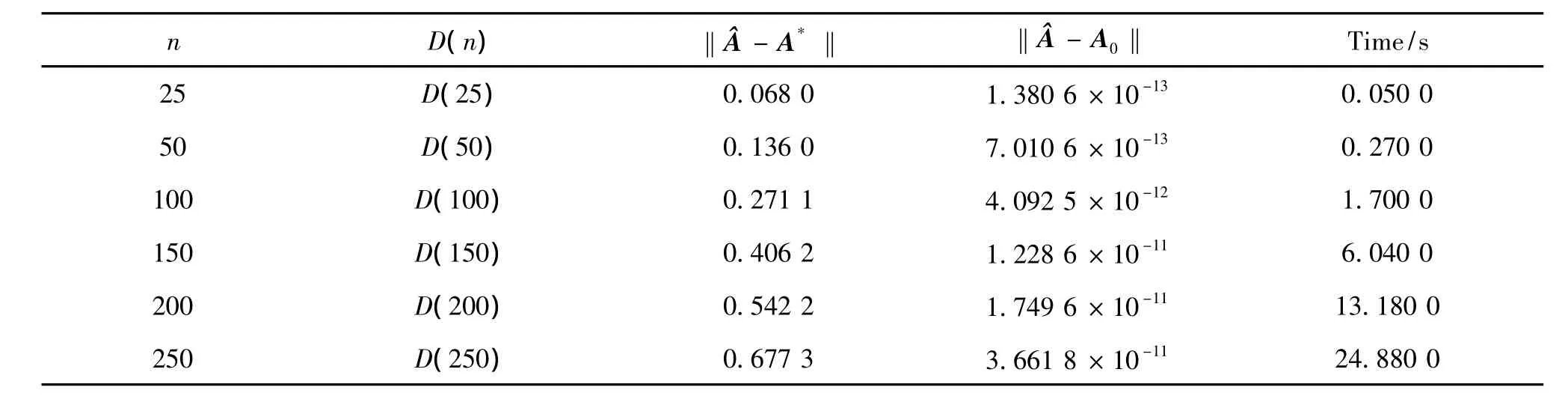
表1 ‖-A*‖与‖-A0‖的结果比较
Time/s 25 D(25) 0.068 0 1.380 6 × 10-13 0.nD(n) ‖^A-A*‖ ‖^A-A0‖880 0 050 0 50 D(50) 0.136 0 7.010 6 × 10-13 0.270 0 100 D(100) 0.271 1 4.092 5 × 10-12 1.700 0 150 D(150) 0.406 2 1.228 6 × 10-11 6.040 0 200 D(200) 0.542 2 1.749 6 × 10-11 13.180 0 250 D(250) 0.677 3 3.661 8 × 10-11 24.
其中‘Time(s)’表示程序的CPU运行时间.
[1]DAVIS P J.Circulant matrics[M].New York:John Wiley Sons,1979.
[2]徐 仲,张凯院,陆 全.TOEPLITZ矩阵类的快快速算法[M].西北工业大学出版社,1999.
[3]陈景良,陈向晖.特殊矩阵[M].北京:清华大学出版社,2001.
[4]郭训香,吴冬香.循环矩阵的一些性质[J].赣南师范学院学报,2007(6):8-9.
[5]李天增,王 瑜.循环矩阵的性质及求逆方法[J].四川理工学院学报:自然科学版,2009,22(4):47-49.
[6]张贤达.矩阵分析与应用[M].北京:清华大学出版社,2004.
[7]黄廷祝,钟守铭,李正良.矩阵理论[M].北京:高等教育出版社,2003.
[8]PENG Z Y.The inverse eigenvalue problem for hermitian anti-reflexive matrices and its approximation[J].Appl Math Comput,2005,162(3):1337-1389.
[9]彭振赟.几类约束矩阵反问题[D].长沙:湖南大学,2003.
[10]袁中扬.几类循环矩阵的算法及其反问题的最小二乘解[D].西安:西安电子科技大学,2006.
Least-Square Solution to Inverse Problem of Circulant Matrices
ZHANG Xiang-lin*,LI Yun-xiang
(College of Mathematics and Computing Science,Hunan City University,Yiyang 413000,China)
The least-square solution to inverse problem of one kind of circulant matrix is presented.The existence theorems are obtained,and a general representation of such a matrix is presented.In addition,the nearst matrix problem for the given matrix is discussed.The unique nearst matrix solution and an expression for this nearest matrix is provided.Two examples are given at last.
circulant matrix;matrix inverse problem;matrix norm;the least-square solution
O241.6
A
1000-2537(2011)05-0017-05
2011-05-25
湖南省教育厅基金资助项目(10C0501);湖南城市学院教改基金资助项目(2011)
*
,E-mail:zxl030612@sina.com
(编辑 沈小玲)
