Decoherence of temporal quantum correlation in electrically controllable quantum-dots molecules
2023-10-07XIEJialingYANKaiTANJiaCAOZhaoliangHAOXiang
XIE Jia-ling,YAN Kai,TAN Jia,CAO Zhao-liang,HAO Xiang
(Jiangsu Key Laboratory of Micro and Nano Heat Fluid Flow Technology and Energy Application, School of Physical Science and Technology, Suzhou University of Science and Technology,Suzhou 215009, China)
* Corresponding author,E-mail: xhao@mail.usts.edu.cn
Abstract: The decoherence of temporal quantum correlation is explored in a voltage-controlled quantum dots molecule coupled to a cavity.The temporal correlation in the optoelectronic hybrid system is studied based on Leggett-Garg inequalities.The inequality violations can be interpreted as the existence of temporal quantum correlation during dynamical evolution.The temporal quantum correlation is enhanced by its electron tunnel’s strength and cavity frequency detuning.It is found that there is no temporal quantum correlation in the regions where the values of spatial quantum correlation are zero and the maximal violations occur in conditions with high values of quantum correlation.In contrast, the spatial quantum coherence can still exsit when the value of temporal quantum correlation is zero.The method of open quantum system dynamic is used to study the effect of reservoir memory on temporal quantum correlation.The temporal quantum correlation can be suppressed due to the spontaneous decay of the quantum dots and cavity leakage.These results are helpful for quantum information processing technology in hybrid quantum systems.
Key words: temporal quantum correlation; quantum dots molecule; Leggett-Garg inequalities; hybrid quantum systems
1 Introduction
Quantum correlations[1-2]have become necessary resources in quantum information sciences and technologies[3-5].The presence of nonclassical correlations can distinguish quantum worlds from classical worlds[6-8].Bell inequalities[9]play a fundamental role in the nature of correlations between spatially separated systems.Test of the violations of Bell or Clauser-Horne-Shimony-Holt (CHSH) inequalities can demonstrate quantum nonlocality[10].Spatial correlations can be quantitatively evaluated by quantum entanglement[11-12], quantum discord[13-14], quantum coherence[15-17]and other characteristics[18-19].From the viewpoint of time sequential measurements,Leggett and Garg put forward a similar inequality whose violation can be qualitatively used to estimate temporal correlations[20].Leggett-Garg inequalities (LGIs) must be verified by sequential measurements acting on a single system at different times,which is distinct from Bell inequalities that concern multiple parties spatially separated from each other.The violations of LGIs are inconsistent with two assumptions of macroscopic realism and noninvasive measurability[21].The macroscopic realism of physical states implies that measurements on a system simply reveal the values which exist at previous times.Noninvasive measurability ensures that such measurements can be performed without disturbing the dynamical evolution of the states.
In recent years, many experimental tests of LGIs have been carried out in setups including Rydberg atoms[22], photonic systems[23], superconducting circuits[24]and a quantum computer[25].With the rapid development of quantum engineering technologies, feasible quantum systems have extended from qubits to multilevel quantum systems[26-28].Among these fantastic quantum systems, electrically controllable quantum dots molecules have been extensively applied in quantum optics and condensed matter physics because of their high nonlinear optical susceptibility, large electrical dipole moments of band transition and great flexibility in designing devices[29-30].A unity of two or more quantum dots with closely spaced couplings can be referred to as a quantum dots molecule which is modeled as one kind of artificial multilevel atom[31].Electric voltages can be used to generate electron tunnels between two neighboring quantum dots[32].Meanwhile, level transitions can be induced by direct couplings between a cavity and a quantum dots molecule[33].How do electron tunnels, cavity couplings and environmental noises affect quantum correlations in such systems? This question motivates us to theoretically evaluate temporal and spatial quantum correlations in hybrid multilevel systems.
The structure of this paper is organized as follows.In Sec.2, we present witnesses about temporal and spatial quantum correlations.The general expression of LGI based on two-time correlation functions is given by the open system approach.Temporal correlations are qualitatively estimated by violations of LGI and quantum coherence based onl1-norm is used to measure spatial correlations.In Sec.3, we describe the model and Hamiltonian of an electrically controllable quantum dots molecule in a cavity.Quantum correlations with respect to level transitions are established by external voltages and cavity couplings.In Sec.4, we provide our main analytical and numerical results about the dynamics of temporal and spatial correlations.The explicit expressions of the LGI violation and quantum coherence are analytically obtained by the quantum dynamic method.The quantum master equation for a quantum dots molecule is exploited to numerically study environmental effects on temporal correlation.We will discuss our conclusions in the last section.
2 Quantum correlation in a general evolution
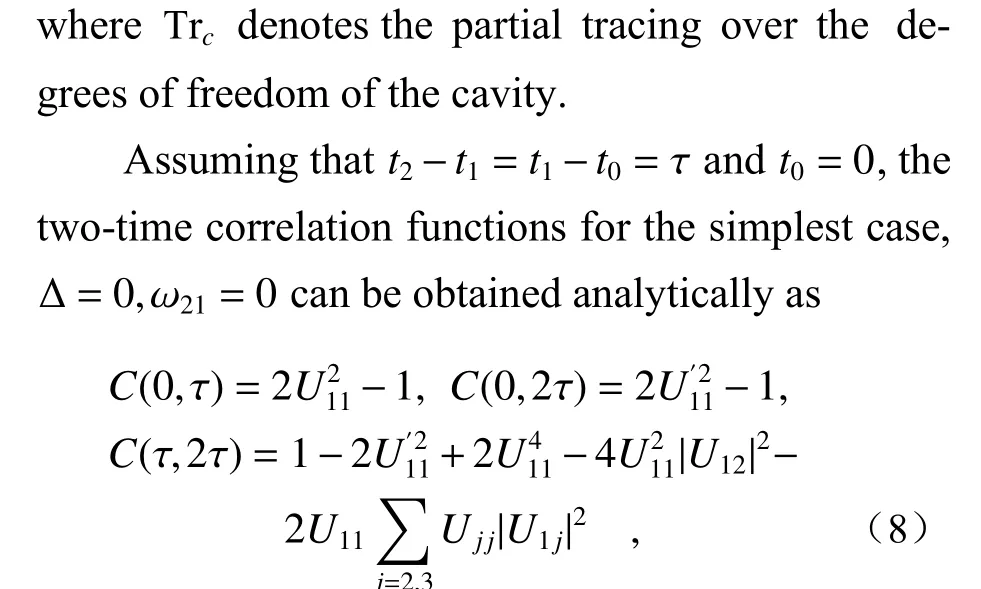
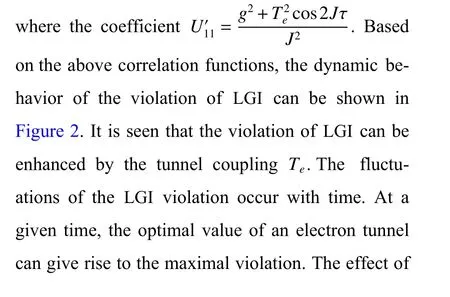
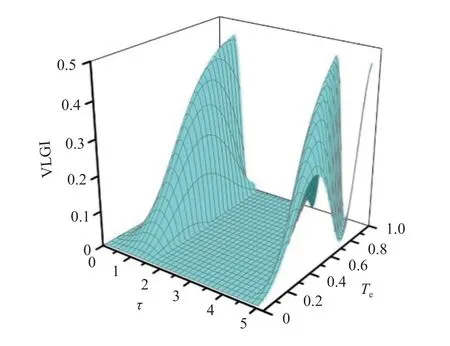
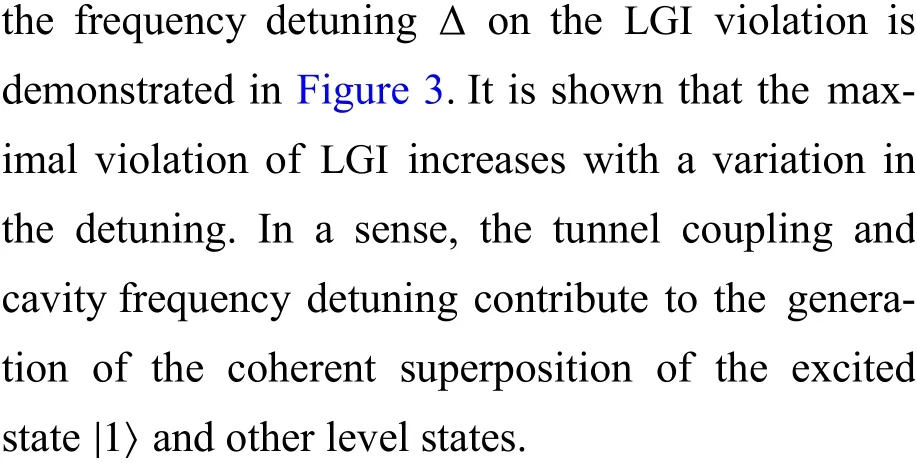
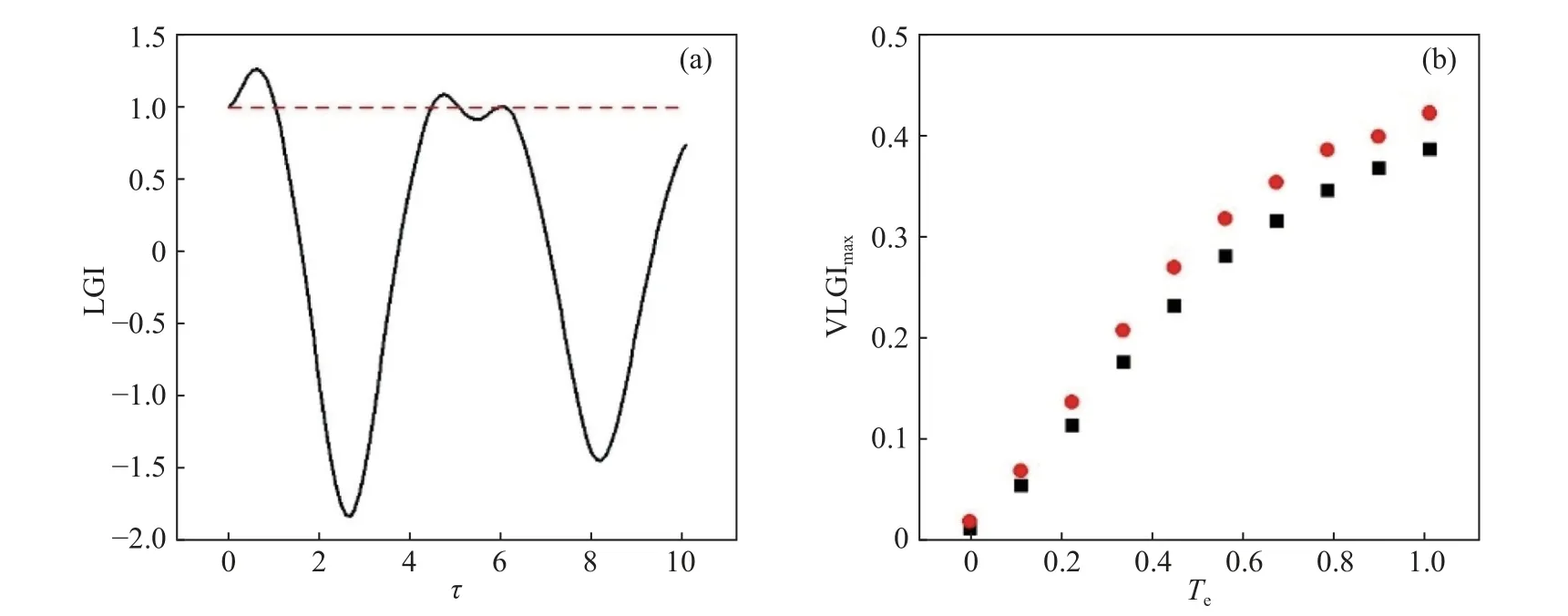
As a temporal analog version of Bell inequalities, one simple form of LGI[21]can be obtained by performing three measurements of time such thatt0 Joint measurability[18]plays an important role in determining two-time correlation functions. Spatial quantum correlation can be treated quantitatively by defining thel1-norm as a coherence measurement[17].Thel1-norm of a matrix is related to the absolute value of the elements of the matrix.Where a definite Hilbert space has a reference orthonormal basis {|j〉} and al1-norm of coher- Rapid development in laser and semiconductor technologies has made it possible to fabricate hybrid systems applied to quantum information tasks[33-36].Among these hybrid systems, quantum dots molecules coupled to cavities have attracted much attention in recent years.It is known that level transitions between different electronic states in quantum dots molecules can be excited by external voltages and laser fields.We consider a quantum dots molecule composed of two self-assembled(In,Ga)As/GaAsquantum dots with a lateral quantum interaction.The interdot coupling is controlled by an electrical voltage.This system is coupled to a microscopic cavity.A scheme for a quantum dots molecule coupled to the cavity is illustrated in Figure 1(a) (color online).Some present technologies can be exploited to produce a homogeneous ensemble of molecules consisting of two dots aligned along the [110] direction of the G aAs substrates[37].A scheme for a double coupled quantum dots system is illustrated in Figure 1(b) (color online).The ground state |0〉 denotes a level where two quantum dots are not excited.The state |2〉 represents a level in which an electron is directly excited by a laser to the conduction band in the left dot.The parameter∆is the frequency detuning between the laser’s driving and level transition.gdenotes the strength of electrical dipole interactions between a molecule and cavity fields.The application of electrical voltages induces the transfer of an electron from the left dot to the conduction band of the right dot.The indirectly excited state | 1 〉 stands for the level transition by interdot tunnels.Teis the strength of the tunnel coupling which can be varied by the external voltage.The level transition ω21is considered to be zero due to the negligible energy difference between the direct and indirect exciton. By means of the electric dipole and rotating wave approximation, the Hamiltonian of the hybrid system in the interaction picture can be expressed as The closed system evolves in accordance with the unitary generator of Eq.(5).For an initial state|Ψ(0)〉=|1〉|0〉c, the reduced density matrix of the quantum dots molecule after 6 measurements can be given by, Fig.2 The dynamics of the LGI violation are plotted as a function of the tunnel strength Te when g=1 ,∆=0 and ω21=0.The nonzero values of VLGI demonstrate the presence of temporal quantum correlation Fig.3 The maximal LGI violation varies with cavity frequency detuning.The parameters g=1 ,Te=0.5g and ω21=0 are chosen The reduced density matrix for the quantum dots molecule is given byρQ(τ)=Trc[U(τ)|Ψ(0)〉〈Ψ(0)|U+(τ)].The quantum coherence as a measurement of spatial correlation is obtained as Figure 4 (color online) illustrates the fluctuating behavior of temporal and spatial quantum correlations for a quantum dots molecule.There is a relationship between temporal and spatial correlations.In the time domain where the maximal LGI violations happen, the values of quantum coherence remain high.When no spatial correlation is detected,there is also no violation of the Leggett-Garg inequality.However, the system of interest can maintain spatial correlations at a high level even when the LGI is not violated.It is demonstrated that there is a distinction between temporal and spatial correlation due to different criteria. Fig.4 The evolution of the spatial and temporal correlation is shown when the parameters g=1 , Te=0.5g,∆=0 and ω21=0 are chosen.The red dashed line denotes the spatial quantum correlation evaluated by l1-norm quantum coherence.The black solid line describes the behavior of temporal correlation given by the LGI violation When there is spontaneous, decay and photon leakage, the evolution of the hybrid system is subjected to environmental noise.The Markovian dynamics of the total system can be described by the quantum master equation where the dissipators Dˆk[ρ(t)]= σˆkkρ-2 σˆ0kρ σˆk0+ρ σˆkkand Dˆa[ρ(t)]=aˆ+aˆρ-2aˆρaˆ++ρaˆ+aˆ.Here,σˆjk=|j〉〈k|denotes the transition operator from the|k〉 to |j〉 state.The parameters γ and κ stand for spontaneous decay from the quantum dots molecule and photon leakage of the cavity, correspondingly.According to the results[22], a stationarity condition holds given that a quantum system evolves under Markovian dynamics.With stationarity, the evolution of the system fromt0tot1is governed by the same stochastic differential equation as the evolution fromt1tot2.To numerically obtain the temporal correlation, one kind of Leggett-Garg-type inequality is defined asK3′=2C(t0,t1)-C(t0,t2)≤1 where the time interval is equal to τ.The evolution of the violation is numerically calculated and shown in Figure 5 (color online).It is seen that the violations gradually decrease with time before finally disappearing in Figure 5(a).The suppressed behavior of the violation is similar to the decoherence of spatial quantum correlation.The spontaneous decay from a quantum dots molecule can decline the maximal degree of the violation.Similarly, it is demonstrated that the tunneling coupling can also improve the temporal correlation under environmental noise. Fig.5 The effects of environmental noise on the temporal correlation are considered when the parameters are g=1 , Te=0.6g,∆=0 and ω21=0.(a) The decreased oscillation of the LGI violation is plotted when the spontaneous decayγ=0.02g and the cavity leakage κ=0.01g ; (b) The maximal LGI violations are plotted as a function of the tunneling coupling for the different decaying parameters of γ=0.02g, 0.01g which are represented by the red circles and black squares, respectively We have tested the violation of the Leggett-Garg inequalities to evaluate temporal quantum correlations in a quantum dots molecule coupled to a cavity.The coherent superposition between a tunneling excited state and other level states was studied by means of the LGI violation andl1-norm quantum coherence.When there is no spatial correlation with zero quantum coherence, no violation of the Leggett-Garg inequalities happens during the evolution.Moreover, the maximal violations exist in the time intervals where the system has high values of spatial correlations.However, there is also some discrimination between both kinds of quantum correlations.The quantum coherence can persist in time domains where there is no LGI violation.This fact shows that the LGI violation andl1-norm coherence are independent measure criteria of quantum correlation.We have taken into account the impacts of spontaneous decay from quantum dots and photon leakage from a cavity on the dynamics of temporary quantum correlations.And we have proved that these elements can result in the attenuation of the quantum coherence.The decoherence of the LGI violation can lead to macroscopic realism where coherent superposition of the level states will disappear.We have found that the evolution of the hybrid system is subjected to spontaneous decay and photon leakage.The parameters with respect to environmental noise accelerate the decline of temporal quantum correlation.We have demonstrated that LGI violation can be enhanced by electron tunneling interactions.With the development of quantum information processing technology, these results can be applied to hybrid multilevel systems.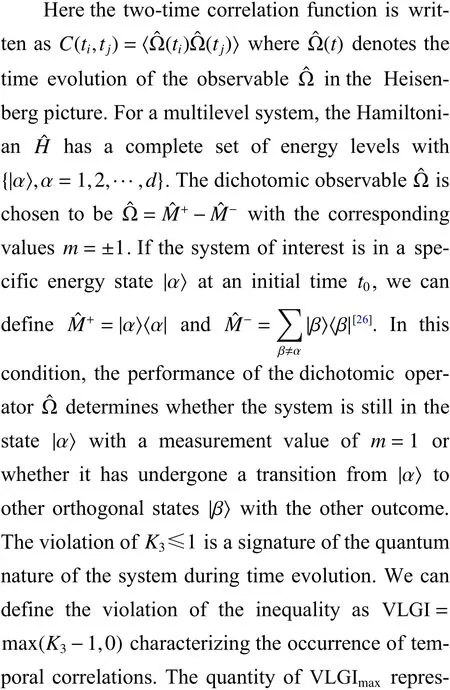
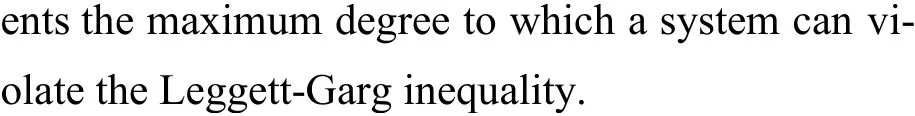
3 Model and Hamiltonian of a hybrid system

4 Dynamics of temporal and spatial correlation



5 Discussion and results
