Quantum carpets: efficiently probing fractional revivals in position-dependent mass systems
2023-02-16ToobaBibiSuniaJavedandShahidIqbal
Tooba Bibi,Sunia Javed and Shahid Iqbal
Department of Physics,School of Natural Sciences,National University of Sciences and Technology,H-12 Islamabad,Pakistan
Abstract Position-dependent-mass systems are of great importance in many physical situations,such as the transport of charge carriers in semiconductors with non-uniform composition and in the theory of many-body interactions in condensed matter.Here we investigate,numerically and analytically,the phenomenon of fractional revivals in such systems,which is a generic characteristic manifested by the wave-packet evolution in bounded Hamiltonian systems.Identifying the fractional revivals using specific probes is an important task in the theory of quantum measurement and sensing.We numerically simulate the temporal evolution of probability density and information entropy density,which manifest self-similarly recurring interference patterns,namely,quantum carpets.Our numerical results show that the quantum carpets not only serve as an effective probe for recognizing the fractional revivals of various order but they efficiently describe the effect of spatially-varying mass on the structure of fractional revivals,which is manifested as a symmetry breaking in their designs.
Keywords: wave-packet dynamics,quantum carpets,information carpets,quantum revivals,fractional revivals,position-dependent mass schroedinger equation
1.Introduction
The idea of position-dependent mass (PDM) can emerge in many physical situations,such as the effective theory of many-body interactions in the field of condensed-matter physics [1–4].Typically,a charge carrier in a crystal may carry effective mass,meff,significantly different from its corresponding free-state massm0.Moreover,in many practical situations,meffmay become anisotropic,which leads to spatially varying mass.Because of advancements in nanotechnology to fabricate ultra-smart semiconductor devices,the theory of PDM has become significantly important to study various physical properties of semiconductor structures[5–16].However,the quantum theory of PDM systems is challenging due to the non-uniqueness of the quantum Hamiltonian within the same many-body approximation and because of the mathematical complexity in finding the analytic solutions.Conventionally,the solutions of PDM systems are obtained by directly solving the underlying Schrödinger equation[17,18],however,various other procedures are also available in the literature,for instance,potential algebras using the notion of shape invariance[19–25],the path integral formalism [26] and by employing the point canonical transformations [27–30].Nonetheless,the possibility of finding analytical solutions of PDM systems has triggered numerous other important explorations,such as the construction of generalized coherent states [31–35] and the calculation of information entropy [36–42],which may be beneficial in quantum technologies.
In bounded systems,wave-packet dynamics manifest the phenomena of quantum revivals and fractional revivals as their inherent features,which have been well studied in various physical contexts [43–53] and [54–57],respectively.In particular,the phenomenon of fractional revivals has enormous significance in the theory of quantum measurement and quantum sensing,since they can retrieve information about the particle even if the corresponding wave packet collapses well before its first full quantum revival time [54].Moreover,the notion of fractional revivals can be useful in mapping the quantum phase of molecular wave-packet in the context of spectroscopy [55],in developing the scanning microscope [56] and even to factorize the prime numbers[57].However,identifying the wave-packet fractional revivals with better resolution using various physically observable quantities as measurement probes [46,51] is still an important task.A conventional approach to studying fractional revivals is to analyze the autocorrelation function[46],which measures the overlap of the time-evolved wave packet onto the initial wave packet.Nonetheless,some other measures,such as the time-evolution of probability density [58]and information entropy[51,59]have also been introduced in this context.In this letter,we are aimed to explore the phenomenon of wave-packet fractional revivals in PDM systems,which has not been much discussed,except in some recent studies [30,53,59,60].However,it has been shown recently [59,60],that the effect of PDM on the structure of fractional revivals cannot be fully captured by usual probes,for instance,by the autocorrelation function.However,in this context,it has been shown [59] that the information entropy does have a better sensitivity to elucidate the effect of spatially-varying mass.
In this work,we investigate the wave-packet fractional revivals in PDM systems by numerically simulating the temporal evolution of probability and Shannon information entropy densities,both in the position as well as momentum space,which manifests the recurrence of self-similar interference patterns,termed as the quantum carpets.The fractional revivals of the wave packet are then identified by the recurrence order of self-similar structures in the design of such quantum carpets.The concept of quantum carpets has been used in numerous contexts,such as,to explore wave-packet quantum recurrences [58],for studying decoherence effects[61],to explain the Talbot effect[62]and to investigate various probability density profiles [63–65].Here,in addition to conventional position-time (spatio-temporal) quantum carpets,we extend this notion to momentum-time(momento-temporal)and entropy-time(information)quantum carpets.It has been shown by our numerical results that the quantum carpets not only serve as an effective probe for identifying the fractional revivals of various orders but they efficiently elucidate the effect of spatially-varying mass on the underlying phenomenon,which is manifested as symmetry breaking in their designs.Moreover,it has been observed that,under the influence of the PDM,the fractional revivals are shifted in position-space towards the region where the particle experiences a higher mass.
The rest of the paper is organized as follows.In section 2 we present the quantization procedure for PDM systems and then present the analytic solutions of the Schrödinger equation corresponding to our model PDM system.Then in section 3 we discuss the analytic formalism regarding probability and information entropy densities required to numerically simulate the respective quantum carpets.We present,in section 4 the numerical results and discussions regarding fractional revivals of the PDM wave packet.Finally,in section 5 we present the conclusion of our work.
2.Schrödinger equation with PDM
While quantizing the PDM Hamiltonian,H=p2/2m(x)+V(x),the operators concerningpandm(x) in the kinetic energy term encounter an ambiguity with respect to their mutual ordering.In this context,a general procedure was proposed by von Roos[10,11],and the corresponding kinetic energy operator is given as
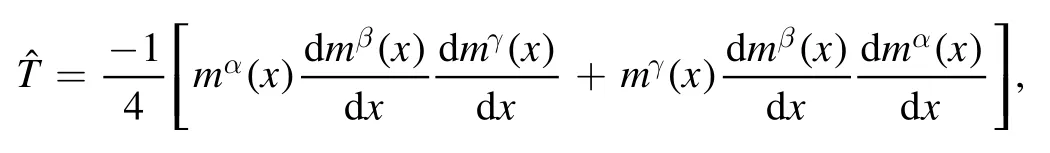
where α,β,γ are so-called ambiguity parameters satisfying the constraint α+β+β=−1.It is to be noted that by choosing various sets of values for α,β and γ,one finds different non-equivalent quantum Hamiltonians [12].Nonetheless,the special choice,α=γ=0 and β=−1,results in a symmetric ordering of the underlying operators [12].In this case,the corresponding Schrödinger equation becomes[17,18] as
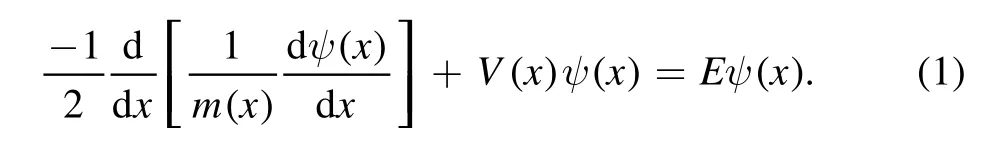
The particular solutions of this equation depend on the choice of the profile of PDMm(x) and on the type of confining potentialV(x).Various authors have attempted to obtain the exact solutions of this equation for various choices ofm(x)andV(x) [17,18].Here we consider a particle with spatiallyvarying mass
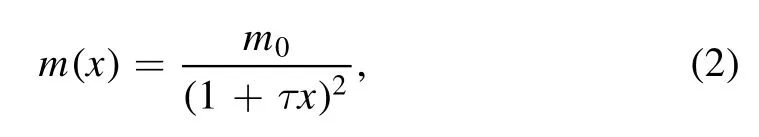
trapped inside a one-dimensional infinitely deep square well of lengthL,defined asV(xˆ)=0 for 0

the Schrödinger equation,given in(1),reduces into a simpler differential equation as
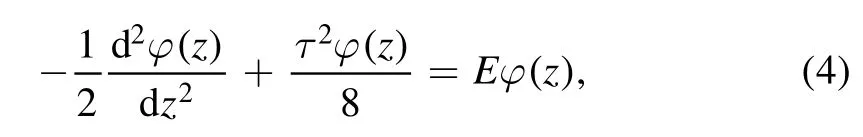
where,z=ln (1+τx),as is evident from equation (3).It is straightforward to find the solutions to equation (4),by applying the boundary conditions,ψ(0)=ψ(L)=0.As a result,we find the normalized eigenfunctions as
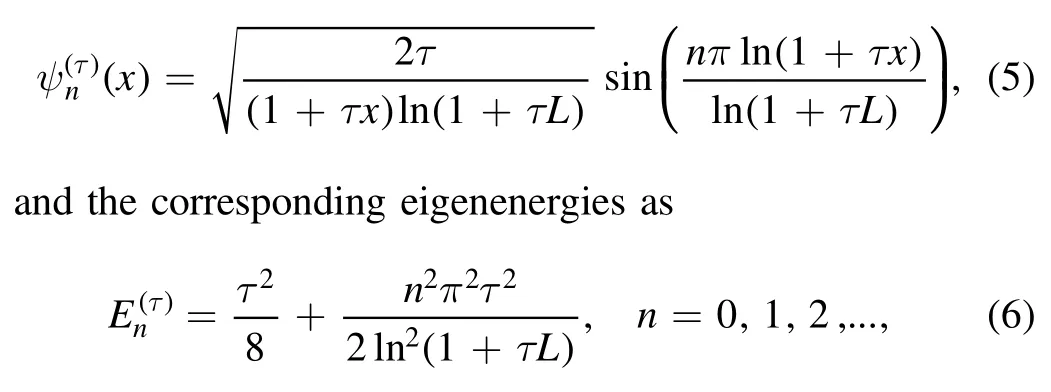
where the ground-state energy takes the value asE0=τ2/8.However,it is to be noted from equation (5) that the eigenfunctions exist only for(i.e.ground-state eigenfunction,,vanishes and constitutesthe so-calledzero-curvature solution[66]).
The eigenfunctions and eigenenergies,given in equations (5) and (6) respectively,enable us to construct the wave-packet for the PDM system and to study the wavepacket time-evolution through probability and information entropy densities both analytically and numerically.
3.Quantum carpets woven by PDM: identifying fractional revivals
As mentioned earlier,the quantum carpets are woven by selfinterference of the probability density of a wave packet during its temporal evolution in a given bounded system.Consequently,the self-similarly recurring interference pattern can serve as a probe to identify the structure of fractional revivals.Mostly,position-time(spatio-temporal)quantum carpets have been discussed in the literature [58,61,63–65],which are woven by the temporal evolution of position-space probability density.In addition,analogously,we also present the momentum-time and the entropy-time quantum carpets in the following subsections.However,the solutions of PDM Schrödinger equation (1),obtained in the previous section 2 provide us the starting point to obtain the analytical expressions for probability and entropy densities in position and momentum spaces for our chosen PDM system.
3.1.Position-time quantum carpets
The time-dependent wave packet for the given PDM system can be expressed as a linear superposition of the corresponding eigenfunctions
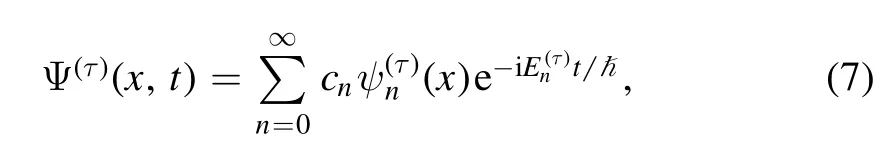

where σ is the spread of the wave packet at FWHM.Using equation (7),the time-dependent probability density in position space is calculated as
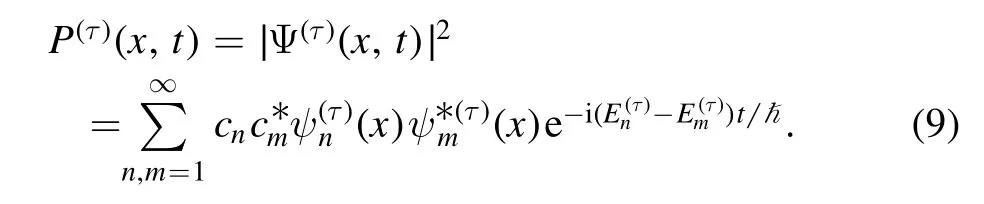
During its temporal dynamics,the wave packet undergoes a series of constructive and destructive interference,which leads to manifesting revivals at various time scales.The natural time scales of these recurrences are defined [46] as
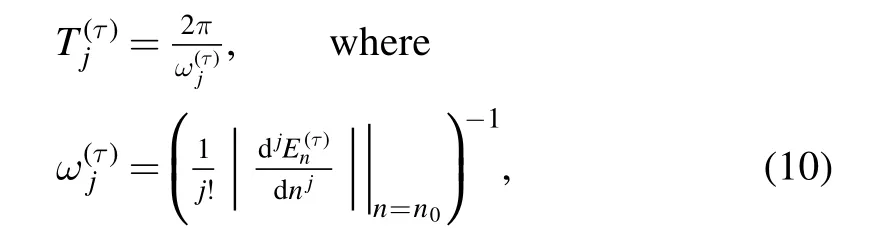
Using equation (6),the classical period and quantum revival time,for PDM particles trapped in an infinite square well,take the values as
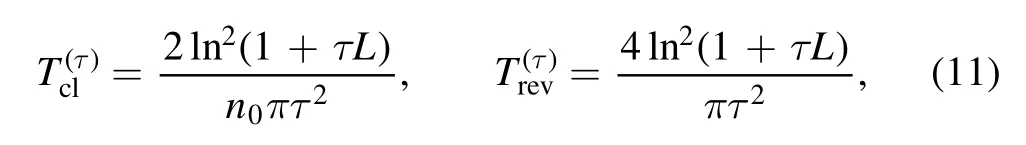
respectively.To recognize the effect of PDM on the structure of quantum recurrence,let us consider the corresponding constant mass system having energyIn this case,thetake the values as
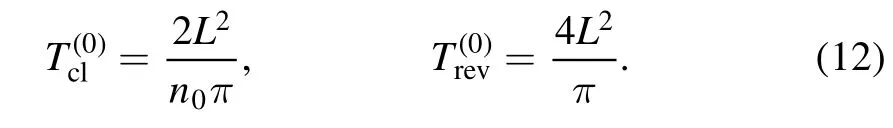
It can be seen from equations (11) and (12),that the ratio,,for both constant mass (CM)and PDM systems are the same.This fact implies that the effect of PDM on classical periodicity and quantum revival time is nothing more than a constant scaling ofwith same magnitude.
However,in addition to full revivals,the wave-packet revivals occur partially at times,whererandsare relative prime numbers.The numerical simulation of probability density,P(τ)(x,t),given in equation (9),as a function of timetand positionxleads to generating the position-time quantum carpets,which exhibit the structure of fractional revivals as displayed in figures 1–4.
3.2.Momentum-time quantum carpets
In order to obtain an analogous description of quantum carpets in momentum space,we first need to transform the eigenstates of the system into momentum space.This can bedone using the Fourier transform ofas
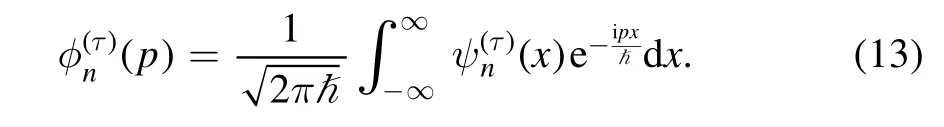
It is worth noting that the Fourier Integral(13)vanishes outside the interval[0,L]because of the boundary conditions chosen to solve the PDM Schrödinger equation in equation (4).
Using equation (13),the time-dependent wave packet in momentum space is given as
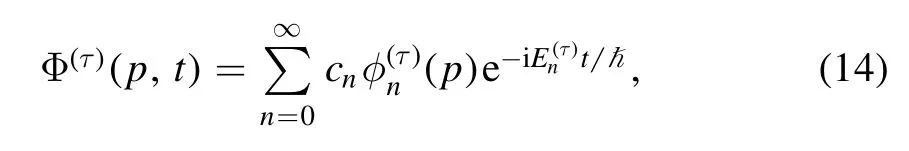
and the corresponding time-dependent probability density in momentum space is now given as
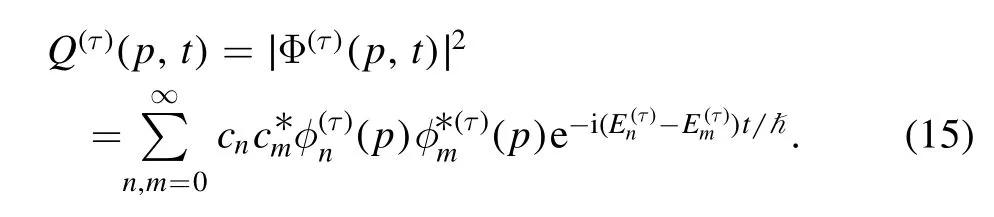
For the momentum-time (momento-temporal) quantum carpets,we numerically compute,Q(τ)(p,t),as a function ofpandtand the corresponding results are displayed in figures 5 and 6.
3.3.Entropy-time quantum carpets: quantum carpets of information
Having defined the probability density of the wave packet in position and momentum space,as given in equations (9) and(15),it is instructive to calculate the corresponding information entropies.For instance,the position-space Shannon information entropy is defined [59,67] as
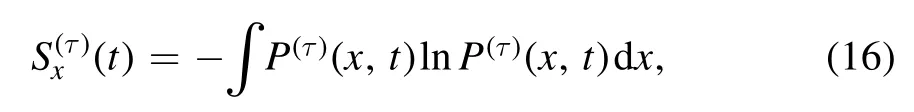
whereP(τ)(x,t) is given above in equation (9).Analogously,using equation (15),the corresponding momentum space information entropy is defined as

It is worth mentioning that the information entropyquantifies the amount of uncertainty associated with the localization of the wave packet in a given space ζ (where ζ denotes eitherxorpspace).For instance,the smaller is the information entropy,and sharply localized is the wave packet.This further implies that the lower the uncertainty,the greater the accuracy) in predicting the particle’s localization.Hence,the temporal evolution of the information entropy,may have the potential to encode the information of the wavepacket fractional revivals.Moreover,it is found that the sum,satisfies the relation [59,67]
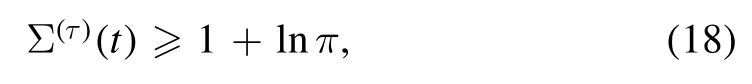
which is an entropy-based version of the Heisenberg uncertainty relation,analogous to the usual variance-based one ΔxΔp≥ħ/2.However,it is found that,in addition tothe sum Σ(τ)(t) can also be used to identify the structure of fractional revivals.In our recent work [59],we have studied the fractional revivals for PDM tapped in an infinite square well[59]using information theoretic measures defined above in equations (16),(17) and (18).
In the present context of quantum carpets,let us define the entropy density as a function of position and time,
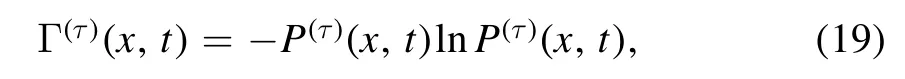
which is essentially the integrand of the integral(16)defining the position-space information entropy.Analogously,using equation(17),the entropy density in momentum space can be defined as
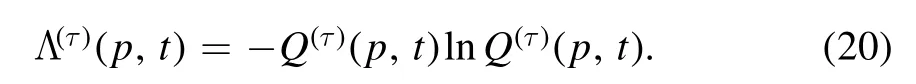
It is important to note that these entropy densities may have the sensitivity to detect the local effect of PDM on the structure of fractional revivals.By numerically simulating information entropy densities Γ(τ)(x,t) and Λ(τ)(p,t),given above in equations (19) and (20),we can generate the information entropy-time quantum carpets which we referred to as information quantum carpets or simply,information carpets.The snapshots of these entropy densities,exhibiting the fractional revivals,are displayed in figure 7.
4.Numerical results and discussions
In order to characterize the phenomenon of fractional revivals using quantum carpets as probes for their identification,we numerically compute the probability and entropy densitiesdefined above in equations (9),(15),(19) and (20).As mentioned earlier in previous section 3,for our numerical simulations,we consider an initial Gaussian wave packet,defined in equation(8),having width σ=0.1 and centered at the middle of the wellx0=L/2.Moreover,for convenience of our numerical computation,we choose the arbitrary units for which ħ=m0=L=1.To better understand the structure of quantum carpets in a given space,we first take the snapshots of corresponding probability and information entropy density after different evolution times,specifically,chosen at fractional revival time of various orders,defined aswithrandsare prime numbers.
For instance,we numerically compute the position-space probability density,P(τ)(x,t),defined by equation (9),as a function of positionxafter different fractional revivals and for various values of PDM strength parameter τ.The corresponding results are displayed in figures 1 and 2.In particular,the plots in figure 1 show that at any chosen fractional revival time,the probability density peaks shift toward the left half of the well where PDM experiences a higher mass.Whereas,figure 2 shows a comparison of the main fractional revivals of the PDM particle with the ones of the corresponding constant mass (CM) particle.It can be seen from these plots that the symmetric probability density peaks,in the case of fractional revivals of CM particle (τ=0),get shifted towards the left region when the spatial dependence of the mass is turned on (τ>0).
Figure 3 shows the generation of position-time quantum carpet,where the probability density function,P(τ)(x,t),has been plotted as a function ofxandtfor short-term temporal evolutionThe strength of probability density has been represented by means of colors,such that,brightness(darkness) indicates the high (low) probability density,as shown by the color scaling in figure 3.It can be seen from these plots that an initially well-localized (Gaussian) wave packet,centered at the middle of the box,starts its time evolution tending to follow a classical trajectory between two boundaries.However,after a few classical periods dephasing among various constituents eigenstates of the wave-packet dominates and as a result wave packet experiences a collapse.Later on,fractional or full revivals of the wave packet occur when the phase-matching condition is regained,respectively,either partially or completely.Moreover,figure 4 shows a comparison of the position-time quantum carpet of the CM particle with that of the PDM particle.It is clearly seen from these plots that the symmetry of quantum carpets gets broken,such that,the probability of finding the particle increases in the region where it experiences a higher mass.
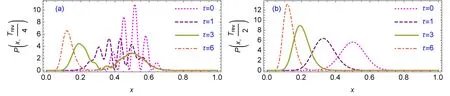
Figure 1.The position-space probability density,P(τ)(x,t),as defined in equation(9),is plotted for different values of PDM strength parameter τ,after evolution time:(a)t =4;(b)t =2.These plots show the effect of spatial dependence(defined by strength parameter τ)on a given fractional revival.Initially,the Gaussian wave packet has width σ=0.1 which is centered at the middle of the well x0=L/2.
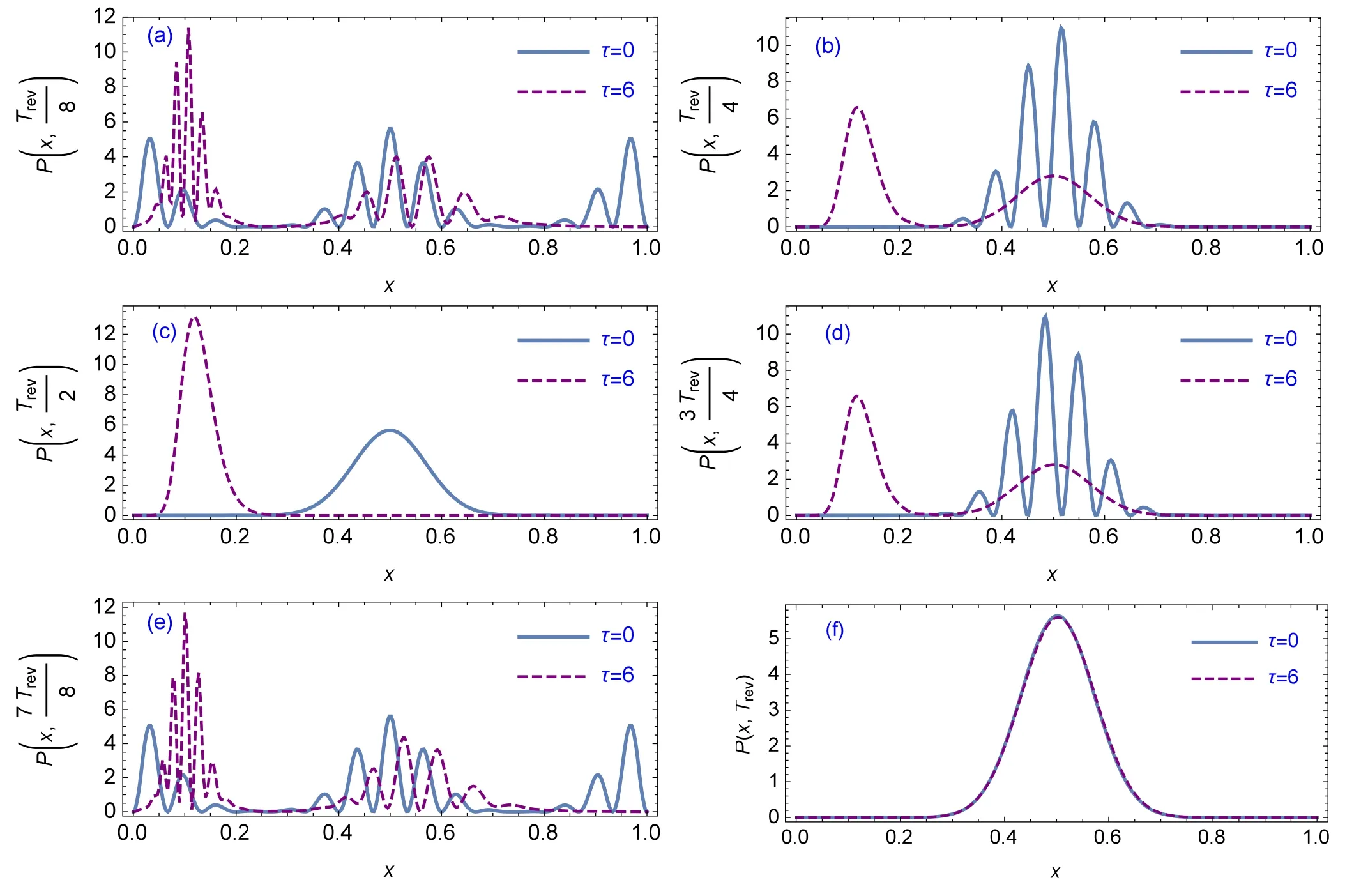
Figure 2.The snapshots of position-space probability density,P(τ)(x,t),as defined in equation(9),are plotted after different evolution time: These plots show a comparison of fractional revivals of the PDM system(τ>0)with those of the corresponding constant mass system(τ=0).The values of characteristic parameters of initial Gaussian are chosen the same as in figure 1.
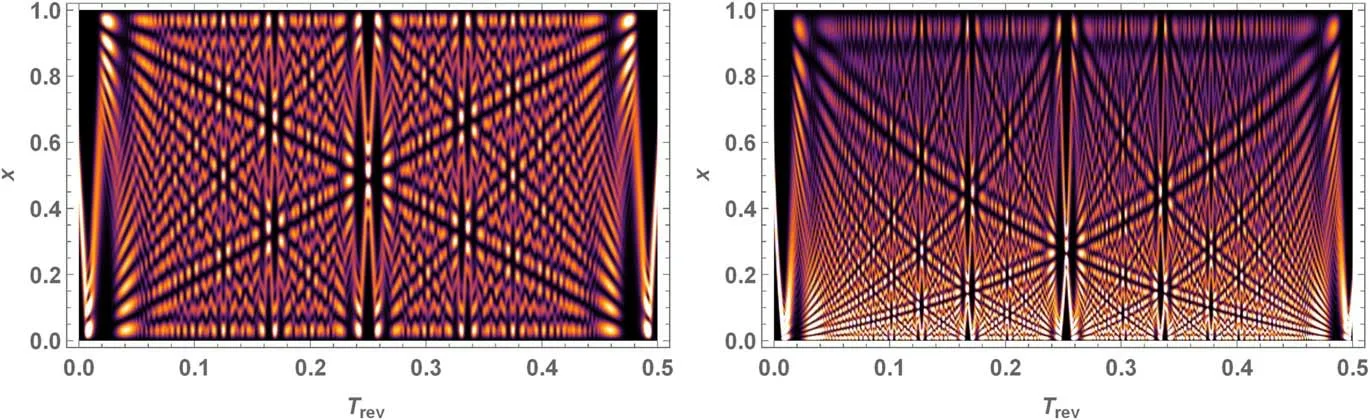
Figure 4.The position-time quantum carpets woven by position-space probability density,P(τ)(x,t),of the wave-packet during its long-time evolution(Δt =2):(a) CM particle;(b) PDM particle.The initial profile of the wave packet is the same as in figure 1.
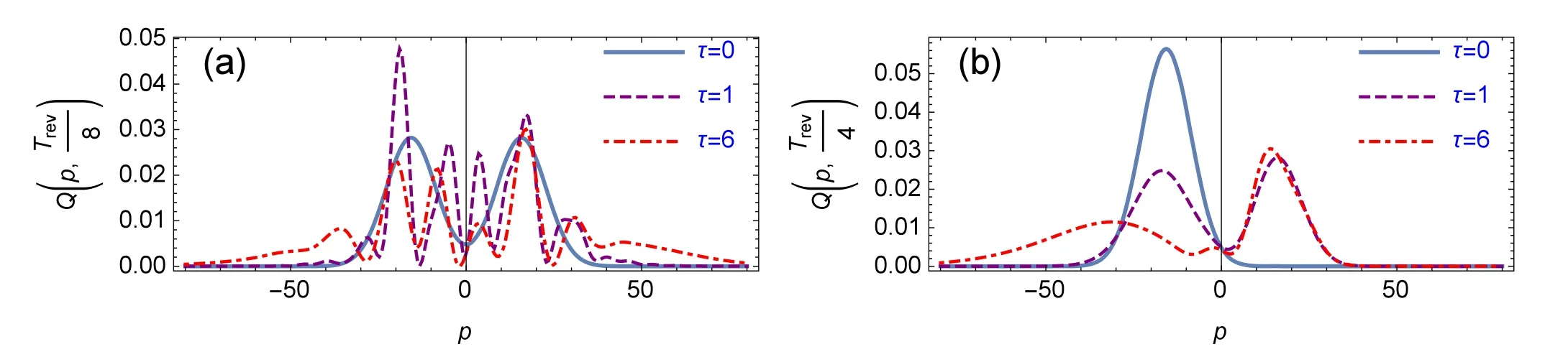
Figure 5.The momentum-space probability density, Q(τ)(p, t),as defined in equation (15),is plotted for different values of PDM strength parameter τ,after evolution time: (a) t=Trev/8;(b) t=Trev/4.These plots are aimed to show the effect of PDM on fractional revivals in momentum space.The initial profile of the wave packet is kept the same as in figure 1.
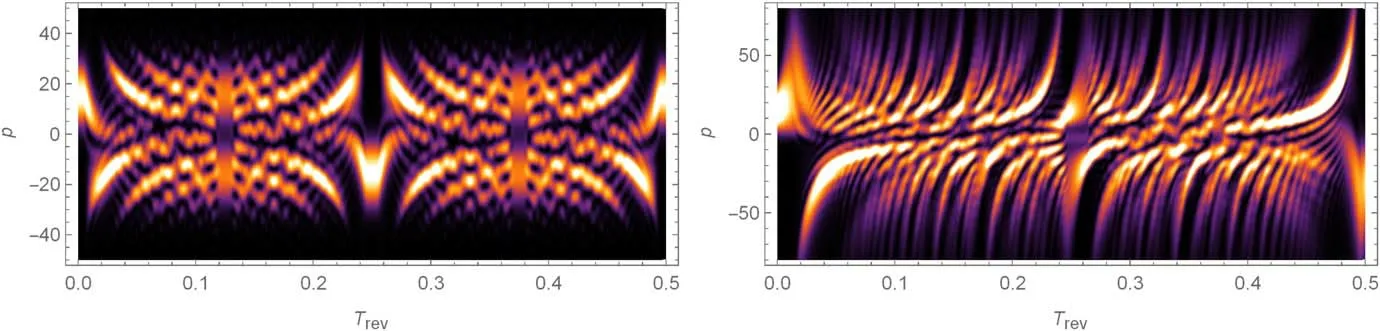
Figure 6.The momentum-time quantum carpets woven by momentum-space probability density, Q(τ)(p, t),during its long-time evolution Δ t=2: (a) CM particle;(b) PDM particle.The initial profile of wave-packet is the same as in figure 1.
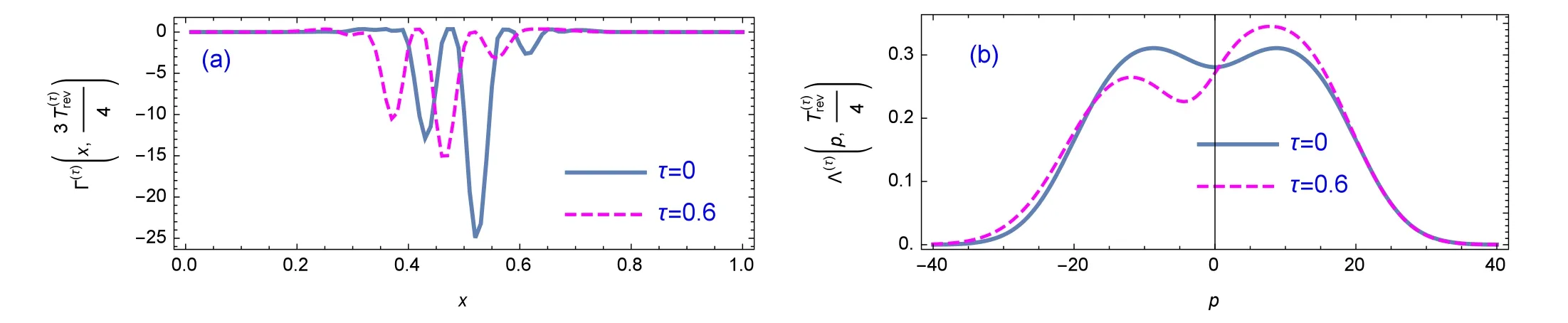
Figure 7.The information entropy densities,defined in equations(19)and(20),are plotted for different values of PDM strength parameter τ:(a)position-space entropy density after evolution time t=3Trev/4;(b)momentum-space entropy density after evolution time t=Trev/4.The initial profile of the wave packet is kept the same as in figure 1.
Similarly,to visualize the analogous description of fractional revivals in momentum-space,we numerically compute the momentum-space probability density,Q(τ)(p,t),as functionpandt,for different values of strength parameter τ.The results regarding the snapshots ofQ(τ)(p,t)at different fractional revivals have been displayed in figure 5 whereas,the momentum-time quantum carpet for an evolution time intervalis shown in figure 6.From these plots,it is evident that probability peaks become asymmetric as soon as the mass of the particle becomes position-dependent(τ>0).Likewise,we numerically simulate information entropy densities in position and momentum spaces,given in equations (19) and (20),and the corresponding results are displayed in figure 7.A detailed analysis of fractional revivals using information entropy densities has recently been presented [59].
From the above analysis,it can be seen from the positiontime quantum carpets that under the influence of PDM,the fractional revivals get shifted in position-space towards the region where the particle experiences a higher mass.However,in any given space(whether position or momentum),the symmetry of the quantum carpets’designs is broken when the position-dependence of the mass is turned on (τ>0).It is therefore concluded that quantum carpets,in any given space,not only serve as an effective probe for recognizing the fractional revivals but they efficiently elucidate the effect of spatially-varying mass on the underlying phenomenon,as indicated by the symmetry breaking in their designs.
5.Summary and conclusions
In the present work,we have investigated the phenomenon of fractional revivals in the varying-mass systems with respect to position.The PDM systems are of great significance in many physical situations of practical interest,such as the transport of charge carriers in semiconductors of non-uniform chemical composition and in the theory of many-body interactions in condensed matter physics.However,quantization of such systems is a challenging task because of the non-uniqueness of quantum Hamiltonian within the same many-body approximation and due to the mathematical complexity in finding the analytic solutions.In our work,we first presented a general procedure for the quantization of a PDM Hamiltonian by symmetrically ordering the operators concerning momentum and PDM.For a specific choice of PDM,we then solved the PDM Schrödinger equation for a special case of a PDM particle trapped in a one-dimensional infinitely deep square well.Such toy models have been very useful in many practical situations,for instance,in the fabrication of semiconductor structures,and are important to understand numerous fundamental notions of quantum mechanics [68].
Then we investigated the characteristic features of wavepacket dynamics in our chosen PDM system.Generally,detecting the fractional revivals in a given system is an important task in the theory of quantum measurement and sensing,which requires the use of some physically observable quantity such as a probe to analyze the underlying phenomena.In our work,we have computed,analytically and numerically,the temporal evolution of probability and information entropy densities in position and momentum spaces which lead to the formation of self-similarly recurring interference patterns known as quantum carpets.The fractional revivals of the wave packet have been identified by the recurrence order of self-similar patterns in the design of these quantum carpets.For our analysis,in addition to conventional position-time (spatio-temporal),we have simulated the momentum-time and entropy-time quantum carpets,which we would like to be namedinformation carpets.It has been shown by our numerical results that the quantum carpets not only serve as an effective probe to identify the fractional revivals but they efficiently describe the effect of spatiallyvarying mass on the structure of fractional revivals,which is manifested as symmetry breaking in their designs.Moreover,it has been observed that,under the influence of spatiallyvarying mass,the fractional revivals are shifted in positionspace towards the region where the particle experiences a higher mass.
Acknowledgments
Financial support from Higher Education Commission(HEC)of Pakistan,under Grant No.20-14808/NRPU/R&D/HEC/2021 2021,is gratefully acknowledged.
杂志排行
Communications in Theoretical Physics的其它文章
- Modification of laser-induced state in atomic attosecond transient absorption by the XUV pulse pair
- Thermoelectric transport in holographic quantum matter under shear strain
- High-sensitive refractive index sensing and excellent slow light based on tunable triple plasmon-induced transparency in monolayer graphene based metamaterial
- Optical forces on neutral atoms in the presence of fluctuating laser fields:numerical analysis
- Thermal Hall conductivity with sign change in the Heisenberg–Kitaev kagome magnet
- A numerical method to calculate dwell time for electron in semiconductor nanostructure
