Optical forces on neutral atoms in the presence of fluctuating laser fields:numerical analysis

Forough Bozorgzadeh,Masoud Yousefiand Mohsen Akbari

1 Physics Department,College of Sciences,Shiraz University,Shiraz,Iran

2 Quantum Optics Group,Iranian Center for Quantum Technologies (ICQT’s),Tehran,Iran

3 Quantum Optics Lab,Department of Physics,Kharazmi University,Tehran,Iran

Abstract Doppler cooling of 88Sr atoms is studied in the presence of off-resonant red-detuned fluctuating laser fields.Using a semi-classical approach,we show that the relevant physical quantities in the cooling process,such as optical forces,the damping coefficient,Doppler temperature,and atom number in the trap,are strongly affected by the laser amplitude and phase fluctuations.We find that the Doppler cooling limit is higher than the predicted Doppler theory for non-fluctuating lasers.This implies an additional heating mechanism exists due to the laser fluctuations.Furthermore,our numerical analysis shows that the effect of laser power stability on reducing the number of trapped atoms in a magneto-optical trap is more substantial than the effect of laser linewidth.

Keywords: force of light,laser cooling and trapping,laser fluctuations,magneto-optical trap(MOT),phase diffusion,two-level atom

1.Introductions

Over the last decade,optical forces have attracted extensive attention in theoretical and experimental studies [1–3].These forces arise in the light–matter interaction due to the momentum exchange between the atoms and the laser field.When an atom absorbs the laser light,it is excited from the ground electronic state to some upper state.The subsequent return to the ground state could be either via spontaneous or stimulated emission.Each process leads to a unique optical force that is entirely different in nature.In this respect,controlling the atom degrees of freedom using laser light fields allowed the accurate analysis of the atom’s inner structure and the atomic collisions [4].Especially,the cold-trapped atoms are well-suited for an extensive range of applications such as atom localisation[5,6],atomic clocks[7–9],quantum sensors[10,11],and quantum gates [12].Nowadays,the alkaline-Earth atoms(such as Mg,Ca,and Sr)are extensively used in laser cooling experiments due to their lack of hyperfine structure for the most abundant bosonic isotopes [13,14].Indeed,this provides narrow electronic transitions which can be well-approximated to actual two-level systems [15,16].Unlike alkali atoms,which can reach very low temperatures with Sisyphus and polarization gradient cooling,temperatures above the predicted theoretical values are reported in alkaline-Earth atoms [16,17].This indicates that some extra-heating mechanisms exist,e.g.in the form of standing wave effects,re-scattering of fluorescence photons at zero,or positive frequency detuning,that are not described by the standard theory of Doppler cooling.

One attempt to move forward was made by Piiloet al[17],which consists of describing the radiative collisional heating quantitatively in a magneto-optical trap (MOT).It is found that the Doppler limit is not affected by the radiative heating in alkaline-Earth-metal atoms at realistic densities.Afterward,it is suggested that the transverse spatial intensity fluctuations of the optical molasses (OMs) are one possible extra-heating mechanism[18].However,this study is limited to intensity fluctuation and does not include the phase fluctuations of the applied laser field.The effect of field fluctuations on heating mechanisms led to the development of numerous theoretical approaches.The number and density of trapped atoms in a vapour-cell Zeeman trap are investigated experimentally and theoretically,and it is shown that this quantity is affected by the laser beam sizes,laser intensities,laser detunings,and magnetic-field gradients [19].The interaction of neutral atoms with the radiation force in OMs is studied using a semi-classical method of the laser-cooling concept [20].Particularly,since the magnitude of the repulsive force depends on the laser intensity,the power of rescattered light limits the number of trapped atoms.Another noteworthy scheme includes studying the optical forces on a two-level atom near an ultrathin optical fibre.In this case,fibre-induced van der Waals potential force imparts in the total force in addition to the spontaneous-emission recoil force and laser field force [21].The phase diffusion process for a pulsed semiconductor laser is comprehensively studied due to its performance as a quantum random number generator [22].The optical force analysis in the Rayleigh scattering regime is performed,and the numerical results are compared with the generalized Lorenz Mie theory [23].Campbellet al[24] utilized the cooling process from photon spin to damp torque on linear small molecules to the Doppler limit of≈500μK.Nowadays,individually controlled neutral atoms,especially with Rydberg states[25],have developed as a promising platform for quantum simulations.

In this work,we investigate the radiation forces and Doppler cooling limit in the presence of laser fluctuations.Using a two-level model,the numerical analysis is performed with realistic data for the most abundant even isotope of strontium (88Sr).First,an OMs is realized with two counterpropagating laser beams,and it is shown that higher values of the Doppler temperature limit are achievable considering both amplitude and phase fluctuations in the laser field.Also,including the laser linewidth leads to an increase in the capture velocity ranges of OMs.Then,to investigate the effect of laser fluctuations on the velocity capture range and trap potential,the analysis is extended to the MOT.Finally,the number of trapped atoms in an MOT is studied under separate amplitude and phase fluctuations,and it is found that the effect of the amplitude fluctuations is almost two times stronger than the one for phase fluctuations.

This paper is organized as follows: section 2 briefly describes the theory of optical forces arising from fluctuating laser fields.The relations for the damping force and cooling limit in OMs are obtained and then generalized to MOTs.The Numerical results and their discussion are given in section 3.Finally,section 4 concludes the paper.

2.Theory

2.1.Optical Bloch equations in steady-state

In the light–matter interaction,the light fields can alter the atoms’ trajectory by applying two kinds of optical forces.These optical forces include dipole (or gradient) and radiation-pressure(or dissipative)forces.The former force resulted from cycles of absorption and stimulated emission and is proportional to the gradient of the light intensity [26],while the latter manifests due to the cycles of absorption and spontaneous emission.In this section,we obtain an expression for these two forces in the presence of a fluctuating laser field.

When a laser field of frequencyωresonantly couples the ground∣g〉 to the excited∣e〉 level of a two-level atom,the light forceF can be determined via the changes in momentum:

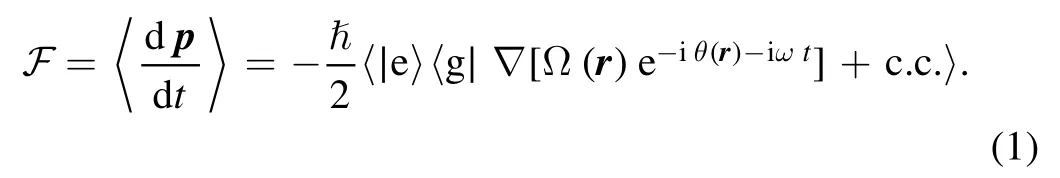
Here Ω(r)=μ·E(r)/ħis the Rabi-frequency,whichμis the electric dipole moment for the transition∣g〉 ↔ ∣e〉,andE(r)is the cycling field vector of frequencyωand phaseθ(r).Note that the expectation value is on both the internal degrees of freedom of the atom and on the center-of-mass state of the atom [27].An illustration of the energy diagram for the twolevel atom,along with the optical forces,is shown in figure 1(a).

In the weak field regime where the laser light does not dress the atomic level,for broad atomic transitions when the characteristic time for the internal dynamics is vastly different from the center-of-mass dynamics,equation (1) can be factorized and rewritten as follows

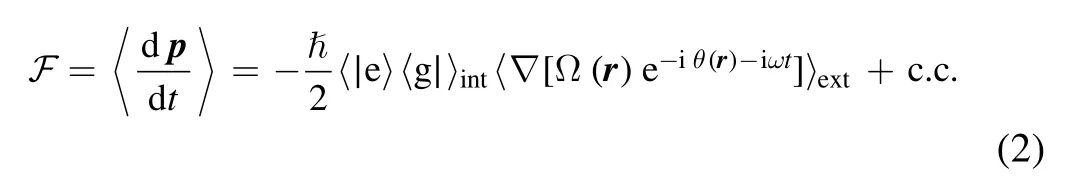
The above relation is validated under the following approximations:

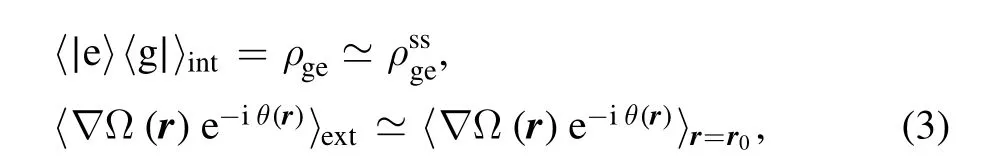
whereρgeis the so-calledelectronic coherenceterm.The first approximation assumes that the internal state reaches a steady state before the recoil timeτr=(ωrec)-1=(ħk2/2m)-1.According to theray atom opticslimit,the center-of-mass wave function of the atom can be approximated by aδ-function cantered atr=r0.Therefore,the second approximation can be applied to the well-localized particles.In this case,the light force can be expressed as

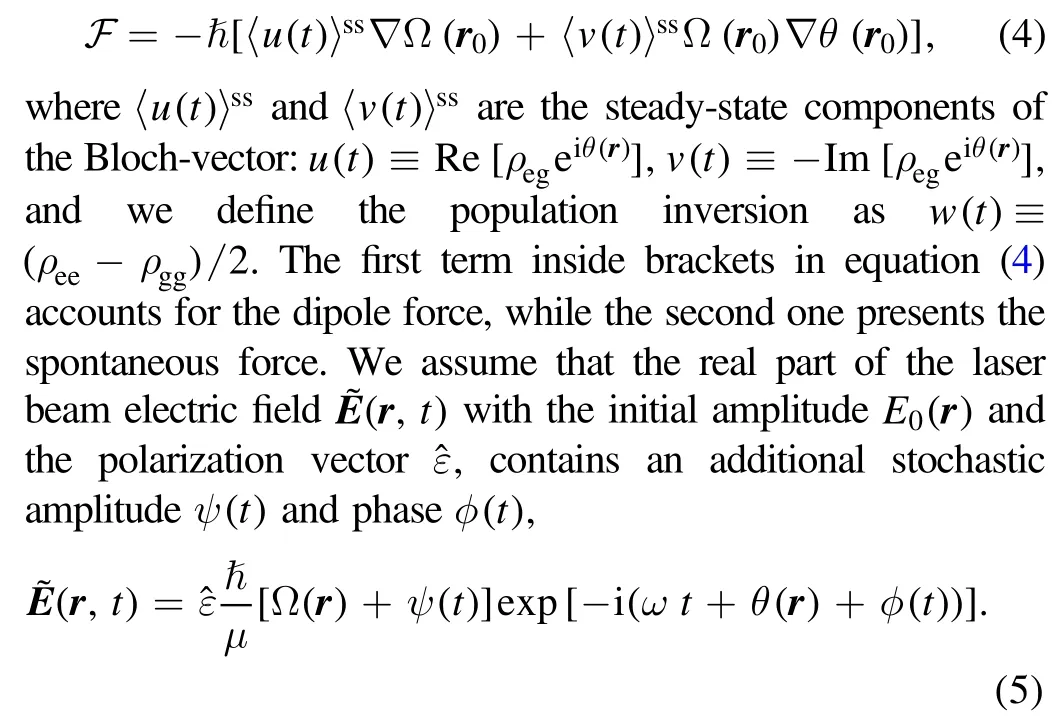
Adding a dissipative contribution and the associated fluctuations to the atomic evolution plays a central role in laser cooling.The characteristic time for the internal dynamics is of the order of the spontaneous lifetime of the transition,while that for the center-of-mass dynamics is the inverse recoil frequency,

The optical Bloch equations take the following form:

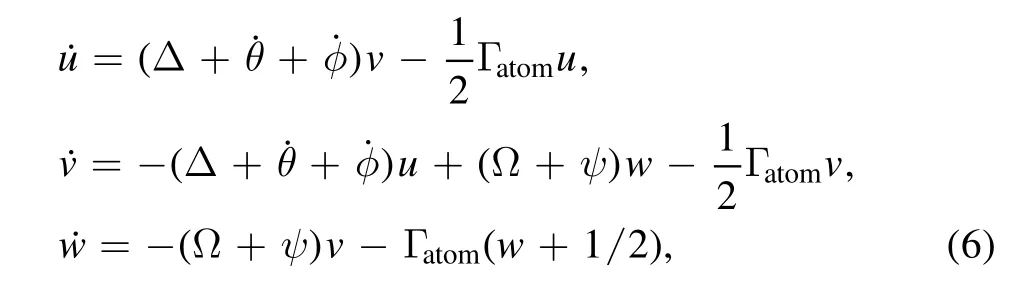
where Γatomis the spontaneous emission rate,and Δ=ω-ω0is the frequency detuning.The limitψ(t)=φ(t)=0 represents the case with no random fluctuations.The phase diffusion model describes the laser fluctuations: the stochastic phase and amplitude and their time derivatives are invariant with the changes in atomic position.Furthermore,the time derivatives areδ−correlated in time[28,29],i.e.

where 2Γamp(related to laser power stability) and 2Γphs(related to laser linewidth) are usually defined as the spectral densities of the stochastic process.

The direct relationship between amplitude fluctuation and spectral width leads us to express intensity fluctuation in terms of spectral width.The phase fluctuations show its spectral width in the spectral density function of the laser source.The spectrum of the radiation is

Bloch equations with stochastic fields lead to the multiplicative stochastic processes [30,31],in which the exact solutions can be obtained for the ensemble-averaged Bloch vector(〈u〉,〈v〉,〈w〉).Accordingly,equation (6) changes as follows

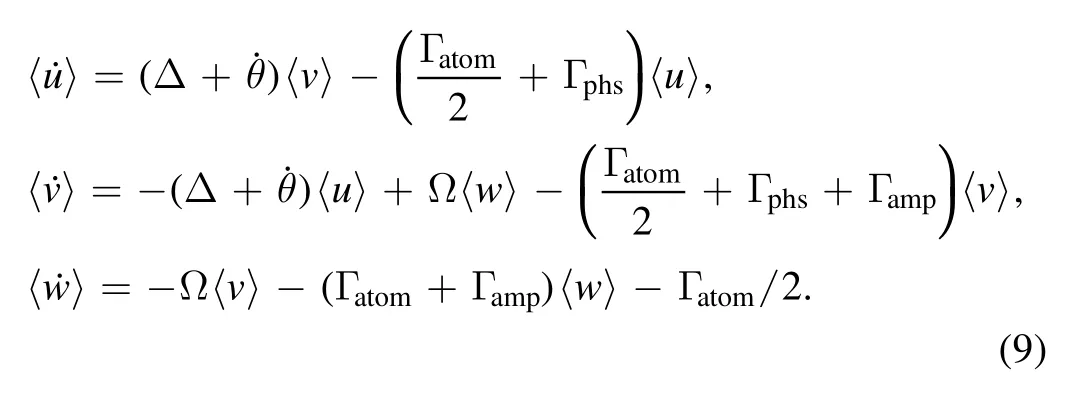
These equations show that bothuandvcomponents relax quickly in the presence of laser fluctuations,and the population inversion is only affected by the amplitude fluctuation.The steady-state values of the components are given by:

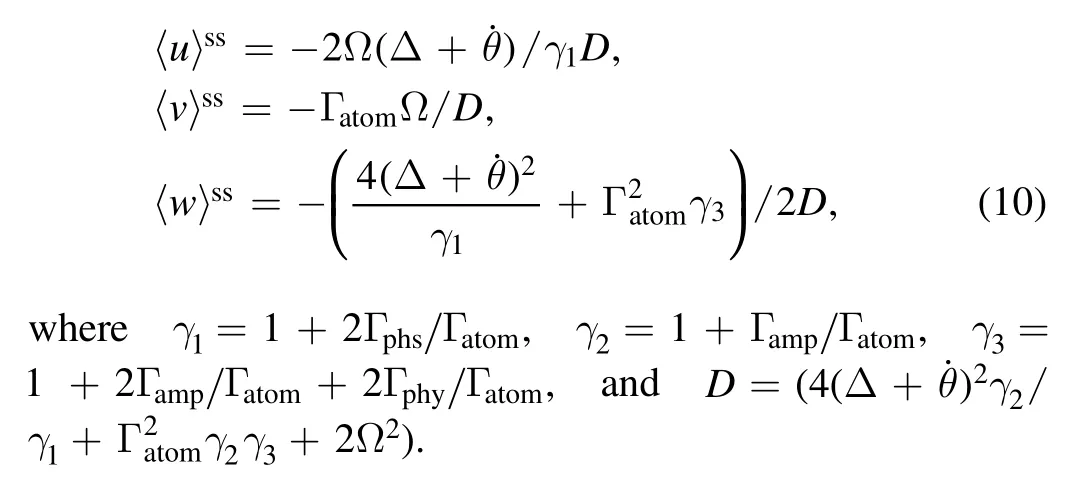
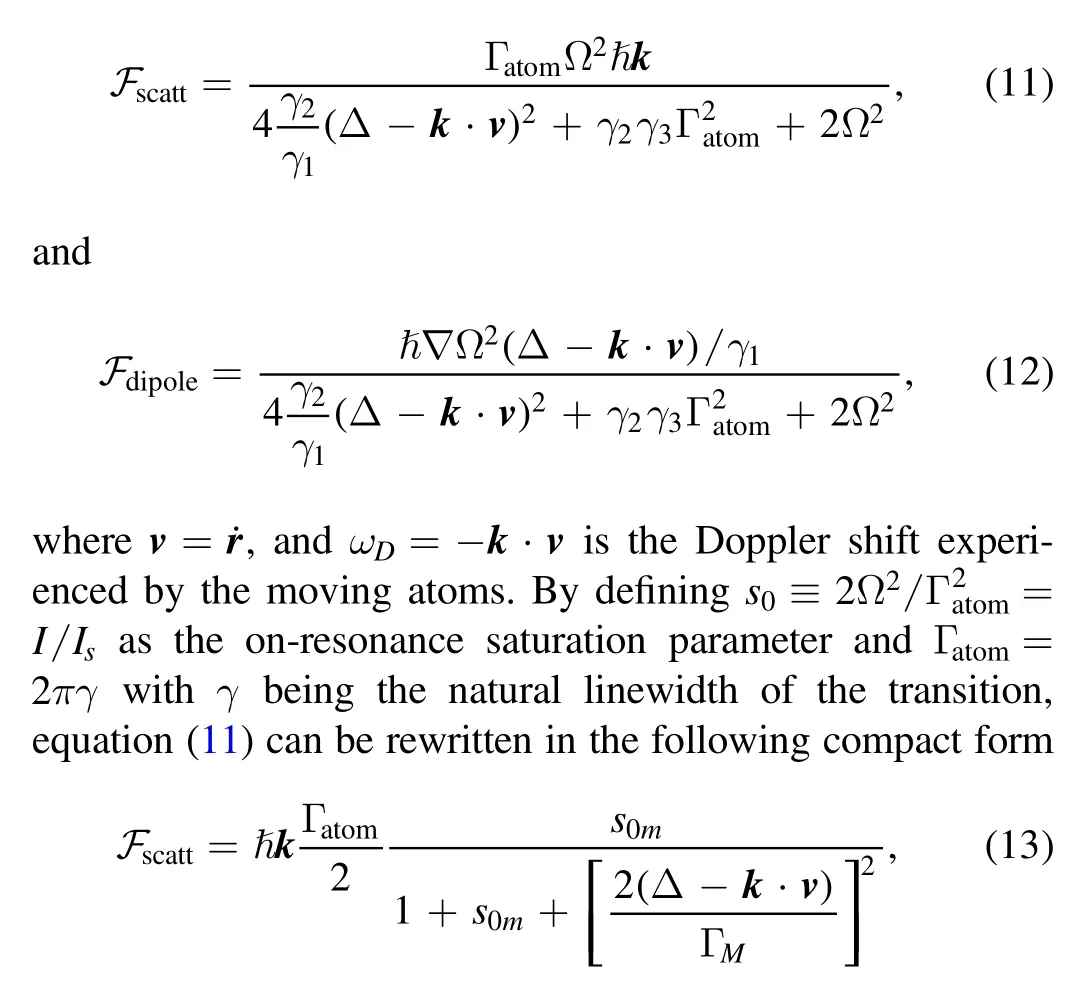
In the presence of laser fluctuations,the ensemble-averaged radiation-pressure (or scattering) force and dipole force for plane traveling wave are respectively

2.2.Damping force and the Doppler cooling limit

As shown in figure 1(b),in the one-dimensional (1D) Doppler cooling,the kinetic energy of the trapped atoms reduces as a result of an overall scattering force applied by two red-detuned(Δ <0)counter-propagating laser beams.Such a configuration of standing-wave laser beams is called ‘OM’.We have

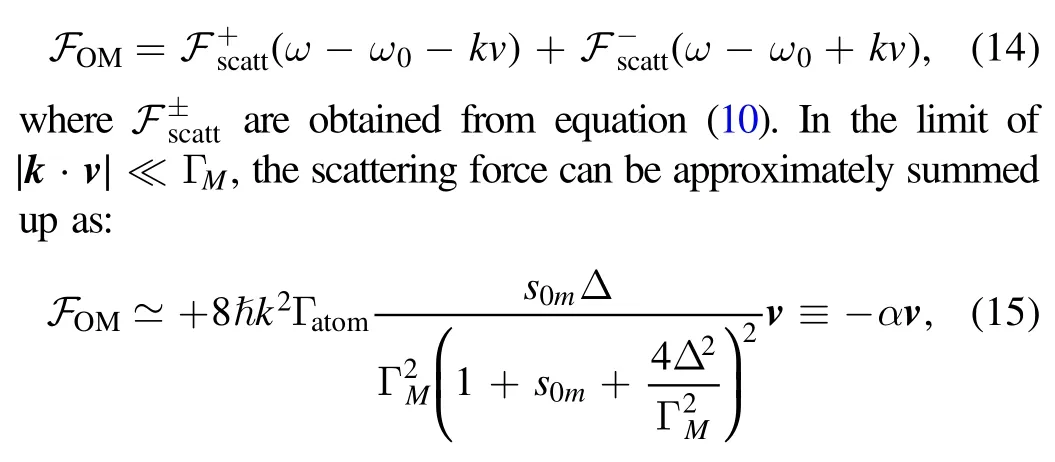
whereα>0 is a damping coefficient.For small enough velocities,the force is proportional to the velocity,which resembles a viscous damping force forΔ <0.Thus,the fluctuating laser field can decelerate atoms in a broad range of velocities.On the other hand,the atomic sample is heated due to the absorption/emission recoils in random directions.In the concept of Doppler cooling,the atom is cooled as a result of the difference in absorption probabilities and red-detuned counter-propagating laser beams.In the steady state,equating the heating with the damping force of equation (15) leads to non-zero kinetic energy.According to the equi-partition theorem [27,32],the Doppler temperature found from this kinetic energy is

that is(γ1γ3)1/2times higher than the limit for the case without laser fluctuations.

2.3.Number of capturing atoms in the MOT

The relatively compact MOT relies on both inhomogeneous magnetic fields and optical pumping that provide a strong radiative force.The local Zeeman shift of each sublevel should be adjusted in pace with the decrease of atomic velocity (the co-called Zeeman-compensated slowing).For the inhomogeneous coaxial fieldB(z)=bz zˆ ,(b>0),the Zeeman shift is expressed as ΔE mF(z)=gFμBmF B(z),wheregFis Landég-factor andμBis Bohr’s magneton.In this case,equation (13) can be modified as

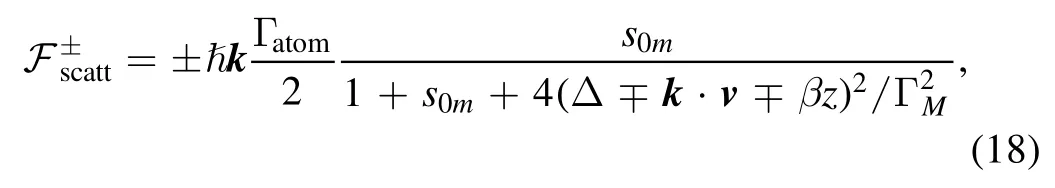
whereβ=μeffb/ħandμeffis the effective magnetic moment for the cooling transition.When both Doppler and Zeeman frequency shifts are small compared to the laser detuningΔ,the approximate Doppler force reads aswhere the damping coefficientαis defined in equation (15),and the spring constantkis given as/k=αβk.This relation is valid for the limit∣βz∣,∣kv∣≪Γatom,and implies that the Doppler force is also affected by the laser fluctuations.

The number of magneto-optically trapped atoms,N,can be determined when the MOT capture rate,R,equals to its loss rate due to collisions between thermal background vapour and cold trapped particles.Note that Γtrap=1/τtrap,whereτtrapis the Lifetime for an atom to remain in the trap.The loading dynamics of an MOT can be described as [33]

Here,ξis a loss rate coefficient andnMOTis the MOT atom density.The third term on the right-hand side of equation(19)represents losses due to two-body intra-trap collisions.When the loading and loss rates are independent ofN,equation(19)can be solved as

In the limit when losses due to intra-trap collisions can be ignored,the equilibrium number of atoms in an MOT,Nss,is given by [33]

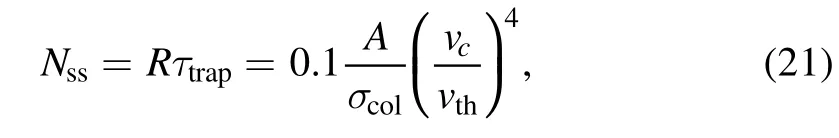
wherevcis the maximum capture velocity,vthis the mean thermal velocity of the background vapour,σcolis the collision cross-section between background and trapped atoms,andAis the cross-section of the trapping area.It is noted that the analysis is performed for a Gaussian beam in the fundamental mode,where the wavefront can be considered as a plane at the waist.In the presence of laser fluctuations,the effective deceleration saturates ataeff=ħk[Γatom/4m(1 +γ2γ3) ],withmbeing the atomic mass.

3.Numerical results and discussions

In this section,we study the effect of laser fluctuations on the radiation pressure force,the Doppler temperature,and the number of88Sr atoms trapped in an MOT.The corresponding atomic system parameters are summarized in table 1.The natural linewidth of the dipole-allowed(5s2)1S0→(5s5p)1P1transition is Γatom=2π×32 MHz,which leads to a Doppler cooling limit at theμK level.This transition has a short lifetime of～5 ns and is well suited for the first stage of cooling and trapping [34].In all numerical calculations,the frequency detuning is set toΔ=-0.5Γatom.

As mentioned,the radiation-pressure(or scattering)force originates from the photon scattered by the atomic dipole and is collinear to the direction of laser propagation.ForΔ <0,this force acts as viscous drag and competes against the atomic velocity,therefore viscously hinders the atomic motion.Figure 2 illustrates the velocity-dependence of the scattering force on a two-level atom in 1D-OMs for three different saturation regimes.Figure 2(a)is plotted for the case Γamp=Γphs=0,while the other ones derived from a fluctuating laser beam.It can be observed that the scattering force is always positive (negative) forv<0 (>0).The maxima for the scattering force occur atanddecreases quickly for larger velocities.A comparison between the figures suggests that both amplitude and phase fluctuations reduce the magnitude of scattering force.With a fluctuating laser field,the scattering force is nearly linear with velocity fors0≤1.Also,it can be inferred that in a strongfield regime (s0≫1),the phase fluctuation (related to the laser linewidth) is the dominant mechanism for damping of the atomic motion.In the weak-field limit,the damping rate,i.e.the slope of the curves nearv=0,is highly sensitive to laser fluctuations.In contrast,this sensitivity reduces in the strong-field limit.Figures 2(c)and(d)show how the range of capture velocity is extended with the phase fluctuation.The capture velocityvccan be estimated as the velocity of an atom that is stopped by a force equal to half of the maximum scattering force.Referring to equation (15),the range of capture velocity isvcΓatom/kfor non-fluctuating laser beams,while this range changes tovc≃ΓM/k=(γ1γ3)1/2Γatom/kfor the fluctuating laser fields.As it is expected,a wider range of capture velocities is attainable for the fluctuating laser fields.In other words,the ability to capture relatively hot atoms from the atomic beam increases by broadening the linewidth of the cooling fluctuating laser.It is worth mentioning that whenΓphs=Γatom,the capture velocity is twice its value for the non-fluctuating case.This doubled value for the capture velocity(≃ 2 8 m s-1) is still relatively small compared to the most probable typical velocities of a88Srthermal beam(≃ 4 00 m s-1).It should be noted that due to the lack of restoring forces,the OMs do not trap neutral atoms that have been displaced from the center.This is why the Zeeman effect is used in an MOT to decelerate atoms before Doppler cooling [35].

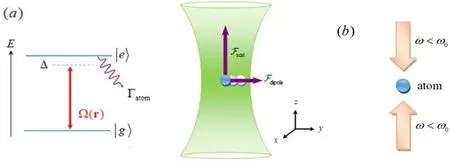
Figure 1.(a) Schematic model for optical force calculation;The ground and excited states∣g〉 and∣e〉 are coupled by a resonant laser of Rabi-frequency Ω (r),(b)cooling process in 1D optical molasses.

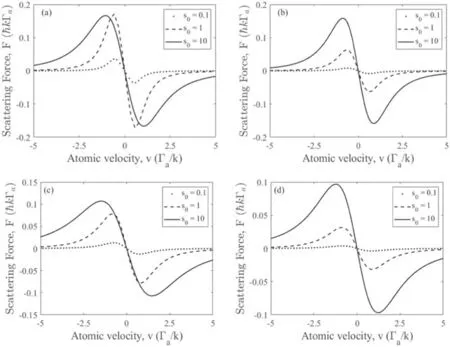
Figure 2.Atomic velocity dependence of the scattering force for 1D optical molasses.(a)Γamp=Γphs=0,(b)Γamp=0.5Γatom,Γphs=0,(c)Γamp=0,Γphs=0.5Γatom,and (d)Γphs=Γamp=0.5Γatom.

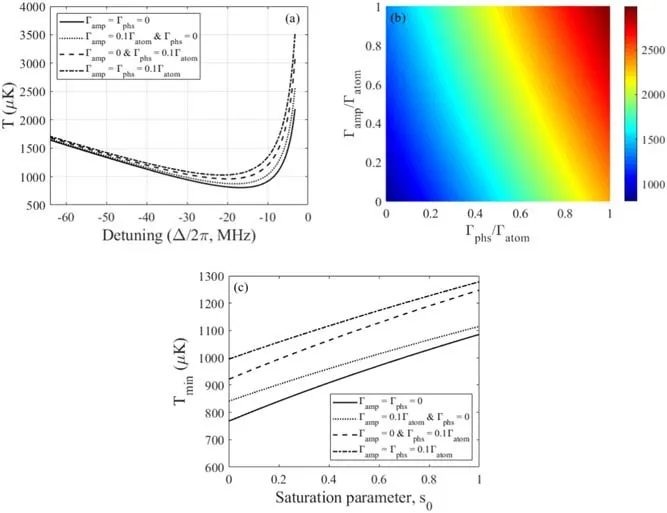
Figure 3.Doppler temperature of the optical molasses as a function of(a)the frequency detuning,(b)laser fluctuations,and(c)Doppler cooling limit Tmin as a function of saturation parameter s0.

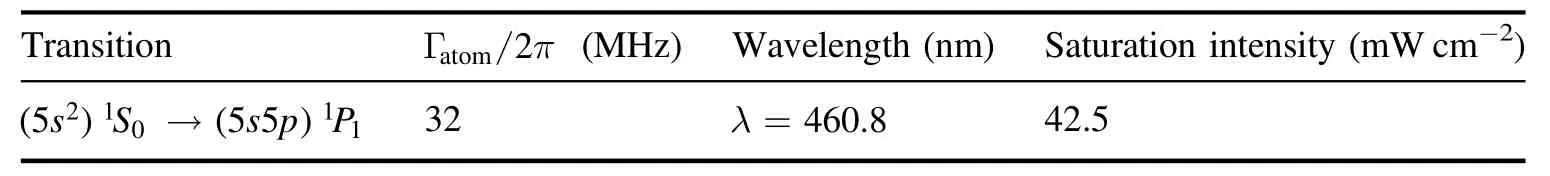
Table 1.Corresponding parameters for the optical transition in a 88Sr atom.

A question may arise in the concept of laser-cooled atoms: whether this collection of atoms can be said to have a temperature.If there were no other external control on the atomic motion,all atoms would quickly decelerate tov=0,and the sample would reach zero temperature,which evidently is a nonphysical result.Doppler cooling forbids cooling to zero temperature because the stochastic feature of the momentum kicks due to the cycles of the absorption and spontaneous emission leading to an atomic momentum fluctuation around the steady-state value〈p〉=0.This process heats up the atom and causes the equilibrium temperature to be a non-zero value.On the other hand,the competition between this heating with the damping force leads to non-zero kinetic energy in a steady state.There is some light-induced heating that must be taken into account,and it derives from the discrete size of the momentum steps that the atoms undergo with each emission or absorption [36].At this state,the heating and cooling rates for atoms in OM should be equal.Figure 3(a) shows the Doppler temperature of the Sr atomic cloud as a function of frequency detuning at a low saturation intensity regime.The Doppler cooling limit changes fromTD=767.9μK forΓphs=Γatom=0toTD=1027.1μK for a fluctuating laser withΓphs=Γatom=0.1 Γatom.As can be seen,the Doppler cooling limit is increased above 1 mK with the stochastic laser fluctuations.To overcome this issue and reach the minimum achievable limit,it is required to increase the red-detuning laser frequency.Figure 3(b)shows the Doppler temperature variation as a result of the amplitude and phase fluctuations.The color bar is scaled toμK.

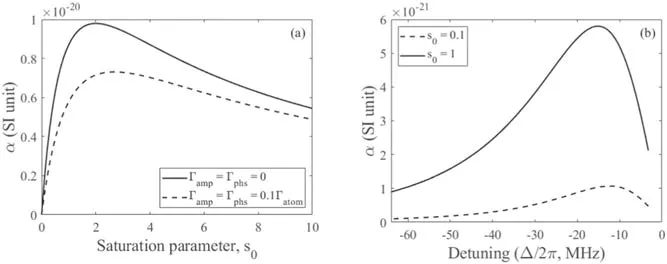
Figure 4.Damping coefficient α as a function of (a) saturation parameter s0,and (b) the frequency detuning.

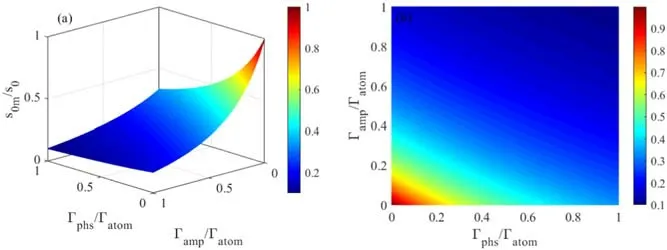
Figure 5.The modified saturation parameter versus laser fluctuations at the low saturation intensity limit.

At low saturation of the atomic transitions0≪1and in the absence of the laser fluctuations,the Doppler limit for88Sr cold atomic sample is found to beħΓatom/ 2KB=767.9μK.Figure 3(c) shows the minimum Doppler temperatureTminis an increasing function of the saturation parameters0.The Doppler limit increases with the laser fluctuations,and the effect of laser linewidth(i.e.Γphs)is more dominant compared to the laser power stability.Fors0≪1,considering laser fluctuations withΓphs=Γamp=0.1Γatom,the Doppler limit is≃995.3μK,which is almost 30%higher than that of the case without fluctuations.

Equation (15) indicates that the damping coefficient is affected by the modified saturation parameter,the modified decay rate,and the frequency detuning.In figure 4(a),we plot the damping coefficientαas a function ofs0with and without laser fluctuations.It can be noticed that for both cases,by increasing the saturation parameter of the laser field,αincreases almost linearly in a low saturation limit,then it reaches a maximum value and decreases uninterruptedly for a high saturation limit.Also,the value of the damping coefficient is higher without laser fluctuations.Figure 4(b) shows the variations ofαin terms of the laser frequency detuning.The curves are obtained for a fluctuating laser field with Γphs=Γamp=0.1Γatom.In both cases,the maximum damping coefficient occurs in

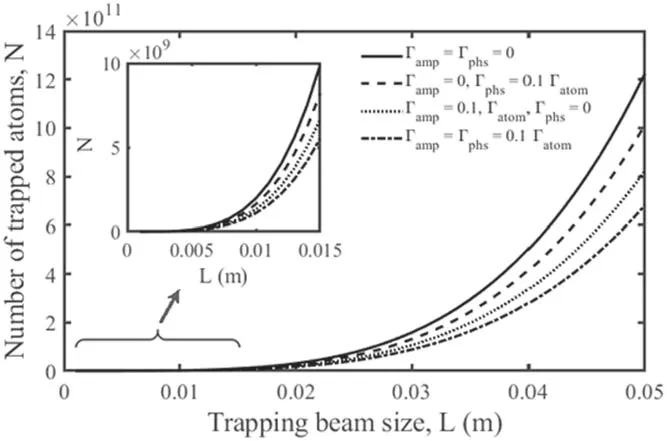
Figure 6.Number of trapped atoms versus 1/ebeam diameter for 10-14 cm -2.s0=1.Theother parameters are vth=470 m s-1,σcol=8.1×

In figure 5(a),we plot the modified saturation parameter,s0m,in terms of laser fluctuations for the low saturation intensity limit and frequency detuningΔ=-0.5Γatom.As it is expected,the modified saturation parameter has its highest value in the absence of laser fluctuations.Like the Doppler limitTD,the modified saturation parameter exhibits more sensitivity to the laser linewidth variation rather than laser power stability.This implies that to drive atoms between the ground and excited states and further control the momentum transfer between laser light and atoms,counter-propagating laser beams of carefully chosen phase differences should be utilized.Figure 5(b) shows the top view of the variations of the modified saturation parameter.

As a final point,in figure 6,we investigate the effect of the stochastic processes associated with the phase and the amplitude of the trapping laser beam on the number of trapped atoms in an MOT with a fixed magnetic-field gradient.The length of the trapping region is defined to be the 1/ e diameter of the trapping laser beam’s intensity.We assume that the surface area of the trapping region is a sphere with a diameter equal to the beam diameter,so the cross-section of the trapping area isA=πL.2 Background particles with a speed lower than the capture velocityvc,are trapped in the MOT.To consider the fluctuation of the force arising from the randomness in the number of photons scattered,we assumedI=Is,i.e.the effective deceleration experienced by the atoms isaeff=(1/2)aFmax(I=Is).Figure 6 illustrates the nonlinear increase of the number of trapped atoms with the beam diameter of the trapping laser.Whileσcolandvthare considered to be constant,vcdepends on the laser fluctuations.The capture velocity relates to the effective surface area of the trap volume via(withLbeing the radius of trapping area),and.For a fixed intensity,a larger beam diameter always causes more atoms to be trapped in an MOT.Also,it can be observed that the number of trapped atoms in the MOT increases very rapidly with the beam diameter and is extremely sensitive to stochastic laser fluctuations.Both amplitude and phase fluctuation of the laser leads to a reduction in the number of trapped atoms.For example,for a laser beam with a diameter of 5 mm,the phase fluctuations decrease by almost 17% of the number of trapped atoms.However,the amplitude fluctuations decrease nearly 33% of the number of trapped atoms under the same conditions.Therefore,it can be concluded that the effect of laser power stability on reducing the number of trapped atoms is more substantial than the effect of laser linewidth.

Note that the present model for Doppler cooling in twolevel atoms does not consider the multi-level structure of realistic atoms,polarizations of the laser fields,and the dipole force.A comprehensive model that imparts these phenomena displays several ‘sub-Doppler’ cooling mechanisms,leading to temperatures two orders of magnitude cooler than the present Doppler limit.

4.Conclusion

In summary,we have investigated the effect of laser fluctuations on the Doppler cooling and trapping of88Sr atoms.Using a semi-classical approach for a two-level atom,the relations for optical forces,the damping coefficient,Doppler temperature,and the number of atoms in the MOT have been obtained.We have shown that the relevant physical quantities in the cooling process are strongly affected by the laser amplitude and phase fluctuations.It is shown that the laser linewidth extends the capture velocity range and decreases the damping rate of the atoms in the OMs.Also,it is found that the Doppler cooling limit is higher than the predicted Doppler theory for non-fluctuating lasers.This indicates that due to temporal laser fluctuations,an extra-heating mechanism in Doppler cooling of88Sr exists,which is an extension of the heating mechanism recognized in [18] due to spatial laser fluctuation.Next,we have expanded the analysis to the MOT to show that both the velocity capture range and trap potential are affected by the laser fluctuations.It is concluded that the effect of laser power stability on reducing the number of trapped atoms is more substantial than the effect of laser linewidth.The results provide an insight into Doppler cooling with fluctuating lasers which finds applications in novel devices such as optical atomic clocks and quantum sensors.

Acknowledgments

The present study is conducted at the Iranian Center for Quantum Technologies (ICQTs).

Simulations

All the simulations are performed using MATLAB software.

Code availability

All relevant code used in this paper are available from the corresponding author upon reasonable request.

Disclosure statement

The authors report no potential conflict of interest.

ORCID iDs