Relativistic calculations on the transition electric dipole moments and radiative lifetimes of the spin-forbidden transitions in the antimony hydride molecule

Yong Liu(刘勇), Lu-Lu Li(李露露), Li-Dan Xiao(肖利丹), and Bing Yan(闫冰)

Institute of Atomic and Molecular Physics,Jilin University,Changchun 130012,China

Keywords: SbH,transition properties,radiative lifetimes

1. Introduction

The significance of the relativistic effect on the accurate descriptions of the transition properties of atoms and molecules containing heavy elements is well known in the quantum chemistry field.[1–3]With the increase in atomic number,the relativistic effects increase gradually. One example is the electronic triple-single transition that is forbidden by the electric dipole selection rule but becomes allowed in the heavy counterpart because of the spin–orbit coupling.

The spin-forbidden transitions in the low-lying states of group V hydrides have attracted considerable research interest because of the···(π∗)2electron configuration. With regard to the antimony hydride molecule (SbH) as an example, early experiments were mainly focused on the spinallowed transition A3Π←X3Σ−,and obtained accurate spectroscopic information of the ground state X3Σ−via analysis of the absorption spectrum,[4]rotation spectrum,[5,6]and infrared spectrum.[7–9]Limited by the technology, the spinforbidden transitions b1Σ+/a1∆–X3Σ−were not investigated until 1996. The vibration–rotation spectrum of the a2–X21 and b1Σ+0+–X3Σ−xof the SbH molecule were observed with a high-resolution Fourier transform spectrometer[9,10]and the spectroscopic constants of the X3Σ−, a1∆, and b1Σ+states were determined. Shestakovet al.[11]measured the b1Σ+–X3Σ−transition of SbH using a laser-induced fluorescence spectrum,and obtained the radiative lifetime(τ=173±3µs),the electric dipole transition moment (µ0=±0.014ea0andµ1=∓0.023ea0) and the magnetic dipole transition moments (|M| = 1.62 Bohr magnetons) of this transition. On the theoretical side, most of the research has focused on valence and Rydberg states. It is worth noting that Alekseyevet al.[12]utilized the MRD-CI method to calculate the transitions of SbH and obtained the electric transition dipole moments of the b0+–X10+and b0+–X21 transitions (0.00409ea0and−0.02682ea0)and the radiative lifetime of the b1Σ+(υ′=0)state (104 µs). The computedµ1agrees well with the experimental value, while theµ0value and the radiative lifetime deviate greatly from the experimental values.

Very recently, our group studied the electronic structure of the low-lying states of the SbH molecule, including spectroscopic and transition properties, with state of the artab initiocalculations using the internally contracted multireference configuration interaction method plus the Davidson correction (icMRCI+Q).[13]It showed that the radiative lifetime of the b1Σ+state should be between 55 µs and 153 µs.There, we took account of spin–orbit coupling via the socalled state-interacting method. With the inclusion of several high-lying spin-free states,the calculated result is 153µs;this is in reasonable agreement with the experimental value of 173±3 µs,[11]while the deviation from the experiment still exists.

In most of the non-relativistic calculations on light atoms or molecules,[14–18]the use of the state-interacting method to account for spin–orbit coupling works quite well. It is nevertheless quite difficult dealing with the elements at the bottom of the periodic table because of the nature of perturbation theory, which is sensitive with regard to the number of spin-free (non-perturbated) states that participate in the computation. On the other hand, it is well known that the most straightforward method to consider the relativistic effect is to directly solve the 4-component Dirac equations. To double check our previous results and provide a benchmark for the spin-forbidden transitions b1Σ+–X3Σ−of SbH theoretically,here, we reconsider the radiative lifetimes of the b1Σ+state using the non-perturbated approach to deal with the relativistic effect,including both scalar correction and spin–orbit coupling, based on the exact 2-component (X2C) equation that can be derived from 4-component Dirac equations.

This paper is organized as follows. The computational method is described in Section 2. In Section 3 we present the computational results and discussions on the electronic transitions of SbH.Finally,a summary is presented in Section 4.

2. Computational details

In the present work,we performed the MRCI calculations based on the X2C Hamiltonians[19–23]for the electronic states of the SbH molecule with the DIRAC2019 code.[24,25]

For the Sb atom, we employed uncontracted corevalence Dyall basis sets: double-ζ(dyall.acv2z), triple-ζ(dyall.acv3z),and quadruple-ζ(dyall.acv4z).[26,27]For the hydrogen,we used Dyall’s triple-ζ(dyall.v3z)and quadruple-ζ(dyall.v4z)basis sets.[28]The first set of calculations was carried out using the two-component Hartree–Fock method. Subsequently, the MRCI calculations, which are implemented in the KRCI module of DIRAC,[29–32]were performed.

Details of the settings of the generalized active spaces(GAS) used are listed in Table 1. The Sb 5σ1/2,σ1/2,π1/2,andπ3/2and the antibondingσ∗1/2were placed in a complete active space(GAS II),yielding a distribution of six electrons in five Kramers pairs (6 in 5). Core–valence and core–core correlations were included by allowing up to two holes in the 4d shell (GAS I) of Sb. Finally, the electronic dynamic correlation is considered by the singles and doubles excitations from the combined spaces of GAS I and GAS II into the virtual spinor space(GAS III)corresponding to the energy thresholds of 1Ehand 5Eh(Hartree), respectively. We keep the remaining 36 electrons frozen; this means that we cause them to be occupied during the CI procedure.

The expressions of the complete basis set(CBS)limit are presented below:[33–37]

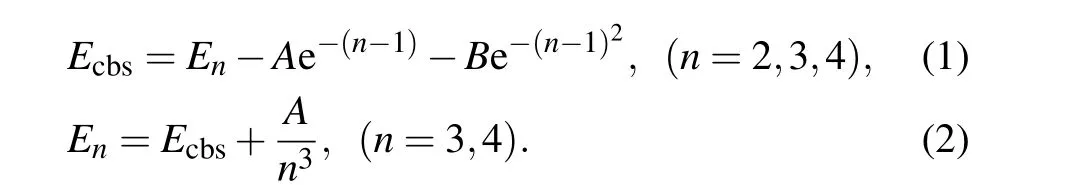
According to the potential energy curves (PECs) of theΩstates,the corresponding spectroscopic constants of the bound states were determined by resolving the one-dimensional nuclear Schr¨odinger equation with the aid of the LEVEL program,[38]including the excitation energyTe, the equilibrium internuclear distanceRe,vibrational constantsωeand the rotation constantBe. The electric transition dipole moments(TDMs) were computed. Finally, the spontaneous radiative lifetimes of the several lowest energy transitions could be predicted.

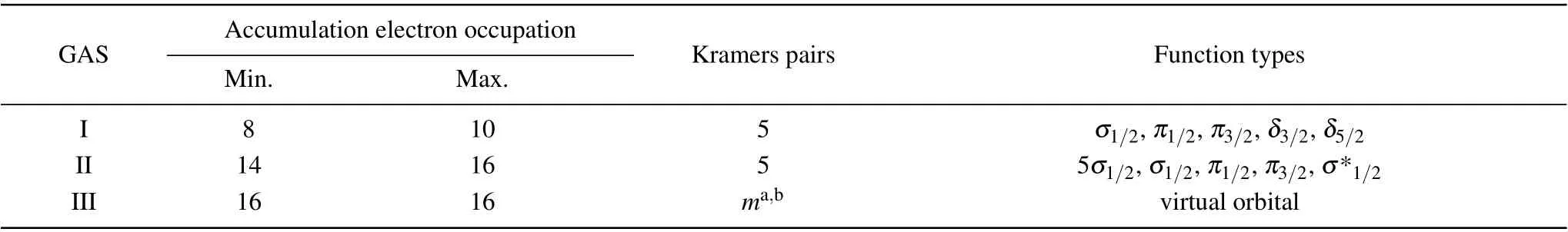
Table 1. Generalized active spaces and occupation constraints for the SbH molecule in the symmetry double group C∞v.

aTriple-ζbasis set:m=30(1Ehthreshold)and 50(5Ehthreshold).bQuadruple-ζbasis set:m=50(1Ehthreshold)and 110(5Ehthreshold).

3. Results and discussion

3.1. Spectroscopic constants of Ω states

We calculated the single-point energies of the lowest four states (X3Σ+0+[X10+], X3Σ+0+[X21], a1∆2[a2], and b1Σ+0+[b0+])in the Franck–Condon region using the MRCI method;the corresponding PECs are depicted in Fig.1,and the data are listed in the supplementary material(Table I).TheseΩstates are deep well-bound states. From the computed energies of theΩstates,the corresponding spectroscopic constants of the bound states were determined. These results are tabulated in Table 2 together with available experimental and theoretical values for comparison.

It indicates that the spectroscopic constants tend to be more accurate with the increase in the cutoff energy and the improvement of the basis set. Taking Dyall’s quadruple-ζbasis set and the cutoff energy 5 a.u.as an example,theRevalues for the X10+and X21 states are calculated to be 1.6947 ˚A and 1.6937 ˚A,which are 0.0053 ˚A and 0.0082 ˚A smaller than the experimental values,[10,39]respectively. After considering the basis set extrapolations,theRevalue of the X10+state can be close to 1.701 ˚A.The spin-splitting energy of the X3Σ−state is 623.1 cm−1, which is only 37 cm−1underestimated from the experimental value of 660 cm−1.[5]The spectroscopic constants of the X10+and X21 states are much closer to the experimental values than those calculated using our previous method.[13]The calculated values ofRefor the a2 and b0+states are 0.02407 ˚A (1.4%) and 0.02045 ˚A (1.2%) smaller than the experimental values,[10,11]and the errors of the correspondingTecalculated value are 782.6 cm−1(11.4%) and 625.2 cm−1(4.6%),respectively.

Fig. 1. The PECs for the X10+, X21, a2, and b0+ states with the Dyall’s quadruple-ζ basis set and the cutoff energy 5 a.u.

Table 2. Computed spectroscopic constants of the Ω states for the X10+,X21,a2,and b0+ states.

3.2. Analysis of the transition properties

Based on the above statements, we calculated the electric transition dipole moments of the b0+–X10+and b0+–X21 transitions and the results are depicted in Fig.2. The data presented in this paper are included in the supplementary material(Tables II and III). As illustrated in Fig. 2, we found that the trends for two transitions are nearly linear with the changes in the internuclear distance.

For the b0+–X10+transition, the change of transition dipole moments obtained by the state interaction methods(a)–(d)is relatively slow with the increase in the internuclear distance, while those calculated using the relativistic methods(e)–(f) increase relatively rapidly. And from the curves from the relativistic method,the TDM values of the b0+–X10+transition are sensitive and improved with the increase in the basis set under the same cutoff energy of 1 a.u. (lines e and f in the upper panel of Fig.2),while they are insensitive and with small improvement for the b0+–X21 transition;also,when the value reaches 5 a.u., the cutoff threshold greatly reduces the uncertainty of the basis sets.The above results indicate the stability and convergence of the present computational scheme.Thus, the basis set extrapolation and the effect of the cutoff threshold are necessary for consideration.

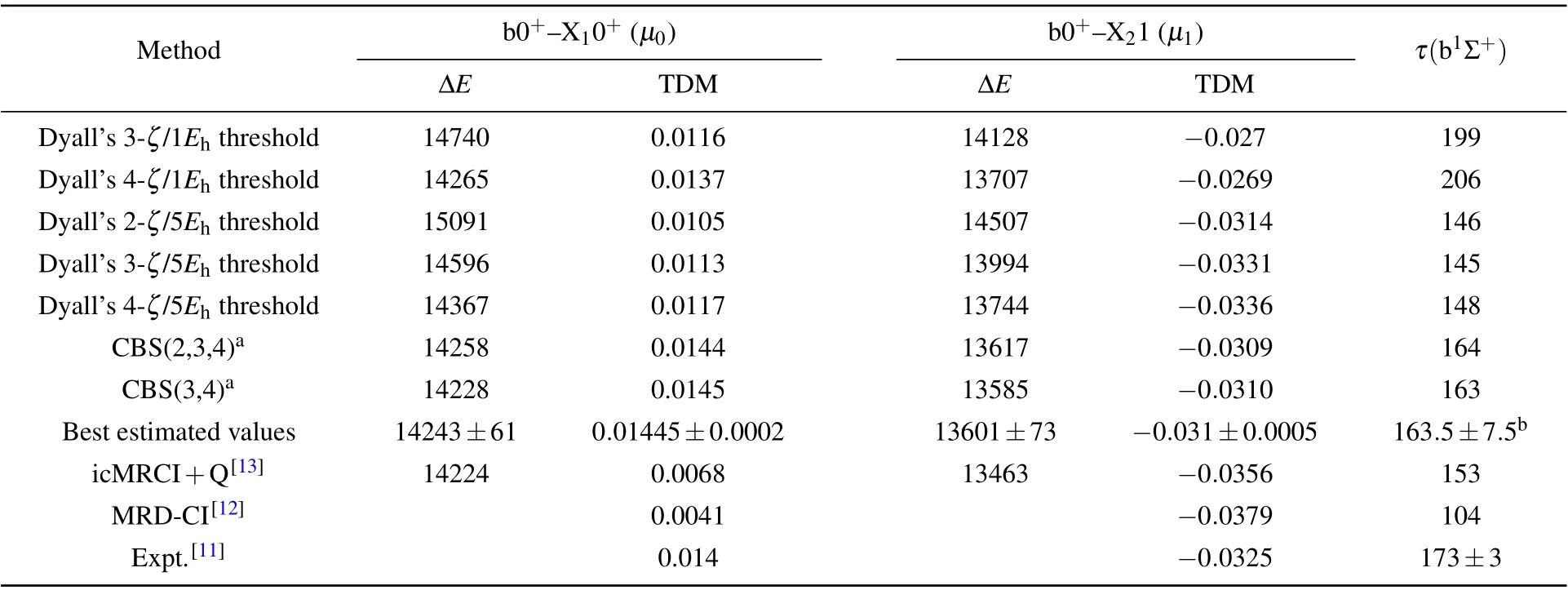
Table 3. The energy difference (cm−1) and transition dipole moments (a.u.) of the b0+–X10+ (µ0) and b0+–X21 (µ1) transitions at Re and the radiative lifetime τ (µs)of the b1Σ+ (v′=0)for the different methods.

The TDM values of the b0+–X10+and b0+–X21 transitions atReand the corresponding radiative lifetimes for the b1Σ+(υ′=0)state are listed in Table 3.According to Table 3,the values ofµ0reported using our previous calculations[13]and other theoretical calculations[12]are about a factor of two to three lower than the experimental value,while theirµ1values agree well with the experimental value.[11]AtRe,the transition dipole moments of the b0+–X21 (µ1) transition with a cutoff energy of 5 a.u. are−0.0331 a.u. and−0.0336 a.u.,respectively,with different basis sets;the best estimated value averaged with formulas(1)and(2)is−0.031±0.005,which is only about 0.001 a.u.overestimated when compared with the experimental value(−0.0325 a.u.). For the b0+–X10+transition, the best estimated value ofµ0is in excellent agreement with the experimental value,[11]and all experimental significant digits are reproduced. The total radiative lifetimeτof the b1Σ+–X3Σ−transition is obtained according to the following formula:

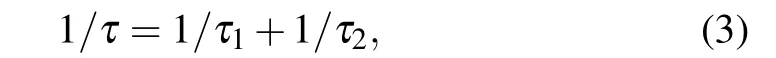
whereτ1andτ2represent the radiative lifetimes of the b0+–X10+and b0+–X21 branch transitions,respectively,andτrepresents the radiative lifetimes of the b1Σ+–X3Σ−transition;that is,the spontaneous radiative lifetimes of the b1Σ+state.

Considering the incompleteness of the basis sets,the twoparameter and three-parameter basis set extrapolation formulas are used to extrapolate the energies and TDM,and obtain the radiative lifetime range(163.5±0.5µs). The two extrapolation schemes (formulas (1) and (2)) determine the uncertainty arising from the basis sets. The detailed data are presented in Table 3. Next,we proceed with uncertainty analysis,and here we mainly consider the influence of the truncated threshold on the energy difference (∆E) and TDM. For the b0+–X10+transition, the uncertainties of ∆Eand TDM are 46 cm−1and 0.0001 a.u.while,for the b0+–X21 transition,the uncertainties are 57 cm−1and 0.0005 a.u.,respectively.Due to the propagation of the uncertainty of the energy difference and TDM, we determined that the error bars of the radiative lifetime for the b1Σ+(υ′=0)state are 7µs. Taking the averaged values of the two extrapolation results as the reference value,the combinations of this value and the uncertainties caused by the extrapolation and truncation threshold are selected as‘best estimated values’. Among these values,the best radiative lifetime of the b1Σ+(υ′=0)state is 163.5±7.5µs,which is in reasonable agreement with the experimental value(173±3µs)measured by Shestakovet al.[11]The present work improves 10µs(∼7%)and 60µs(∼60%)values of the total lifetime of the b1Σ+(υ′=0)state compared with our previous and other theoretical computations and, more importantly, predicts reasonable electric dipole moment values of branch transitions.

Table 4. The transition dipole moments (a.u.) and the radiative lifetime τ(s)of the X10+–X21 and X21–a2 transitions at Re.

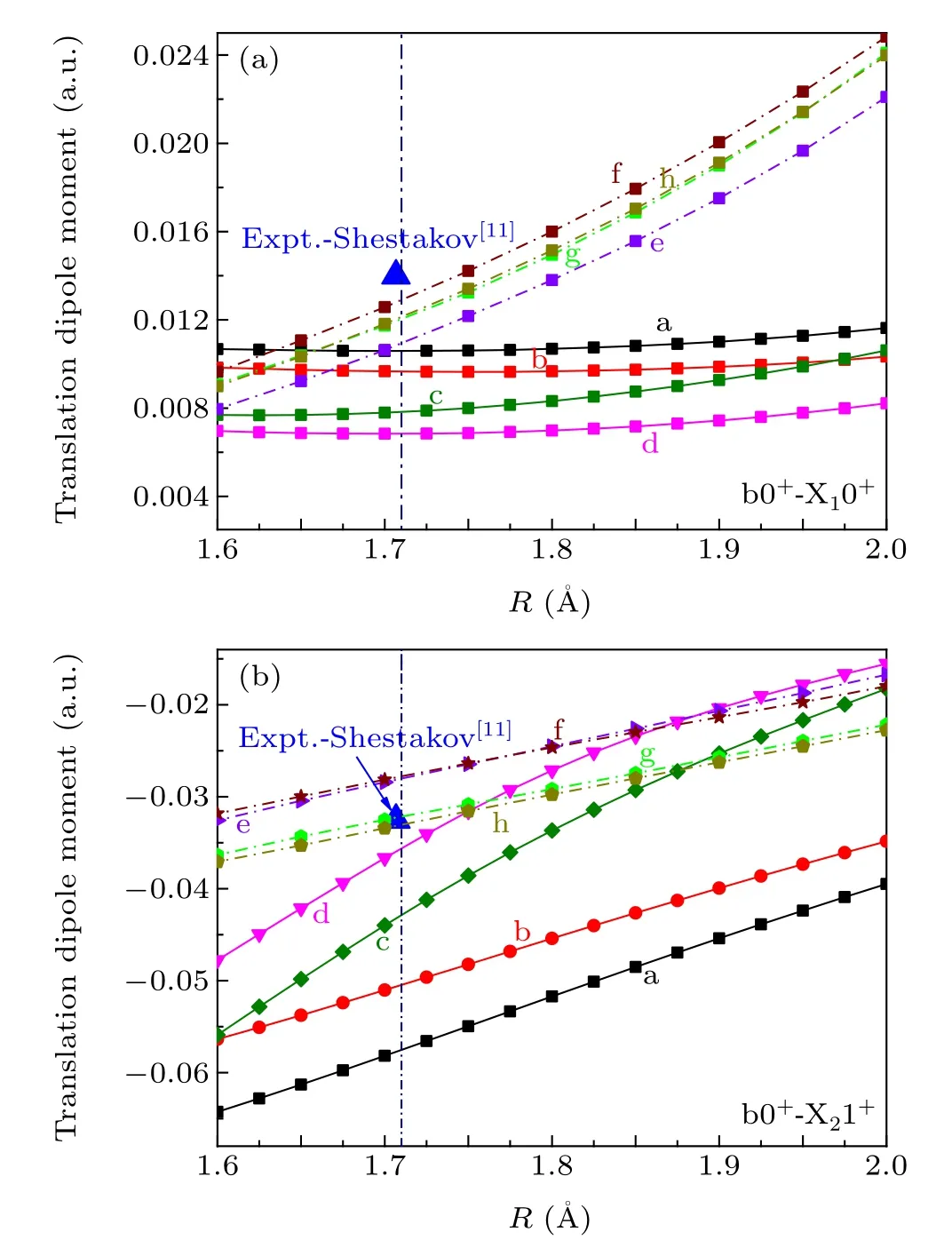
Fig.2.The transition dipole moments of the b0+–X10+and b0+–X21 transitions for SbH.(a)The results of all-electron basis set calculations for the state interaction technique;(b)the results of pseudopotential basis set calculations for the state interaction technique; (c) the same as (a), but with high-lying states included; (d) the same as (b), but with high-lying states included; (e)the results of Dyall’s 3-ζ basis set and energy threshold of 1 Hartree for the relativistic method; (f) the results of Dyall’s 4-ζ basis set and energy threshold of 1 Hartree for the relativistic method;(g)the same as(e),but the energy threshold is 5 Hartree;(h)the same as(f),but the energy threshold is 5 Hartree.

Based on the reasonable results of the b0+–X10+and b0+–X21 transitions using the(g)–(h)computational schemes,we also analyzed the transition dipole moments and the corresponding radiative lifetimes of the X10+–X21 and X21–a2 transitions. The results are shown in Fig. 3 and Table 4, and detailed data of the transition dipole moments are included in the supplementary material(Table IV and Table V).As shown in Fig. 3,the transition strength decreases with the increase in the bond distance. AtRe,the transition dipole moments of the X10+–X21 transition are 0.00638 a.u.and 0.00660 a.u.under the Dyall’s triple-ζand quadruple-ζbasis sets,and the corresponding spontaneous radiative lifetimes of the X21 (υ′=0)state are obtained as 58.2 s and 48.6 s,respectively. These two values are about half of the theoretical result of 105 s obtained by Alekseyev.[12]However, since the radiative lifetime of the b1Σ+state obtained by this group has a difference of about 40%from the experimental value,[11]our results have certain credibility based on this analysis. Furthermore, the spontaneous radiative lifetimes for the a2(υ′=0)state are calculated to be∼8 ms.

Fig.3. The transition dipole moments of the X10+–X21 and X21–a2 transitions for SbH. The meanings of the symbols c, d, g, and h in the figure are the same as those of the remarks in Fig.2.

4. Conclusion

Calculations on the transition properties of the first three states of the SbH molecule were performed under the relativistic framework with the aid of the exact two-component Hamiltonians(X2C).The spectroscopic constants for the first fourΩexcited states were determined and compared with the previous values. In terms of transitions,the radiative lifetimes of the b1Σ+(υ′=0)state were calculated. The best result of 163.5±7.5µs is in reasonable agreement with the experimental value(173±3µs). The spontaneous radiative lifetimes of the X21 (υ′=0) and a2 (υ′=0) states are calculated to be 48.6 s and∼8 ms, respectively. The present work predicts not only the value of the total lifetime of the b1Σ+(υ′=0)state but also the reasonable electric dipole moment values of branch transitions.The present work is a benchmark computation of the electric dipole moments and lifetime of transitions in the SbH molecule, and is of great theoretical significance for the selection of computational schemes in further transition properties investigations in heavy elements containing molecular systems.

Data availability statement

The data that support the findings of this study are openly available in Science Data Bank at https://www.doi.org/10.11922/sciencedb.j00113.00018.

Acknowledgments

We are grateful for the computational support from the High Performance Computing Center (HPCC) of Jilin University and the high performance computing cluster Tiger@IAMP.Project supported by the National Natural Science Foundation of China(Grant No.11874177).