Space-like Blaschke isoparametric submanifolds in the light-cone of constant scalar curvature

Hongru SoNG(宋虹儒)Ximin LIU(刘西民)

School of Mathematical Sciences，Dalian University of Technology，Dalian 116024，China E-mail : gaozheng-shr@163.com; cmliu@dlut.edu.cn

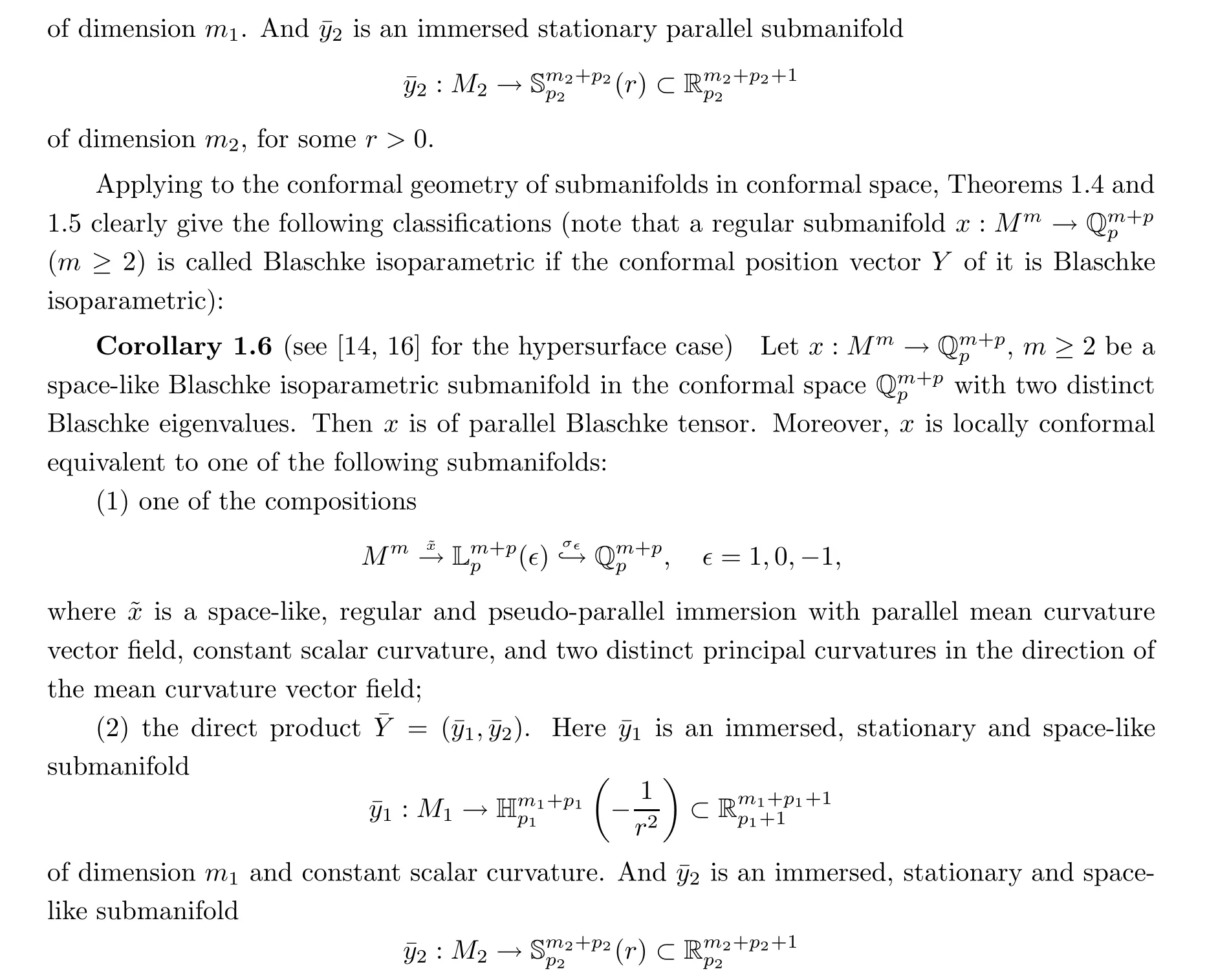
of dimension m2and constant scalar curvature， for some r ＞0.

Remark 1.7 The submanifolds mentioned in (2) of Corollary 1.6 can be explicitly expressed in the same way as we have done previously for hypersurfaces (see [5， 6]); see also the higher codimensional case in [7] for s=0.

2 Basic Geometric Invariants of the Conic Space-Like Submanifolds

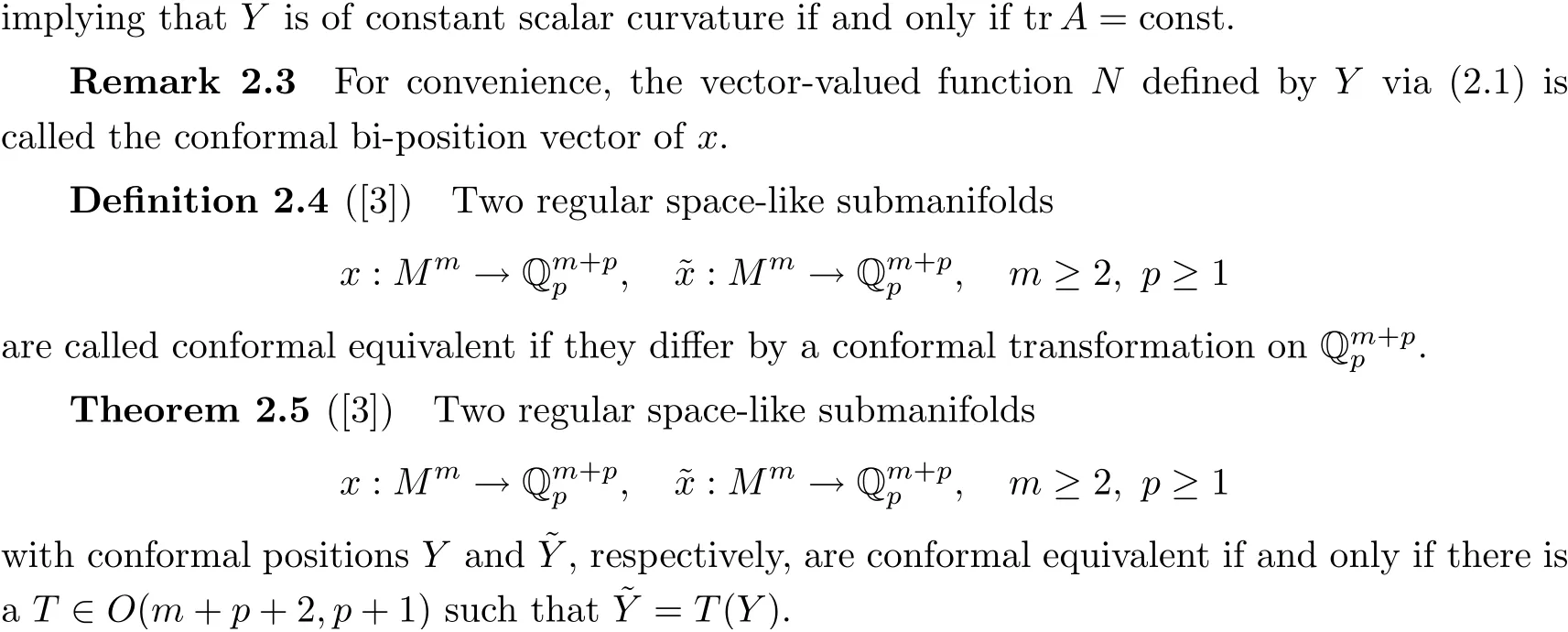
This section provides some geometric basics， essentially coming from the conformal geometry of regular space-like submanifolds in the Lorentzian space forms (see for example [2， 3]or [4]) dating back to the work of C.P. Wang ([1]) on the M¨obius geometry of umbilic-free submanifolds in the unit sphere.

3 Proof of Theorem 1.1 and Examples Appeared in Theorem 1.4

4 Proofs of Theorems 1.4 and 1.5

4.1 On the Blaschke form and connections of Y

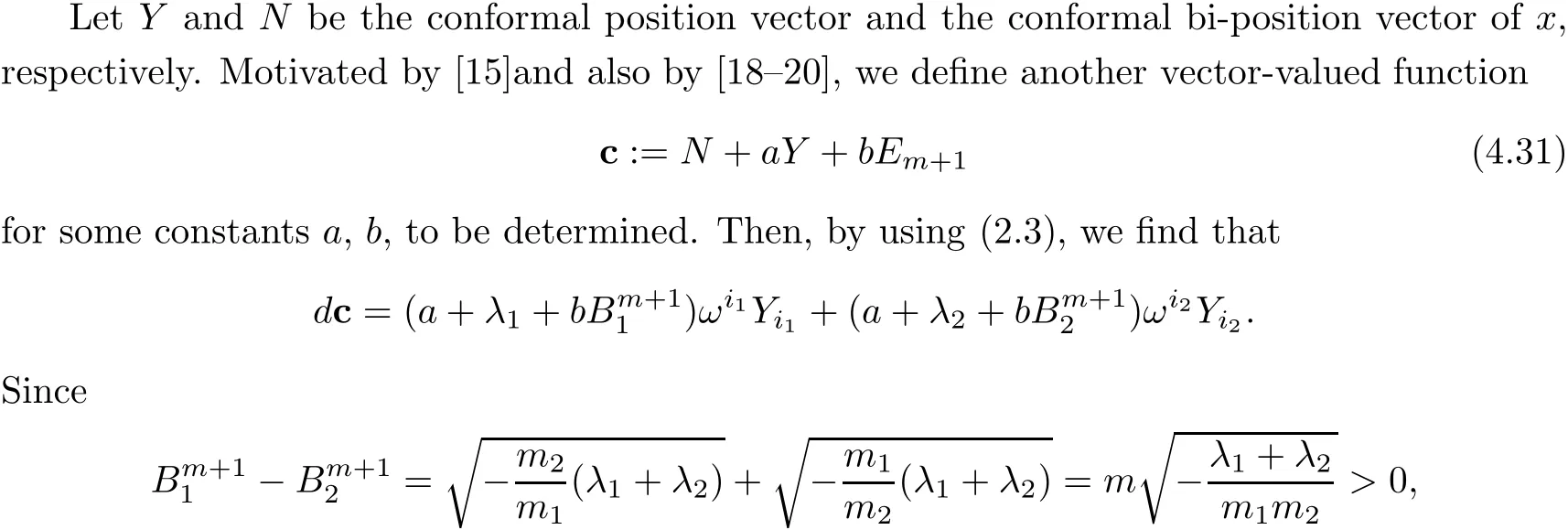
4.3 The associated vector field c