Solutions and memory effect of fractional-order chaotic system:A review
2022-06-29ShaoboHe贺少波HuihaiWang王会海andKehuiSun孙克辉
Shaobo He(贺少波), Huihai Wang(王会海), and Kehui Sun(孙克辉)
School of Physics and Electronics,Central South University,Changsha 410083,China
Keywords: fractional calculus,fractional-order chaotic system,numerical approximation,memory effect
1. Introduction
As with integer-order calculus, fractional-order calculus has been proposed for more than 300 years. In recent years, with the increase of computing power, theoretical foundation and numerical simulation of fractionalorder calculus attracted widespread attention.[1]Meanwhile, fractional-order calculus is very important in many real engineering applications such as signal processing,[2]laser treatment,[3]artificial intelligence,[4]control,[5]biological information,[6]turbulence,[7]non-Newtonian fluid mechanic,[8]image enhancement,[9]chaos,[10]ship power system[11]and complex network.[12]
Among these,fractional-order nonlinear chaotic systems have been extensively studied by researchers. At the beginning of the study,the fractional-order derivative is introduced to the integer-order systems and dynamics of those systems are investigated. Namely, suppose that the integer-order chaotic system is denoted as

wherex1,x2,...,xdare the state variables,dis the system dimension, andDqt0is the fractional-order derivative.There are many such systems such as fractional-order Lorenz system,[13]fractional-order Chen system,[14]fractional-order Liu system,[15]fractional-order L¨u system[16]and fractionalorder hyperchaotic systems.[17–19]It shows that, compared with their integer-order counterparts,those systems also have rich dynamics and the derivative order can be treated as a bifurcation parameter. Since fractional-order derivative can be used to model nonlinear systems, fractional-order chaos in the real systems has been investigated.[20–22]And applications of fractional-order chaotic systems in information security field show potential application value,for example,image encryption[23]and voice encryption.[24]
As a result,if we search the key word“fractional chaotic”in Web of Science,it shows in Fig.1 that the publications regarding fractional-order chaotic systems as well as their citations increase with year dramatically. As mentioned above,researches of fractional-order chaotic systems have became to a hot spot.

Fig.1. Times cited and publications over time with“fractional chaotic”in the title through Web of Science(10th Dec. 2021).
However, there is no uniform definitions for the fractional-order calculus. At the early stage,Riemann[25]and Liouville[25]laid the foundation for fractional definitions.And later, Caputoet al.[27]developed the work and enriched the study of fractional calculus. At present, there are three classic definitions, namely, Riemann–Liouville definition,[28,29]Caputo definition[29]and Grunwald–Letnikow definition.[29]In recent years, there are also many other mathematical researchers who developed the fractional-order calculus. Several new definitions are proposed, such as conformable definition,[30]Weyl definition,[31]fractalfractional definition[32,33]and Caputo–Fabrizio definition.[34]For fractional-order chaotic systems,Caputo fractional calculus are the most widely used and investigated. Other definitions such as Grunwald–Letnikow definition and conformable definition are also introduced to the chaotic systems. As a result, there are also fractional-order chaotic systems investigated with new derivatives, such as fractional derivative with non-local and non-singular kernel[35]and fractal–fractional differential operator.[36]Based on these definitions of calculus operators, the numerical solution algorithms of fractional calculus operators mainly include the following categories.
1) Analytical method: Adomian decomposition algorithm,[37]homotopy perturbation algorithm,[38]differential transformation algorithm[39]and variational iteration algorithm,[40]etc.
2) Finite difference method: Prediction-correction algorithm,[41]Crank–Nicholson format algorithm,[42]etc.
3) Transfer method: Frequency method[43]and Laplace transform method.[44]
4) Others: Meshless algorithm[45]and finite element algorithm.[46]
Moreover, Liet al.[47]summarized the numerical solutions for the fractional ordinary differential equation. As a result,for a given definition,the fractional-order chaotic systems can be solved by different approaches. At present, frequency method,prediction-correction algorithm and Adomian decomposition are the most commonly-used methods to solve the fractional-order chaotic systems. Since fractional-order calculus and numerical solution algorithms are the key to the applications of fractional-order nonlinear systems,it is necessary to summarize existing research and point out future directions.
There are not many review papers regarding fractionalorder chaotic systems. We just found three papers which reviewed the work of fractional-order through different aspects. Tavazoei[48]indicated the history, achievements, applications, and future challenges of fractional-order chaotic systems. Radwanet al.[49]made a comparison with different chaotic systems as well as with their fractional order counterparts. Tariqet al.[50]reviewed the synchronization of fractional-order chaotic system with sliding mode controller.However, it is necessary to sort out the problems existing in the research,which are listed as below:
1) How to select a proper fractional-order derivative for the chaotic systems?
2) How to select a proper solution algorithm for a given fractional-order chaotic system?
3) How is dynamics of the fractional-order chaotic systems determined by the memory effect?
The memory effect of the fractional-order chaotic systems is affected by two aspects. One is the fractional-order derivative itself, the other is the used numerical solutions. Thus, in this paper,we will review definitions and the most used solution algorithms for the fractional-order chaotic systems, and will focus on the memory effect of the two aspects. Moreover,due to the importance of the memory effect, applications of the fractional-order chaos in real applications are discussed.
The rest of this paper is organized as follows. In Section 2, definitions of fractional-order calculus are presented.In Section 3, the most commonly used numerical solution algorithms for fractional-order chaotic systems are discussed,and a comparison is carried out. In Section 4, memory effect in the fractional-order calculus,memory effect of different solution algorithms and short memory effect are discussed. In Section 5,based on the memory effect of fractional-order calculus, several aspects of applications are mentioned. Finally,we summarize the results and present a clear direction for future research.
2. Preliminaries
In this section, definitions of different fractional derivatives for fractional-order nonlinear systems are presented,and four definitions of fractional derivatives for the continuous chaotic systems are considered.
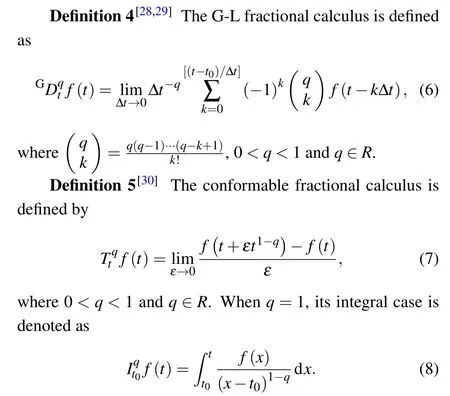
Definition 1[28,29]Caputo fractional derivative definition is given by

where 0<q <1,q ∈R.
As mentioned in the abstract, until now, there is no uniform definition for the fractional calculus. Except the above mentioned definition,there are also many other definitions.
Ortigueira and Machado[51]discussed the issue on what is a fractional-order derivative. It indicates that an operator called fractional-order derivative should satisfy the wide sense criterion including linearity,identity,backward compatibility,the index law holdingDq1+q2f(t)=Dq1Dq2f(t)and the generalized Leibniz rule. Although there is no uniform definition for the fractional-order calculus, Fernandez and Baleanu[52]indicated that some operators can be rewritten as finite or infinite sums of Riemann–Liouville differintegrals,which make it possible to build a uniform definition for fractional-order calculus.
3. Numerical methods for the fractional-order chaotic systems
To employ the fractional-order calculus in the real applications,it is key to find a proper solution method for the built fractional-order model.[48,53]Due to this non-idea memory effect,it is still a challenge to solve fractional-order differential equation efficiently. At present, there are many different approaches used to solve the fractional-order chaotic systems.Without loss of generality, we take the fractional-order simplified Lorenz system[54]as an example to show the analysis results of different numerical methods, and the system is defined by
whereDqt0is the fractional-order derivative. The integer-order counterpart is proposed by Sun and Sprott,[55]which is a Lorenz system with only one parameter. Later,analog circuit implementation,[56]DSP digital circuit implementation,[57]chaotification[58]of this system are investigated.
According to Zhao,[59]if a functionf(t)is absolutely integrable in (0,T) and satisfies the initial condition, the equationDqt y(t)=f(t)has a unique solution in this interval. Thus if the fractional-order chaotic system satisfies this condition,it has a unique solution. For instance, the simplified Lorenz system should have an unique solution. Moreover,since there are many different approaches for fractional-order chaotic systems,solutions obtained based on those numerical methods are approximations of the unique solution. Finally, the presented example is the simplified Lorenz system, which is a typical chaotic system. However, when the system has constant or external disturbance, the system can be solved using the the following methods,but there could be some processing skills.For instance, when the system have trigonometric perturbation, like, cos(ωt), the system can be revised by adding one more dimension to become an autonomous differential equation.
3.1. Frequency domain method
The principle of frequency domain (FD) approximation algorithm is designed according to the Laplace transform of the fractional-order calculus. It transfers the fractional-order system from time domain to frequency domain. As a result,we can use mature theories for the integer-order system to approximate the fractional-order system.[56]If the initial conditions of the function is zero,the Laplace transform of the fractional operator can be expressed by the transfer function in the frequency domain as[10]

here,pt= 0.01, 1/pTis the relaxation time constant of a single-order fractional system.qis the fractional power factor of a single fractional order system. Considering the actual situation,the working frequency band of the dynamic system is usually limited. So line segments of 0 dB and-20 dB are used to approximate in the calculation. And Eq. (12) can be approximated asN-1s


The approximate solution of a fractional-order system can be obtained by the above method. Based on the frequencydomain approximation method of the Bode diagram,Ahmadet al.[60]calculated and obtained the approximate expressions of the transfer function in the frequency domain for error=2 dB and error=3 dB,q ∈[0.1,0.9],and the step size of derivative orderqis 0.1. Later,Minet al.[61]calculated the approximate solutions,where error=1 dB,q ∈[0.8,1)and the step size of derivative orderqis 0.01. It can be found that,for differentq,when solving fractional-order chaotic system,it is necessary to obtain the integer order approximation of the fractional-order operator respectively,and the approximate process is complicated and inflexible.

Fig.2. Numerical model of fractional-order simplified Lorenz system using Simulink.

Using Matlab/Simulink module “State-Space”, the model for fractional-order simplified Lorenz system is built as presented in Fig.2. The initial conditions can be set in the“State-Space”modules. Thus the system is solved. Moreover, the transfer function provides a reference for analog circuit implementation of fractional-order chaotic systems. Firstly, the analog circuit implementation of fractional-order simplified Lorenz system is reported in Ref.[56]. Secondly,there are also many other researchers realized fractional-order chaotic systems using analog circuits,such as Jia,[62]Lu,[63]Kingni,[64]Teng.[65]
3.2. Predictor-corrector algorithm
Predictor-corrector algorithm is also called Adams–Bashforth–Moulton method, and it is widely used to solve the fractional-order nonlinear systems. The algorithm is proposed by Diethelm[41,66]and Garrappa.[67]Supposed that the fractional-order nonlinear system is defined as

According to Diethelm and Ford,[68]system(20)is equivalent to the Volterra integral equation


Applying the above solution algorithm to the fractionalorder simplified Lorenz system,we have Thus, the numerical solution of the proposed fractional-order system is obtained. At present, Roberto Garrappa[69]from University of Bari developed the algorithm. The function is given by [t,y]=fde12(alpha,fdefun,...), which is easy to call and can be used to solve different fractional-order differential equations.
In fact, predictor-corrector algorithm is the most widely used method to solve the fractional-order nonlinear systems.According to different application situations, this method is modified. Bhalekaret al.[70]employed the predictor-corrector algorithm to solve the time delayed fractional chaotic system.Manoj and Varsha[71]proposed a new predictor-corrector method to solve the fractional-order chaotic systems which has much less estimating time comparing with the classical ones. Deng[72]considered the short memory principle of fractional differential equations based on the Adams-type predictor-corrector approach. Mekkaouiet al.[73]extended the predictor-corrector method to solve nonlinear fractalfractional differential equations. Shahbaziet al.[74]analyzed the error and stability of the predictor-corrector method for nonlinear systems. Fractional dynamics in practical application models such as the HIV model,[75]COVID-19 epidemic model,[76]magnetorheological suspension system[77]and centrifugal flywheel governor system[78]are analyzed based on the predictor-corrector method.
3.3. Semi-analytic algorithms
The analytic algorithms are designed to obtain exact solution of fractional-order differential equations. Adomian decomposition algorithm,[37]homotopy perturbation algorithm,[38]differential transformation algorithm[39]and variational iteration algorithm[40]are widely used analytic algorithms.To simulate the fractional-order chaotic systems,researchers divide the time into sub-intervals and semi-analytic algorithms or multi-step algorithms are designed.
3.3.1. Adomian decomposition method
For a given fractional-order chaotic system with the form of

wherex(t) = [x(t),y(t),z(t)]Tare the state variable. Here,m=ceil(q),bkis a specified constant relating to the initial values,andLx(t)andNx(t)are the linear and nonlinear terms of the fractional differential equations,respectively. By applying the R-L fractional integral operator to both sides of Eq. (29),the following equation is obtained:[79]

Because ADM converges fast,the first 6 terms are used to get a satisfying solution of system(29). In the real cases,it is impossible to estimate the high accuracy value ofxwhenttakes large value. So,it is necessary to design a time discretization method. That is to say,for a time interval[t0,T],we divide the interval into subintervals [tn,tn+1]. Then we get the value ofx(tn+1)based onx(tn)by applying functionF(·). Finally,the numerical solution of the fractional-order chaotic system are denoted as a discrete mapx(n+1)=F(x(n)).


More itemsCi,j(i=1,2,3 andj=3,4,5,6) can be found in Refs. [57,82]. For given initial conditions [x(t0),y(t0),z(t0)],we can get time series of the system.
In fact,it is Cafagnaet al.who introduced ADM to solve the fractional-order chaotic Chen system[83]and fractionalorder R¨ossler system[84]for the first time, in 2008 and 2009,respectively. Then, Heet al.[82]solved the fractional-order simplified Lorenz and analyzed dynamics of the system used the Lyapunov exponents in 2014 and Wanget al.[57]realized the system using DSP digital circuit in 2015. At present,ADM has been employed to solve fractional-order chaotic systems for applications,like fractional-order chaotic systems with line equilibrium,[85]fractional-order Lorenz system and fractional-order L¨u system,[86]variational iteration method based on Adomian polynomials,[87]fractional-order system with delay,[88]fractional smoking habit model.[89]
3.3.2. Homotopy analysis method

Suppose that we have a fractional-order dynamical system,and it is denoted as respectively. Thus, as long aspincreases from 0 to 1, the solutionsφ(x,t;p)changes from the initial valuex(tn)to the solutionx(t). If we expandφ(x,t;p) to Taylor series with respect to the embedding parameterp,we obtain

Differentiating Eq.(41)m-times with respect top,then arrangingp=0 and dividing bym!,we obtain themthlinear equation as[90,91]


wherex=[x,y,z]T. Thus, we get the solution of fractionalorder simplified Lorenz system using the homotopy analysis method.
However, there are not many works regarding the analysis of fractional-order chaotic systems based on the homotopy analysis method. Here, four papers are presented. Yuet al.[92]firstly designed a multistage homotopy-perturbation method to solve a classes of fractional-order hyperchaotic systems. Alomariet al.[93,94]designed a step homotopy analysis method and fractional-order Lorenz system and fractionalorder Chen system were analyzed.Veereshaet al.[95]proposed aq-homotopy analysis transform method to solve nonlinear models and chaos was observed.
3.3.3. Differential transform method
Differential transform method is one of the most effective methods for semi-analytic analysis of differential equations.[96–98]Here, the fractional differential transform method is introduced to solve the fractional-order simplified Lorenz system.
Firstly, the basic principles of differential transform method are presented. The analytical functionf(t) in terms of a fractional power series is denoted as follows:
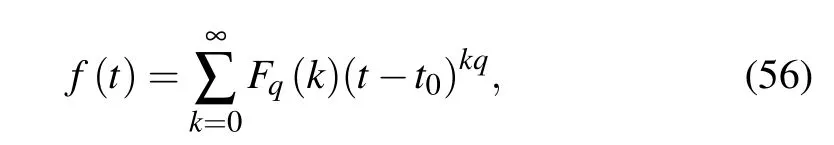

Currently, differential transform method has been employed to solve the fractional-order chaotic systems,but there are not many reports. Alomari[99]introduced differential transform method to obtained the analytic solution of fractional chaotic dynamical systems. Freihatet al.[100]obtained numeric-analytic solution of fractional-order R¨ossler chaotic and hyperchaotic systems.Momaniet al.[101]analyzed fractional-order multiple chaotic FitzHugh–Nagumo neurons.Mohammedet al.[102]solved the fractional-order L¨u chaotic and hyperchaotic system using the multistep generalized differential transform method.
3.3.4. Relationship between different algorithms
The analytical methods are designed to obtain exact solutions of fractional-order differential equations. Thus there should be relationship between the three algorithms.
Suppose that we have a system which is given by

where

Obviously, differential transform method solution and Adomian decomposition method solution are the same for the given system, and the relationship betweenKkandckis described as

Meanwhile, according to Ref. [103], the homotopy analysis method solution is the same as the Adomian decomposition method solution when ¯h=-1. Thus, it shows that the solutions of fractional-order chaotic systems by those three methods are the same. It shows that different approaches yield the same answer.
3.4. Sayed’s discretization method
Firstly, discretize the simplified Lorenz system with piecewise constant arguments as[104]

with the initial conditionsx(t0)=x0,y(t0)=y0andz(t0)=z0.Secondly, we divide the time astn=nh,n=0,1,2,...,N.Steps of the discretization process for time delay systems are given as follows.[104,105]

fort=tn+1. Whenq=1, the integer-order system has the Euler solution. Moreover, it can be treated as semi-analytic algorithm solutions with one item. Obviously,it has lower accurate compared with the three multi-step algorithms. Due to its simpleness, Sayed’s discretization method has been widely used to solve fractional-order chaotic systems in real applications.[106–109]
3.5. Solution for G-L fractional-order systems
In order to limit the memory for binomial coefficients,short memory principle is used, and the G-L derivative order is denoted as[110,111]

To numerically simulate the G-L fractional-order chaotic system,the following coefficients are employed:

whereNis the memory length.
3.6. Solutions for conformable fractional-order systems
Research of conformable fractional-order chaotic systems is a recent year hot topic. Those systems including conformable fractional-order simplified Lorenz system,[112]conformable fractional-order 4D fractional-order chaotic system,[113]conformable fractional-order tumor model,[114]conformable fractional-order two-machine interconnected power system,[115]have rich dynamics. Meanwhile, sliding mode control technique[116]and exponential quasisynchronization[117]are investigated. There are several numerical solution algorithms[112,118–120]for conformable fractional-order chaotic systems proposed.

3.6.1. Multi-step solutionsAs a result, the dynamic of the system will be static witht →∞.
According to the above method, the conformable fractional-order simplified Lorenz system is converted to[121]
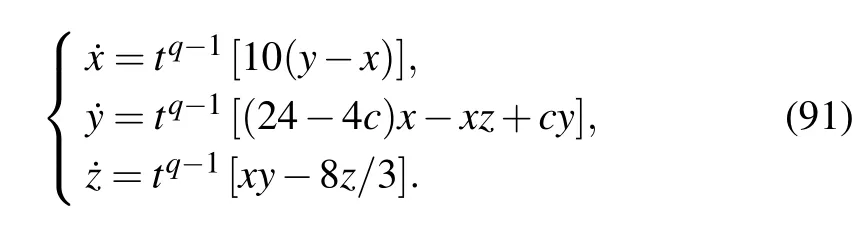
Until now, the conformable system is converted to a nonautonomous integer-order chaotic system with time-varying parameters. There are many methods for the integer-order differential equation such as Runge–Kutta algorithm and Euler algorithm. Sinceq ∈(0,1],whent →∞,the right side of the equations will become zero. Based on this scheme, the system will become stable and chaos will disappear finally. Obviously,the system has two different solutions with two different methods.
3.7. Other solution algorithms
At present, there are many different methods designed to fractional-order differential equations, such as Laplace decomposition method,[122]shifted Legendre polynomials,[123]integral transforms,[124]Legendre wavelets approach,[125]and intelligence algorithm.[126]Among these, the intelligence algorithm has potential application value due to the fast development of artificial intelligence science. For a given fractionalorder chaotic system, there are many different approaches to solve it. So it is important to indicate which method is used and what are the simulation conditions in the analysis. However,it is still a challenging research point to design an effective method to analyze the fractional-order nonlinear chaotic systems.
3.8. Characteristics of different algorithms
Firstly, we summarize the characteristics of different algorithms mentioned above and the results are presented in Table 1.Three categories including frequency method,predictorcorrector algorithm and multi-step methods are considered,where Adomian decomposition method, homotopy analysis method,differential transform method and Sayed’s discretization method belong to the multi-step method category. It shows that the predictor-corrector algorithm costs the most computational resources. Frequency method can be used to build simulation model and design analog circuit of fractionalorder chaotic systems,and multi-step methods can be used to realize the fractional-order chaotic systems in the digital circuits including digital signal processor (DSP) and field programmable gate array (FPGA), while predictor-corrector algorithm is used to do the numerical simulations only.
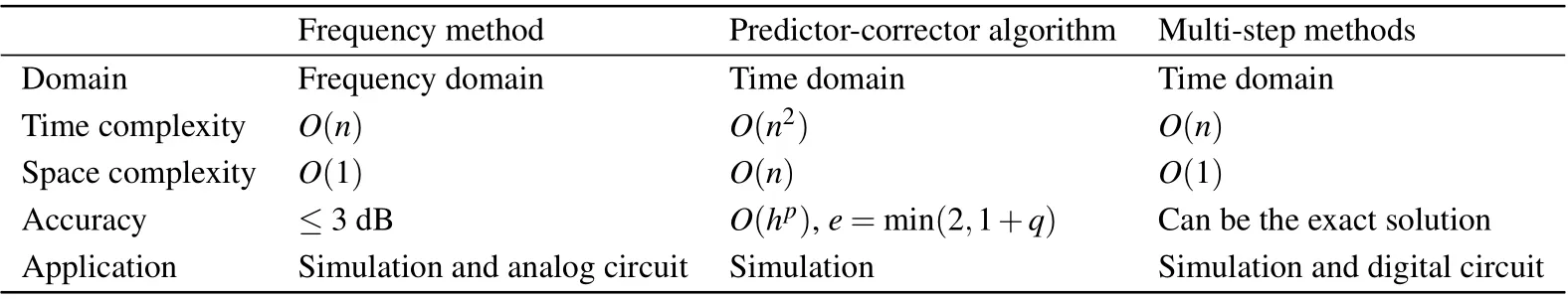
Table 1. Comparison of three kinds algorithms for fractional-order chaotic systems.
On the one hand, the semi-analytic solution is exact approach for the nonlinear system when takes infinite items. In the real applications, finite items can deduce higher accuracy solutions. On the other hand, there are no exact solutions for the chaotic systems.[127]Thus it is impossible to estimate the value of the chaotic systems for a long time. In the real applications, the multi-step methods are introduced to solve the chaotic systems. Generally, we divide the time interval[0,T]into small subintervals[tn,tn+1]wheren=0,1,2,...,N.Thus we only need to focus on the accuracy of the solution in the subintervals. However, the accuracy presented in Table 1 for frequency method and predictor-corrector algorithm is the global error.
Letc=5, time step sizeh=0.01, derivative orderq=0.98,initial conditions be[x0,y0,z0]=[1,1,1]andN=20000,phase diagrams of the fractional-order simplified Lorenz system with different solutions are presented in Table 2. It shows that the butterfly chaotic phases are observed in most cases except the case when the system is solved by Sayed’s method,and when it is solved by the direct method with conformable derivative,chaos will disappear with time.

Table.2 Phase diagrams of the simplified Lorenz with different solution algorithm.

Table 2. Phase diagrams of the simplified Lorenz with different solution algorithm(continue).
4. Memory effect in the numerical approaches
4.1. The memory effect with kernel function
Here,we present an example to show that the fractionalorder calculus provides a natural way for modeling of real systems, and memory effect should be considered. Ahmed and Elgazzar[128]investigated a fractional order differential equations model for nonlocal epidemics. And the results are listed as follow. Firstly,the system is defined as


Letq=1,0.7,0.5 and 0.3,the curves of the kernel function are illustrated in Fig.3. It shows memory effort is different with different derivative orderq. Whenq=1,the memory strength keeps same over time and the value is one. Ifq <1, the memory strength decreases with time and largerqdecreases slower.Generally,researchers emphasize the memory effect in fractional calculus. However, both integer-order calculus and fractional-order calculus have memory effect. Since the memory strength does not change with time, we hold the opinion that integer-order calculus has“ideal memory effect”. Meanwhile,when the derivative order is not integer,the system has“nonideal memory effect”.

Fig.3. Memory effect with different order q.
4.2. Memory effects in the variable-order calculus
For variable-order fractional-order calculus, time is used as the independent variable of most existing variable-order functions.[129]Variable-order fractional-order can express the physical properties and memory characteristics of materials in a certain period of time by changing different orders.[130]Obviously,the memory of the variable-order fractional calculus changes with time. More details regarding variable order fractional calculus can be found,and it has been employed to model complex real-world problems including transport processes,control theory and biology.[130,131]A general definition for variable-order calculus is presented in Definition 6.
Definition 6 The variable fractional-order calculus can be derived from the existing definitions,but the derivative orderqis a function of timet.
For instance, the variable-order fractional Caputo calculus is defined by

Letq(t) be different functions including constant, sinusoidal function, increasing function and decreasing function. The curves for the kernel function are presented in Fig. 4. Compared withq(t)=0.5, the variable fractional order calculus can accelerate or retard the memory through the kernel function. Whenq(t)=0.5-0.05t, the tail of kernel function is longer,which means that the system has a stronger memory for the state further away from the current moment. Whenq(t)=0.5+0.05t,the tail of kernel function is shorter,which means that the system has a weaker memory for the state further away from the current moment. Whenq(t)=0.5±0.05sin(2πt),the curves fluctuate around the curve withq(t)=0.5, thus it has wave memory effect.

Fig.4. Two different approaches for numerical solutions of fractionalorder chaotic systems.
Three different situations aboutq(t)are considered.
(1) Constant order. Most research regarding fractionalorder calculus is carried out based on a fixed order,i.e.,q(t)=c. Since the evolution of the system does not affect the order,it is called constant fractional order.
(2)Piecewise fractional order. The case where the order remains the same for a period of time is called piecewise fractional order.[132]q(t)is a piecewise constant function, before and after the piecewise points,the functions have different values,and the expression forq(t)is
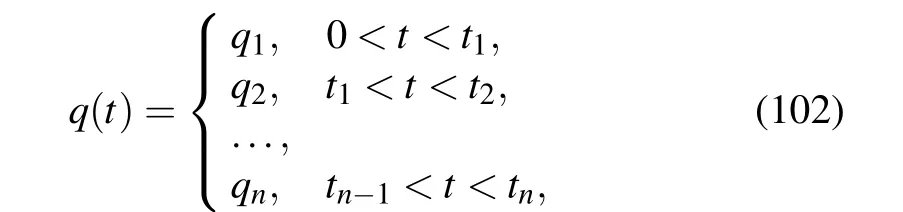
whereq1,q2,...,qn ∈(0,1]are constants.
(3)Transient fractional order. The time-varying variableorder functionq(t) corresponds to different orders over time.Compared with the piecewise fractional order, transient fractional order can describe the change process of physical quantities with time more accurately. The difficulty lies in choosing a appropriateq(t) according to the characteristics of the physical quantity,which requires a combination of fitting and estimation algorithms.
The selection of the specific order can be achieved by fitting, e.g., Kang[133]used the quantum particle swarm optimization algorithm to solve the order of fractional derivative and accumulation, and Gao[134]manually selects the optimal order in different time periods by comparison. In addition,the variable-order fractional-order model can also be used as a chaotification method,[132]The variable-order increases the nonlinearity of the system. From the perspective of chaotification, except for 0<q <1, the form ofq(t) is free. And the expression of the variable-order function can be a power function,exponential function,trigonometric function or other types of functions.[135]Finally, to obtain numerical solution of transient fractional order, it can also be defined based on the piecewise constant function. Let us take the variable-order fractional Caputo calculus as a example,the discrete variableorder fractional Caputo derivative is given in Definition 7.
Definition 7[135]The discrete variable-order fractional Caputo derivative is defined as

wheretn-tn-1=h(n=0,1,2,...)is the width of time interval,andq(t)∈(0,1]can be a continues function.
4.3. Memory loss effect
Suppose that there is a integer-order chaotic system,which is defined by



we call the solution as“non-local solution”. It means that the solution depends on all the history data. Figure 5(a) is the schematic diagram of this case. For instance, the predictorcorrector algorithm belongs to this kind of method. If the solution of a fractional-order system is denoted as

then we call the solution as “local solution”. It means that the solution focuses on the time interval[tn,tn+1]. Figure 5(b)is the schematic diagram of this case. If we focus on a short time interval, the “local solution” can better approximate result. If in the long run,the“non-local solution”shows a global memory effect. As for the memory effect of this two kinds of methods,the“non-local solution”related to all the history data directly, while the “local solution” related to the history step by step. But it should be indicated out that “local solution”losses some memory according to Eq. (109), which is given by
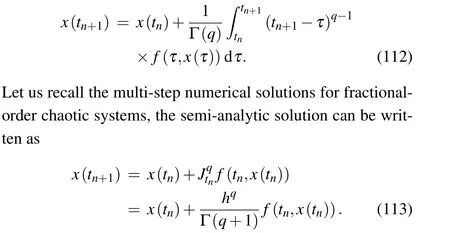
Obviously,this is the Sayed’s discretization solution,[104]and Eq. (113) is the solution of Eq. (112). Thus it verifies that there is a memory loss effect in the Sayed’s discretization solution. As a matter of fact,the loss of memory is found when the fractional-order is simulated using the“local solution”.
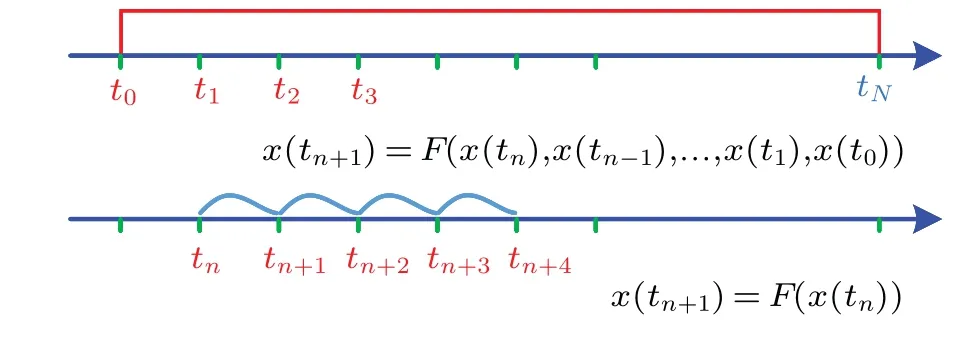
Fig.5. Two different approaches for numerical solutions of fractionalorder chaotic systems.
4.4. Short time memory effect
Since“local solution”methods solve the fractional-order chaotic systems in the subintervals and loss some memory,there is no rush to discuss the short time memory effect for this kind methods. Here we focus on the“nonlocal solution”methods which shown in Fig.5(a). Those“nonlocal solution”methods,for example,the predictor-corrector algorithm,need all history data for each step of calculation,thus,for each step,computing time increases with step numbernasO(n2) and storage space needed increases with step numbernasO(n).So it is difficult to employ this kind of methods in engineering applications.
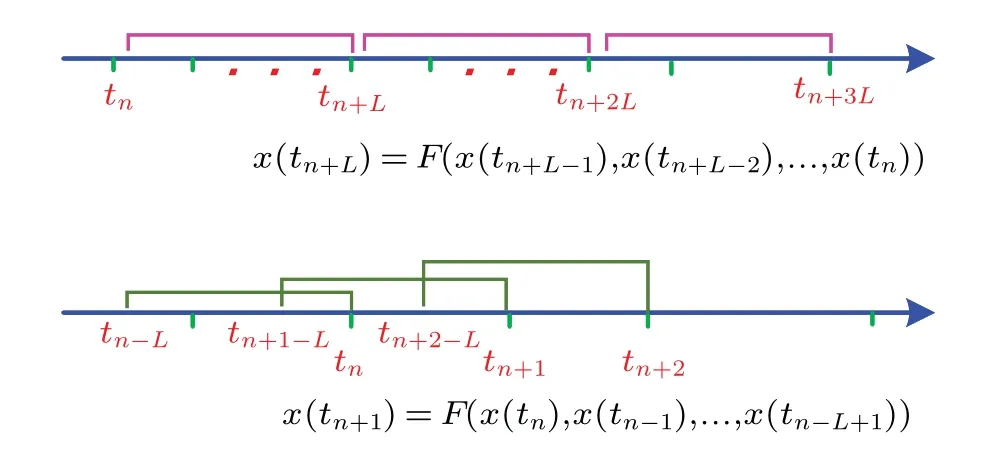
Fig. 6. Two schemes for short time memory effect of fractional-order systems.
To reduce the amount of computation, short memory effect is considered in the real applications. In Refs.[136,137],short memory effect for the fractional-order discrete chaotic maps is investigated.Usually,the G-L fractional-order chaotic systems are solved with short time memory effect.[138,139]Naturally,there exists two ways to handle the short time memory effect,[140]and the schematic diagram is shown in Fig.6. Figure 6(a)shows that time is divided into many slices with same length,the fractional-order system is solved with full memory effect in these time slices. Since the length of time slices is limited, thus the fractional-order system can be solved faster.For instance, to estimate Lyapunov exponents of fractionalorder chaotic systems, this kind short time memory effect is considered.[141]Figure 6(b) illustrates that there are limited number of data points are used to estimate the current state.This method can be picturesquely described as the sliding window method. Obviously,it losses memory out of the window,and will generate much errors.However,due to its stable solving speed, this method are employed to solve the fractionalorder chaotic systems.[138,139,142]
5. Memory effects in the applications
5.1. Lyapunov exponents
The“local solution”of fractional-order chaotic system is given by


wherek=1,2,...,d. For instance,dynamics of the system is investigated using Lyapunov esponents in Refs.[57,82].wheredis the dimension of the system. The resulting LEs measure the rate of growth of infinitesimald-dimensional volumes. The Matlab code of this method is reported in Ref.[141].

Fig. 7. Dynamics of the fractional-order simplified Lorenz system with short memory effect. (a)Bifurcation diagram and Lyapunovs;(b)q=0.92 and L=1;(c)q=0.92 and L=2.
Leth=0.01,t0=0,tfinal=100,y0=[1,1,1]T,memory lengthL=2,and the derivative orderqincrease from 0.9 to 1 with step size of 4×10-4. Dynamics of the fractional-order simplified Lorenz system versus derivative orderqis analyzed as shown in Fig. 7(a). It shows that bifurcation diagram and Lyapunov exponents do not match well nearq=0.92.According to the bifurcation diagram,the system is not chaotic whenq <0.93,but it shows that the system is chaotic according to the Lyapunov exponents. As shown in Fig.7(c),the system is convergent withL=2,but it shows in Fig.7(b)the system is chaotic withL=1. It should be noted that, measuring result itself is correct and chaos is indeed generated.Since Lyapunov exponents do not agree with its corresponding bifurcation diagram and short memory effect could change the dynamics of fractional-order chaotic system, the measuring results should be verified by other methods such as phase diagram and bifurcation diagram. However,the method proposed in Ref.[141]provides an effective tool for diagnosing chaos of fractionalorder chaotic systems when solved by the predictor-corrector algorithm.
5.2. Physical implementation
Physical implementation of fractional-order chaotic systems is an important way for real applications.[110]There are two approaches which are the analog circuit implementation and the digital circuit implementation used to realize the fractional-order chaotic systems.[145]
Firstly, analog circuit implementation can generate real chaos due to nature of the electron components. The key is to design the fractional-order integrating circuit which has fractional memory effect. Figure 8 shows two different ways including chain structure[146]and tree (fractal) structure[147]to design fractional-order capacitance for the integrating circuit.Meanwhile,fractance which holds memory effect is employed to design fractional-order systems where ladder circuit for the fractional-order memory effect is introduced.[148]Analysis and circuit implementation of fractional-order chaotic systems are the currently research topic.[149–152]However, since the frequency-domain solution is an approximation of the original fractional-order, unreliability of this method in recognizing chaos in fractional-order systems is discussed.[153]

Fig.8. Analog circuit for the fractional-order calculus. (a)Chain structure;(b)tree(fractal)structure.
Secondly,the digital circuit implementation of fractionalorder chaotic systems is mainly carried out with the“local solution”. For instance, Wanget al.[57]realized the fractionalorder simplified Lorenz system based on the Adomian decomposition method using digital signal processor (DSP).Meanwhile, DSP implementation of fractional-order chaotic system with hidden attractor[154]with infinitely many coexisting attractors,[155,156]with nonhyperbolic equilibrium[157]are carried out. FPGA implementation of fractional-order chaotic systems provides another way which is far more effective due to the parallel arithmetic, such as fractional-order financial chaotic system,[158]fractional-order neuron,[159]fractional-order chaotic oscillators,[138]variable-order fractional chaotic system,[160]fractional-order chaotic sound encryption system.[161]It should be noted that the length of the digital word can degrade the desired response due to the finite number of bits to perform computer arithmetic.[150]However, digital circuit implementation has the advantage including repeatability, implementing simple, high level of integration,good stability and high reliability.
5.3. Fractional-order memristor
As mentioned, the integer-order calculus has the ideal memory effect, thus memristors modeling with integer-order derivative have idea memory effect. However, it is nature to have the non-idea memory effect in the real electron components.[162]So modeling and application of memristors using fractional calculus becomes more and more important.Coopmanset al.[163,164]did the pioneering work for designing fractional-order memristor. Later different kinds of fractionalorder memristor are reported.[165–170]At present, memristor modeling with fractional-order difference becomes a new hot topic.[171,172]Here,we use an example to show how the fractional derivative order affect the dynamics of the memristor.The memristor system is denoted as
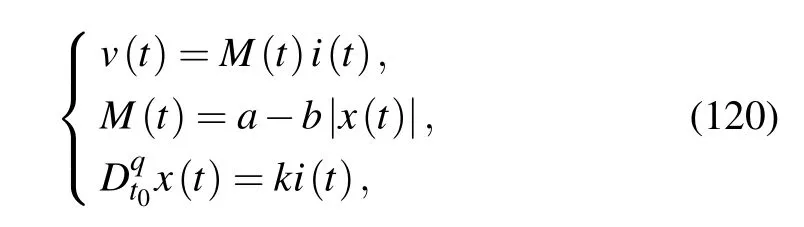
whereqis the derivative order,k=1,a=1 andb=0.1. LetA= 4,ω= 0.1, andI=Asin(2πωt), hysteresis curves of the fractional-order memristor with different orderqare presented in Fig. 9. The fractional-order equation is solved by the predictor-corrector algorithm with step size ofh=0.01. It shows that the area in the hysteresis loops decrease with the decrease of derivative order. Since the fractional-order calculus has non-idea memory effect,dynamics of the memristor is changed with shape and area of the hysteresis loops.
In 2010,Petras[173]designed fractional-order memristorbased Chua’s circuit and analyzed dynamics of the system. Later, several simplest fractional-order memristorbased chaotic systems are investigated by Cafagna,[174]Leng[65]and Ruan.[175]Meanwhile, there are many different kinds of fractional-order memristor chaotic systems designed, such as fractional-order delayed memristor chaotic system,[176]FPGA implementation of fractional-order memristor chaotic oscillator,[177]fractional-order memristor with smooth quadratic nonlinearity[178]and wien bridge oscillator with fractional-order memristor.[179]

Fig.9. Hysteresis curve of the fractional-order memristor with different order.
5.4. Fractional-order chaotic neural networks
Based on the neural system proposed by Maet al.[180]a fractional-order chaotic neural network is given by Fig.10,where the network structure is reported by Yanet al.[181]The neural model is given in the blue part of Fig.10(a),while the structure of the network is decided by the red part. Obviously,this is a coupled network.

Fig.10. A possible fractional-order chaotic neural network. (a)Network model;(b)chaotic attractor;[182] (c)structure of network.
In fact, chaotic dynamics has been found and investigated in neural systems and neural networks during the last few decades.[183–185]Moreover,by introducing the fractionalorder calculus to the neural networks, chaotic dynamics in the fractional-order chaotic neural networks have been intensively investigated.[186–188]It shows that the fractional-order derivative order can affect the dynamics of the neural network since dynamics of the single is affected by the derivative order. Moreover, the fractional-order bidirectional associative memory[189]in the neural networks is a recent hot topic. However, synchronization and control of fractionalorder chaotic neural networks are widely investigated[190–192]where the coupling controller is most used method and different spatiotemporal patterns can be observed with different coupling methods.[182]Chimera states which are related to the actual neuronal activities are found in such as fractional-order chaotic neural networks.[182,193]However, to our knowledge,this topic is still in the early stages of research.
6. Discussion and conclusion
This review paper provided a comprehensive overview of fractional-order nonlinear chaotic systems from a memory effect perspective. As a result, memory effect to the dynamics of the fractional-order nonlinear chaotic systems is discussed based on the fractional-order calculus and numerical solution algorithms.
It shows that the kernel function withq= 1 is always one over time, while the kernel function decreases with time whenq <1. Thus the integer-order system has full memory effect, while the fractional-order system has nonideal memory effect. Whenqis a function of time, the memory effect changes with time. Thus the fractional-order chaotic systems have rich dynamics. However,since there are many different calculus, what is the physics significance to introduce fractional-order derivatives to those systems becomes a crucial issue. How memory effect in different calculus and numerical algorithms affects the dynamics of fractional-order chaotic systems deserves further investigations. Meanwhile, stability of fractional-order nonlinear systems[194]related to the controller design and dynamics is still a challenge to investigate.
Numerical solution algorithms, including frequency method, predictor-corrector algorithm, semi-analytic algorithms, Sayed’s discretization method, G-L fractional-order solution, and conformable fractional-order solution of simplified Lorenz system are presented and chaotic attractors with same parameters but different approaches are summarized. According to how the fractional-order chaotic is solved,namely, solved over a long period of time or solved in a subinterval,solution algorithms are divided into two types. They are “local solution” algorithms and “nonlocal solution” algorithms. Different kinds of methods can be used for different applications of fractional-order chaotic systems. It is found that there are memory losses when the system is solved by“local solution”algorithms or when the system has short term memory. For a given fractional-order system and its obtained results,the simulation conditions including definition of fractional calculus, solution method, time step, system parameters and initial condition should be illustrated clearly. Until now,there are many different other kinds of methods proposed to solve the fractional-order equations. It will be interesting to investigate how to introduce those methods to solve the fractional-order chaotic systems.For instance,the intelligence algorithm[126,195]has been designed to solve the fractionalorder systems. How to establish a relationship between the artificial intelligence and fractional-order chaotic systems will be helpful to build better numerical models of fractional-order nonlinear chaotic systems.
At present, fractional-order chaotic systems have been employed to model physics process and used in the engineering application field, such as circuit implementation,fractional-order memristor chaotic systems, and fractionalorder neural networks. Although there are some progresses,the further researches are still needed including more effective solution algorithms,new dynamical behaviors,new fractionalorder chaotic systems,new fractional-order memristor chaotic systems and networks.
Acknowledgements
Project supported by the Natural Science Foundation of China (Grant Nos. 61901530, 62071496, and 62061008) and the Natural Science Foundation of Hunan Province, China(Grant No.2020JJ5767).
杂志排行
Chinese Physics B的其它文章
- Ergodic stationary distribution of a stochastic rumor propagation model with general incidence function
- Most probable transition paths in eutrophicated lake ecosystem under Gaussian white noise and periodic force
- Local sum uncertainty relations for angular momentum operators of bipartite permutation symmetric systems
- Quantum algorithm for neighborhood preserving embedding
- Vortex chains induced by anisotropic spin–orbit coupling and magnetic field in spin-2 Bose–Einstein condensates
- Short-wave infrared continuous-variable quantum key distribution over satellite-to-submarine channels
