Dynamic Feedback Stabilization for Timoshenko Beam with Locally Input Controls and Input Distributed Delay
2021-10-20WANGXiaorui王小瑞LIUXilan刘喜兰
WANG Xiaorui(王小瑞),LIU Xilan(刘喜兰)
( 1.School of Mathematics and Statistics,Qinghai Nationalities University,Xining 810007,China;2.School of Mathematics and Information Science,Baoji University of Arts and Sciences,Baoji 721013,China)
Abstract:In this paper,based on the method of dynamic feedback controller treating the boundary control with delays,we consider the exponential stabilization for a Timoshenko beam with interior locally input delayed controls and input distributed delay control.We design a new dynamic feedback control law to stabilize exponentially this system,and show that the original system is stabilized exponentially.
Key words:Timoshenko beam;Input distributed delay;Dynamic feedback controller;Exponential stabilization
1.Introduction



2.Design of Dynamic Feedback Controller







3.Representation of the System(2.2)



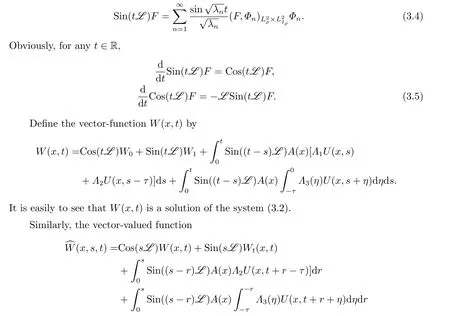
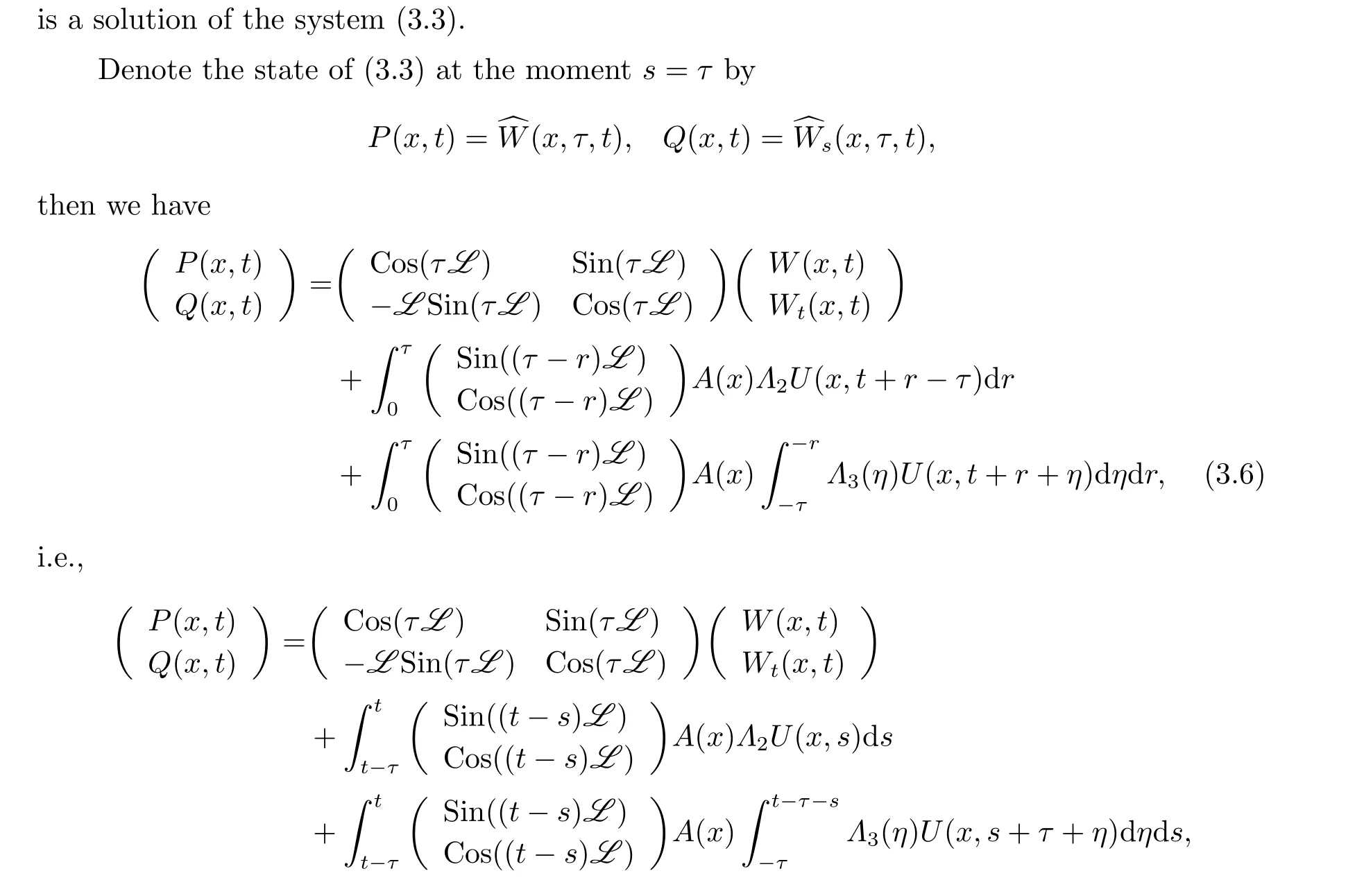



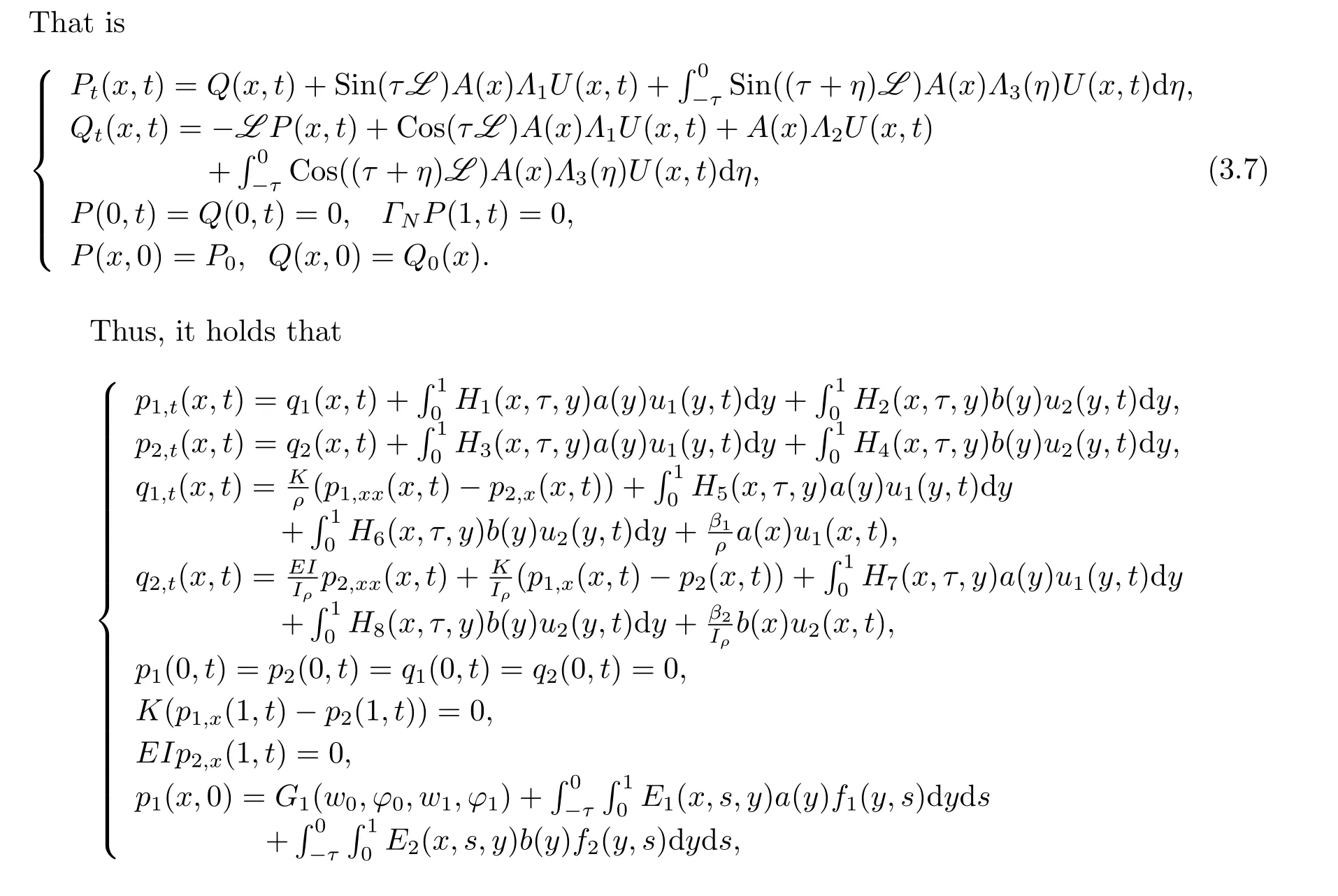



4.The Proof of Theorem 2.1


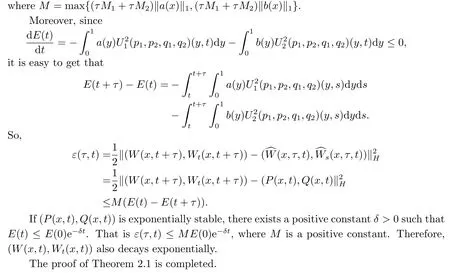
5.The Proof of Theorem 2.2
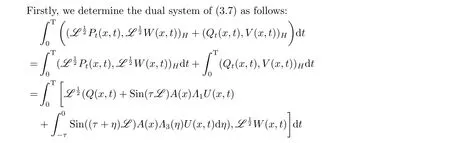







6.Conclusion
In this paper,we design a dynamic feedback controller to treat the stabilization of Timoshenko beam with locally input controls and input distributed delay.Under this control strategy,we prove that the closed-loop system(2.8)is exponentially stable for any time delayτ >0 by verifying the exponential stability of the system(2.7)on the some certain conditions.We mainly use the exact observability of the dual system in finite time to verify the exponential stabilization of closed-loop system.The approach proposed in this paper can be applied to more complicated models theoretically.
杂志排行
应用数学的其它文章
- Global Boundedness and Asymptotic Behavior in a Chemotaxis Model with Indirect Signal Absorption and Generalized Logistic Source
- Performances of Preliminary Test Estimator for Error Variance Under Pitman Nearness
- 一类梯度自然增长的拟线性椭圆型方程分布正解的存在性与多重性
- 一类由原根生成的伪随机子集
- 混合次分数布朗运动下永久美式回望期权的定价
- 双水平控制策略和延迟不中断单重休假的M/G/1排队系统分析
