Bimigrativity of Overlap and Grouping Functions

XIE Hai

(School of Science, Center for Data Analysis and Algorithm Technology,Guilin University of Technology， Guilin 541004, China)

Abstract：This paper focuses mainly on the bimigrativity between overlap functions, grouping functions and uninorms or nullnorms. First, we investigate the bimigrativity of overlap and grouping functions by using the notions of bimigrativity of aggregation functions. Second, we introduce the concepts and properties of bimigrativity of uninorms (resp. nullnorms) over overlap and grouping functions. Finally, we discuss the concepts and properties of bimigrativity of overlap (resp. grouping functions) over uninorms and nullnorms.

Key words：bimigrativity; overlap functions; grouping functions; uninorms; nullnorms

0 Introduction

The concepts of overlap functions and grouping functions were firstly introduced by Bustinceetal. In[1-2] and[3], respectively. Overlap functions and grouping functions are two particular cases of bivariate continuous aggregation functions[4-5]. Those two concepts have been applied to some interesting problems, such as image processing[6], classification[7-8]and decision making[9]. Some new interesting results about overlap and grouping functions were presented in[10]. Dimuro and Bedregal[11]presented the concept of Archimedean overlap functions and studied the cancellation, idempotency and limiting properties of Archimedean overlap functions. In[12], Gómezetal. introduced the definition ofn-dimensional overlap functions and the conditions under whichn-dimensional overlap functions are migrative, homogeneous or Lipschitz continuous. The concept of general overlap functions was introduced by De Miguel in[13], the difference betweenn-dimensional overlap functions and general overlap functionsis in the boundary conditions. Durante and Riccib[14]studied the supermigrativity of aggregation functions. Theα-migrativity of an aggregation function was introduced by Durante and Sarkoci[15].In[16], Bustinceetal. introduced a generalization of the concepts ofα-migrativity and migrativity.Lopez-Molinaetal.[17]introduced the notions of bimigrativity and total bimigrativity of an aggregationfunction w.r.t. another aggregation function, as a natural generalization of the notions of migrativity and bisymmetry. In[18], Qiao and Hu generalized theα-migrativity of any overlap functionOfrom the usual formulaO(αx,y)=O(x,αy) to the so-called (α,O*,O†)-migrativityO(O*(α,x),y)=O(x,O†(α,y)),whereO*andO†are two fixed overlap functions. Qiao and Hu[19]discussed the migrativity property of uninorms over overlap and grouping functions and the migrativity property of nullnorms over overlap and grouping functions. Zhu and Hu[20]investigated theα-migrativity of overlap functions and grouping functions over uninorms and nullnorms, and pointed out the similarities and differences between theα-migrativity of a uninorm over an overlap functionOas well as a grouping functionGand theα-migrativity of an overlap functionOas well as a grouping functionGover a uninormU.

Our study is mainly motivated by the bimigrativity of binary aggregation functions introduced by Lopez-Molinaetal.[17]. We firstly investigate the bimigrativity of overlap and grouping functions. Moreover, the migrativity of uninormsUover overlap functionsO(resp. grouping functionsG)U(O(α,x),y)=U(x,O(α,y)) (resp.U(G(α,x),y)=U(x,G(α,y)) is generalized to the bimigrativity of uninormsUover overlap functionsO(resp. grouping functionsG)U(O(x,α),O(β,y))=U(O(x,β),O(α,y)) (resp.U(G(x,α),G(β,y))=U(G(x,β),G(α,y)). Similarly, we study the bimigrativity of nullnorms over overlap and grouping functions, the bimigrativity of overlap functions over uninorms and nullnorms, and the bimigrativity of grouping functions over uninorms and nullnorms.

The rest of this paper is organized as follows. In Section 1, we present some basic definitions and vital properties on overlap functions,grouping functions, uninorms and nullnorms, the notions of bimigrativity and total bimigrativity of an aggregation function. In Section 2, the bimigrativity of overlap and grouping functions is discussed. In Section 3, we study the bimigrativity of uninorms over overlap and grouping functions. In Section 4, we investigate the bimigrativity of nullnorms over overlap and grouping functions. In Section 5, we briefly discuss the bigrativity of overlap and grouping functions over uninorms (resp. nullnorms). Finally, the main results are summarized.

1 Preliminaries

In this section, we recall some concepts and properties related to overlap functions, grouping functions,uninorms and nullnorms which shall be needed in the sequel.

Definition1 (See Bustinceetal.[1]) A bivariate functionO: [0,1]2→ [0,1] is said to be an overlap function if it satisfies the following conditions:

(O1)Ois commutative;

(O2)O(x,y)=0 ifxy=0;

(O3)O(x,y)=1 ifxy=1;

(O4)Ois increasing;

(O5)Ois continuous.

Example1 (See Qiao and Hu[18]) For anyp>0, consider the bivariate functionOp:[0,1]2→[0,1] given by

Op(x,y)=xpyp

for allx,y∈[0,1]. Then it is an overlap function and we call itp-product overlap function, here.

Definition2 (See Bustinceetal.[1]) A bivariate functionG: [0,1]2→ [0,1] is said to be a grouping function if it satisfies the following conditions:

(G1)Gis commutative;

(G2)G(x,y)=0 ifx=y=0;

(G3)G(x,y)=1 ifx=1 ory=1;

(G4)Gis increasing;

(G5)Gis continuous.

Definition3 (See Bustinceetal.[16]) Consider an aggregation functionB. An aggregation functionAis called:

(i)α-B-migrative, withα∈[0,1], if the identity

A(B(x,α),y)=A(x,B(α,y));

holds for anyx,y∈[0,1];

(ii)B-migrative if it isα-B-migrative for anyα∈[0,1].

Definition4 (See Lopez-Molinaetal.[17]) Consider an aggregation functionB. An aggregation functionAis called (a,b)-B-bimigrative, with (a,b)∈[0, 1]2, if the identity

A(B(x,a),B(b,y))=A(B(x,b),B(a,y))

(1)

holds for anyx,y∈[0, 1]. Furthermore, we introduce the setB(A,B):

B(A,B)={(a,b)∈[0, 1]2|Ais

(a,b)-B-bimigrative}.

(2)

Definition5 (See Lopez-Molinaetal.[17]) Consider an aggregation functionB. An aggregation functionAis calledB-bimigrative if the identity

A(B(x,a),B(b,y))=A(B(x,b),B(a,y))

(3)

holds for anyx,y,a,b∈[0,1], or, equivalently, ifB(A,B)=[0,1]2.

Definition6 (See Lopez-Molinaetal.[17]) Consider an aggregation functionA.

(i) An elemente∈[0, 1] is called a neutral element ofAifA(x,e)=A(e,x)=xfor anyx∈[0,1].

(ii) An elementa∈[0,1] is called an absorbing element (or annihilator) ofAifA(x,a)=A(a,x)=afor anyx∈[0, 1].

Remark1 By Definition 6 and Definition 1, any overlap functionOhas only one absorbing element (or annihilator) 0. By Definition 6 and Definition 2, any grouping functionGhas only one absorbing element (or annihilator) 1.

Definition7 (See Yager and Rybalov[21]) A bivariate functionU:[0,1]2→[0,1] is said to be a uninorm if, for anyx,y,z∈[0,1], it satisfies the following conditions:

(U1)U(x,y)=U(y,x);

(U2)U(U(x,y),z)=U(x,U(y,z));

(U3)Uis non-decreasing in each place;

(U4) There has a neutral elemente∈[0, 1], that is,U(x,e)=x.

Definition8 (See Qiao and Hu[19]) Considerαin [0,1] andagiven overlap functionO. A uninormU:[0,1]2→[0,1] is said to beα-migrative overO((α,O)-migrative, for short) if

U(O(α,x),y)=U(x,O(α,y))

(4)

for allx,y∈[0,1].

In [22], Li and Shi had provedU(0,1)∈{0,1} for any uninormU. And, a uninormUis called conjunctive ifU(1,0)=0 and disjunctive ifU(1,0)=1.

Proposition1 (See Qiao and Hu[19]) Suppose thatαin [0,1],Ois a given overlap function andUis a uninorm with neutral elemente∈[0,1]. Consider the following statements:

(i)Uis (α,O)-migrative;

(ii)O(α,x)=U(O(α,e),x) for allx∈[0,1].

Then (i)⟺(ii).

Definition9 (See Masetal.[23]and Zongetal.[24]) A conjunctive (resp. disjunctive) uninormUis said to be locally internal on the boundary if it satisfiesU(1,x)∈{1,x} (resp.U(0,x)∈{0,x}) for allx∈[0,1].

Definition10 (See Qiao and Hu[19]) Considerα∈[0,1] and a given grouping functionG. A uninormU: [0,1]2→[0,1] is said to beα-migrative overG((α,G)-migrative, for short) if

U(G(α,x),y)=U(x,G(α,y))

(5)

for allx,y∈[0,1].

Proposition2 (See Qiao and Hu[19]) Suppose thatα∈[0,1],Gis a given grouping function andUis a uninorm with neutral elemente∈[0,1]. Consider the following statements:

(i)Uis (α,G)-migrative;

(ii)G(α,x)=U(G(α,e),x) for allx∈[0,1].

Then (i)⟺(ii).

Definition11 (See Calvoetal.[25]and Masetal.[26]) A bivariate functionF:[0,1]2→[0,1] is said to be a nullnorm if, for anyx,y,z∈[0,1], it satisfies the following conditions:

(F1)F(x,y)=F(y,x);

(F2)F(F(x,y),z)=F(x,F(y,z));

(F3)Fis non-decreasing in each place;

(F4) There has an absorbing elementk∈[0, 1], that is,F(k,x)=kand the following statements hold.

(i)F(0,x)=xfor allx≤k.

(ii)F(1,x)=xfor allx≥k.

Notice that whenk=0,Fis at-norm, and whenk=1,Fis at-conorm. In general,kis always given byF(1,0).

Definition12 (See Qiao and Hu[19]) Considerαin [0, 1] and a given overlap functionO. A nullnormF:[0,1]2→[0,1] is said to beα-migrative overO((α,O)-migrative, for short) if

F(O(α,x),y)=F(x,O(α,y))

(6)

for allx,y∈[0,1].

Definition13 (See Qiao and Hu[19]). Considerαin [0, 1] and a given grouping functionG. A nullnormF:[0,1]2→[0,1] is said to beα-migrative overG((α,G)-migrative, for short) if

F(G(α,x),y)=F(x,G(α,y))

(7)

for allx,y∈[0, 1].

Definition14 (See Zhu and Hu[20]). Considerα∈[0,1] and a given uninormU. An overlap functionOis said to beα-migrative overUor (α,U)-migrative if

O(U(α,x),y)=O(x,U(α,y))

(8)

for allx,y∈[0,1].

Proposition3 (See Zhu and Hu[20]) LetObe an overlap function andUbe a uninorm with neutral elemente∈[0, 1]. ThenUis conjunctive if and only ifOis (0,U)-migrative.

Definition15 (See Zhu and Hu[20]) Considerα∈[0, 1] and a given uninormU. A grouping functionGis said to beα-migrative overUor (α,U)-migrative if

G(U(α,x),y)=G(x,U(α,y))

(9)

for allx,y∈[0,1].

Definition16 (See Zhu and Hu[20]) Considerα∈[0,1] and a given nullnormF. An overlap functionOis said to beα-migrative overFor (α,F)-migrative if

O(F(α,x),y)=O(x,F(α,y))

(10)

for allx,y∈[0,1].

Definition17 (See Zhu and Hu[20]) Considerα∈[0,1] and a given nullnormF. A grouping functionGis said to beα-migrative overFor (α,F)-migrative if

G(F(α,x),y)=G(x,F(α,y))

(11)

for allx,y∈[0,1].

Proposition4 (See Wang and Liu[27]) LetO:[0,1]2→[0,1] be an overlap function,G:[0, 1]2→[0,1] be a grouping function, andφ:[0,1]→[0,1] be a strictly increasing automorphism. Then the following statements hold:

(i)Oφ:[0,1]2→[0,1] is an overlap function given byOφ(x,y)=φ-1(O(φ(x),φ(y))), for allx,y∈[0,1].

(ii)Gφ:[0,1]2→[0,1] is a grouping function given byGφ(x,y)=φ-1(G(φ(x),φ(y))), for allx,y∈[0,1].

2 Bimigrativity of overlap and grouping functions

In this section, we mainly propose the bimigrativity between overlap functions and grouping functions.

Proposition5 LetO,O*:[0, 1]2→[0, 1] be two overlap functions,G,G*:[0, 1]2→[0, 1] be two grouping functions, andφ:[0, 1]→[0, 1] be an automorphism. Then the following statements hold:

(i)OisG-bimigrative if and only ifOφisGφ-bimigrative.

(ii)GisO-bimigrative if and only ifGφisOφ-bimigrative.

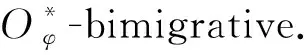
ProofHere we only prove the item (i).

(⟹) IfOis (φ(a),φ(b))-G-bimigrative, then one can has

Oφ(Gφ(x,a),Gφ(b,y))=

φ-1(O((φ(Gφ(x,a)),φ(Gφ(b,y))))=

φ-1(O((G(φ(x),φ(a)),G(φ(b),φ(y)))))=

φ-1(O((G(φ(x),φ(b)),G(φ(a),φ(y)))))=

φ-1(O((φ(Gφ(x,b)),φ(Gφ(a,y))))=

Oφ(Gφ(x,b),Gφ(a,y)).

(⟸) IfOφisGφ-bimigrative, then it is obvious thatOisG-bimigrative because (Oφ)φ-1=Oand (Gφ)φ-1=G.

Proposition6 LetO1is a 1-product overlap function. Then an overlap functionOisO1-bimigrativeif and only if it isO1-migrative.

ProofIfOisO1-bimigrative, then for allx,y,α∈[0, 1], one can has

O(xα,y)=O(O1(x,α),O1(1,y)=

O(O1(x, 1),O1(α,y)=O(x,αy).

ThereforeOisO1-migrative.

Conversely, ifOisO1-migrative, then for allx,y,a,b∈[0, 1], one can has

O(O1(x,a),O1(b,y))=O(xa,by)=

O(xab,y)=O(xb,ay)=

O(O1(x,b),O1(a,y)).

ThereforeOisO1-bimigrative.

Example2 LetO1is a 1-product overlap function andOpis ap-product overlap function. The overlap functionOpisO1-bimigrative.

Proposition7 LetO*be an overlap function with neutral element 1. If an overlap functionOisO*-bimigrative, then it is alsoO*-migrative.

ProofIfOisO*-bimigrative, then for allx,y,α∈[0, 1], it holds that

O(O*(x,α),y)=O(O*(x,α),O*(1,y))=

O(O*(x, 1),O*(α,y))=O(x,O*(α,y)).

ThereforeOisO*-migrative.

Lemma1 (See Gómezetal.[12]) Letφ: [0,1]→[0,1] be an automorphism. Then, for every overlap functionO,φ° andO(φ(x),φ(y)) are also overlap functions.

In this paper, the overlap functionO(φ(x),φ(y)) will be denoted byOφ(x,y).

Proposition8 AnyO-bimigrative overlap functionO*is alsoOφ-bimigrative.

ProofIfO*isO-bimigrative, then for allx,y,a,b∈[0, 1], we have

O*(Oφ(x,a),Oφ(b,y))=

O*(O(φ(x),φ(a)),O(φ(b),φ(y)))=

O*(O(φ(x),φ(b)),O(φ(a),φ(y)))=

O*(Oφ(x,b),Oφ(a,y)).

ThereforeO*isOφ-bimigrative.

Proposition9 LetO,O*:[0,1]2→[0,1] be two overlap functions with neutral element 1.OisO*-bimigrative if and only ifO=O*.

ProofThe sufficiency is obvious. In the following, we only prove the necessity.

IfOisO*-bimigrative, then for anyx,y∈[0, 1],

O(x,y)=O(O*(1,x),O*(1,y))=

O(O*(1,1),O*(x,y))=

O(1,O*(x,y))=O*(x,y).

ThereforeO=O*.

In a similar way, one can has the following proposition.

Proposition10 LetG,G*: [0,1]2→[0,1] be two grouping functions with neutral element 0.GisG*-bimigrative if and only ifG=G*.

Proposition11 LetO,O*: [0,1]2→[0,1] be any two overlap functions.Ois always (0,0)-O*-bimigrative. If 1 is the neutral element ofO*, thenOis always (1,1)-O*-bimigrative.

By Definition 2 and Definition 4, the following proposition holds.

Proposition12 LetG,G*: [0,1]2→[0,1] be any two overlap functions.Gis always (1,1)-G*-bimigrative. If 0 is the neutral element ofG*, thenGis always (0,0)-G*-bimigrative.

Proposition13 LetObe an overlap function with 1 as neutral element andb∈[0, 1], ifOis (1,b)-Op-bimigrative, then it follows that

O(xp,bp)=xpbp

for anyx∈[0,1].

ProofIfOis (1,b)-Op-bimigrative, then for anyx∈[0,1], we have

O(xp,bp)=O(xp1p,bp1p)=

O(xpbp, 1p1p)=

O(xpbp, 1)=xpbp.

3 Bimigrativity of uninorms over overlap and grouping functions

In this section, we discuss the bimigrativity of uninorms over overlap and grouping functions.

3.1 Bimigrativity of uninorms over overlap functions

Definition18 Considerα,β∈[0,1] and a given overlap functionO. A uninormU:[0,1]2→[0,1] is said to be (α,β)-O-bimigrative if

U(O(x,α),O(β,y))=U(O(x,β),O(α,y))

(12)

for allx,y∈[0, 1].

Clearly, for any overlap functionOandα∈[0,1], a uninormUis (α,α)-O-bimigrative.

Definition19 Consider a given overlap functionO. A uninormU:[0,1]2→[0,1] is said to beO-bimigrative if

U(O(x,α),O(β,y))=U(O(x,β),O(α,y))

(13)

for allx,y,α,β∈[0,1].

Proposition14 LetObe an overlap function with neutral element 1 andα∈[0,1]. A uninormUis (α,1)-O-bimigrative if and only ifUis (α,O)-migrative.

ProofUis (α, 1)-O-bimigrative

⟺U(O(x,α),O(1,y))=U(O(x, 1),O(α,y))

⟺U(O(x,α),y)=U(x,O(α,y))

⟺Uis(α,O)-migrative.

Proposition15 LetObe an overlap function with neutral element 1 andUbe a uninorm with neutral elemente∈[0, 1]. IfUis (0,e)-O-bimigrative, thenU(O(x,e), 0)=0 for anyx∈[0,1].

ProofIf a uninormUis (0,e)-O-bimigrative, then for anyx∈[0, 1], we have

U(O(x,e), 0)=U(O(x,e),O(0,1))=

U(O(x, 0),O(e,1))=U(0,e)=0.

Proposition16 LetObe an overlap function with neutral element 1 andUbe a uninorm with neutral elemente∈[0,1]. IfUis (1,e)-O-bimigrative, thenU(O(x,e), 1)=xfor anyx∈[0,1].

ProofIf a uninormUis (1,e)-O-bimigrative, then for anyx∈[0,1], we have

U(O(x,e),1)=U(O(x,e),O(1,1))

=U(O(x,1),O(e,1))=U(x,e=x.

Proposition17 LetObe an overlap function with neutral element 1 andUbe a uninorm with neutral elemente∈[0,1].Uis (0,1)-O-bimigrative if and only ifUis conjunctive.

ProofIfUis (0,1)-O-bimigrative, then

U(0,1)=U(O(0,0),O(1,1))=

U(O(0,1),O(0,1))=U(0,0)=0.

HenceUis conjunctive.

Conversely, ifUis conjunctive,i.e.,U(0,1)=0, then for anyx∈[0,1],U(0,x)=0 by the monotonicity ofU. For anyx,y∈[0,1],U(O(x,0),O(1,y))=U(0,y)=0,U(O(x,1),O(0,y))=U(x,0)=0. HenceU(O(x,0),O(1,y))=U(O(x,1),O(0,y)),i.e.Uis (0,1)-O-bimigrative.

Proposition18 LetO1is a 1-product overlap function. Then a uninormUisO1-bimigrative if and only ifUisO1-migrative.

ProofThe proof is similar to Proposition 6.

Proposition19 LetObe an overlap function with neutral element 1. If a uninormUisO-bimigrative,then it is alsoO-migrative.

ProofThe proof is similar with Proposition 7.

Proposition20 AnyO-bimigrative uninormUis alsoOφ-bimigrative.

ProofThe proof is similar to Proposition 8.

Proposition21 LetObe an given overlap function andUbe a uninorm with neutral elemente∈[0, 1].

If for anyα∈[0,1],Uis (α,1)-O-bimigrative, thenU(0, 1)=0.

ProofTakex=0 andy=1 in Eq. (12). Assume thatU(0, 1)=1. Then, it follows that

1=U(0, 1)=U(O(0,α),O(1, 1))

=U(O(0, 1),O(α, 1))=U(0,O(α, 1))

≤U(e,O(α, 1))=O(α, 1).

Thus, one gets thatO(α,1)=1. Moreover, from item (O3) of Definition 1, one can get thatα=1,which is a contradiction.

By Proposition 1 and Definition 18, the following proposition holds.

Proposition22 LetObe an given overlap function with neutral element 1,α∈[0,1] andUbe a uninorm with neutral elemente∈[0,1]. Consider the following statements:

(i)Uis (α,1)-O-bimigrative;

(ii)O(α,x)=U(O(α,e),x) for allx∈[0,1].

Then (i)⟺(ii).

Proposition23 LetUis conjunctive and locally internal on the boundary. If, for a givenα∈[0,1] and an overlap functionOwith neutral element 1,Uis a (α,1)-O-migrative uninorm with neutral elemente∈[0, 1], then the following statements hold

(i)O(α, 1)=O(α,e) ande>0;

(ii)O(α,1)

ProofBy Eq. (12), we have

O(α,1)=U(O(α,1),e)=

U(O(1,α),O(1,e))=

U(O(1,1),O(α,e))=

U(1,O(α,e)) ∈{1,O(α,e)}.

Sinceα<1, by item (O3) of Definition 1, it follows thatO(α,1)<1. Thus, one has thatO(α,1)=O(α,e).

Moreover, ife=0, thenO(α,1)=O(α,e)=0. Thus, by item (O2) of Definition 1, we get thatα=0, which is a contradiction. Thereforee>0.

(ii) Suppose thatO(α, 1)≥e, then, by item (i), it follows that

O(α,1)=U(O(α,1),e)=

U(O(1,α),O(1,e))=

U(O(1,1),O(α,e))=

U(1,O(α,e))=

U(1,O(α,1))≥U(1,e)=1.

But, from the proof of item (i), we know thatO(α, 1)<1. Hence, one get thatO(α, 1)

From Proposition 22 and item (i) of Proposition 23, one can immediately obtain the followingconclusion.

Proposition24 LetObe a given overlap function with neutral element 1,α∈[0,1] andUbe auninorm with neutral elemente∈[0,1]. Consider the following statements:

(i)Uis (α,1)-O-bimigrative;

(ii)O(α,x)=U(O(α,1),x) for allx∈[0,1].

Then (i)⟺(ii).

3.2 Bimigrativity of uninorms over grouping functions

Definition20 Considerα∈[0,1] and a given grouping functionG. A uninormU:[0,1]2→[0,1] is said to be (a,b)-G-bimigrative if

U(G(x,α),G(α,y))=U(G(x,β),G(α,y))

(14)

for allx,y∈[0,1].

Clearly, for any grouping functionGandα∈[0,1], a uninormUis (α,α)-G-bimigrative.

Definition21 Consider a given grouping functionG. A uninormU:[0,1]2→[0,1] is said to beG-bimigrative if

U(G(x,α),G(β,y))=U(G(x,β),G(α,y))

(15)

for allx,y,α,β∈[0, 1].

Proposition25 LetGbe a grouping functions with neutral element 0 andα∈[0,1]. A uninormUis (α, 0)-G-bimigrative if and only ifUis (α,G)-migrative.

ProofIt can be proven in a similar way as Proposition 14.

Proposition26 LetGbe a grouping functions with neutral element 0 andUbe a uninorm with neutral elemente∈[0, 1]. IfUis (1,e)-G-bimigrative, thenU(G(x,e), 1)=1 for anyx∈ [0, 1].

ProofIt can be proven in a similar way as Proposition 15.

Proposition27 LetGbe a functions with neutral element 1 andUbe a uninorm with neutral elemente∈ [0,1]. IfUis (0,e)-G-bimigrative, thenU(O(x,e), 0)=xfor anyx∈[0, 1].

ProofIt can be proven in a similar way as Proposition 16.

Proposition28 LetGbe a grouping functions with neutral element 0 andUbe a uninorm with neutral elemente∈[0,1].Uis (0,1)-G-bimigrative if and only ifUis conjunctive.

ProofIt can be proven in a similar way as Proposition 17.

Proposition29 A uninormUisG1-bimigrative if and only ifUisG1-migrative.

ProofThe proof is similar to Proposition 6.

Proposition30 Let G be a grouping function with neutral element 0. If A uninormUisG-bimigrative, then it is alsoG-migrative.

ProofThe proof is similar to Proposition 7.

Proposition31 LetGbe a given grouping function andUbe a uninorm with neutral elemente∈[0,1]. If for anyα∈[0,1],Uis (0,α)-G-bimigrative, thenU(0,1)=1.

ProofTakex=0 andy=1 in Eq. (14). Assume thatU(0, 1)=0. Then, it follows that

0=U(0,1)=

U(G(0,0),G(α,1))=

U(O(0,α),G(0,1))=

U(G(0,α), 1)≥

U(G(0,α),e)=G(0,α).

Thus, one gets thatG(0,α)=0. Moreover, from item (G2) of Definition 2, one can get thatα=0,which is a contradiction.

By Proposition 2 and Definition 20, the following proposition holds.

Proposition32 LetGbe a given grouping function with neutral element 0,α∈[0,1] andUbe a uninorm with neutral elemente∈[0,1]. Consider the following statements:

(i)Uis (0,α)-G-bimigrative;

(ii)G(α,x)=U(G(α,e),x) for allx∈[0, 1].

Then (i)⟺(ii).

Proposition33 LetUis disjunctive and locally internal on the boundary. If, for a givenα∈[0,1] and a grouping functionGwith neutral element 0,Uis a (0,α)-G-migrative uninorm with neutral elemente ∈[0,1], then the following statements hold

(i)G(α,0)=O(α,e) ande<1;

(ii)G(α,0)>e.

ProofIt can be proven in a similar way as Proposition 23.

From Proposition 32 and item (i) of Proposition 33, one can immediately obtain the following conclusion.

Proposition34 Let G be a given grouping function with neutral element 0,α∈[0,1] andUbe auninorm with neutral elemente∈[0,1]. Consider the following statements:

(i)Uis (α,0)-G-bimigrative;

(ii)G(α,x)=U(G(α,0),x) for allx∈[0, 1].

Then (i)⟺(ii).

4 Bimigrativity of nullnorms over overlap and grouping functions

Similarly to the case of uninorms, we study the bimigrativity of nullnorms over overlap and grouping functions in this section.

4.1 Bimigrativity of nullnorms over overlap functions

Definition22 Considerα,β∈[0,1] and a given overlap functionO. A nullnormF:[0,1]2→[0,1] is said to be (α,β)-O-bimigrative if

F(O(x,α),O(β,y))=F(O(x,β),O(α,y))

(16)

for allx,y∈[0,1].

Clearly, for any overlap functionOandα∈ [0, 1], a nullnormFis (α,α)-O-bimigrative.

Definition23 Consider a given overlap functionO. A nullnormF:[0,1]2→[0,1] is said to beO-bimigrative if

F(O(x,α),O(β,y))=F(O(x,β),O(α,y))

(17)

for allx,y,α,β∈[0, 1].

Proposition35 LetObe a given overlap function with neutral element 1 andFbe a nullnorm with absorbing elementk∈[0,1]. ThenFis not (0,1)-O-bimigrative.

ProofSuppose thatFis not (0,1)-O-bimigrative, then for allx,y∈[0,1],

F(O(x,0),O(1,y))=F(O(x,1),O(0,y))⟹

F(0,y)=F(x, 0)

Takex=0 andy=k, we havek=F(0,k)=F(0,0)=0, which is a contradiction.

Proposition36 LetObe an overlap function with neutral element 1 andα∈[0,1]. An nullnormFis (α, 1)-O-bimigrative if and only ifFis (α,O)-migrative.

ProofIt can be proven in a similar way as Proposition 14.

Proposition37 LetObe an overlap function andFbe a nullnorm with absorbing elementk∈[0, 1]. IfFis (0,k)-O-bimigrative, thenF(O(x,k), 0)=0 for anyx∈[0, 1].

ProofIf F is (0,k)-O-bimigrative, then for anyx∈[0, 1], one has that

F(O(x,k), 0)=F(O(x,k),O(0,0))

=F(O(x,0),O(k,0))=F(0,0)=0.

Proposition38 LetObe an overlap function with neutral element 1 andFbe a nullnorm with absorbing elementk∈[0, 1]. IfFis (k,1)-O-bimigrative, thenF(O(x,k), 1)=kfor anyx∈[0,1].

ProofIfFis (k, 1)-O-bimigrative, then for anyx∈[0, 1], one has that

F(O(x,k), 1)=F(O(x,k),O(1,1))

=F(O(x,1),O(k,1))=F(x,k)=k.

4.2 Bimigrativity of nullnorms over grouping functions

Definition24 Considerα,β∈[0,1] and a given grouping functionG. A nullnormF: [0,1]2→[0,1] is said to be (α,β)-G-bimigrative if

F(G(x,α),G(β,y))=F(G(x,β),G(α,y))

(18)

for allx,y∈[0, 1].

Clearly, for any overlap functionGandα∈[0,1], a nullnormFis (α,α)-G-bimigrative.

Definition25 Consider a given grouping functionG. A nullnormF:[0,1]2→[0,1] is said to beG-bimigrative if

F(G(x,α),G(β,y))=F(G(x,β),G(α,y))

(19)

for allx,y,α,β∈[0,1].

Proposition39 LetGbe a given grouping function with neutral element 0 andFbe a nullnorm with absorbing elementk∈[0, 1]. ThenFis not (0,1)-G-bimigrative.

ProofIt can be proven in a similar way as Proposition 35.

Proposition40 LetGbe a grouping function with neutral element 0 andα∈[0,1]. An nullnormFis (α, 0)-G-bimigrative if and only ifFis (α,G)-migrative.

ProofIt can be proven in a similar way as Proposition 14.

Proposition41 LetGbe a grouping function andFbe a nullnorm with absorbing elementk∈[0,1]. IfFis (1,k)-G-bimigrative, thenF(G(x,k), 1)=1 for anyx∈[0,1].

ProofIt can be proven in a similar way as Proposition 37.

Proposition42 LetGbe a grouping function with neutral element 0 andFbe a nullnorm with absorbing elementk∈[0,1]. IfFis (k, 0)-O-bimigrative, thenF(O(x,k), 0)=kfor anyx∈[0,1].

ProofIt can be proven in a similar way as Proposition 38.

5 Bimigrativity of overlap and grouping functions over uninorms (resp. nullnorms)

In section 3 and section 4, the bimigrativity of uninorms and nullnorms over overlap and grouping functions are discussed respectively. For simplicity, in this section, we highlight only the bimigrativity of overlap and grouping functions over uninorms (resp. nullnorms), but no proofs are provided.

5.1 Bigrativity of overlap functions over uninorms

Definition26 Considerα,β∈[0,1] and a given uninormU. An overlap functionOis said to be (α,β)-U-bimigrative if

O(U(x,α),U(β,y)=O(U(x,β),U(α,y))

(20)

for allx,y∈[0, 1].

Clearly, for any uninormUandα∈[0, 1], an overlap functionOis (α,α)-U-bimigrative.

Definition27 Consider a given uninormU. An overlap functionOis said to beU-bimigrative if

O(U(x,α),U(β,y)=O(U(x,β),U(α,y))

(21)

for allx,y,α,β∈[0, 1].

Proposition43 LetObe an overlap function andUbe a uninorm with neutral elemente∈[0,1].Ois (α,e)-U-bimigrative if and only ifOis (α,U)-migrative.

Proposition44 LetObe an overlap function andUbe a uninorm with neutral elemente∈[0, 1].ThenUis conjunctive if and only ifOis (0,e)-U-bimigrative.

Proposition45 LetObe an overlap function with neutral element 1,Ube a uninorm with neutral elemente∈[0, 1]. IfOis (α,e)-U-bimigrative, thenO(U(1,α),e)=α.

Proposition46 LetObe an overlap function with neutral element 1,Ube a uninorm with neutral elemente∈[0, 1]. IfUis disjunctive, thenOis (α,e)-U-bimigrative if and only ifα=e.

Proposition47 LetObe an overlap function with neutral element 1,U∈CLIB with neutral elemente ∈[0, 1], andα=e. IfOis (α,e)-U-bimigrative, thenO(α,x)=U(α,x) for allx∈[0,1].

Proposition48 LetUbe a uninorm with neutral elemente∈[0,1]. If an overlap functionOisU-bimigrative, then it is alsoU-migrative.

ProofThe proof is similar with Proposition 7.

5.2 Bigrativity of grouping functions over uninorms

Definition28 Considerα,β∈[0,1] and a given uninormU. A grouping functionGis said to be(α,β)-U-bimigrative if

G(U(x,α),U(β,y)=G(U(x,β),U(α,y))

(22)

for allx,y∈[0,1].

Clearly, for any uninormUandα∈[0,1], a grouping functionGis (α,α)-U-bimigrative.

Definition29 Consider a given uninormU. A grouping functionGis said to beU-bimigrative if

G(U(x,α),U(β,y)=G(U(x,β),U(α,y))

(23)

for allx,y,α,β∈[0,1].

Proposition49 LetGbe a grouping function andUbe a uninorm with neutral elemente∈[0,1].Gis (α,e)-U-bimigrative if and only ifGis (α,U)-migrative.

ProofThe proof is similar with Proposition 43.

Proposition50 LetGbe a grouping function andUbe a uninorm with neutral elemente∈[0,1].ThenUis disjunctive if and only ifGis (1,U)-migrative.

By Proposition 49 and Proposition 50, the following proposition holds.

Proposition51 LetGbe a grouping function andUbe a uninorm with neutral elemente∈[0,1].ThenUis disjunctive if and only ifGis (1,e)-U-bimigrative.

Proposition52 LetGbe a grouping function with neutral element 0,Ube a uninorm with neutral elemente∈[0, 1]. IfGis (α,e)-U-bimigrative, thenG(U(0,α),e)=α.

Proposition53 LetGbe a grouping function with neutral element 0,Ube a uninorm with neutralelemente∈[0,1]. IfUis conjunctive, thenOis (α,e)-U-bimigrative if and only ifα=e.

Proposition54 LetGbe a grouping function with neutral element 0,U∈DLIB with neutralelemente∈[0, 1], andα=e. IfGis (α,e)-U-bimigrative, thenO(α,x)=U(α,x) for allx∈[0, 1].

Proposition55 LetUbe a uninorm with neutral elemente∈[0,1]. If a grouping functionGisU-bimigrative, then it is alsoU-migrative.

5.3 Bigrativity of overlap functions over nullnorms

Definition30 Considerα,β∈[0,1] and a given nullnormF. An overlap functionOis said to be (α,β)-F-bimigrative if

O(F(x,α),F(β,y))=O(F(x,β),F(α,y))

(24)

for allx,y∈[0, 1].

Definition31 Consider a given nullnormF. An overlap functionOis said to beF-bimigrative if

O(F(x,α),F(β,y))=O(F(x,β),F(α,y))

(25)

for allx,y,α,β∈[0, 1].

Proposition56 LetObe an overlap function andFbe a nullnorm with absorbing elementk∈[0,1].IfOis (0,k)-F-bimigrative, thenO(F(x, 0),k)=0 for anyx∈[0,1].

Proposition57 LetObe an overlap function with neutral element 1 andFbe a nullnorm with absorbing elementk∈[0,1]. IfOis (1,k)-F-bimigrative, thenO(F(x,1),k)=kfor anyx∈[0,1].

5.4 Bigrativity of grouping functions over nullnorms

Definition32 Considerα,β∈[0,1] and a given nullnormF. A grouping functionGis said to be (α,β)-F-bimigrative if

G(F(x,α),F(β,y))=G(F(x,β),F(α,y))

(26)

for allx,y∈[0,1].

Definition33 Consider a given nullnormF. A grouping functionGis said to beF-bimigrative if

G(F(x,α),F(β,y))=G(F(x,β),F(α,y))

(27)

for allx,y,α,β∈[0,1].

Proposition58 LetGbe a grouping function andFbe a nullnorm with absorbing elementk∈[0,1].IfGis (1,k)-F-bimigrative, thenG(F(x,1),k)=1 for anyx∈[0,1].

Proposition59 LetGbe a grouping function with neutral element 0 andFbe a nullnorm with absorbing elementk∈[0,1]. IfGis (0,k)-F-bimigrative, thenG(F(x, 0),k)=kfor anyx∈[0,1].

6 Conclusions

In this paper, we mainly devote to characterizing the bimigrativity between overlap functions,grouping functions and uninorms or nullnorms. We also discuss the relationship between the bimigrativity and migrativity overlap functions, grouping functions and uninorms or nullnorms. In the further work, we will investigate the bimigrativity of other aggregation functions.