EXISTENCE AND MULTIPLICITY OF SOLUTIONS FOR A COUPLED SYSTEM OF KIRCHHOFF TYPE EQUATIONS∗

Yaghoub JALILIAN

Department of Mathematics,Razi University,Kermanshah,Iran

E-mail:y.jalilian@razi.ac.ir

Abstract In this paper,we study the coupled system of Kirchhoff type equationswhere a,b>0,α,β>1 and 3<α+β<6.We prove the existence of a ground state solution for the above problem in which the nonlinearity is not 4-superlinear at infinity.Also,using a discreetness property of Palais-Smale sequences and the Krasnoselkii genus method,we obtain the existence of infinitely many geometrically distinct solutions in the case when α,β≥2 and 4≤α+β<6.

Key words Kirchhoff equation;Nehari-Poho˘zave manifold;constrained minimization;ground state solution

1 Introduction

In this article,we investigate the existence and multiplicity of solutions to the coupled system of Kirchhoff type equations

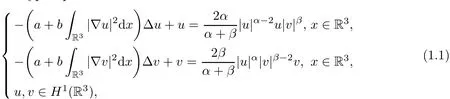
wherea,b>0,α,β>1 and 3<α+β<6.

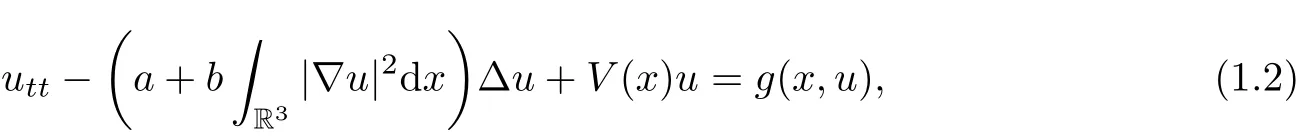
Kirchhoff type equations are related to the stationary analogue of the equation proposed by Kirchhoff[1]which is an extension of the classical D’Alembert’s wave equation for free transversal vibrations of a clamped string.Equation(1.2)arises in various physical and biological systems.For example,in biological systems,ucould describe the population density in a biological phenomena such as bacteria spreading(see[2]).

The assumptions considered in[5]imply the Cerami compactness condition for the energy functional.As far as we know[5]is the only paper considering the existence of infinitely many solutions for(1.3)in whichf(x,u)may not be 4-superlinear at infinity.To the best of our knowledge,there are a few results concerning the existence of solutions for coupled Kirchhoff type systems.Shi and Chen[16]investigated the existence of a ground state solution for the system

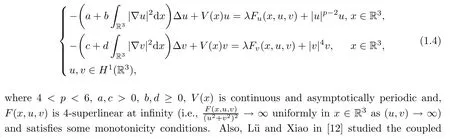
wherea,b>0,λ>0 is a real parameter,α>2,β>2,α+β<6 andV(x),W(x)are nonnegative continuous functions on R3.They established the existence and multiplicity of solutions to(1.5)forλ>λ0>0.In this paper,the assumption 4<α+βis crucial to prove the boundedness of Palais-Smale sequences.This assumption implies the 4-superlinearity of the nonlinearity at infinity.Therefore,the results of[12,16]are not applicable for(1.1)when 3<α+β≤4.

The aim of this paper is to prove the existence of a ground state solution to the coupled Kirchhoff type system(1.1)when 3<α+β<6.We use an argument developed by[11],to obtain a natural constraint via the Nehari manifold and the Poho˘zave identity.Moreover,using the Krasnoselskii genus,we investigate the existence of infinitely many geometrically distinct solutions in the case whenα,β≥2 and 4≤α+β<6.

In our first main result we prove the existence of a ground state solution for(1.1).

Theorem 1.1Assumeα,β>1 and 3<α+β<6.Then problem(1.1)has a ground state solution.

In our next result we study the multiplicity of solutions for(1.1).Letw=(u,v)be a solution of(1.1).Define the orbit ofwunder the action of Z3as O(w):={w(·−k):k∈Z3}.Two solutionsw1andw2are said to be geometrically distinct if O(w1)and O(w2)are disjoint.

Theorem 1.2Letα,β≥2 and 4≤α+β<6.Then problem(1.1)has infinitely many geometrically distinct solutions.

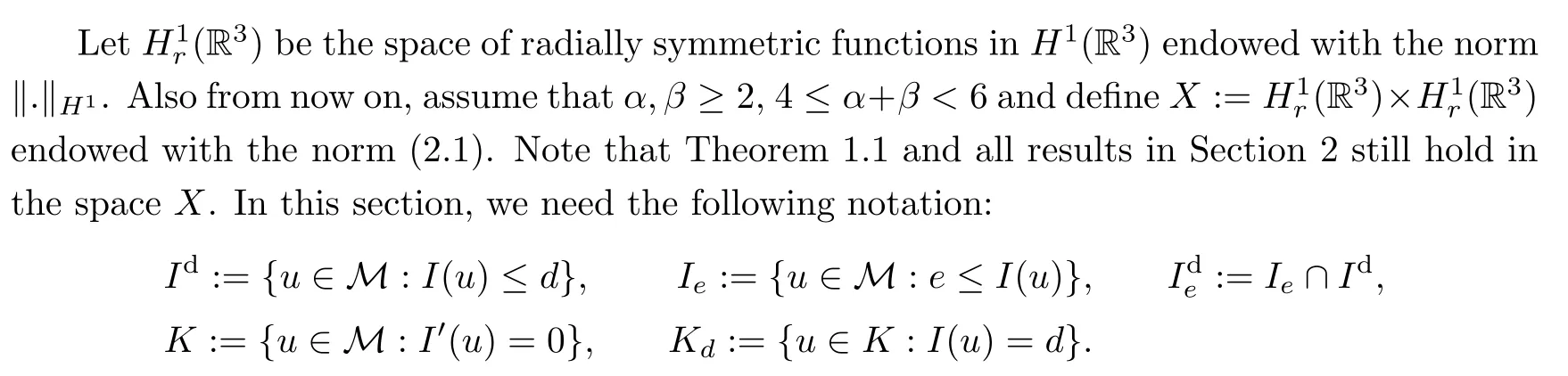
NotationsC,C1,C2,···are positive constants.B(r,x):={y∈R3:|x−y|

2 Nehari-Poho˘zave Manifold

4 Proof of Theorem 1.2

We also recall the definition of the Krasnoselskii genus[19].A setF⊂Xis said to be symmetric ifF=−F.Let

ForF≠∅andF∈Σ,the Krasnoselskii genus ofFis the least integernsuch that there exists an odd functionf∈C(F,Rn{0}).The genus ofFis denoted byγ(F).Setγ(∅):=0 andγ(F):=∞if there exists nofwith the above property for anyn.

According to Theorem 1.1 the setKis nonempty.Let G be a subset ofKsuch that G=−G and each orbit O(u)⊂Khas a unique representative in G.We shall show that G has infinitely many elements.In the sequel,by contradiction,we assume that G is a finite set and this will lead to a contradiction.

Lemma 4.1κ:=inf{‖v−w‖:v,w∈K∪{0},v≠w}>0.

ProofThe proof is exactly the same as Lemma 2.13 in[20]and we omit it.We have to mention that in[20]the infimum is taken over allv,w∈K.Since 0 is an isolated critical point,κremains positive.