弯曲载荷作用下薄壁方形截面管周期角裂纹的应力强度因子
2020-08-25李龙袁浩谢禹钧
李龙 袁浩 谢禹钧


摘 要:薄壁方形截面管广泛应用于建筑、机械、石油化工等领域,随着使用年限的增加和人为因素的影响,薄壁管会产生裂纹。应力强度因子作为一个断裂参量,在工程构件断裂分析过程中起着重要的作用。在以往的研究中,几乎所有关于同一个裂纹截面含多个不同奇异应力场的情形都被回避。以弯曲载荷作用下薄壁方形截面管周期角裂纹问题为例,利用初等力学和守恒律的概念,通过找出不同应力强度因子之间的简单关系,提出了一种简便的求解同一截面上不同应力强度因子的方法。同时也给出了有限元数值解与本文解的比较,两者吻合较好。
关 键 词:薄壁方形截面管;应力强度因子;裂纹;守恒律;有限元
中图分类号:TE832 文献标识码: A 文章编号: 1671-0460(2020)05-0903-05
Abstract: Thin-walled square-section tubes are widely used in construction, machinery, petrochemical and other fields. With the increase of service life and human factors, thin-walled tubes will crack. As a fracture parameter, the stress intensity factor plays an important role in the fracture analysis of engineering components. In previous studies, almost all cases that the same crack section contained multiple different singular stress fields were avoided. In this paper, taking the periodic angle crack of thin-walled square-section tube under bending load as an example, using the concept of elementary mechanics and conservation law, a simple relationship between different stress intensity factors was proposed to solve a simple cross section. At the same time, the comparison between the finite element numerical solution and the solution was carried out, and the results from present method were accordant with those from finite element method.
Key words: Thin-walled square section tube; Stress intensity factor; Crack; Conservation law; Finite element
在實际工程结构中,大多数裂纹问题是三维有限边界问题,裂纹应力强度因子的确定一直是断裂力学领域的主要问题。对于含有一个裂纹或者多个裂纹的应力强度因子相同的情形,利用 积分法[1-10]给出解析解相对容易,如方形截面薄壁管横向裂纹的应力强度因子K1 [11]。由于J2积分只提供一个与应力强度因子有关的方程,该方法只能应用于求解含一个未知应力强度因子的情形,当同一个截面有多个不同的奇异应力场时,仅用J2积分则无法求解。
本文基于材料力学中平截面的变形假设,提出了求解不同奇异应力场对应的应力强度因子的补充方程,进而得到了弯曲载荷作用下薄壁方形截面管周期角裂纹的应力强度因子。
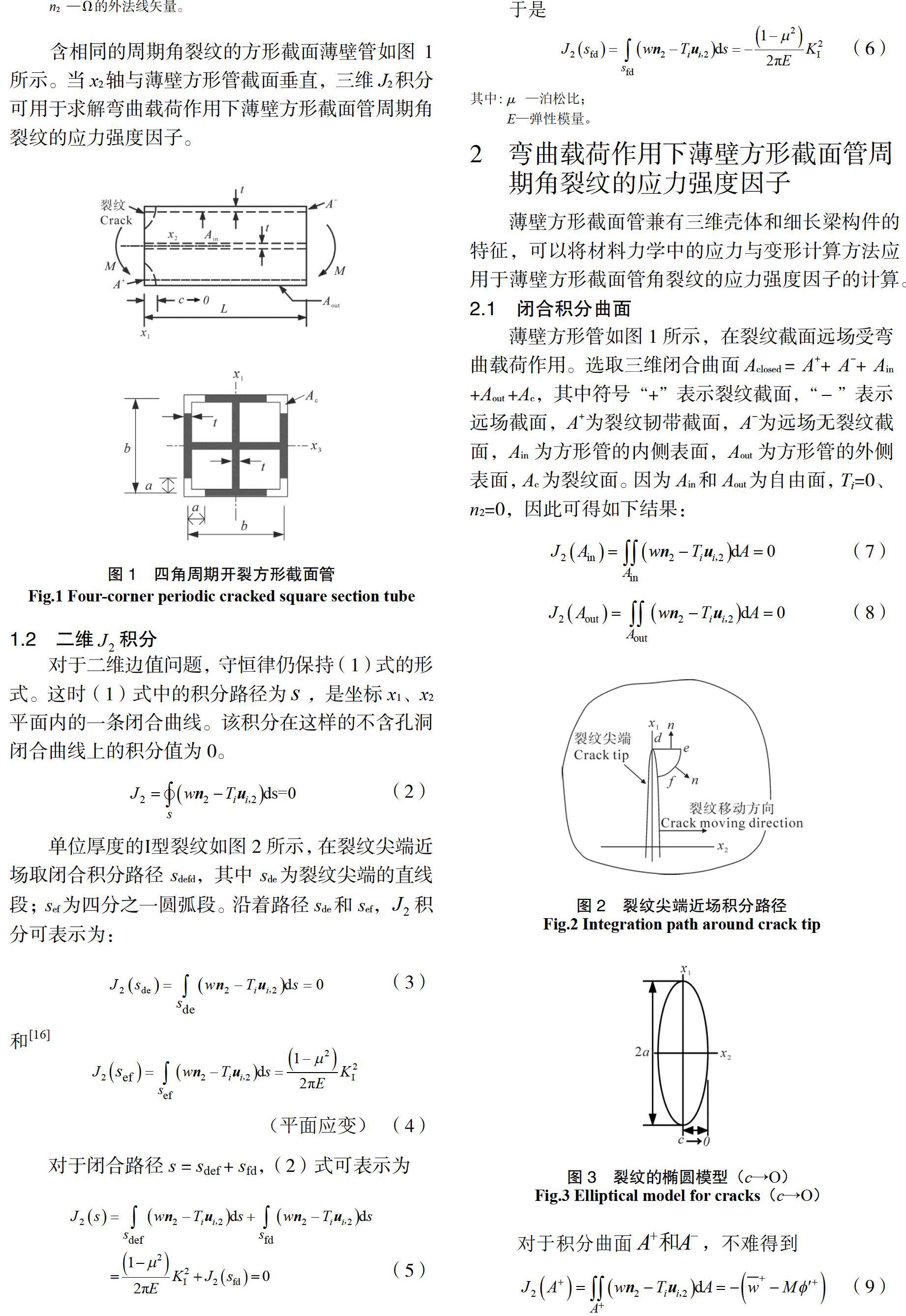
含相同的周期角裂纹的方形截面薄壁管如图1所示。在平面应变状态下薄壁管共有8个裂纹尖端,x1-x3平面为含裂纹的对称平面,所有的载荷均作用在该平面上,同时弯曲变形也将发生在这个平面。很显然,当满足 时,薄壁管的形变具有细长梁构件和三维壳体的特征。
1 积分
1.1 三维J2积分
考虑三维位移场,其位移矢量u是x1、x2和x3的函数,对于闭合曲面Ω, 根据守恒律,(1)式的积分为0[12-15]。
利用ABAQUS软件对本文模型进行有限元分析。选用的单元类型为八结点线性六面体单元(C3D8R)[20]。模型材料属性设置为弹性模量E=200 GPa,泊松比μ=0.3。有限元模型的几何尺寸为:边长b=300 mm,壁厚t =10 mm,加强筋厚t =10 mm,L=1 000 mm。裂纹尖端采用四分点退化而成的奇异单元。有限元网格划分如图5所示。本文解与有限元解的比较结果如图6所示。
3 结论
本文根据材料力学平截面的变形假设,提出了同一含裂纹截面不同应力场对应的应力强度因子之间的补充方程,并通过与 积分方程联立,建立了弯矩作用下薄壁方形截面管周期角裂纹应力强度因子的求解方法。最后,采用ABAQUS对本文模型进行数值模拟,将本文解与有限元法得出的解进行比较,验证本文方法的有效性。本文提出的方法丰富了 积分在求解应力强度因子方面的应用,并适用于其他类似结构在平面弯曲作用下同一截面多个不同裂纹应力强度因子的求解。
参考文献:
[1]Xie YJ. A theory on cracked pipe. International Journal of Pressure Vessels and Piping [J].1998, 75: 865-969.
[2]尤国武, 谢禹钧. 工字型截面梁腹板中心裂纹的应力强度因子[J].辽宁石油化工大学学报, 2006, 26(2): 63-65.
[3]谢禹钧.弯曲载荷作用下工字形截面梁腹板中心穿透裂纹的应力强度因子[J]. 机械强度,2006, 28(3):397-400.
[4]Cortínez VH, Dotti FE. Mode I stress intensity factor for cracked thin-walled open beams[J]. Engineering Fracture Mechanics,2013, 110: 249-257.
[5]Nobile L. Mixed mode crack initiation and direction in beams with edge crack[J]. Theoretical and Applied Fracture Mechanics,2000(33): 107-116.
[6]袁浩,谢禹钧. D型截面管应力强度因子分析方法[J]. 机械强度,2018, 40(6):1473-1478.
[7]谢禹钧, 王晓华,王伟. 拉伸环向周期裂纹管的应力强度因子[J]. 工程力学,2006, 23(6):173-176.
[8]王晓华, 蔡永梅,谢禹钧. 平板双侧触压的应力强度因子[J]. 工程力学,2009, 26(3):6-35.
[9]王伟,蔡永梅,谢禹钧. 椭圆形截面管环向裂纹应力强度因子分析方法[J]. 工程力学,2011,28(11): 197-201.
[10]邵菁,张德琦,付路路,等. 管道表面椭圆裂纹的断裂力学有限元分析[J]. 当代化工, 2015, 44(8):1972-1976.
[11]谢禹钧. 方形截面薄壁管横向裂纹的应力强度因KI[J]. 工程力学, 2004, 21(6):183-186.
[12]Xie YJ. An analytical method on circumferential periodic cracked pipes and shells[J]. International Journal of Solids and Structures, 2000, 37: 5189-5201.
[13]Budiansky B, Rice JR. Conservation laws and energyrelease rates[J]. Journal of Applied Mechanics, 1973, 40: 201-203.
[14]Eshelby JD. The force on an elastic singularity[J]. Philosophical Transactionsof the Royal Society, 1951, 244: 87-112.
[15]Rice JR. A path independent integral and approximate analysis of strain concentration by notchs and cracks[J]. Journal of Applied Mechanics, 1968, 35: 379-386.
[16]Xie YJ, Xu H, Li PN. Crack mouth widening energy-release rate and its application[J]. Theoretical and Applied Fracture Mechanics, 1998, 29 (3): 195-203.
[17]Xie Y J, Li P N, Xu H. On KI estimates of cracked pipes using an elliptical hole model and elementary beam strength theory of cracked beams[J]. Engineering Fracture Mechanics, 1998, 59 (3): 399-402.
[18]H. Yuan, W. J. Liu, Y. J. Xie. Mode-I stress intensity factors for cracked special-shaped shellsunder bending[J]. Engineering Fracture Mechanics, 2019, 207: 131-148.
[19]Tada H, Paris PC, Irwin GR. The stress analysis of cracks handbook. [M]. Beijing:Science Press,2000:481-482.
[20]莊茁,由小川,廖建辉, 等. 基于ABAQUS的有限元分析和应用[M]. 北京:清华大学出版社,2009.
