国产结构用铝合金断裂韧性参数校准

张超众 郭小农 宗绍晗 陈宇 朱劭骏

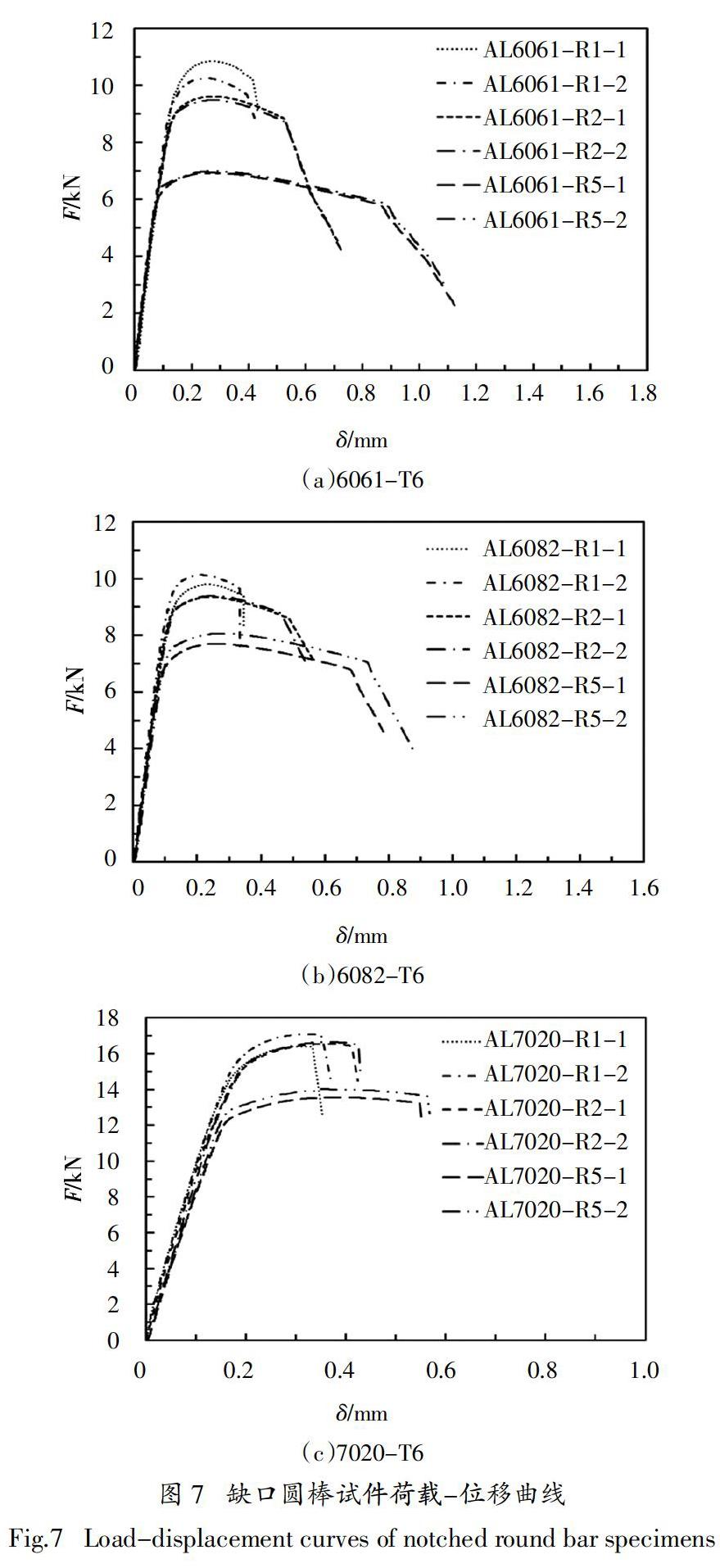
摘   要：为了将空穴增长模型（VGM）和应力修正临界应变模型（SMCS）应用于国产结构用铝合金的韧性断裂预测，完成了国产6061-T6、6082-T6和7020-T6 3种牌号的铝合金标准圆棒试件和缺口圆棒试件的单轴拉伸试验，并结合有限元分析，校准了3种牌号铝合金的VGM和SMCS模型断裂韧性参数. 研究结果表明：槽口半径大小对各牌号铝合金试件的断裂韧性参数校准值影响较小，离散系数均在20%以内;断裂韧性参数是铝合金材料固有属性，可用于国产结构用铝合金在不同应力状态下的韧性断裂预测;与SMCS模型相比，VGM模型能够更为精确地预测铝合金材料的韧性断裂.

关键词：铝合金;韧性断裂;VGM模型;SMCS模型;韧性参数校准

中图分类号：TU395                            文献标志码：A

Abstract：In order to apply the Void Growth Model （VGM） and Stress Modified Critical Strain model （SMCS） to predict the ductile fracture of Chinese structural aluminum alloys， uniaxial tensile tests on aluminum alloy 6061-T6， 6082-T6 and 7020-T6 were carried out， including smooth round bar specimens and notched round bar specimens. Besides， finite element analyses were conducted to calibrate the toughness parameters of three brands of aluminum alloys. The results show that the radius of the notch has slight influence on the toughness parameters of aluminum alloys， and the dispersion coefficients are all within 20%. It is indicated that the toughness parameter is an inherent attribute of the aluminum alloy material， and it can be used to predict the ductile fracture of Chinese structural aluminum alloys under various stress states. Compared with the SMCS model， the VGM model can predict the ductile fracture of aluminum alloys more accurately.

Keywords：aluminum alloy;ductile fracture;VGM model;SMCS model;toughness parameter calibration

铝合金材料具有轻质高强、可模性好、延展性好、耐腐蚀性好等优点，被越来越广泛地应用于现代结构工程领域，尤其是大跨度空间结构领域[1-2]. 断裂破坏是铝合金结构的一种主要失效模式，节点或构件的断裂失效可能导致结构发生整体倒塌[3]，因此，有必要对结构用铝合金的断裂行为进行深入研究.

铝合金的断裂行为属于典型的韧性断裂. 金属材料的韧性断裂理论从裂纹产生和发展的微观机制出发，可以较为准确地描述材料的宏观断裂行为，具有良好的适用性. 单调荷载作用下，应用较为广泛的断裂預测模型主要有Kanvinde等[4]提出的空穴增长模型（Void Growth Model，简称VGM）以及Hancock等[5]提出的应力修正临界应变模型（Stress Modified Critical Strain model，简称SMCS）. 基于上述模型，国内外学者对建筑钢材的断裂行为进行了广泛研究[6-15]，结果表明，单调荷载作用下，VGM和SMCS模型可以准确预测钢材的韧性断裂，并可应用于钢结构节点的断裂预测. VGM和SMCS模型在钢结构中的应用已较为成熟，而对于国内建筑结构常用的铝合金材料，其研究应用却几乎为空白. 铝合金与钢材相比，材料微观结构有所不同，其延性较差，韧性断裂行为可能会存在一定差别. 上述断裂预测模型能否有效应用于铝合金结构，还需进一步通过试验验证.

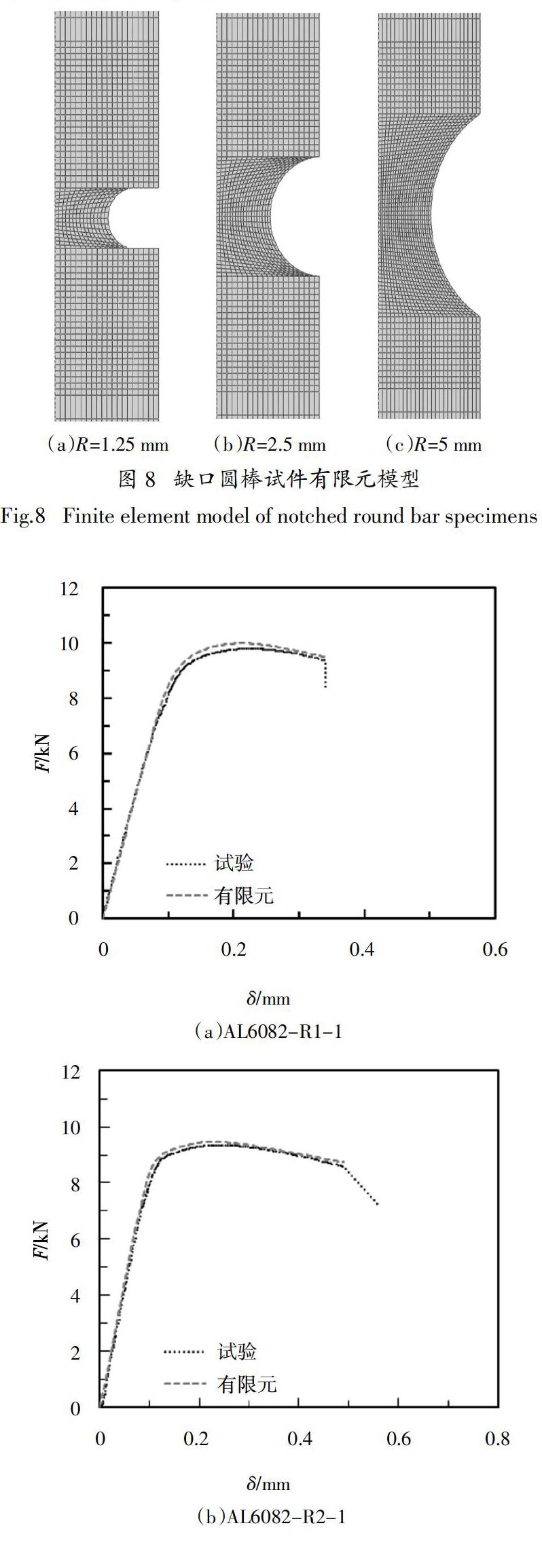
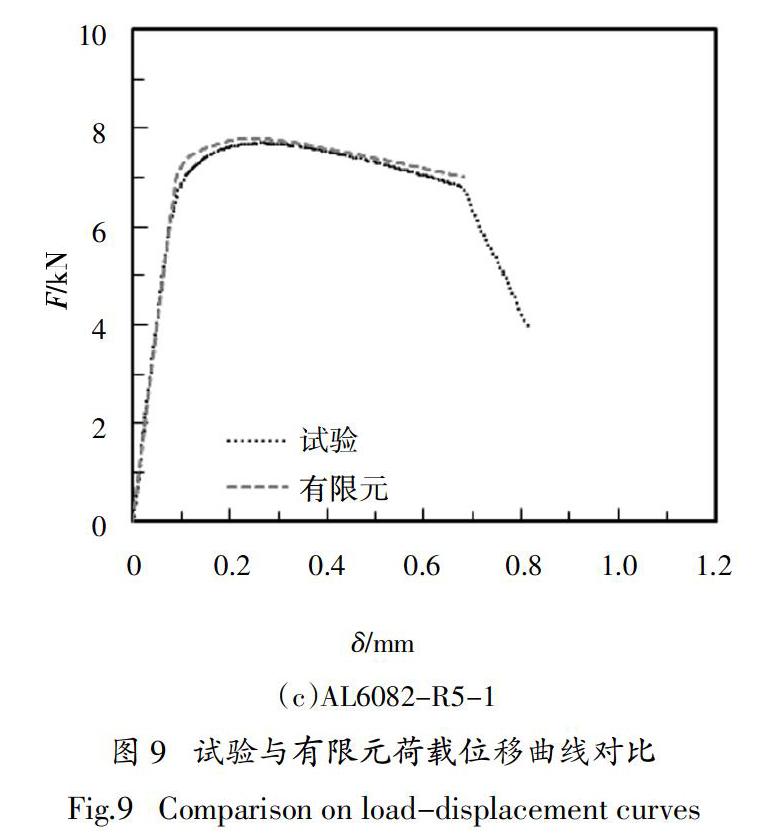
基于以上研究不足，本文选取国产6061-T6、6082-T6和7020-T6牌号的铝合金，加工了9个标准圆棒试件和18个缺口圆棒试件进行单轴拉伸试验，并结合有限元软件ABAQUS进行数值模拟，校准了不同牌号铝合金的VGM和SMCS模型韧性参数.

1   金属材料韧性断裂预测模型

Rice等[16]首先推导了单个圆柱形或球形空穴在理想弹塑性材料中的空穴增长公式，指出空穴半径增长率与应力三轴度和塑性应变有关. 其表达式如下：

式中：α为材料韧性参数，含义与VGM模型中的η类似;IF，SMCS为断裂指标，当金属材料在荷载作用下的等效塑性应变达到临界等效塑性应变，即断裂指标IF，SMCS≥0时，材料点发生启裂.

SMCS模型忽略了应力三轴度随等效塑性应变的变化，临界塑性应变取决于启裂时应力三轴度的值，而没考虑加载历史的影响. 因此，与VGM模型相比，SMCS模型计算精度稍差，不适用于材料塑性变形较大（即应力三轴度变化较为剧烈）的情况. 但在工程应用中，SMCS模型不需要积分，计算简便快捷，并且与试验结果吻合较好.

5）VGM和SMCS模型能够较好地预测国产结构用铝合金材料的韧性断裂，并且VGM模型具有更高的精度.

参考文献

[1]    沈祖炎，郭小农，李元齐. 铝合金结构研究现状简述[J]. 建筑结构学报，2007，28 （6）：100—109.

SHEN Z Y，GUO X N，LI Y Q. State-of-the-arts of research on aluminum alloy structures [J]. Journal of Building Structures，2007，28 （6）：100—109. （In Chinese）

[2]     杨联萍，韦申，张其林. 铝合金空间网格结构研究现状及关键问题 [J]. 建筑结构学报，2013，34（2）：1—19，60.

YANG L P，WEI S，ZHANG Q L. Aluminum reticulated spatial structures：state of the art and key issues [J]. Journal of Building Structures，2013，34（2）：1—19，60. （In Chinese）

[3]     郭小农，邹家敏，刘林林，等. 两种结构用铝合金循环加载试验研究[J].湖南大学学报（自然科学版），2018，45（9）：57—64.

GUO X N，ZOU J M，LIU L L，et al. Cyclic loading tests of two types of structural aluminum alloy [J]. Journal of Hunan University （Natural Sciences），2018，45（9）：57—64. （In Chinese）

[4]     KANVINDE A M，DEIERLEIN G G. Void growth model and stress modified critical strain model to predict ductile fracture in structural steels [J]. Journal of Structural Engineering，2006，132（12）：1907—1918.

[5]     HANCOCK J W，MACKENZIE A C. On the mechanisms of ductile failure in high-strength steels subjected to multi-axial stress-states [J]. Journal of the Mechanics & Physics of Solids，1976，24（2）：147—160.

[6]     KANVINDE A M，DEIERLEIN G G. Prediction of ductile fracture in steel moment connections during earthquakes using micromechanical fracture models [C]//13th World Conference on Earthquake Engineering. VANCOUVER B C. Canada：WCEE，2004：297.

[7]     KANVINDE A M，DEIERLEIN G G. Finite-element simulation of ductile fracture in reduced section pull-plates using micromechanics-based fracture models [J]. Journal of Structural Engineering，2007，133（5）：656—664.

[8]     MYERS A T，KANVINDE A M，DEIERLEIN G G. Calibration of the SMCS criterion for ductile fracture in steels：Specimen size dependence and parameter assessment [J]. Journal of Engineering Mechanics，2010，136（11）：1401—1410.

[9]     WANG Y Q，ZHOU H，SHI Y J，et al. Fracture prediction of welded steel connections using traditional fracture mechanics and calibrated micromechanics based models [J]. International Journal of Steel Structures，2011，11（3）：351—366.

[10]   邢佶慧，郭長岚，张沛，等. Q235B钢材的微观损伤模型韧性参数校正 [J]. 建筑材料学报，2015，18（2）：228—236.

XING J H，GUO C L，ZHANG P，et al. Calibrations of toughness parameters of microscopic damage model for steel Q235B [J]. Journal of Building Materials，2015，18（2）：228—236.（In Chinese）

[11]   刘希月，王元清，石永久. 基于微观机理的高强度钢材及其焊缝断裂预测模型研究 [J]. 建筑结构学报，2016，37（6）：228—235.

LIU X Y，WANG Y Q，SHI Y J. Micromechanical fracture prediction model of high strength steel and its weld [J]. Journal of Building Structures，2016，37（6）：228—235. （In Chinese）

[12]   廖芳芳，王睿智，李文超，等. Q460钢基于微观机制的延性断裂判据研究 [J]. 西安建筑科技大学学报（自然科学版），2016，48（4）：535—543，550.

LIAO F F，WANG R Z，LI W C，et al. Study on micro mechanism-based ductile  fracture criteria for Q460 steel [J]. Journal of Xi'an University of Architecture & Technology （Natural Science Edition），2016，48（4）：535—543，550. （In Chinese）

[13]   王元清，关阳，刘明，等. 建筑钢材微观损伤模型的韧性参数校正 [J]. 天津大学学报（自然科学与工程技术版），2018，51（S1）：1—9.

WANG Y Q，GUAN Y，LIU M，et al. Toughness parameter correction of microstructure damage model of building steel [J]. Journal of Tianjin University （Science and Technology），2018，51（S1）：1—9. （In Chinese）

[14]   王伟，廖芳芳，陈以一. 基于微观机制的钢结构节点延性断裂预测与裂后路径分析 [J]. 工程力学，2014，31（3）：101—108，115.

WANG W，LIAO F F，CHEN Y Y. Ductile fracture prediction and post-fracture path [J]. Engineering Mechanics，2014，31（3）：101—108，115. （In Chinese）

[15]   施刚，陳玉峰. 基于微观机理的Q460钢材角焊缝搭接接头延性断裂研究 [J]. 工程力学，2017，34（4）：13—21.

SHI G，CHEN Y F. Investigation on the ductile fracture behavior of Q460 steel fillet welded joints based on micro-mechanics [J]. Engineering Mechanics，2017 ，34（4）：13—21. （In Chinese）

[16]   RICE J R，TRACEY D M. On the ductile enlargement of voids in triaxial stress fields [J]. Journal of the Mechanics and Physics of Solids，1969，17（3）：201—217.

[17]   JIA L J，KUWAMURA H. Ductile fracture simulation of structural steels under monotonic tension [J]. Journal of Structural Engineering，2014，140（5）：04013115.

[18]   RAMBERG W，OSGOOD W R. Description of stress-strain curves by three parameters[R]. Washington D C：University of Washington. National Advisory Committee for Aeronautics，1943：56—58.

[19]   王丽，郭小农，刘林林，等. 国产铝合金材料滞回本构模型研究[J]. 湖南大学学报（自然科学版），2018，45（11）：29—36.

WANG L，GUO X N，LIU L L，et al. Study on hysteretic constitutive model of domestic aluminum alloy [J]. Journal of Hunan University （Natural Sciences），2018，45（11）：29—36. （In Chinese）

[20]   石亦平，周玉蓉. ABAQUS有限元分析实例详解 [M]. 北京：机械工业出版社，2006：166—167.

SHI Y P，ZHOU Y R. Finite element analysis and example explanation of ABAQUS [M]. Beijing：China Machine Press，2006：166—167. （In Chinese）