Numerical investigation on the drag force of a single bubble and bubble swarm *
2020-04-02LingxinZhangZecaiZhouXuemingShao
Ling-xin Zhang, Ze-cai Zhou, Xue-ming Shao
Department of Engineering Mechanics, Zhejiang University, Hangzhou 310027, China
Abstract: The bubble drag force correlation plays an important role in the numerical simulation accuracy of gas/liquid flows. In order to systematically investigate the interphase drag force of non-buoyancy driven bubbly flows, a dynamic-positioning body force (DPBF)method is developed in this study. It is proved that this method has an enough computation precision. Using this method, a series of direct numerical simulation (DNS) cases of a single bubble with low-intermediate Re(1≤Re≤200) and a bubble swarm with low Re(5.6≤Re≤45) are carried out and the bubble drag coefficients are calculated. Based on all the DNS data, the drag correlations with dimensionless parameters (Re, We for a single bubble and Re, We, gas fraction for bubble swarm) are systematically investigated and reported in this paper, which can provide a reference to the development of drag force closure model for non-buoyancy driven bubbly flows.
Key words: Gas/liquid flow, bubble, direct numerical simulation, drag force
Introduction
Gas/liquid flows exist widely in nature and are applied widely in engineering. Computational fluid dynamics (CFD) has become an important technical means for investigating the two-phase flow characteristics. Depending on the different resolution scales,three numerical approaches are mostly used to simulate gas/liquid flows: direct numerical simulation(DNS), Euler-Lagrangian (E-L) simulation and Euler-Euler (E-E) simulation[1-3]. On the three approaches, the resolution scales increase and the computation costs decrease in turn. Limited by the current computation ability, DNS is still unrealistic for a large scale gas/liquid flow simulation. Thus, compared with DNS, the E-L and E-E simulation have wider applications in engineering[4-6]. As an important term of the interphase force in the E-E and E-L approach, the drag force closure model has an enormous influence on the simulation accuracy of gas/liquid flows.
The bubble drag correlation with dimensionless parame- ters such as Re, Eo, Mo and gas fraction has been investigated by many researchers for buoyancy driven bubbly flows. For a single bubble rising in stagnant liquid, Moore[7], Mei et al.[8]proposed drag models containing Re for spherical bubble. Grace[9], Ishii and Zuber[10], Dijkhuizen et al.[11]investigated the drag correlation with Re and Eo for a deformed rising bubble. A widely used drag closure model was presented by Tomiyama et al.[12].His drag model consists of three parts: slightly contaminated and contaminated systems. Lain et al.[13],Zhang and VanderHeyden[14], Murray et al.[15]also carried out some related work on the dynamics of a rising bubble. For rising bubble swarms, considering the effect of Re and gas fraction, Grevskott et al.[16]presented a drag closure model and applied it in an E-E simulation. Roghair et al.[17]developed a closure model with considering Re, Eo and gas fraction for rising mono-disperse bubble swarms at intermediate and high Re and refined their correlation to make it applicable for a large range of bubble sizes[18].Some relevant works on the drag force of bubble swarms were also carried out by Rusche and Issa[19],Simonnet et al.[20].
However, for non-buoyancy driven bubbly flows,such as the high velocity gas jet in liquid and the bubble swarms transportation in cloud cavitation, the bubble driven force is mainly from the liquid surface force rather than the gravity. When the time scale of the transportation process is very small, the bubble motion driven by gravity can even be ignored. In this case, those drag correlations containing Eo and Mo are no longer applicable. In this study, based on the DNS data, the bubble drag force correlation is systematically investigated by considering both Re,We as well as the gas fraction.
1. Numerical method and validation
For non-buoyancy driven bubbly flows, if bubbles move freely with liquid, it is difficult to obtain the steady interphase drag force characteristics and set the bubble dimensionless parameters accurately. In this paper, a simulation method for fixed bubble flow based on dynamic-positioning body force (DPBF) is proposed. By applying the DPBF, the position of the bubble volume-centre can be fixed, but the bubble deformation is not affected. Based on this method, the drag force of a single bubble or bubble swarm can be studied systematically in a large parametric range.
The general form of the DPBF is proposed as follows

In the present study, the fluid is considered to be incompressible. Based on the volume of fluid (VOF)method to capture the two-phase interface and the finite volume method to discretely solve the control equation, the direct numerical simulations of single bubble and bubble swarm are carried out.
Figure 1 shows the output of DPBF, displacement and velocity of a single bubble with Re=200 and We=4. It can be seen that the output of DPBF finally reaches a stable state, and the bubble displacement and velocity both converge on zero. This shows that the bubble is stably fixed in the target position and the control performance can meet the calculation requirements.
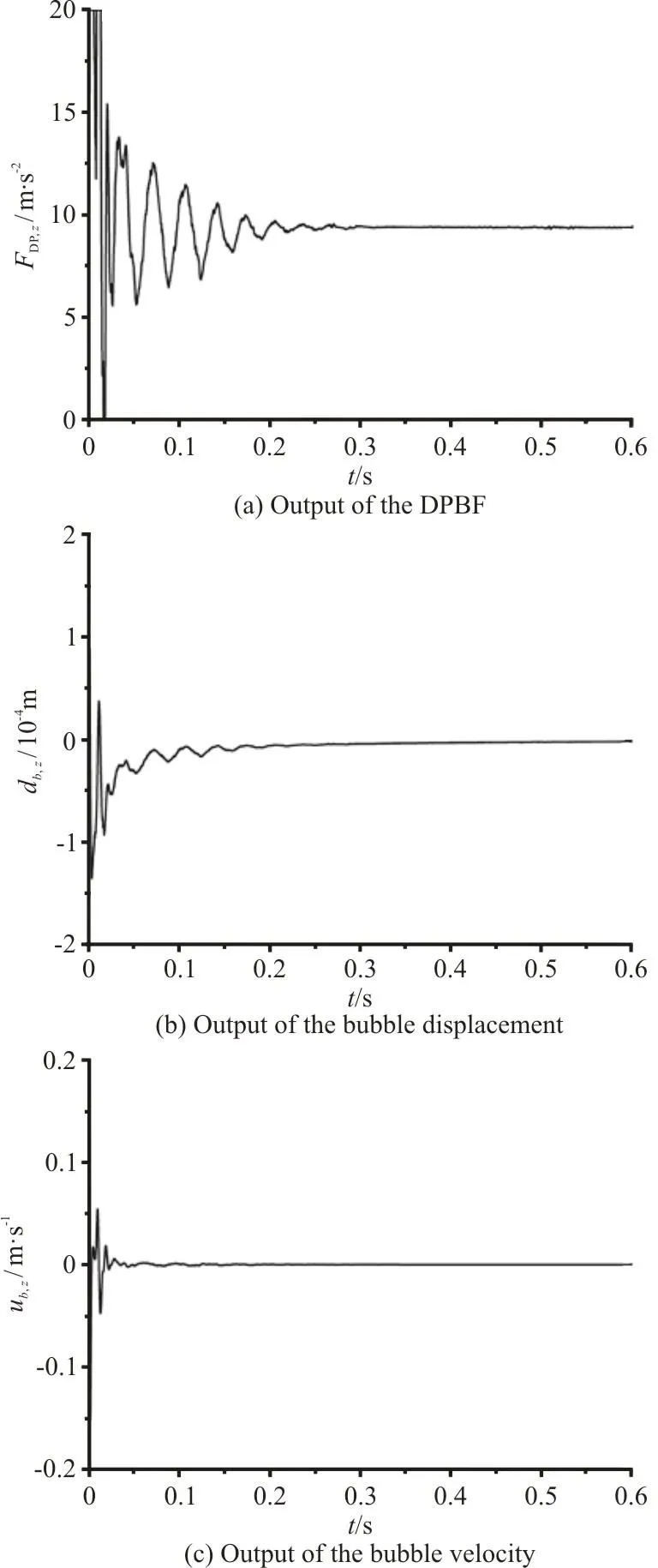
Fig. 1 Output of the DPBF, bubble velocity and bubble displacement in the flow direction with Re=200 and We=4
In this paper, the numerical simulation of the bubble swarm has applied a multi-marker VOF method. Each mixture of bubble and liquid is individually marked as a phase fraction so that an individual DPBF control can be applied to each bubble in a swarm. Figure 2 shows the DPBF output of 40 bubbles in a bubble swarm with Re=45,We=0.63 and gas fraction α=0.203. It can be seen that the DPBF control for each bubble converges to a steady state eventually. Therefore, the DPBF control is robust in the numerical simulation of bubble swarm.
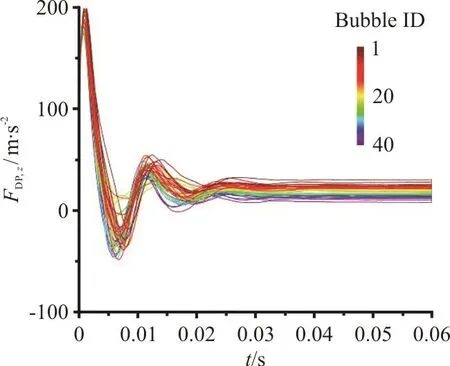
Fig. 2 (Color online) The DPBF output of 40 bubbles in a bubble swarm with Re=45, We=0.63 and α=0.203
Through time-averaging the output of DPBF, the bubble drag coefficient is calculated as follows

With different Re, bubble drag coefficients calculated by this paper as well as Ryskin and Leal[22]are compared as shown in Fig. 3. It can be seen that the two are consistent and the error is within an acceptable range, which validates the calculation accuracy of the DPBF method in this paper.
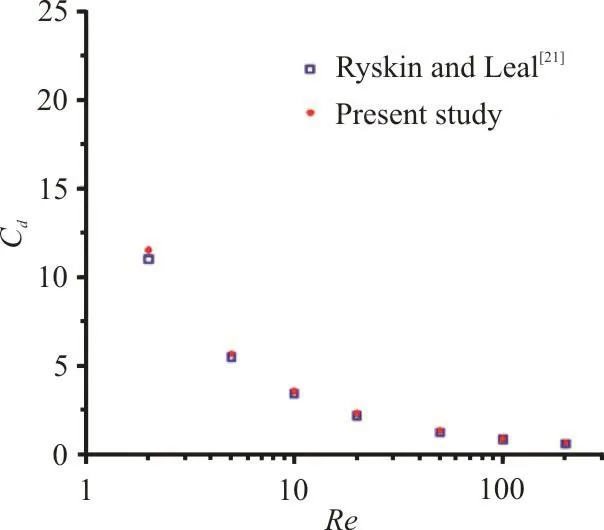
Fig. 3 (Color online) Comparison of the bubble drag coefficients calculated by present study with Ryskin and Leal[21] in different (=4)ReWe
2. Drag correlation of single bubble
Using the DPBF method, the direct numerical simulation of a single slipping bubble in a pure liquid is carried out. The calculation parameter settings are shown in Table 1. Given the bubble slip velocity and bubble equivalent diameter, Re and We are independently adjusted by changing the liquid viscosity and surface tension respectively.

Table 1 Parameter settings of the single bubble simulation
Figure 4 shows the velocity field of a single bubble for different Re with We=2. Uzis the liquid velocity in the flow direction. It can be seen that the bubble deforms slightly from spherical to ellipsoidal as Re increases. The bubble has a larger wake scale with higher Re. This indicates that the bubble wake decays more slowly with higher Re.Due to the slipping of gas-liquid interface, there is a significant difference between the flow around a gas bubble and a solid sphere with a no-slip boundary.Compared with a solid sphere with the same Re, the flow separation is significantly delayed and the bubble wake is more stable. In fact, when Re increases to 200, the bubble wake is separated but is still stable.Figure 5 shows the velocity field for different We with Re=20. As We increases from 2 to 10, the bubble deforms from nearly spherical to ellipsoidal to dish-shaped finally. Basically, the larger the We, the flatter the bubble shape. This deforma- tion obviously increases the projected frontal area of the bubble,which will increase the drag force.
All of the bubble drag coefficients calculated by DNS are shown in Fig. 6. Within the parameter range studied in this paper, the single bubble drag force coefficient Cdis negatively correlated with Re and positively correlated with We. With different We,the change of Cdfollows a similar trend as Re increases. With Re=1, the drag coefficient of the spherical bubble is close to the analytical solution Cd=16/Re.

Fig. 4 (Color online) The flow direction velocity field of single bubble with We=2
3. Drag correlation of bubble swarm

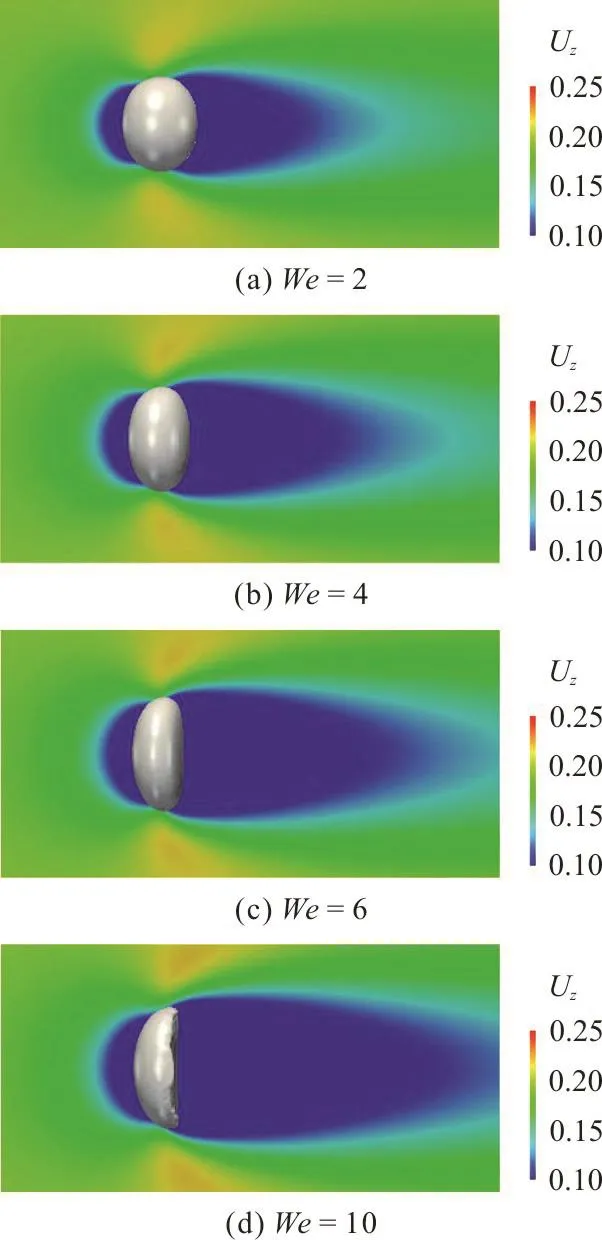
Fig. 5 (Color online) The flow direction velocity field of single bubble with Re=20

Fig. 6 The drag coefficients of a single bubble with incresing Re


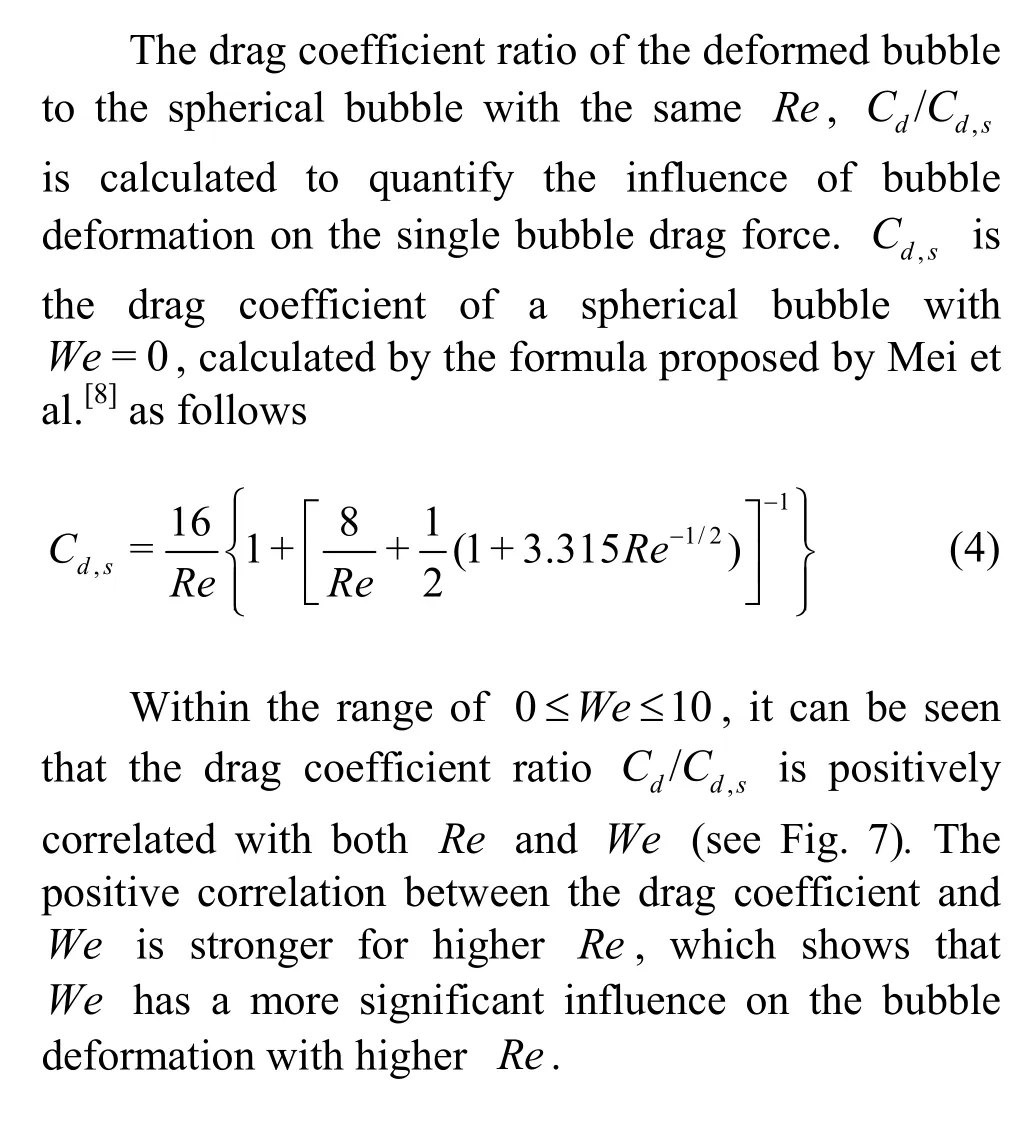
Fig. 7 The drag coefficient ratios of a deformed bubble to a spherical bubble with incresing We
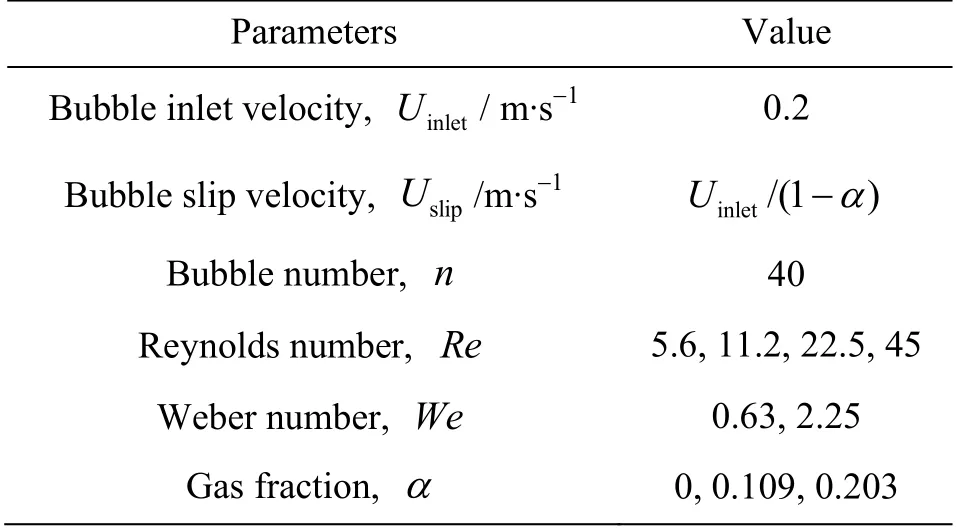
Table 2 Parameter settings of bubble swarm simulation

Fig. 8 (Color online) Flow direction velocity field of spherical and ellipsoidal bubble swarm with Re=11.2 and α=0.109
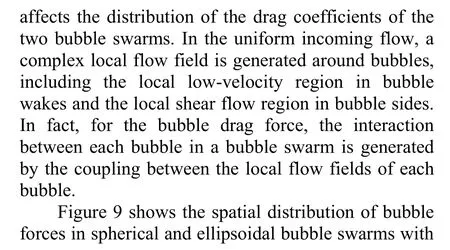

where rbis the bubble equivalent radius.

Fig. 9 (Color online) Forces on each bubble in bubble swarmwith Re=11.2 and α=0.109
Compared with the spherical bubble swarm, it can be seen that the bubbles force direction in the ellipsoidal bubble swarm is more inconsistent. This is because ellipsoidal bubbles are relatively more susceptible to de formed under the flow shear effect.
For a large-scale bubbly flow with an interphase slip velocity, the numerical solution of the continuous phase under the Euler framework requires a control volume that satisfies the space average requirement.In the direct numerical simulation of a bubble swarm,a cubic space in the middle position is taken for the control volume in this study. The averaged drag force coefficient of bubble swarm in the control volume Vcis calculated as follows
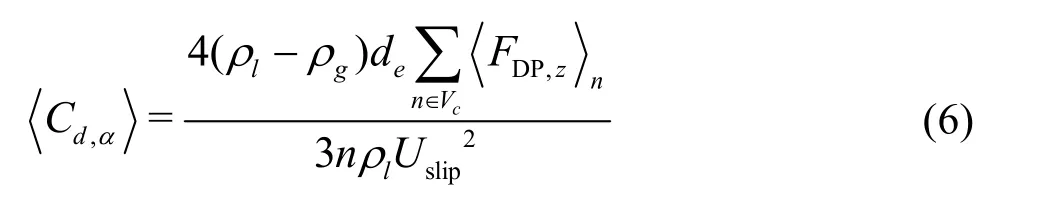


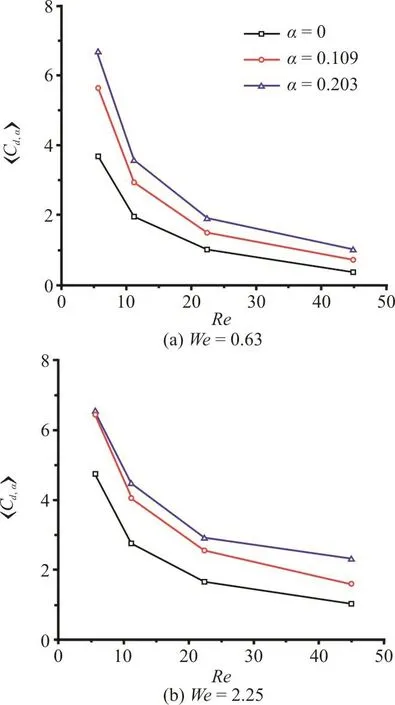
Fig. 10 (Color online) The average drag coefficients of bubble swarm
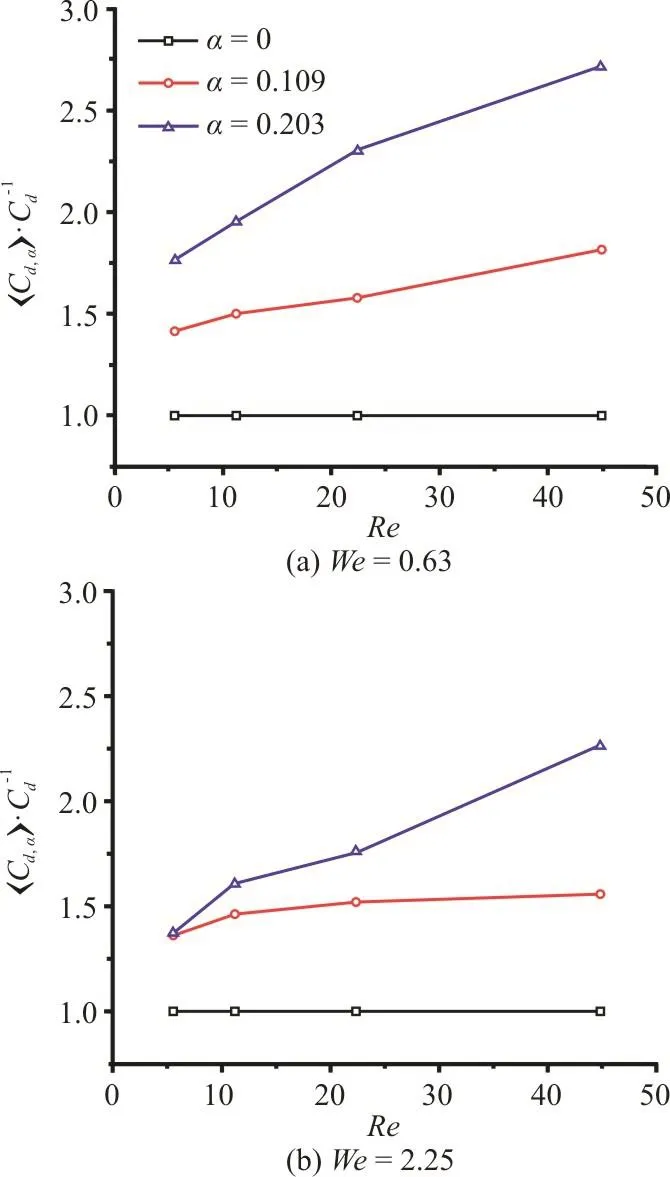
Fig. 11 (Color online) The ratios of averaged drag coefficient of bubble swarm to the drag coefficient of single bubble with same Re and We
4. Conclusions
Based on a dynamic-positioning body force method, the drag force coefficients of a single bubble with low-intermediate Re and bubble swarm with low Re are investigated through direct numerical simulation and the drag force correlation with dimensionless parameters including Re, We and gas fraction is analyzed in this study.


These qualitative conclusions of drag force correlation obtained in this paper can provide references to the drag model development of the non-buoyancy driven bubbly flow. Admittedly, the investigated Re range of bubble swarm in this study is relatively low at present, which needs to be further expanded in the future.
杂志排行
水动力学研究与进展 B辑的其它文章
- Liutex core line and POD analysis on hairpin vortex formation in natural flow transition *
- Numerical study on the bubble dynamics in a broken confined domain *
- Flow characteristics in open channels with aquatic rigid vegetation *
- A study of the evolution of nanoparticle dynamics in a homogeneous isotropic turbulence flow via a DNS-TEMOM method *
- Local scour by multiple slit-type energy dissipaters *
- Liutex-based vortex dynamics: A preliminary study *
