Dynamics response analysis of airborne external storage system with clearance between missile-frame

Yongfeng YANG, Qingyng ZHENG, Jinjun WANG, Zhongfu MA,Xingqiu LIU

a Department of Engineering Mechanics, Northwestern Polytechnical University, Xi’an 710072, China

b Key Laboratory of Vibration and Control of Aero-Propulsion System Ministry of Education, Northeastern University,Shenyang 110819, China

c China Airborne Missile Academy, Luoyang 471099, China

KEYWORDS Airborne;Dynamic response;Finite element method(FEM);Missile-frame;Random vibration

Abstract Considering the aircraft and its external components are subjected to complex and variable aerodynamic loads during the working process, the missile-frame clearance system of the airborne external missile is investigated.The random vibration characteristics of the airborne external components are analyzed by finite element method.The finite element model is optimized with reference to the test results, and the effects of different clearance on the dynamic response of the missile-frame system are compared.The result shows that the frequency response curves of the same position and the resonant peak frequencies are consistent under different clearances. The acceleration response at both ends of the missile is large and the amplitude near the center of mass is gentle.The results can be used to predict reasonable missile-frame clearance and make guidance to the structural design and reliability analysis of the missile-frame system.

1. Introduction

During the flight, the external airflow load of the aircraft is extremely complicated.Under the combined action of different types of loads such as lift, drag and self-gravity, the aircraft shows a high degree of complexity.1-4Stability and safety performance of aircraft is determined by reliability of aviation airborne equipment, airborne equipment mounting rack work under severe mechanical vibration.5,6

For random vibration of airborne platform, the accurate evaluation is the key indicator to ensure normal operation of airborne equipment during flight.7The flutter problem of missile caused by airflow disturbance, random load or eccentric thrust of missile startup has one thing in common: random excitation. Fatigue life, stability and performance of majority of the structures and systems depend significantly on dynamic loadings applied on it.8-12Modern aircraft attaches great importance to the problem of vibration environment, in the process of aircraft operation, it will experience more complex vibration excitation, especially random vibration excitation.Random vibration is thought of as the random motion of a structure excited by a random input.The mathematical theory of random vibration is essential to the realistic modeling of structural dynamic systems.13Li et al.14proposed an algorithm that integrates Karhunen-Loeve Expansion (KLE) and Finite Element Method(FEM)to carry out random vibration analysis of complex dynamic systems excited by stationary or nonstationary random processes.Zhu and Cai15proposed an analytical method to study the response of a viscoelastic system with strongly nonlinear stiffness force and under broad-band random excitations.Qin et al.16improved the empirical formulation with three-dimensional finite element model, and the improved formulation can accurately predict the load capacity of marman clamps. Chen CL and Chen LW17employed the finite element model to investigate the mean-square response and reliability of a rotating blade with external and internal damping under stationary or non-stationary random excitation.Qiu et al.18investigates the axial-torsional coupled vibration of a drill-string under combined deterministic and random excitations.Finite element method(FEM)is used to model the system. Considering the effect of band clamp band, the dynamic model for the launching system proposed can be conveniently used to evaluate the system dynamics and the influence of the clamp band joint.19Based on the random vibration of aviation airborne equipment, Zhang et al.5established accurate parametric finite element model, obtained modal of frequency and vibration by analysis.

For the rigid launcher, the clearance is positively related to the lateral displacement deviation of the missile. However, as the clearance decreases, the frequency of vibration coverage increases and the change of vibration is aggravated.20,21For the flexible launcher, when the clearance is too large, the coupling constraint between the missile-frame is relatively reduced,and the projectile body will have a large displacement and deformation.22Casini and Vestroni23studied the twodegree-of-freedom piecewise linear system and analyzed its modal frequency, mode shape and bifurcation conditions. In the field of weak nonlinearity, Butcher24obtained the nonlinear modes of a single-degree-of-freedom bilinear stiffness system by using the perturbation approximation of the modal parameters of the linear system. In large amplitude strongly nonlinear vibration systems, Pierre et al.25based on the concept of invariant manifold theory, the modal information of system is obtained by solving partial differential equations by Galerkin method. Aykan and Mehmet26compared fatigue cumulative damage under uniaxial and multiaxial loads.According to the relationship between the excitation power spectral density matrix and the response power spectral density matrix of linear system, the dynamic response of the system is calculated in frequency domain. Wu et al.27based on a group of 7075-T651 aluminium alloy specimens, tested the practicability of the fatigue damage and fatigue life estimation method under random load, and verified the method through a series of low cycle fatigue test results.A combination of the Effective Strain Damage (ESD) model based FORTRAN code with finite element software was proposed to predict fatigue life under random loadings.28A standard gradient-based algorithm with line search, Jensen et al.29presents an approach for solving reliability-based optimization problems involving structural systems under stochastic loading.Moon and Yoon30developed test specifications for components, which are applicable to predict fatigue life at the stage of initial product design, for the unit brackets by using a vibration fatigue technique. Fatigue life forecast of airborne equipment during product design, based on fatigue numerical analysis of wide band random vibration, was brought out by Wang and Zhang31to ensure the rate of success at first time of projects. Zaitsev32proposed a method for recounting the value of the specified unit service life with regard for only vibration effect.

In this paper, in order to avoid the adverse effects of the non-linear behavior of the airborne external storage on the working state of the missile, the dynamic response of an airborne external storage missile-frame system under complex external loads is studied. The vibration characteristics of the airborne external storage are analyzed by finite element calculation and ground test simulation, and the effects of different clearance sizes on the dynamic response of the missile-frame system are compared. The results can be used to predict reasonable missile-frame clearance and guide the structural design and reliability analysis of the missile-frame system.

2. Analytical method and modeling

This chapter used the conventional finite element analysis method33and the finite element software ANSYS as the platform according to the above analysis.Depending on the simulation process shown in Fig. 1, the finite element model of the missile-frame clearance system is established and analyzed,and its dynamic response under random excitation is studied.This result can be used to guide subsequent experimental studies.

The three-dimensional model of missile-frame system is built on CATIA platform. In order to facilitate subsequent meshing, unit definition, and post-processing, this section will independently model each component of the missile-frame system. Finally, the whole geometric model for finite element analysis is obtained by assembling all the components. Fig. 2 show the three-dimensional geometric model of each component which includes four parts: missile body, pylon, guide rail and suspension.

The research object is an airborne missile and its suspension device, in which the materials used for the pylon and the suspension components are steel.The missile body is composed of steel and titanium alloy. The specific parameters of the material are shown in Table 1. The distribution of the material of the projectile is shown in Fig. 3, in which the material at both ends of the projectile is steel and the middle part is titanium alloy.

When calculating contact problem with finite element method, it is often necessary to consume a large amount of computing resources, and it is difficult to obtain effective results. Based on the above two problems, it is necessary to establish a reasonable and effective contact model on the basis of a clear physical model.

Fig. 1 Finite element analysis process.

Fig. 2 Three-dimensional geometric model.

Table 1 Material composition and density of each component.

In this chapter, CONTA174 and TARGE170 elements will be selected as contact elements in ANSYS for contact analysis.Among them, the CONTA174 element is a three-dimensional 8-node surface-surface contact element, which can analyze the contact and slip state of the flexible surface and is suitable for three-dimensional structural analysis. In the geometric model, the rigid rail components and part of the surface of the pylon components belong to the target surface. Combine the grid elements of each component to get the full finite element mesh of the whole system, as shown in Fig. 4.

In this paper, three-dimensional solid elements are used to mesh the structure. After the conical like structure of the warhead is cut, the tetrahedron element is used to mesh the grid,and the remaining cylinder structure is meshed into hexahedron element by sweeping.After refining the grid,the structure contains 630407 nodes and 5412636 elements.

3. Finite element simulation results

Each analysis module of the finite element simulation can obtain the relevant calculation results and have selected output parameter variables as needed. These variables are important feedback information and references to the rationality of the finite element model.The main significance of structural static analysis is to provide prestressing conditions for subsequent calculations.

3.1. Modal analysis

The natural frequencies of the first 9 modes of the system (as shown in Table 2) and their mode shapes (as shown in Fig.5)are extracted.According to the results,the first 6 modes of the system are purely curved modes in all directions,and the fundamental frequency is about 19 Hz. The remaining modes are coupled modes involving bending and torsion.

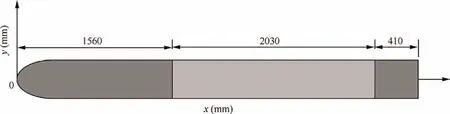
Fig. 3 Projectile material distribution diagram.

Fig. 4 Integral element mesh generation.

Table 2 Natural frequencies of the first 9 modes by FEM.

Fig. 5 Mode shapes of the first 9 orders by FEM.

By observing the sixth to ninth mode modes, it can be found that the deformation is particularly remarkable because the geometric model of the project body retains only the structure of the shell thickness.The mode shapes are also very complicated,and the modal shapes of bending and torsion exhibits a high degree of irregularity. It can be concluded that torsion has a great influence on the bending deformation of the projectile under this geometric structure, which means that torsion will also have a great impact on the calculation of random vibration spectrum,which may lead to the deviation of the calculation results.

3.2. Random vibration analysis

Fig. 6 Straight spectrum load diagram.

Fig. 7 Waterfall map of flat spectrum response curve by FEM.

In this section, the ’Acceleration Power Spectral Density of Gravity (G Acceleration PSD)’ is used to define and load the excitation load. After the convergence is calculated, the ’G Acceleration PSD’ response curve of the system structure is also output. The reference point of the output is selected on a geometric node at the tip, center and end of the projectile.The selection of the clearance value samples includes three types of 0.08 mm, 0.2 mm and 0.4 mm, which correspond to clearances from small to large. The main content of finite element analysis is to study the frequency domain response (G acceleration response) of the missile-frame system samples with different clearance values under the random excitation.Analyze the relationship between the peak frequency and amplitude of the acceleration response at each reference point to obtain the clearance, and the influence of clearance size on the dynamic response of the system is obtained.

In this paper, the G acceleration power spectral density commonly used in engineering is selected.The frequency range is 20-2000 Hz, and the corresponding amplitude of each frequency is the same 0.02 g2/Hz. This is the simplest random excitation spectrum transmitted by the body. As shown in Fig. 6, Wfis power spectral density and f is the frequency,the amplitude-frequency curve is a horizontal straight line,referred to as the straight spectrum.

The random vibration calculation is performed. Reference points are selected on the tip, centroid and end section of the projectile respectively, and the G acceleration response curves and the peak point data on these reference points are output.Fig.7 shows the frequency domain acceleration response comparison curves of all reference points of each sample under the excitation of flat spectrum. For the sake of comparison, the response frequency of less than 200 Hz is ignored.

By analyzing the acceleration response under random excitation, it can be found that the curves of the three different clearances have similar trend.The tip has a significant formant and two smaller formants, and both the centroid and the end contain a significant and a small formant. The frequency of the resonance peak has little differences under different clearances.

According to the formant statistics in Table 3, the main peak of the tip appears near 25 Hz, the main peak of the centroid appears near 78 Hz, and the main peak of the end appears at about 45 Hz. In terms of amplitude, the response of the tip and end of the first-order peak is larger, while the response of the centroid is much smaller. From the point of the clearance, with the increase of the clearance, the acceleration response between the two ends and the centroid is just the opposite with the change of clearance.

Table 3 Formant parameters of Straight spectrum response by FEM.

4. Vibration experiment and model optimization

4.1. Experimental result

Airborne missile flying vibration is one of the main dynamic environments that the missile endured in service. Due to its high vibration intensity, complex type and long duration, it has significant influence on the structural reliability of the airborne plug and the attitude of the missile. The actual flight environment test is quiet difficult and extremely expensive. It becomes an indispensable and effective method that simulates the flight vibration environment of airborne missile on the ground.

The test only considers the vibration transmission load of the body.The loading position is located on the upper surface of the pylon, and is connected to the vibration table by two vibration fixtures, so that the missile-frame is fixed on the vibration table by the top suspension. As shown in Fig. 8,make ensure that the center of the system is on the same vertical line with the center of the vibration table.The load application position is fixed at the two vibration fixtures, and the acceleration sensors are respectively fixed at the fixture, the projectile tip,middle and end of the body,to monitor the input of the load and the output of the vibration response of each point.

The load curve is input to the random vibration exciter,and the vibration signal is applied to the pylon in the vertical direction through the transmission mechanism.The missile is forced to vibrate under the driving of the pylon.The test samples consist of 0.08 mm, 0.2 mm and 0.4 mm clearance size missileframe system,and the acceleration response data of each measuring point is counted and output in the data acquisition system.

Fig. 8 Schematic diagram of missile suspension fixed mode.

According to the formant statistics, no matter the size of the clearance value and the selection of the reference points,the corresponding frequencies of the first-order peak in the acceleration frequency response curve is between 25 Hz and 30 Hz. The second-order peak frequencies vary greatly, the corresponding frequency of the tip position is at the range of 110 Hz to 120 Hz, and the centroid position is 45 Hz, while the end is 85 Hz or 115 Hz. According to the results of modal analysis, the second-order mode of the missile is the bending deformation of the two ends upward and the middle section downward. The deformation of the projectile is limited by the hanger constraint,so the frequency at the centroid is small and the second-order peak frequencies vary greatly.The thirdorder peaks are quite consistent, all of them are 195 Hz. The maximum amplitudes of the tip and the end are both at the first formant, while the centroid is at the second formant.From the angle of the clearance sample,the peak value of each reference point increases as the clearance increases, except the centroid.

4.2. Comparison of experiment and simulation

The acceleration frequency response curves of the finite element method and experiment of the same scheme are placed in the same curve,and the similarities and differences between them are compared, as shown in Figs. 9-11.

According to the comparison above, the curve trend of the simulated frequency response of each sample tip position fits well with the experimental results, and both have one large and two small formants. The simulation frequency response curves of the centroid and the terminal are both missing a resonance peak, and the order of the formants is not consistent with the experimental result. In addition, for the corresponding frequencies value of each order of formants, the finite element results are not consistent with the experimental results.

4.3. Finite element optimization result

Combined with the discussion of the modal analysis results in the finite element, only the three-dimensional geometry of the missile shell of the projectile is retained, which may cause the deformation form of the projectile has a large difference with the actual situation.Therefore,the missile’s geometry structure is optimized, and it is considered to be filled. The shell structure is changed to solid structure, as shown in Fig. 12.

Fig. 9 Comparison of simulation test of clearance 0.08 mm sample.

Fig. 10 Comparison of simulation test of clearance 0.2 mm sample.

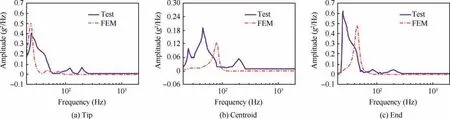
Fig. 11 Comparison of simulation test of clearance 0.4 mm sample.

Fig. 12 Finite element mesh of optimized model.

Table 4 Natural frequencies of the first 9 modes by optimizing.

Adjust the material density so that the quality of the missile geometry is consistent with the actual missile mass. A correction factor of 0.12 is given to achieve a relatively reasonable value for the system stiffness. The contact pair between the upper surface of the missile suspension and the lower surface of the pylon is adjusted from bonded to rough, and the actual clearance value corresponding to each clearance sample is given. This method can satisfy not only displacement constraints for static analysis but also free collision conditions in vertical direction.

The first nine order modal parameters are extracted, and the natural frequencies of each order are shown in Table 4.

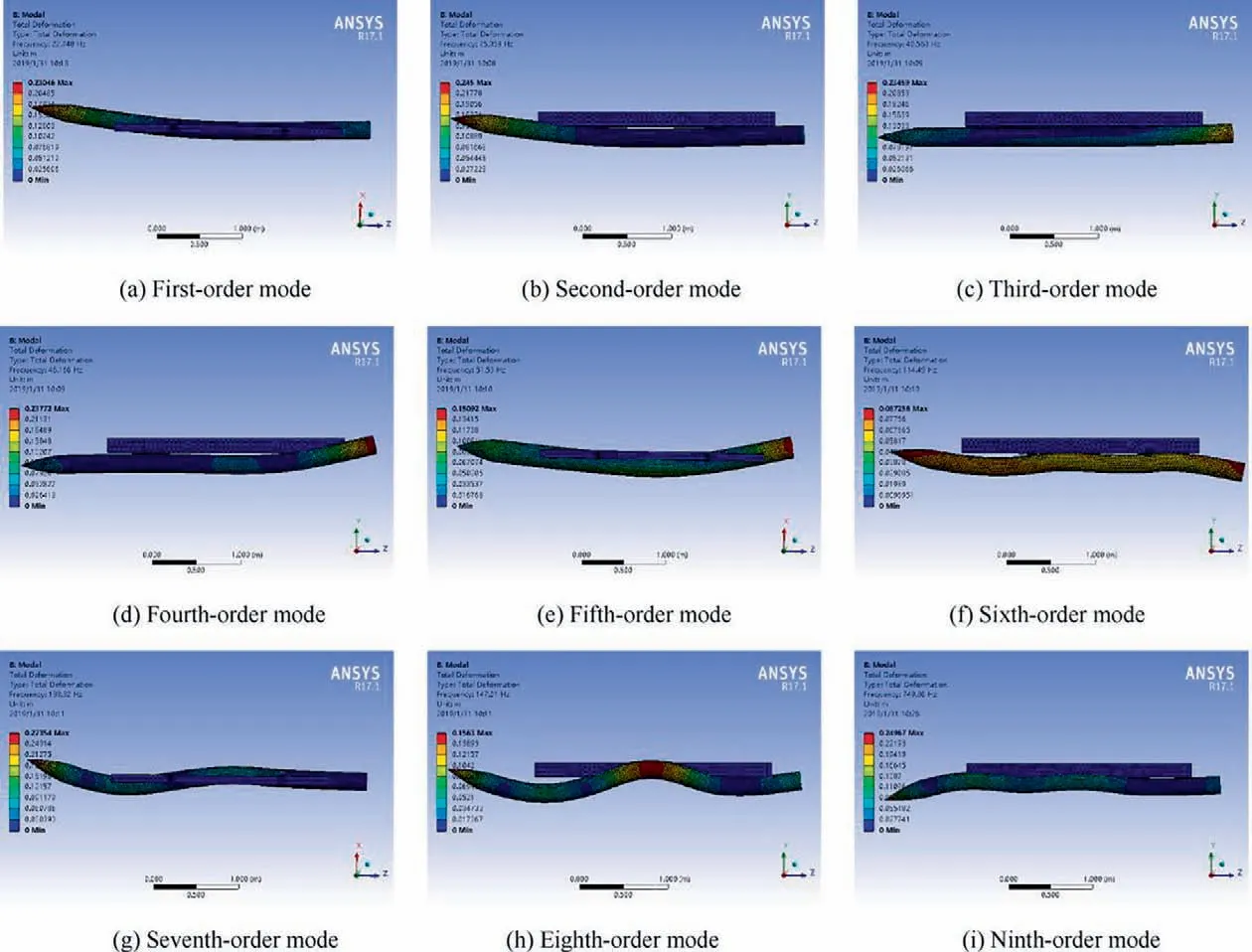
Fig. 13 Optimizing the first nine mode shapes of model.

Fig. 14 Comparison of clearance 0.08 mm sample.

The corresponding modes of each order are shown in Fig.13.It can be seen in the figure that the modes corresponding to the first nine modes are bending modes in all directions.Compared with the previous modal analysis results,the modal curves of the first nine modes reduce the influence of torsion effect on one hand, and are more closer to the bending vibration of simple beams on the other hand. The reliability of the results is greatly increased.

Figs. 14-16 show the comparison results of the frequency response curves of the reference points in the finite element method, the experiment and the optimization method.Compared with the original model, the optimized model captures more resonance peaks, and the curve form is closer to the experimental results. Combined with the frequency data of each order peak,there are more resonance frequency values between the optimization model and the experimental result, what’s more, the corresponding frequency of the main peak is basically consistent with the experimental result,which greatly improves the reliability of the finite element simulation.

Fig. 15 Comparison of clearance 0.2 mm sample.

Fig. 16 Comparison of clearance 0.4 mm sample.

5. Conclusion

Based on the random vibration problem of airborne external plug-in, the finite element random vibration analysis and suspension vibration test are used to describe the dynamic response of the airborne external system with clearance. The credible finite element model is established and the rationality of finite element simulation is verified by the suspension vibration test,which provides reference and guidance for the design of airborne missile suspension clearance.

(1) According to the analysis of the experimental and optimized model results, under the excitation spectrum,the amplitude increases with the increase of the gap,and different excitation spectra have influence on the results. It is found that the frequency response curves of the same position are consistent under different clearances, and the frequency of each formant is basically stabled.

(2) The acceleration response at both ends of the missile is large and the amplitude value near the centroid is gentle.

(3) The finite element model is optimized by comparing the frequency response curve and the formant parameters.The optimized model greatly improves the ability to capture the formant, and the main peak frequency of each order is highly fit.

Furthermore, for the model simplification problem in both the internal structure of missile and the guide rail, we are working on establish an accurate model to deal with it.Reducing the difference between simulation and experimental results is a key point in our future work.

Declaration of Competing Interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.

Acknowledgements

This study was funded by National Natural Science Foundation of China (No. 11972295), the Key Laboratory of Vibration and Control of Aero-Propulsion System Ministry of Education, Northeastern University (No. VCAME201803),Aeronautical Science Foundation of China (Nos.20182953025, 2016ZD12032), Graduate Innovation Fund of Northwestern Polytechnical University (No. ZZ2019126).