Yield-density effects on growth and biomass partitioning in Leucaena leucocephala seedlings

Tongtong Zhou·Li Xue

Abstract Experiments were conducted to study the effects of density on growth and biomass partitioning of Leucaena leucocephala seedlings.Four plantations with densities of 10,000,20,000,40,000,and 80,000 seedlings ha-1 were evaluated only from 15 to 25 months after planting.At 15 months, crown height and width decreased with increasing density.Seedling height/dbh ratios increased with increasing density.Biomass increased with greater density according to the yield-density effect equation,which was evident for all densities.With increasing age,biomass division to branches and leaves increased,whereas partitioning to roots decreased in the 10,000 and 20,000 seedlings ha-1 plantings.Partitioning to branches and leaves remained relatively steady,while partitioning to roots increased in the 40,000 and 80,000 seedlings ha-1 plantings.Biomass division into stem and bark components remained relatively steady in all densities.Yield-density and organ yield-density curves shifted upward with increasing seedling age on a log-log graph throughout the experimental period.

Keywords Competition·Leucaena leucocephala seedlings·Yield-density effect·Biomass partitioning

Introduction

As seedlings grow larger,growth is constrained by available resources.Thus,there is competition among plants which decreases average plant mass with increasing density,whereas yield(mean plant mass×density)increases with increasing density(Xue and Hagihara 2008a).Several studies have examined the relationship between yield and stand density as this relationship has long been regarded as important from both theoretical and practical viewpoints(Xue and Hagihara 2008a).

Forest biomass is composed of different tree organs or components-stem or trunk,branches,leaves,and roots(Lie and Xue 2016).Biomass partitioning is affected by competition between plants.This results in differences in organ growth(Cahill 2003;Weigelt et al.2005),which influences the future rate of resource capture(Poorter et al.1990;Aikio et al.2009).Seedling growth is a critical period in the life cycle of tree species(Comita et al.2010),and the partitioning patterns of biomass during seedling growth contribute to determining photosynthetic capacity and the costs of construction and maintenance(Ding et al.2016).Numerous studies have analyzed biomass distribution patterns in tree seedlings.Toledo-Aceves and Swaine(2008)examined biomass partitioning and photosynthetic responses of lianas and pioneer tree seedlings to light;Mediavilla and Escudero(2010)compared differences in biomass partitioning patterns between saplings of two cooccurring Mediterranean oaks. Sevillano et al. (2016)reported biomass distribution of Fagus sylvatica L.and Quercus robur L.seedlings.Although biomass allocation in tree species has been studied extensively(e.g.,Parresol 1999,2001;Bi et al.2004;Enquist and Niklas 2002;McCarthy and Enquist 2007; Peichl and Arain 2007;Gargaglione et al.2010),relatively little is known about the effects of density on tree component yields(Burkes et al.2003;Litton et al.2003;Fang et al.2007;Xue and Hagihara 2008a).As a result,relationships among component yields and density are largely unknown. Competition mechanisms may differ among tree organs(Weiner 1990)because of their different biomass partitioning patterns under competition stress(Xue et al.2010).

The fact phenomenon that stand yields increase with increasing density is called the Y-D effect.Because growth rates in lower density stands are higher than in higher density stands,differences in stand yields decrease with increasing age. Stand yields finally become constant regardless of final density(Burkes et al.2003;Xue and Hagihara 2008a).The relationship between yield and stand density is often modeled mathematically.The reciprocal linear equation of Shinozaki and Kira(1956)for fitting yield-density data was used originally in Y-D studies.De Wit(1970)re-formulated it as an asymptotic equation(still algebraically equivalent to the linear reciprocal equation).Later Watkinson(1980,1984)and Vandermeer(1984)modified the asymptotic version by adding an exponent that allowed the shape of the curve to vary from the asymptotic form.

Leucaena leucocephala(Lam.)de Wit.is a fast-growing,leguminous small tree from Central America that is frequently used as poles for construction,firewood,and shade provision in permanent plantations(Prasad et al.2011).It is widely distributed in tropical and subtropical regions.It has a well-developed root system with nodules of symbiotic bacteria capable of fixing nitrogen,and is commonly used to reforest barren hills in southern China.In recent years,this species has become one of the most widely used for improving soil fertility via nitrogen fixation,preventing soil erosion,as well as for restoration projects.The leaves can improve soil fertility(Mugwe et al.2009),and the crown provides an effective cover to prevent nutrient leaching of soils.Stems of leucaena are strong,light in weight and easy to work(Rao 1984),and suitable for a wide range of uses from those of the traditional small-scale farmers and small holders to the more recent utilization by large-scale industries for pulp and energy generation(Pottinger and Hughes 1995).Leucaena leucocephala has a porous wood structure,long fibers,and high holocellulose,a-cellulose and low lignin contents with xylan-type hemicellulose,making it a suitable raw material in the pulp and paper industry(Prasad et al.2011).Current densities of L.leucocephala plantations range from 3330 to 83,333 trees/ha in China(Xing 1986;Xing and He 1988;Jiang et al.1990;Xu et al.2014).Although density is of fundamental importance to the function of L.leucocephala,information regarding the effects of competition on the component yields is scarce.

The Y-D effect alters the biomass partitioning among organs(Xue et al.2012),which alters the ability of plants to compete for limited resources(Poorter 2005).A quantitative understanding of biomass partitioning patterns is of fundamental importance to plant ecology and for forest management(Liu and Su 2016).The objectives of this study are to determine how stand biomass is allocated under different densities,and to examine whether the Y-D effects on organs,such as branches,leaves,stems,bark and roots,can be explained by the parabolic equation derived from the reciprocal equation of the Y-D effect and the allometric relationship of mean organ mass to mean mass.

Materials and methods

The study was conducted at the nursery (113°21′E,23°09′N) at the South China Agricultural University,Guangzhou City,Guangdong Province.The nursery has a humid subtropical monsoon climate characterized by warm temperatures and ample rainfall.The annual average temperature,the temperature of the coldest month(January),and for hottest month (August), was 21.8, 13.3, and 28.1°C,respectively.Annual rainfall averages 1900 mm,and occurs mainly from April to October.The elevation of the nursery is 20 m.a.s.l.with yellow soil and peaty soil,with a pH of 7.7.Before planting L.leucocephala seedlings,the nursery soil organic matter was 7.63 g kg-1,and total N,P and K were 0.21,0.28 and 7.88 g kg-1respectively,and effective N,P and K were 18.45,1.05 and 40.19 mg kg-1,respectively,in the upper 40 cm soil layer(Zhang et al.2015).

The study was established in July 2011 as a randomized complete block design with four density treatments replicated in three 0.48 ha L.leucocephala blocks.Three-monthold L.leucocephala container-grown seedlings with an average basal diameter of 0.35 cm and an average height of 27 cm were planted in a square spacing and densities of 10,000,20,000,40,000,and 80,000 seedlings ha-1in each block.One plot of 0.03 ha(20 m×15 m)was established in a randomized complete block design and density effects were analyzed.Plots were spaced apart to minimize any interaction effects.

Seedlings were watered every day to maintain the soil at field capacity and no fertilization was used during the experimental period.Starting at the 15 month after planting,diameter at breast height(d),height(h),crown length,and crown width were measured monthly.Seedlings were also measured for height to the base of the live crown.For each seedling,the height of the live crown was calculated by subtracting the height to the base from the seedling height.Tree mortality of each block was less than 2%.The characteristics of the seedlings are shown in Table 1.

Fifteen seedlings in each plot were harvested after 25 months in October 2013.The root biomass(＞0.2 cm diameter)down to maximum depth of roots was estimated by excavating the soil around the roots according to the spacing(Xue et al.2011).Each seedling was divided into branches,leaves,stem,bark,and roots,and the biomass of these organs quantified immediately using an electronic balance.The samples were then dried at 85°C to a constant weight to determine dry:fresh biomass ratios.

For each of the different densities, the relationship between mass,wo,of an organ and d and h was examined by the following allometric equation:

where a and b are coefficients.

Using this allometric equation,the masses of the components for all individuals of each plot were estimated monthly from 15 to 25 months after planting.Mean seedling mass,w,was calculated as the sum of the mean masses of the seedling organs.Separate equations for each component and stand density were fitted using ordinary leastsquares estimates(Table 2).

The coefficients of the allometric equations were determined by the standardized major axis (SMA)according to Warton et al.(2006).The study,established with four density treatments replicated in three blocks,had 11 degrees of total freedom.Turkey's multiple comparisons determined significant differences over time in each density block and differences in growth and organ biomass partitioning at 25 months.Differences were deemed significant when P ＜0.05.All statistical analyses were performed using Excel 2003 and the Statistical Analysis System(SAS 9.0).

Model fitting

The relationship between the mean organ mass,wo,and mean tree mass,w,was formulated as:

where g is a coefficient and k an allometric constant specific to different growth stages(Kira et al.1956;Xue and Hagihara 2008a),g and k were obtained by the method of least squares(Table 3).

The ratio of each organ mass to seedling mass,wo/w,was derived by dividing both sides of Eq.2 with the mean seedling mass,w.

The reciprocal equation of the competition-density(CD)effect is expressed as(Shinozaki and Kira 1956):

where w is the mean tree mass,ρ is the stand density,and A and B are coefficients specific to different growth stages.

Combining Eqs.2 and 4 gives:

We can obtain the Y-D equation by dividing both sides of Eq.4 by stand density

By multiplying both sides of Eq.5 by ρ,the organ yield-density equation can be derived as:

Results

Effect of planting density on seedling growth

Density had a noticeable effect on diameter;diameter decreased with increasing density throughout the study period.At harvest,the d values of the 10,000,20,000,and 40,000 seedlings ha-1blocks were approximately 44,24,and 16% greater, respectively, than that of the 80,000 seedlings ha-1block(Fig.1a).The difference indiameters between the 10,000 and 20,000 plantings was significant,and the diameters of the 20,000 density planting were significantly greater than those of the 40,000 and 80,000 seedlings ha-1blocks at 25 months.

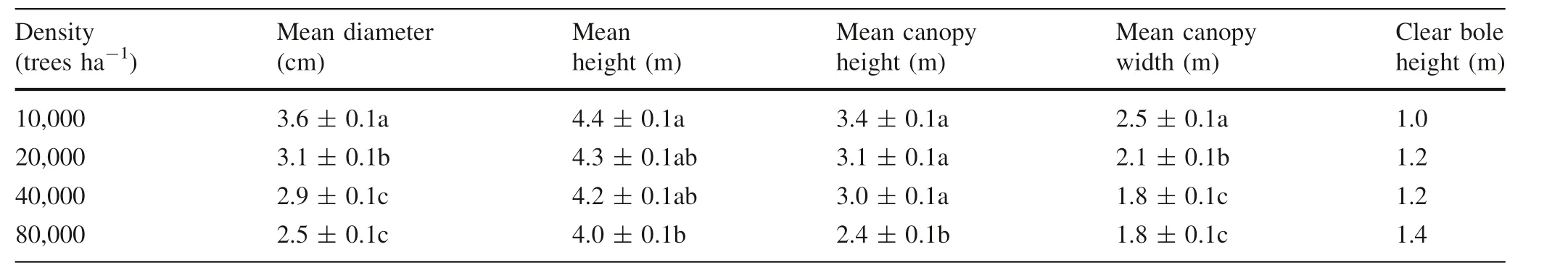
Table 1 Characteristics of seedlings at different densities(mean±standard error)

Table 2 Equations of the organ mass of L.leucocephala seedlings at different densities

Mean heights ranged from 3.8 to 4.3 m,3.6 to 4.2 m,3.5 to 4.1 m,and 3.5 to 4.0 m for the 10,000,20,000,40,000,and 80,000 seedlings ha-1blocks,respectively(Fig.1b).Tree height was higher in the 10,000 and 20,000 densities and lower in the 40,000 and 80,000 seedlings ha-1plantings.The differences in seedling height among all density plantings were significant at harvest.

The H:D ratios calculated from mean height and diameter at breast height were 1.06,1.08,1.19 and 1.39 for the 10,000,20,000,40,000,and 80,000 density plantings at 25 months.

Crown width varied from 1.8 m in the 80,000 seedlings ha-1plot to 2.5 m in the 10,000 density planting.At harvest,crown heights in the 10,000,20,000,and 40,000 seedlings blocks were significantly greater than that in the 80,000 seedlings ha-1block(P ＜0.05),and mean crown widths were significantly greater in the 10,000 than in the 20,000 seedlings ha-1block. Mean crown heights were significantly greater in the 20,000 density planting than in the 40,000 and 80,000 seedlings ha-1blocks.Crown heights and widths increased with decreasing density(Fig.1c,d).Crown heights ranged from 2.4 m in the 80,000 seedlings ha-1block to 3.4 m in the 10,000 seedlings planting.Clear bole heights were 1.0,1.2,1.2,and 1.4 m for seedlings in the 10,000,20,000,40,000,and 80,000 density plantings,respectively(Table 1).

Effect of density on biomass partitioning

Density influenced the allocation of biomass into branches,leaves,stem,bark,roots,as well as the total biomass on a per hectare basis. With increasing age from 15 to 25 months,biomass partitioning into branches increased from 17.8 to 19.5%,and from 17.4 to 19.5%in the 10,000 and 20,000 seedlings ha-1plantings,respectively,while biomass division to leaves increased from 6.2 to 7.3%,and from 6.7 to 8.1%,respectively.These values remained relatively steady in the 40,000 and 80,000 density plantings(Fig.2).Allocation of biomass into stems and bark components remained relative steady in all densities.Partitioning to roots decreased from 24.6 to 22.2%,and from 27.2 to 22.5%in the 10,000 and 20,000 seedlings ha-1plantings, respectively, whereas it remained relatively constant in the other density plantings.At the time of harvest,there was a decrease in the proportion of biomass allocated to branches and roots with increasing density.In the case of stem biomass,greater density increased the proportion of biomass accumulation.For all densities,the stems contained the greatest proportion of biomass(43.5-51.0%),followed by roots(18.7-22.5%),branches(15.3-19.5%),leaves(7.3-9.0%),and bark(5.8-6.2%).

Table 3 Values of coefficients g(kg1-k)and k in Eq.7 and coefficients A and B in Eq.6

The Y-D effect of L.leucocephala seedlings

Figure 3 shows the relationship between mean organ mass and density of L.leucocephala plantings.The relationship between yield(y)and density(ρ)is shown in Fig.3a.The Y-D effect is evident and led to an increase in yield(y),with increasing density(ρ).The y-ρ relationships fitted well by the reciprocal equation of the Y-D effect for the experimental period,and the y-ρ curve on a log-log graph shifted upward with increasing age.

Y-D effect on tree components was evident for all densities,and yields of branches,leaves,stems,bark,and roots significantly increased with seedlings density from 15 to 25 months(Fig.3b-f).The yBr-ρ data points were well described by the yBr-ρ curve.The time trend of the relationships among yL-ρ,yS-ρ,yBa-ρ,and yR-ρ was similar to that of the yBr-ρ relationship,although the scale of the yield differed.Curves of yL-ρ,yS-ρ,yBa-ρ,and yR-ρ shifted upward on a log-log graph throughout the experimental period.

Discussion

Effect of density on seedling growth

Fig.1 Growth of L.leucocephala seedlings.a Mean diameter at breast height;b seedling height;c crown width;d crown length.is 10,000 seedlings ha-1;, 20,000 seedlings ha-1;,40,000 seedlings ha-1;×,80,000 seedlings ha-1

Competition in high density plantings led to growth suppression, especially in the 40,000 and 80,000 seedlings ha-1blocks.Seedling growth decreased with increasing density.Generally,competition levels that decrease resource availability also decrease seedling growth(Jensen et al.2011).This occurs because space decreases with increasing density,and results in competition for light,water,and nutrients.L.leucocephala is a light demanding species.High-density seedlings are characterized by small crown widths and lengths because lateral growth is impeded in the early growth phase,and pronounced natural pruning occurs during the early growth phase as well.Small crowns are unfavorable for diameter growth because of their low photosynthesis levels(Xue and Hagihara 2008a).Moreover,soil fertility in high density stands is lower than in low density stands(Yang et al.2013;Lie et al.2017)because the rapid growing of more tree individuals in higher density stands absorbs a larger amount of soil nutrients(Lie et al.2017).Litter in higher density stands is less compared with lower density stands(Yang et al.2013),which results in decreased soil fertility(organic matter,N,P and K)with increasing density in L.leucocephala stands.Consequently,intense competition for light and soil fertility in higher density stands may be a limitation to seedling growth. A decrease in seedling diameter growth with increasing planting density was reported for Cordia spp. (Hummel 2000), eucalyptus(Bernando et al.1998;Pinkard and Neilsen 2003;Alcorn et al.2007;Xue et al.2011).),six subtropical rainforest species(Grant et al.2006),and for L.leucocephala stands(Prasad et al.2010).

Fig.2 Relationships between organ mass ratios (wo/w).a 10,000 seedlings ha-1 block; b 20,000 seedlings ha-1 block;c 40,000 seedlings ha-1 block;and d 80,000 seedlings ha-1 block.,branches;,leaves;,stem;+,bark;,roots

The height growth of white oak(Quercus alba L.)(Wichman and Coggeshall 1983,1984)and Eucalyptus urophylla S.T.Blake(Xue et al.2011)was relatively unaffected by seedling density.However,in this study,heights of high-density seedlings were significantly influenced by density at harvest.The mean height decreased with increasing density,indicating that intense competition among high-density seedlings reduced photosynthesis as a result of leaf overlap within and among individual seedlings.Prasad et al.(2011)found that density significantly influenced the height of L.leucocephala seedlings.Howell and Harrington(1998)and Buckley(2002)also reported that seedling density significantly affected height of bareroot oak and northern red oak (Quercus rubra L.)seedlings.

Fig.3 The Y-D effect for relationships between organ,tree yield and density a tree;b branches;c leaves;d stem;e bark;and f roots.filled circle,15-month-old;circle,17-month-old;filled square,19-monthold;square,21-month-old;filled triangle,23-month-old;and triangle,25-month-old seedlings.The curves were fitted using Eq.6 or Eq.7

The H:D ratio of seedlings increased with greater density,which might suggest that seedlings in higher density stands prioritized the allocation of biomass to height growth at the expense of diameter growth.This trend is confirmed by the greater diameter growth per unit of stem biomass in lower density stands.

The increase in height of the lowest green branch in high-density planted L.leucocephala seedlings is evidence of increased branch suppression and competition for resources.Generally,the rate of increase of the green crown height increases with increasing stand density(Neilsen and Gerrand 1999;Klootwijk 2001)as competition for resources,particularly light,intensifies(Opie et al.1984).Alcorn et al.(2007)also found that green crown height increased with increasing density.

Effect of density on biomass partitioning

With increasing density,the division of biomass to branches decreased,whereas it increased to the stems in all density plantings. High-density seedlings increased the biomass allocation to the most merchantable portion of the stem,as branch size,the number of laterals,and crown length gradually decreased with increasing density(Neilsen and Gerrand 1999;Xue and Hagihara 2008a;Japhet et al.2009).Our results are similar to those from studies of loblolly pine(Pinus taeda L.)(Ares and Brauer 2005),Scots pine(Pinus sylvestris var.mongolica Litv.)(Kellomaki et al.1989;Nilsson and Albrektson 1993),Eucalyptus urophylla S.T.Blake and E.camaldulensis Dehn(Bernando et al.1998)and E.pilularis SM,E.cloeziana F.MUELL.(Alcorn et al.2007),and E.urophylla S.T.Blake plantations(Xue et al.2011).Biomass allocation from branches to stem can be explained by resource reduction,i.e., lower light resources to high-density seedlings.Increased biomass to the higher density stems reduced the investment in branch and root biomasses which weakened their growth.This is unfavorable in competing for water and nutrients,decreasing growth efficiency of individual seedlings during early development.On the other hand,high density stands increase total stem productivity by increasing soil nutrient use and increasing partitioning to stem(Burkes et al.2003).

The Y-D effects

Seedling yield-and organ yield-density relationships were validated using data for 25-month-old L.leucocephala seedlings at seedling growth stage(Fig.3),indicating that our equations are comprehensive and convenient for analyzing Y-D effects.This is useful for explaining the partitioning of photosynthates among components under density stress,as well as the adaptation of morphology in response to competition(Xue et al.2012).Numerous tree species are planted in China and plantations are widely distributed over 6.2×107ha, accounting for approximately 32%of China's forested area.The Y-D effect of these plantations has rarely been reported. Therefore,Eqs.6 and 7 are important for studying the Y-D effect on trees and tree organs from theoretical and practical viewpoints.

In a study of the Y-D effect in Pinus densiflora Siebold& Zucc. stands, Xue and Hagihara (2008a) found an optimum density for needle and branch yields.However,in this study,all organ yields increased with increasing density,and an optimum density for leaf yields or branch yields was not found.The 25-month-old L.leucocephala seedlings are young material compared with the 10-33-year-old P.densiflora trees;thus,the effect of density is only expressed as a reduction of the seedling or component yields,without seedling mortality.However,as time progresses and trees grow larger,available resources become increasingly limited and self-thinning will eventually occur.Therefore,there will ultimately be an optimum density for leaf and branch yields.Because the applicability of Eqs.6 and 7 is restricted to the Y-D effect in nonself-thinning plantations,a parameterized form of Eq.2,such as suggested by Xue and Hagihara(2008a),is suitable for examining the Y-D effect in self-thinning plantations.

Increasing stand density increases the acquisition and use of resources such as light,nutrients,and water during early seedling growth. As a result, the total biomass increment of stands increases with density even though the growth rate of individual trees decreases.The biomass increment of higher density stands converge at a maximum that represents the upper limit of productivity for the specific site and environmental conditions(Burkes et al.2003).In such conditions,the value of coefficient B in Eqs.6 and 7 tends to be zero (Xue and Hagihara 2001,2008b).As a result,Eqs.6 and 7 take the form:

This means,after a sufficient lapse of time,stands of different densities all reach a constant yield per unit area 1/A.In this growth stage,y is independent of density(the law of constant final yield),indicating yield per unit area cannot increase without limit.On the other hand,component yield per unit area changes with the relative growth coefficient k.When the value of k is smaller than 1.0,the final organ yield Yoincreases with increasing density.When the h-value is larger than 1.0,Yodecreases with increasing density.When the h-value is equal to 1.0,Yoreaches a constant with a value of g/Ak.In such cases,organ yield per unit area reaches a plateau.

AcknowledgementsWe thank two anonymous reviewers for their helpful comments.The study was partially supported by the Forestry Technology Popularization Demonstration Project of the Central Government of China(2015-GDTK-07).