The role of crystal lattice free volume in nitramine detonation
2019-10-31SvatoplukZemanNingLiuAhmedHussein
Svatopluk Zeman ,Ning Liu ,Ahmed K.Hussein
a Institute of Energetic Materials,Faculty of Chemical Technology,University of Pardubice,CZ-532,10 Pardubice,Czech Republic
b Xi'an Modern Chemistry Research Institute,Xi'an,Shaanxi,710065,China
c Military Technical College,Kobry Elkobbah,Cairo,Egypt
Keywords:Crystal lattice Detonation Initiation reactivity Nitramines
A B S T R A C T Crystal lattice free volumes,ΔV,of 22 nitramines introduced into relationships of maximal theoretical crystal densities(TMD),detonation velocities(D)and volume heats of explosives(ρ.Q).Values of all these characteristics increase with an increase in the ΔV values.However,more realistic dependencies for the TMD and D values are obtained by using correlations with ratio of the intrinsic molecular volume,Vint,to ΔV(i.e.Vint/ΔV),whereby the dependence on the D values reflects their similar relationship to a sum of the positive(VS,max)and negative(VS,min)extremes of the molecular surface electrostatic potentials(VS,∑).Also,directly proportional linear relationships are specified between the VS,∑values on the one hand,and the ΔV and Vint/ΔV values,on the other.It is stated that the crystal lattice free volumes might have a similar role in detonation of nitramines as the intermolecular force effect in their molecular crystals(they are as if representative of such forces).
1. Introduction
Recently our attention has focused on the role of the crystal lattice free volume in the initiation of nitramines by impact[1],friction[2]and thermal stimulus[3].Initiation by means of shock(initiation and development of detonation),has not been studied until now in this sense.Various models of initiation by shock have been described(see Ref.4 and references therein).Information concerning the shock wave chemistry of molecular explosives can be found for example in monographs[4,5]or papers[6—8].In conception of initiation both on the basis of the excited bulk phonons[4,6,7]or plastic deformations[4,8]the defects of crystal lattice have theirs place;these defects enhance the chemical reaction probability in this process because of the hot spots generated[4—8].
Crystal lattice free volumes are given by the conformation and mutual force effect of molecules in the crystal lattice and cannot be considered as classical defects-rather they represent“void spaces”in the lattice.Therefore,it is not without interest to assess whether there could been a relationship of these “void spaces” with detonation parameters,including the crystal density,in the case of nitramines.This subject is addressed in this study,using the nitramines and their crystal lattice free volume data found in these papers[1—3].
2. Data sources
2.1. Nitramines under study
Chemical names,code designations,maximal crystal densities and detonation parameters(D and Qreal)of the nitramines studied are summarized in Table 1.For a better illustration,the structural formulae of the nitramines used are presented in Scheme 1.
2.2. Detonation parameters
Detonation velocities D were calculated using the Kamlet&Jacobs method[9];in the case of ε-HNIW the value of D was obtained by extrapolating the experimental data to the value of its theoretical maximum crystal density[10].The heats of explosion,Qreal,calculated according to Pepekin et al.[11]should correspond to the values determined using a calorimeter,i.e.they involve the effect of the charge density.
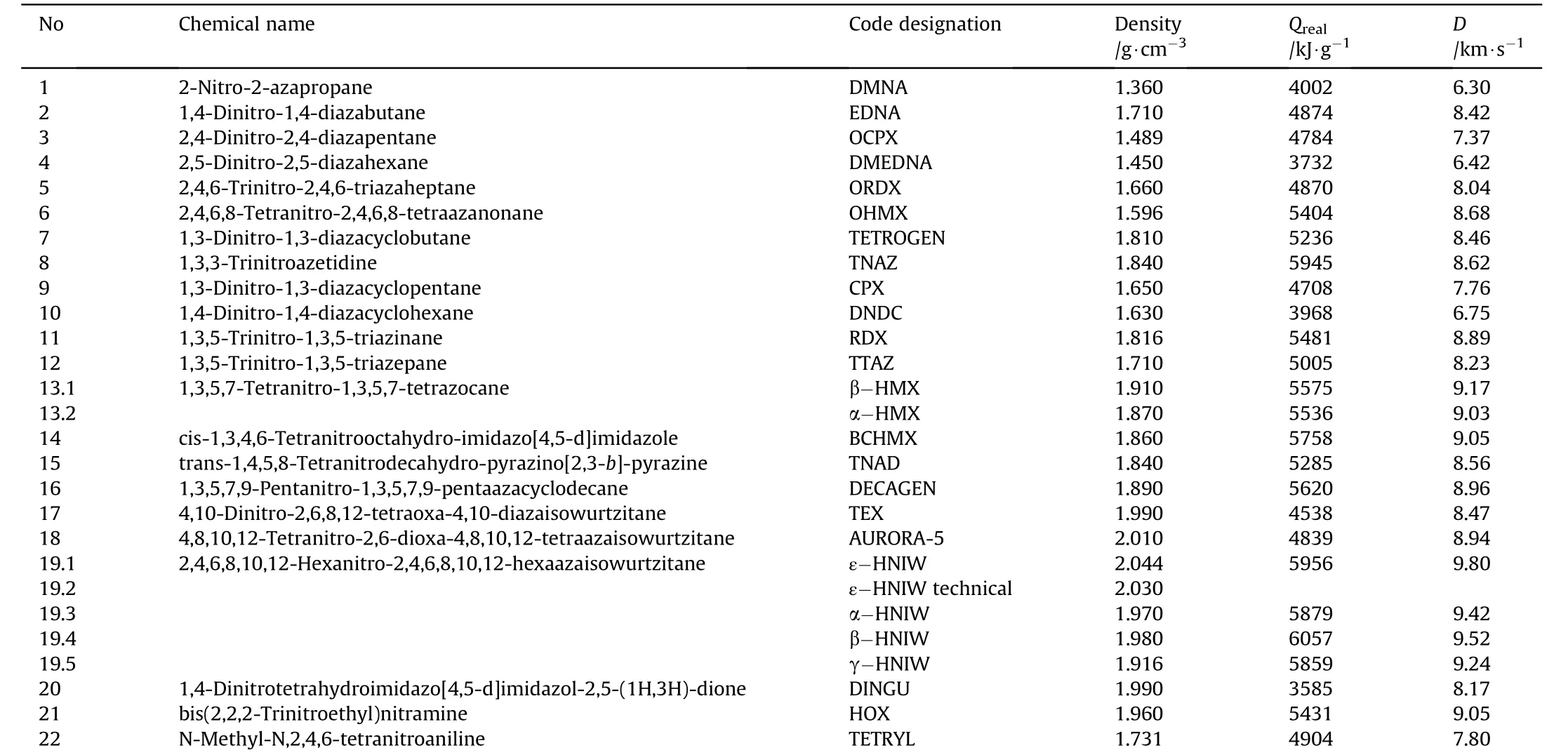
Table 1 Details of the nitramines studied showing their maximal crystal densities,heats of explosion,Qreal,and detonation velocities D.
2.3. Results of calculations for crystal lattice free volume of nitramine explosives
All of the compounds were optimized at computational level of B3LYP/6-311+g(d,p)by using the Gaussian 09 software.The crystal volume[V(0.003)]was calculated by using the Multiwfn 3.3.9 software.The effective volume per molecule(Veff)is calculated as:Veff=M/(NAρ),where M is molecular mass,NAis the Avogadro's number and ρ is crystal density.The intrinsic gas phase molecular volume(vint)is calculated by the 0.003 au surface according to Ref.12,Vint=V(0.003).Therefore,the free space per molecule(ΔV)is:
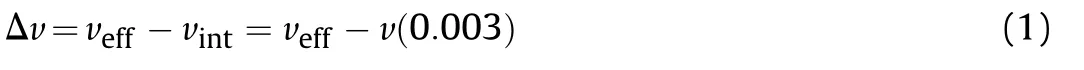
Results of these calculations are summarized in Table 2.
3. Results and discussion
3.1. Theoretical maximum crystal density
Crystal density is a very important property of energetic materials(EMs)because it has a very strong influence on the energy content of the given material.And because this property is related to the quality of the crystal,it is interesting to know what relationship it could have with the crystal lattice free volume.
Using a simple approach for derivation of relationships in this area,presented in paper[12],a semi-logarithmic relationship was found between the theoretical maximum density(TMD)and the ΔV values,as shown in Fig.1.In this chart,the group of nitramines is divided into two sub-groups,one as if derived from 2,4,6,8,10,12-hexanitro-2,4,6,8,10,12-hexaazaisowurtzitane(HNIW),i.e.straight lines I-IV,and one related to 2-nitro-2-azapropane(DMNA),straight lines VII—IX.In Fig.1 there is also a group of structurally similar cyclic nitramines represented by the straight line V.In all cases,an increase in the ΔV values corresponds to an increase in TMD;this increase in the‘void spaces’between molecules seems to increase as if“a dilution of nitramine in its crystal”.The chart also confirms that,in these types of nitramine,it is possible to achieve a maximum theoretical density,corresponding to the crystal density of ε-HNIW,but not more.It should be added here that,with exception for the use of the logarithms,the principle of the construction of dependences in Fig.1 is basically the same as the mutual comparison of the ΔV values with impact sensitivity of nitramines[12].
However,another view of the relationship between the ΔV values and TMD is created when the free volume is replaced by the ratio of the intrinsic volume of molecule,vint,to the ΔV value,as shown in Fig.2.Here,the impression of“a crystal dilution”is lost and more realistic volume ratios in the crystals are more easily seen.Values for the intrinsic volumes exceed the values of the free volumes mentioned more markedly in the cyclic nitramines perhaps due to a higher intermolecular force effect in their crystals and their more rigid molecular skeletons compared to those of the linear nitramines(DMNA,DMEDNA,OCPX,OHMX,Tetryl,with the exception of HOX and ORDX).
3.2. Detonation velocity
A simple mutual comparisons of detonation velocities for theoretical maximum densities with the ΔV values of the nitramines studied gives Fig.3.Similarly,as in Fig.1,dependencies are partly as if derived from DMNA(straight lines A,D,E and F)and partly from HNIW(straight lines B and D).In all these cases(also separated line C)an increase in the ΔV values corresponds to an increase in detonation velocities in the nitramines studied.Considering the dependencies in Fig.1 and Fig.2,this trend seems to be logical(influence of the crystal density on detonation).
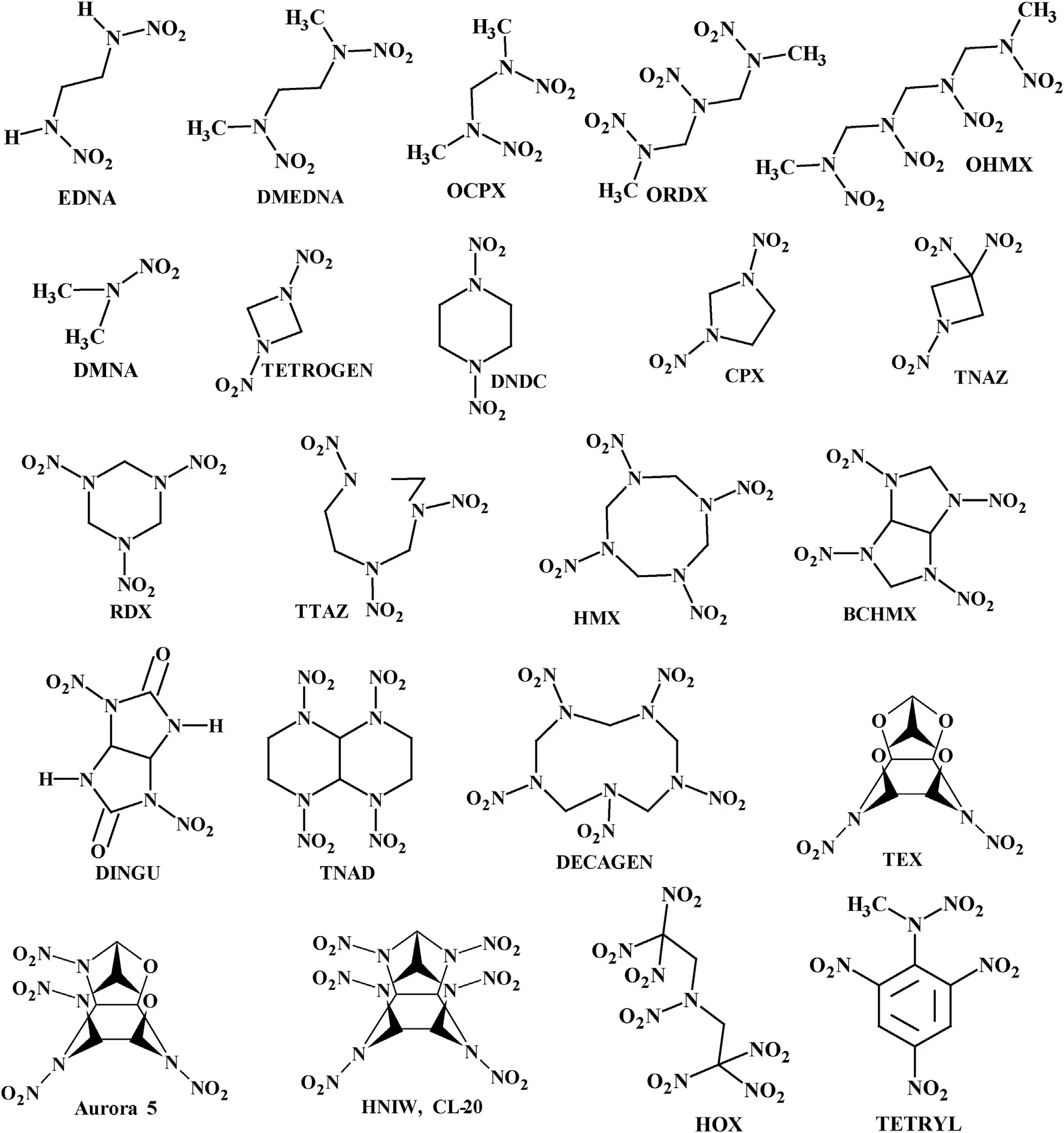
Scheme 1.Structural formulae of the nitramines studied.
Replacing the ΔV values on the X-axis of Fig.3 by the ratio Vint/ΔV(similar to that in Fig.2)provides Fig.4.In this way constructed dependences relatively strongly remind relationships of detonation velocities with the sum of positive(VS,max)and negative(VS,min)extremes of the molecular surface electrostatic potentials,VS,∑,for studied nitramines,as described in paper[13](the VS,maxand VS,minvalues were calculated at the DFT B3LYP/6-31G(d,p)//6-311 + G(d,p) level [13,14]). Fragmental correlations are here generated from 2-nitro-2-azapropane(DMNA),which is logical,because the methylene-nitramino grouping is a part of all the nitramine molecules studied and thus it is also a part of initiation centers in these molecules[4].
3.3. Heat of explosion
From the point of view of classical reaction kinetics a detonation can be taken as the zero order reaction which corresponds to heat of reaction(heat of explosion)in units of Joules per volume(ρ.Qreal).The relationship of this volume heat of explosion with the crystal lattice free volume is documented by Fig.5.It clearly shows that,in the cyclic nitramines studied, ε-HNIW is a “top energetic compound”,and that linear nitramines cannot achieve the performance even of RDX(compare it with OHMX).With reference to Fig.1 and Fig.3,it is understandable that the growing volume heat of explosion corresponds to the increasing free volume ΔV.Two relationships in Fig.5 with the negative slope correspond to the“derived ones”on the base of the close molecular structure similarities and thus they have no effect on the basic trend.
3.4. Comments
In the case of Fig.1 and Fig.3 it is stated that the group of nitramines is divided into two sub-groups,one as if derived from 2,4,6,8,10,12-hexanitro-2,4,6,8,10,12-hexaazaisowurtzitane
(HNIW), and one related to 2-nitro-2-azapropane (DMNA). A similar distribution of the nitramines studied was found in the relationships between the energies of thermal decomposition ofnitramines and their ΔV values[3],on the one hand,and the sum of positive and negative extremes of their electrostatic surface potentials,VS,∑[13,14],on the other.That means that the crystal lattice free volume should have a relationship to these electrostatic surface potentials,shown by Fig.6;partial dependencies here are formed by data of nitramines with very similar structures.
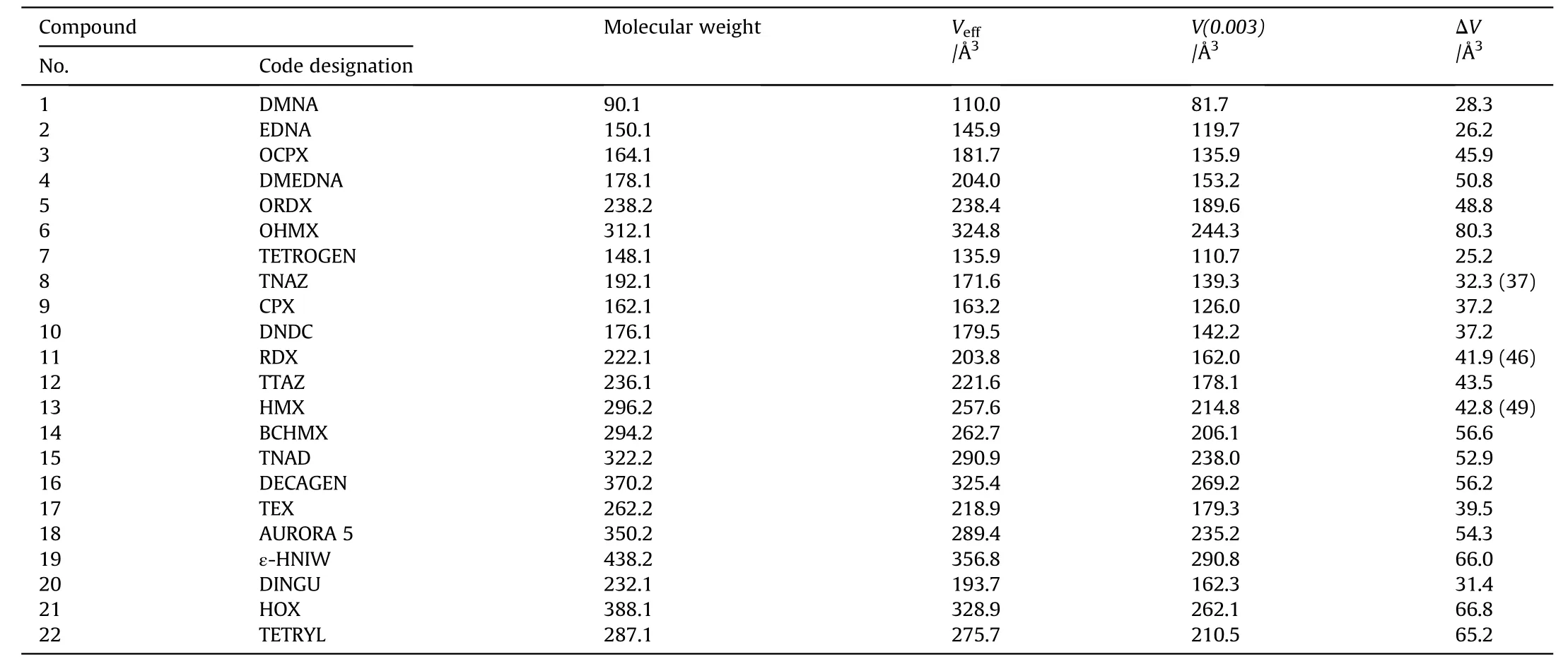
Table 2 Details,for each compound,of the molecular mass,M,effective volume,Veff,crystal volume,V(0.003)and free space per molecule,ΔV.
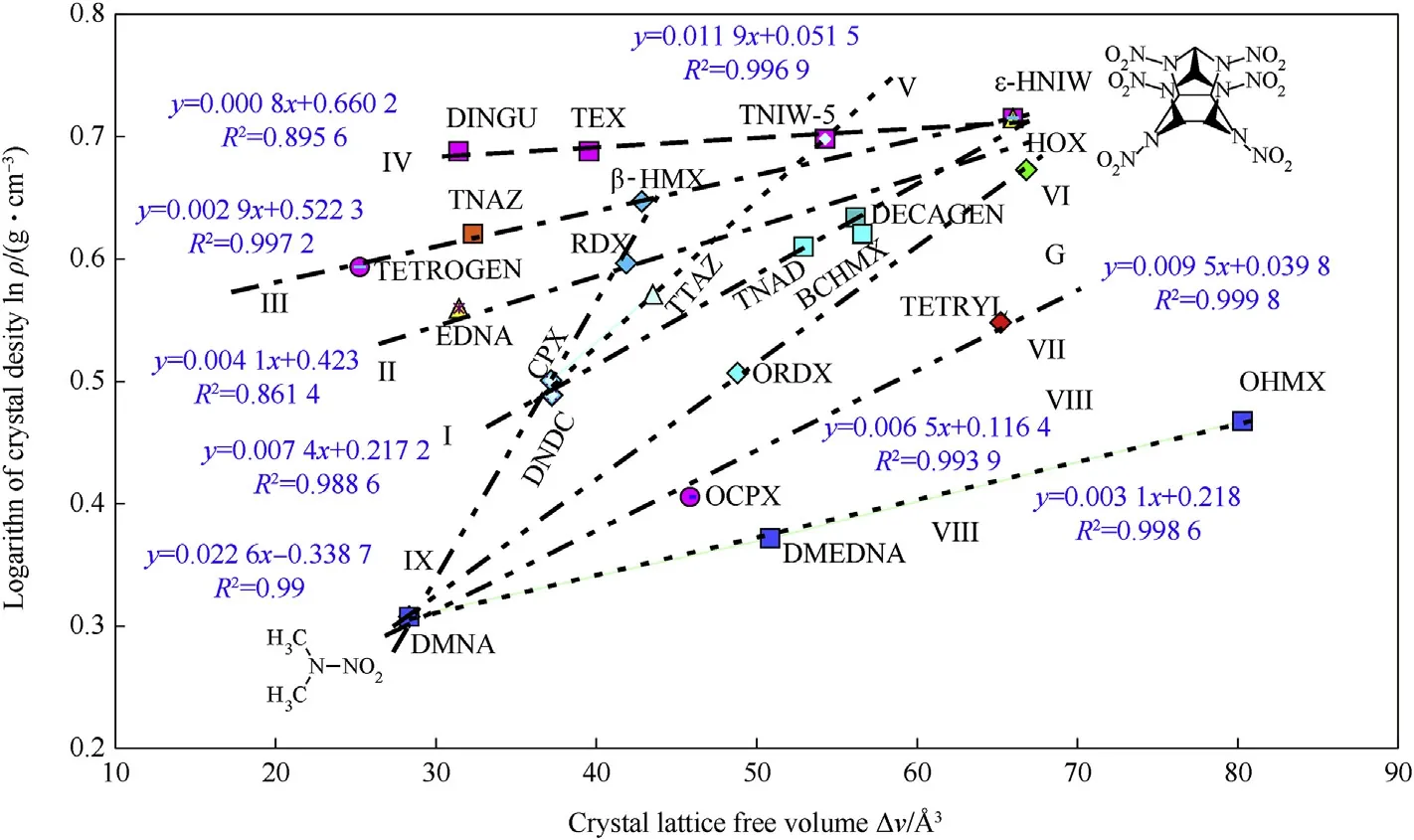
Fig.1.Semi-logarithmic relationship between theoretical maximum density and crystal lattice free volume of the nitramines studied.
The already mentioned comparison of Fig.4 with the results of paper[13]leads to the presumption that the ratio Vint/ΔV should also have a relationship with the VS,∑values,which is confirmed by Fig.7.
In the same way as described in recent papers in this area[1—3],here all partial shapes of relationships are limited by a similarity in the molecular structures.This fact,together with the well-known importance of the dipole-dipole interactions of the oxygen and nitrogen atoms of nitro groups in neighboring nitramine molecules in the crystal structure of these nitro compounds[15—18],confirms the much greater importance of the intermolecular interactions(molecular electrostatic surface potentials)in comparison with the influence of the crystal lattice free volume for initiation of detonation in the crystalline EMs[1].Nevertheless,a study of the energetic materials using crystal lattice free volume can help to explain some interesting,though sometimes,expected relationships in this category of compound.
4. Conclusion
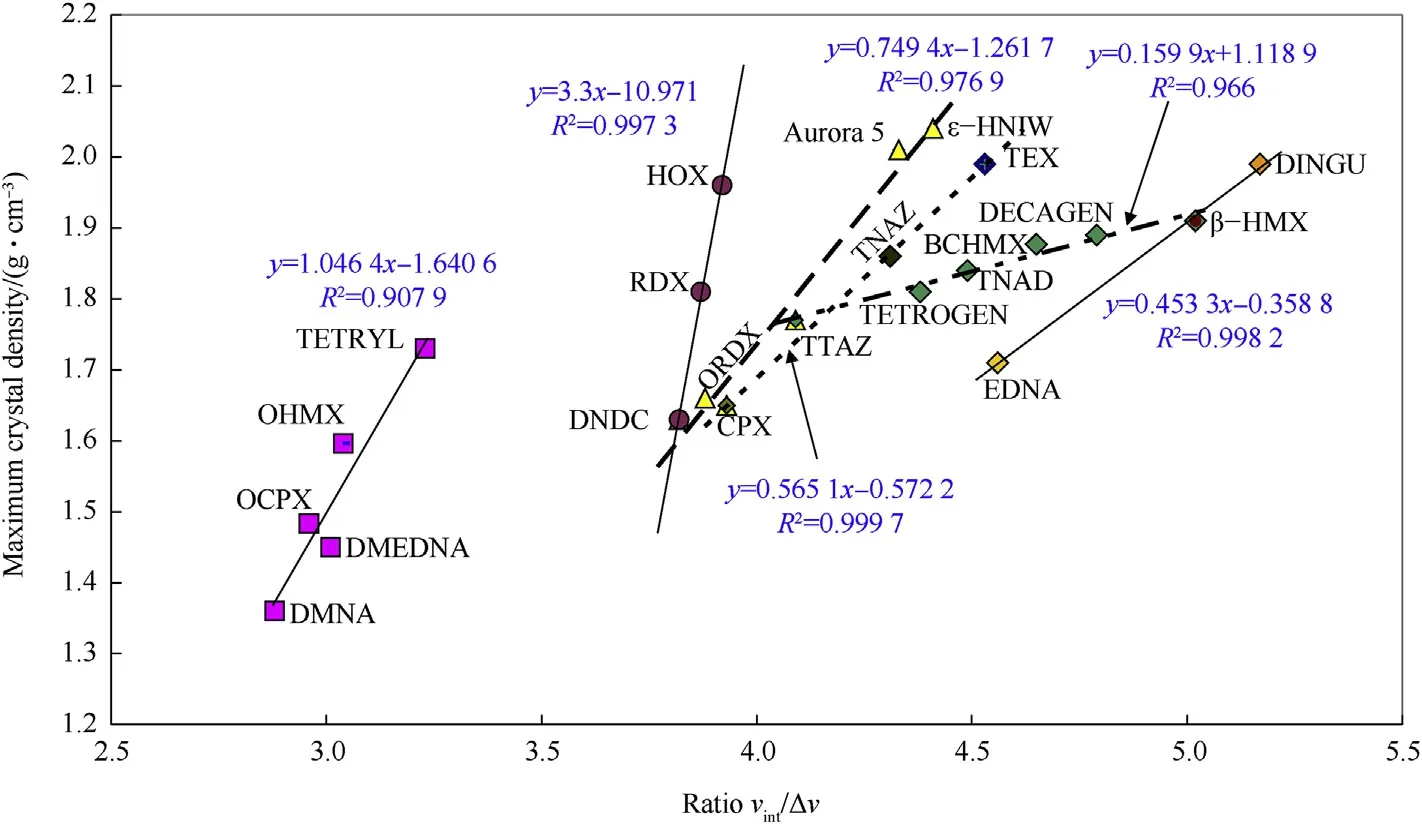
Fig.2.Relationship between the maximum theoretical density and ratio of intrinsic molecular volume,Vint,to corresponding crystal lattice free volume,ΔV,of the nitramines studied.
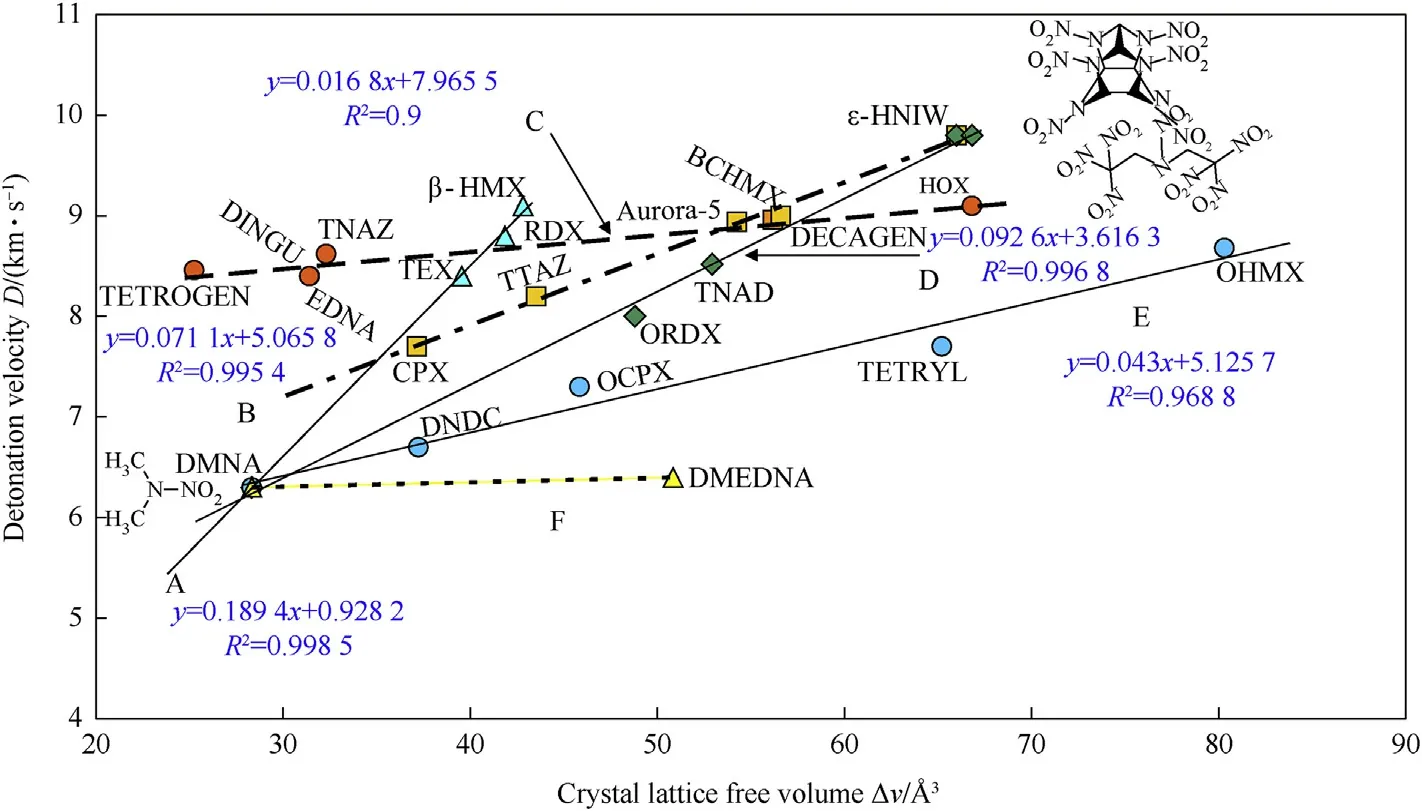
Fig.3.Relationship between detonation velocities for TMD and the crystal lattice free volume of the nitramines studied.
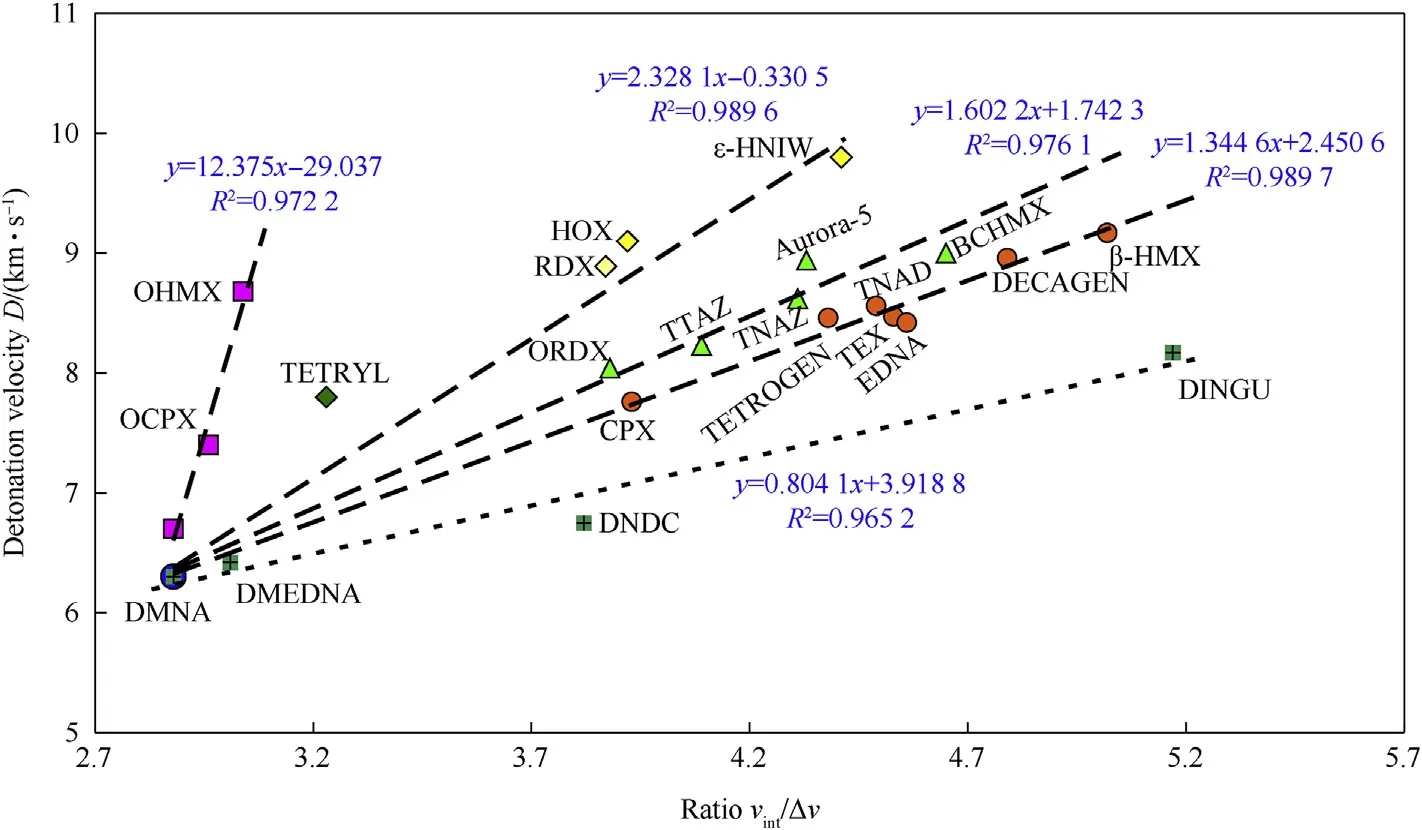
Fig.4.Relationship between detonation velocities for TMD and ratio of intrinsic molecular volume,Vint,to the corresponding crystal lattice free volume of the nitramines studied.
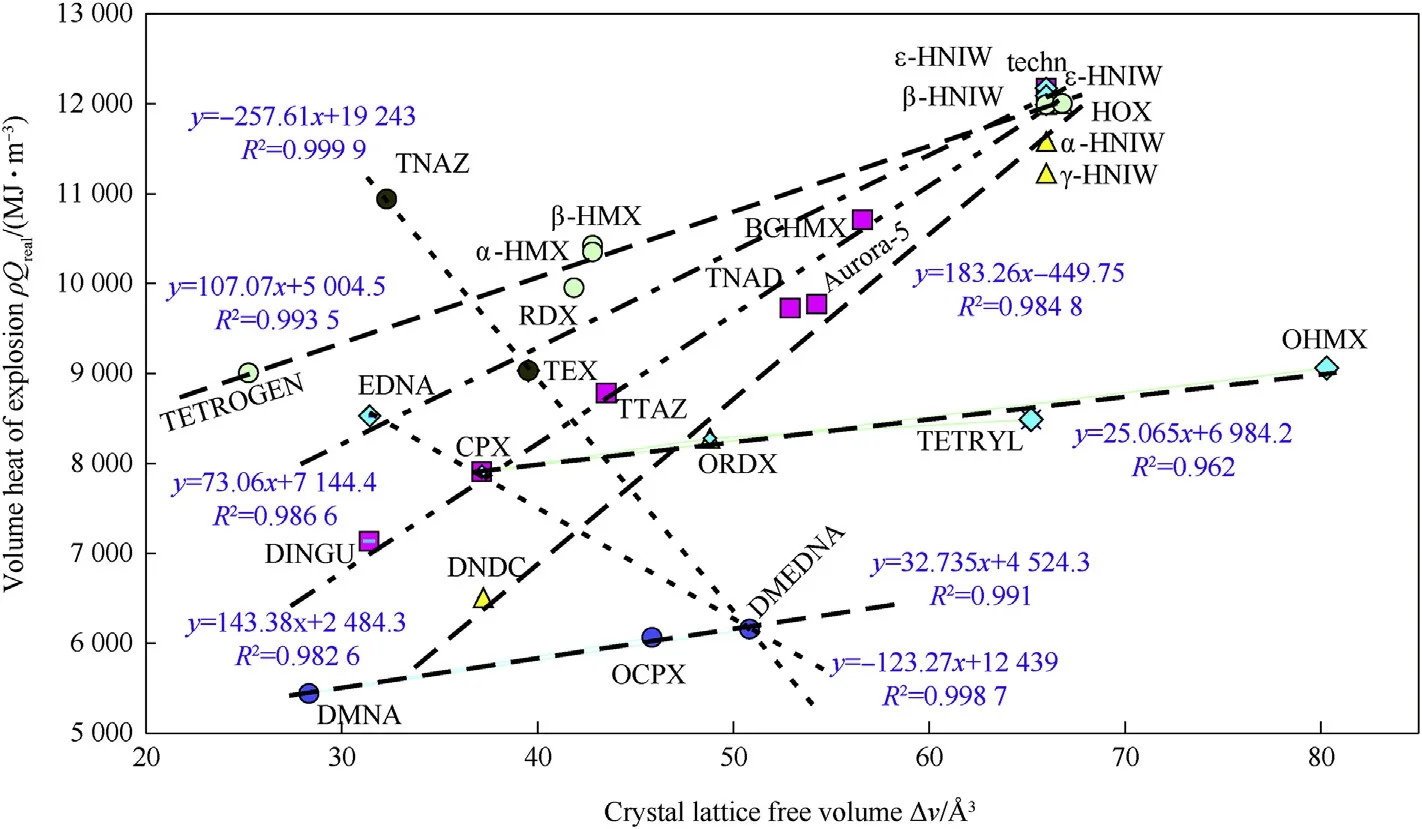
Fig.5.Relationship between volume heat of explosion for TMD(Qreal calculated according to Pepekin et al.[11])and crystal lattice free volume of the nitramines studied.
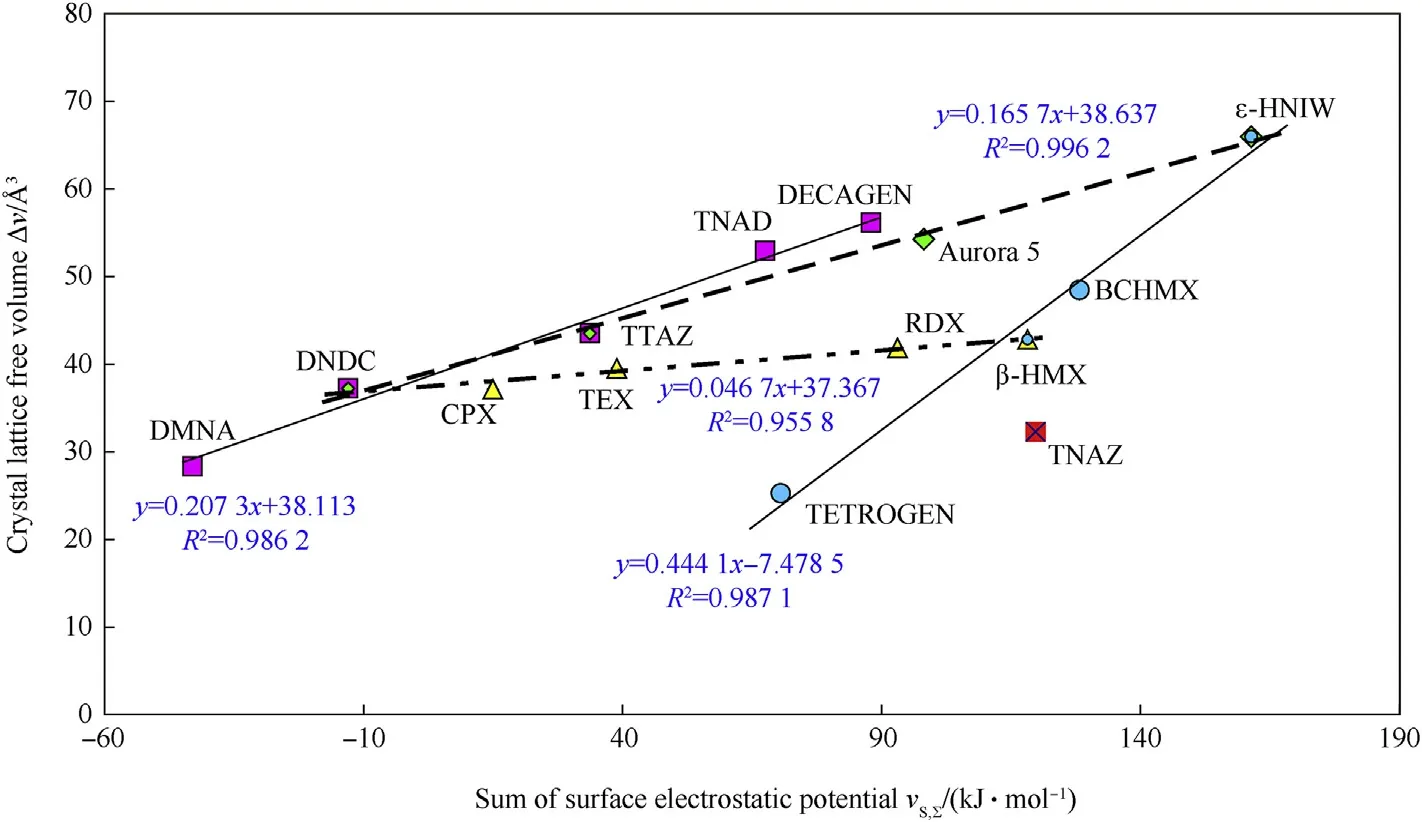
Fig.6.Relationship between sum of the surface electrostatic potentials,VS,∑,(taken from Refs.13 and 14)and crystal lattice free volume of the cyclic nitramines studied.
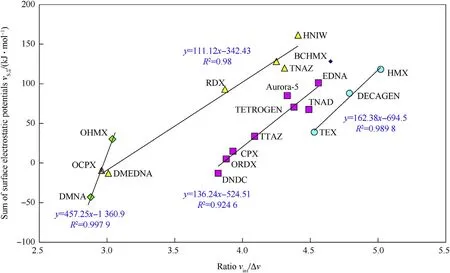
Fig.7.Relationship between sum of the surface electrostatic potentials,VS,∑,(taken from Refs.13 and 14)and ration of intrinsic molecular volume,Vint,to corresponding crystal lattice free volume of the nitramines studied.
Crystal lattice free volumes,ΔV,are a result of the joint influence of both the conformation and mutual force effect of molecules in the crystal lattice.Their relationship with the maximum theoretical density of crystals,TMD,for 22 nitramines is described as a semilogarithmic one,with the fact that an increase in the ΔV values corresponds to an increase in the TMD;this trend evokes the impression of an increased“crystal dilution”in this sense.This impression disappears when the TMD values are introduced into a linear,directly proportional,relationship with a ratio of intrinsic molecular volume,Vint,to the ΔV values(i.e.Vint/ΔV).Detonation velocities D have a linear,directly proportional relationship to the ΔV values.All the above-mentioned dependences consist of several partial relationships.In the case of TMD vs.ΔV and of D vs.ΔV,this divides the group of nitramines into mainly two sub-groups,one as if derived from 2,4,6,8,10,12-hexanitro-2,4,6,8,10,12-hexaazaisowurtzitane(HNIW)and one being related to 2-nitro-2-azapropane (DMNA). Directly proportional linear relationships between the D values and the Vint/ΔV ratios are logically generated from DMNA and reflect similar dependencies of D with a sum of the positive(VS,max)and negative(VS,min)extremes of the molecular surface electrostatic potentials(for this sum,see Refs.13 and 14).It is shown that both the ΔV values and the vint/ΔV ratios have a directly proportional linear relationship with the mentioned sum of potentials.Concerning the volume heats of explosion,a directly proportional linear dependence exists with the ΔV values,whose partial relationships for cyclic nitramines have a common intersect in data for HNIW.
It is possible to deduce from the results obtained here that the crystal lattice free volumes might have a similar role in the detonation of nitramines as the intermolecular forces have in their molecular crystals(the ΔV values are as if representatives of these forces).The results obtained for the type of cyclic nitramines studied also confirm that the highest theoretical densities of crystals and the highest heats of explosion can be expected only from the molecular structures of the HNIW type.
Acknowledgement
The work described in this paper was funded partially by Faculty of Chemical Technology at the University of Pardubice by the financial resources of Student's Grant Project No.SGS_2018_002,and partially by the financial support for a six-month traineeship in 2016 for Dr.LIU Ning in the Institute of Energetic Materials at University of Pardubice from the State Administration of Foreign Experts Affairs,Peoples Republic of China.
杂志排行
Defence Technology的其它文章
- Modeling on the shock wave in spheres hypervelocity impact on flat plates
- Insensitive high explosives:IV.Nitroguanidine—Initiation&detonation
- Effect of energy content of the nitraminic plastic bonded explosives on their performance and sensitivity characteristics
- Effect of wave shaper on reactive materials jet formation and its penetration performance
- A comparative study for the impact performance of shaped charge JET on UHPC targets
- Optimizing the defensive characteristics of mild steel via the electrodeposition of Zn—Si3N4 reinforcing particles
