LAZY 2-COCYCLE ON RADFORD BIPRODUCT HOM-HOPF ALGEBRA

MA Tian-shui, ZHENG Hui-hui

(School of Mathematics and Information Science, Henan Normal University,Xinxiang 453007, China)

Abstract: In this paper,we study Lazy 2-cocycle on Radford’s biproduct Hom-Hopf algebra.By using twisting method, we mainly investigate the relations between the left Hom-2-cocycles σ on (B,β) andon (,β ⊗α) which generalise the corresponding results in the case of usual Hopf algebras.

Keywords: lazy 2-cocycle; Radford’s biproduct Hom-Hopf algebra; Yetter-Drinfeld category

1 Introduction and Preliminaries

A lazy 2-cocycle of a Hopf algebra H is a 2-cocycle σ :H ⊗H −→K, which commutes with multiplication in the Hopf algebra. The second lazy cohomology group generalizes Sweedler’s second cohomology group of a cocommutative Hopf algebra and the Schur multiplier of a group. Letbe a Radford biproduct, where H is a Hopf algebra and B is a Hopf algebra in the category of Yetter-Drinfeld modules over H. A group morphismis constructed by Cuadra and Panaite in [1]. In [2], Panaite et al.introduced the concepts of pure and neat lazy 2-cocycle and extended pure and neat lazy cocycles to the Radford biproducts.

The origins of the study of Hom-algebras can be found in [3] by Hartwig, Larsson and Silvestrov, and earlier precursors of Hom-Lie algebras can be found in Hu’s paper (see [4]).Subsequently, Hom-type algebra has been studied by many researchers. Especially, in 2014,Li and Ma introduced the notions of Radford biproduct Hom-Hopf algebraand Hom-Yetter-Drinfeld category(see[5]),which generalize the corresponding concepts in usual Hopf algebras. In 2017, the authors presented a more general version of(see [6]).

Radford biproduct Hom-Hopf algebra was given below.

Theorem 1.1Let(H,α)be a Hom-bialgebra,(B,β)a left(H,α)-module Hom-algebra with module structureH ⊗B −→B and a left (H,α)-comodule Hom-coalgebra with comodule structure ρ:B −→H ⊗B. Then the following conclusions are equivalent.

(i)is a Hom-bialgebra, where (B#H,β ⊗α) is a smash product Homalgebra (see [7]) and (B×H,β ⊗α) is a smash coproduct Hom-coalgebra.

(ii)The following conditions hold (∀a,b ∈B and h ∈H)

(R3) εBis a Hom-algebra map and ∆B(1B)=1B⊗1B,

Definition 1.2Let(H,α)be a Hom-bialgebra,(M,αM)a left(H,α)-module with actionm and (M,ρM,αM) a left (H,α)-comodule with coaction ρM: MH ⊗M,mThen we call (M,) a (left-left)Hom-Yetter-Drinfeld module over (H,α) if the following condition holds:

where h ∈H and m ∈M.

When (H,α) is a Hom-Hopf algebra, then the condition (HYD) is equivalent to

So it is natural to consider the relations between the 2-cocycles σ on (B,β) andon

In this paper,we mainly investigate the relations between the left 2-cocycles σ on(B,β)andonand also provide two non-trivial examples.

Next we recall some definitions and results in [8] which will be used later.

Definition 1.3A left 2-cocycle on a Hom-bialgebra(H,α)is a linear map σ :H⊗H →K satisfying

for all a,b,c ∈H.

Furthermore, σ is normal if σ(1,h)=σ(h,1)=ε(h) for all h ∈H.

Remarks(1) Similarly if eq. (1.2) is replaced by

then σ is a right 2-cocycle.

(2) If σ :H ⊗H −→K is a normal and convolution invertible, then σ is a left 2-cocycle if and only if σ−1is a right 2-cocycle.

Proposition 1.4Let (H,α) be a Hom-Hopf algebra.

(1) If σ is a normal left 2-cocyle on (H,α), for all h,g ∈H, define a new multiplication on H as follows

Then (H,·σ,α) is a Hom-algebra, we denote the algebra by (σH,α).

(2) If σ is a normal right 2-cocyle on (H,α) for all h,g ∈H, define multiplication on H as follows hσ·g = α−1(h1g1)σ(h2,g2). Then (H,σ·,α) is also a Hom-algebra, we denote the algebra by (Hσ,α).

Definition 1.5A left 2-cocyle σ on (H,α) is called lazy if for all h,g ∈H,

RemarkA lazy left 2-cocyle on (H,α) is also a right 2-cocyle on (H,α).

Lemma 1.6Let γ : H −→K be a normal (i.e. γ(1) = 1) and convolution invertible linear map such that γ ◦α=γ, define D1(γ):H ⊗H −→K by

for all h,g ∈H. Then D1(γ) is a normal and convolution invertible left 2-cocycle on (H,α).

Remarks(1) The set Reg1(H,α) (respectively Reg2(H,α)) consisting of normal and convolution invertible linear maps γ : H −→K such that γ ◦α = γ (respectively σ :H ⊗H −→K such that σ ◦(α⊗α)=σ),is a group with respect to the convolution product.

(2) γ is lazy if for all h ∈H, γ(h1)h2=h1γ(h2). The set of all normal and convolution invertible linear maps γ : H −→K satisfying γ ◦α = γ is denoted bywhich is a group under convolution.

Lemma 1.7The set of convolution invertible lazy 2-cocycle on (H,α) denoted byis a group.

Proposition 1.8D1:is a group homomorphism, whose image denoted by(its elements are called lazy 2-coboundary), is contained in the center ofThus we call quotient groupthe second lazy cohomology group of H.

2 Main Results and Examples

In this section,we investigate the relations between the left 2-cocycles σ on(B,β)andon,and also provide two non-trivial examples. In what follows,let(H,α)be a Hom-Hopf algebra with bijective antipode S andRadford biproduct Hom-Hopf algebra such that α2=id.

First we give some useful formulas. The Hom-coalgebra structure on (B ⊗B,β ⊗β) inis given by

So by (2.1), if σ,τ :B ⊗B −→K are morphisms intheir convolution inis given by

Let σ :B ⊗B −→K be a morphism inthat is, it satisfies the conditions

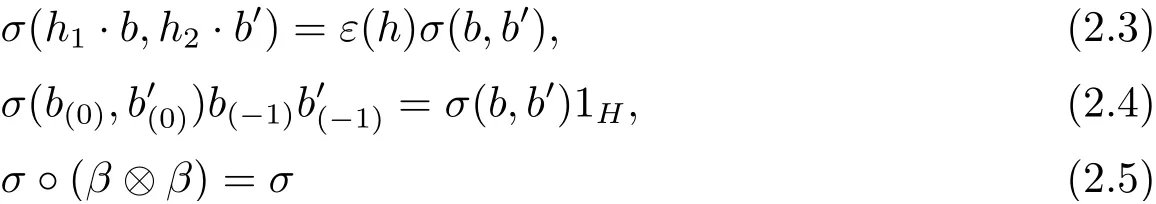
Lemma 2.1For a morphism, we can get the following useful formula

for all a,b ∈B and h ∈H.

ProofWe can check that as follows

Definition 2.2Let σ :B ⊗B −→K be a morphism inThen σ is lazy inif it satisfies the categorical laziness condition (for all

Definition 2.3Let σ :B ⊗B −→K be a morphism in. Then σ is a normal left 2-cocycle on (B,β) inif it is a normal morphism inand satisfies the categorical left 2-cocycle condition

for all a,b,c ∈B.

Proposition 2.4If we define a Hom-multiplication ·σon (B,β) by

(1) (σB,β) is a Hom-algebra if and only if σ is a normal left 2-cocycle in

(2) (σB,β) is a left (H,α) Hom-module algebra with the same action as (B,β).

Proof(1) For any b ∈B, it is easy to check that b ·σ1B= β(b) if and only if σ(b,1B)=ε(b) and 1B·σb=β(b) if and only if σ(1B,b)=ε(b). For any a,b,c ∈B, we have

and

Hence, if ·σis Hom-associative, we get

Applying ε to both sides of the above equation, we get (2.8).

Conversely, if σ is a left 2-cocycle inwe have

and

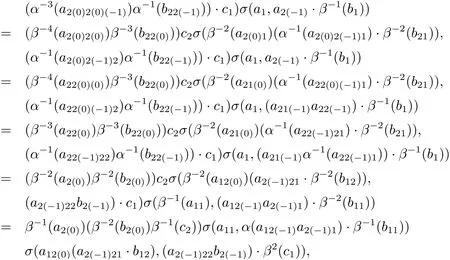
then we can get β(a)·σ(b·σc)=(a·σb)·σβ(c), i.e., ·σis Hom-associative.

(2) We check that(σB,β)is a left(H,α)-Hom-module algebra. Clearly,h·1B=ε(h)1Bfor any h ∈H. Next we only need to check the identityfor any h ∈H andIndeed, we have

the proof is completed.

Let γ :B −→K be a morphism inthat is

for all h ∈H and b ∈B.

If γ is a normal and convolution invertible linear map in, with convolution inverse γ−1in, the analogue of the operator D1is given inby

For a morphism γ : B −→K inthe laziness condition is identical to the usual one: γ(b1)b2=b1γ(b2) for all b ∈B.is a group in

Theorem 2.5(i) For a normal left 2-cocycle σ : B ⊗B −→K in, define σ :by

(iii) If σ is convolution invertible inthenis convolution invertible, with inverse

where σ−1is the convolution inverse of σ in

(v) If σ,τ :B ⊗B −→K are lazy 2-cocycles inthenhence the map σis a group homomorphism fromto

(vi) If γ : B −→K is a normal and convolution invertible morphism in, defineby

Proof(i) It is easy to see thatnormal. Sinceis a Hom-algebra, we have

Applying εB⊗εHto both sides of (2.16), thenis a normal left 2-cocycle on

We prove that the multiplication inandcoincide.

The uniqueness of σ follows easily by applying εB⊗εHto the multiplications inandWe check that as follows

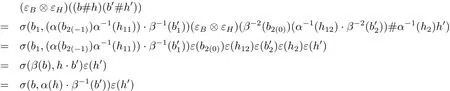
and

(ii) Let a,b,c ∈B and h,g,l ∈H, we have

Then

and

Let h=g =l=1H, we can get (2.8).

(iii)

and

then we can get

Applying id⊗ε to both sides of the above equation and let h=1H,we get σ is lazy in

(v) Using (2.2) for the convolution inwe compute

Hence we have indeed D1(γ)=. If γ is lazy inthen we have

Remarks(1)is a group by Lemma 1.7.

(2) If σ,τ are left lazy 2-cocycles on (B,β) inwe can getare left lazy 2-cocycles onby(i)and(iv). Thenis a left lazy 2-cocycle onCombining (ii) with (v), we have σ ∗τ is a left lazy 2-cocycle on (B,β) in

In a word, the set of convolution invertible lazy 2-cocycle on (B,β) indenoted byis a group.

Proposition 2.6(B,β)(B,β) is a group homomorphism in,whose image denoted by(B,β)(its elements are called lazy 2-coboundary inis contained in the center ofThus we call quotient groupthe second lazy cohomology group of (B,β) in

ProofIt is easy to check thatis a group homomorphism inNow we proveis contained in the center of

Then σ ∗D1(γ)=D1(γ)∗σ. The proof is completed.

Proposition 2.7If σ is a lazy 2-coboundary for (B,β) inthenis a lazy 2-coboundary forso the group homomorphismβ ⊗α),σfactorizes to a group homomorphism

ProofIt follows immediately from (vi) in Theorem 2.5.

Example 2.8Let A = sp{1A,z} and the automorphism β : AA, β(1A) =1A,β(z)=−z. Then (A,β) is a Hom-algebra with multiplication: 1A1A=1A,1Az =z1A=−z,z2=0, and (A,β) is a Hom-coalgebra with comultiplication and counit

Let H =sp{1H,g} be the group Hopf algebra with g2=1Hand ∆(g)=g ⊗g,SH(g)=g =g−1. Then (H,idH) is a Hom-Hopf algebra.

Define ·:H ⊗A −→A such that 1H·1A=1A,1H·z =−z,g·1A=1A, and g·z =z. It is easy to check (A,β) is a left (H,idH)-module Hom-algebra and module Hom-coalgebra.

Define ρ:A −→H ⊗A such that ρ(1A)=1H⊗1Aand ρ(x)=g ⊗(−z). We get (A,β)is a left (H,idH)-comodule Hom-algebra and comodule Hom-coalgebra. Then we can get a Radford biproduct Hom-bialgebra(see [5]).

Define σ :A ⊗A −→K by

where ∀s ∈K.

Then we can check that σ is normal left 2-cocycle on (A,β) inand σ is lazy inBy Theorem 2.5,is defined as follows

Let γ(1A) = 1,γ(z) = 0. Then γ is normal and lazy in. By Theorem 2.5,γ is defined as follows

Example 2.9Let KZ2= K{1,a} be Hopf group algebra. Then (KZ2,id) is a Hom-Hopf algebra. Let T2,−1=K{1,g,x,y|g2=1,x2=0,y =gx,gy =−yg =x} be Taft’s Hopf algebra, its coalgebra structure and antipode are given by

and S(g) = g,S(x) = y,S(y) = −x. Define a linear map α :by α(1) =1,α(g)=g,α(x)=−x,α(y)=−y. Then α is an automorphism of Hopf algebra.

So we can get a Hom-Hopf algebraDefine module action

Then by a routine computation we can get () is a (KZ2,id)-module Hom-algebra.Therefore, (Hα#KZ2,α ⊗id) is a smash product Hom-algebra.

Define comodule action ρ:HαKZ2⊗Hαby

Then we can get (Hα,ρ,α) is a left (KZ2,id)-comodule Hom-coalgebra. Therefore (Hα×KZ2,α ⊗id) is a smash coproduct Hom-coalgebra.

Then we can get a Radford biproduct Hom-bialgebra

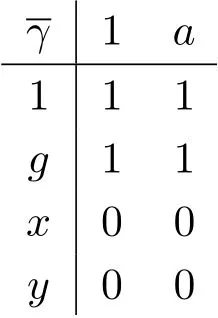
Define σ :Hα⊗Hα−→K by

where ∀s ∈K.

Then we can check that σ is a normal left 2-cocycle on (Hα,α) in, and σ is lazy inBy Theorem 2.5,is defined as follows andis a normal lazy left 2-cocycle on

Let γ(1) = 1,γ(g) = 1,γ(x) = 0,γ(y) = 0. Then γ is normal and lazy inBy Theorem 2.5is defined as follows