Some Generalized Normal Subgroups and p-nilpotency of Finite Groups
2018-07-06ZHONGGuoLINShiXunCHENQileQINLihua
ZHONG Guo,LIN Shi-Xun,CHEN Qi-le,QIN Li-hua
(1.Faculty of Science and Technology,University of Macau,Macau SAR,China;2.School of Mathematics and Statistics,Zhaotong University,Zhaotong 657000,China;3.School of Software Engineering,South China University of Technology,Guangzhou,Guangdong,China;4.College of Mathematics and Computer Science,Guangxi Normal University for Nationlities,Chongzuo Guangxi,China.)
§1. Introduction
All groups in this paper are assumed to be finite.We use standard terminology and notions on groups.For more details see[2].
In the study of finite groups,the notion of normal subgroup plays an important role.There are many generalizations of normality in finite groups.Kegel[6]introduced the concept of S-quasinormal subgroup of a group G to describe the subgroup that permutes with every Sylow subgroup of G and every S-quasinormal subgroup is a subnormal subgroup.Then Ballester[1]generalized S-quasinormal subgroups to S-quasinormally embedded subgroups.H is said to be S-quasinormally embedded in G if for each prime p dividing|H|,a Sylow p-subgroup of H is also a Sylow p-subgroup of some S-quasinormal subgroup of G.It is clear that S-quasinormally embedded subgroups generalize normally embedded subgroups,too.Recently,Huang[4]noted the concept of subnormally embedded subgroups in[2].H is said to be subnormally embedded in G if for each prime p dividing|H|,a Sylow p-subgroup of H is also a Sylow p-subgroup of some subnormal subgroup of G.There is another generalization of S-quasinormal subgroups in[15].H is called s-semipermutable in G if H permutes with every Sylow p-subgroup Gpof G with(|H|,p)=1.An s-semipermutable subgroup is not needed to be a subnormal subgroup.
In recent years,it has been more and more popular to use supplement properties of subgroups to characterize properties of a group.For example,Wang[10]introduced the concept of cnormal subgroups.A subgroup H of a group G is said to be a c-normal if there exists a normal subgroup K such that G=HK and H∩K≤HG,where HGis the maximal normal subgroup of G contained in H.In 2008,Li[7]introduced the concept of ss-quasinormal subgroups.H is said to be an ss-quasinormal subgroup of G if there is a subgroup B such that G=HB and H permutes with every Sylow subgroup of B.
Let A/B be a chief factor of a group G.We say that(i)H covers A/B if HA=HB;(ii)H avoids A/B if H∩A=H∩B.H is called a CAP-subgroup of G if H either covers or avoids any G-chief factor.In 2011,Wei[12]introduced the concept of c#-normal subgroups.H is called c#-normal in G if there is a normal subgroup K of G such that G=HK and H∩K is a CAP-subgroup of G.The c#-normality is a common generalization of c-normality and CAP-subgroups.
By applying the above concepts to investigate the structure of finite groups,many meaningful results have been obtained,such as[1,4,7-11,15].Based on the observation of the above concepts,it is natural to ask the following question:whether some of these concepts and the related results can be uni fied and generalized?The purpose of this article is to present an answer to the above question.The aim of this article is to unify and improve some earlier results using c#-normal,s-semipermutable,subnormally embedded and ss-quasinormal subgroups.
§2. Preliminaries
In this section,we recall some known results that are useful later.
Lemma 1[15]Suppose that H is an s-semipermutable subgroup of G.Then
(1)If H≤K≤G,then H is s-semipermutable in K;
(2)Let N be a normal subgroup of G.If H is a p-group for some prime p∈ π(G),then HN/N is s-semipermutable in G/N;
(3)If H≤Op(G),then H is s-permutable in G.
Lemma 2[8,Lemma2.1]Let S be a CAP-subgroup of a group G andThen SN/N is a CAP-subgroup of G/N.
Lemma 3[12,Lemma2.5]Let G be a group,H a c#-normal subgroup of G and N a normal subgroup of G.Then
(1)If N≤H,then H/N is a c#-normal subgroup of G/N;
(2)Let π be a set of primes,H a π-group and N a π0-group.Then HN/N is a c#-normal subgroup of G/N.
Lemma 4[4,Lemma1.2]Let G be a group,H a subnormally embedded subgroup of G and N a normal subgroup of G.Then
(1)If H≤M≤G,then H is subnormally embedded in M;
(2)HN/N is subnormally embedded in G/N.
Lemma 5[7,Lemma2.1]Let H be an ss-quasinormal subgroup of a group G.
(1)Ifthen H is ss-quasinormal in L;
(2)Ifthen HN/N is ss-quasinormal in G/N.
Lemma 6[2,I.7.5Proposition]Let G be a group.If V is a normally embedded subgroup of G,then V is a CAP-subgroup of G.
Lemma 7[12,Corollary3.2]Let P be a Sylow p-subgroup of a group G,where p is a prime divisor of|G|with(|G|,p−1)=1.Then G is p-nilpotent if and only if every maximal subgroup of P is c#-normal in G.
Lemma 8[11,Lemma2.7]Let G be a group and p a prime dividing|G|with(|G|,p−1)=1.
(1)If N is normal in G of order p,then N≤Z(G);
(2)If G has a cyclic Sylow p-subgroup,then G is p-nilpotent;
(3)If M is a maximal subgroup of G and|G:M|=p,then
Lemma 9[2,ALemma1.2]Let U,V and W be subgroups of a group G.The following statements are equivalent.
(1)
(2)
§3.Main Results
Firstly,we unify and generalize the s-semipermutable subgroups and c#-normal subgroups,and then give the following:
Theorem 1Let G be a group and P a Sylow p-subgroup of G,where p is the smallest prime dividing|G|.If every maximal subgroup of P is either s-semipermutable or c#-normal in G,then G is p-nilpotent.
ProofAssume that the theorem is false and let G be a counter-example with minimal order.To derive a contradiction,We will divide into several steps.
(1)G has a unique minimal normal subgroup N such that G/N is p-nilpotent and Φ(G)=1.
Let N be a minimal normal subgroup of G.We will show that quotient group G/N satis fies the hypotheses of the theorem.Let M/N be a maximal subgroup of PN/N.It is clear that M=P1N for some maximal subgroup P1of P.By the hypotheses,P1is either s-semipermutable or c#-normal in G.If P1is s-semipermutable in G,then M/N=P1N/N is s-semipermutable in G/N by Lemma 1.If P1is c#-normal in G,then there is a normal subgroup K1of G such that G=P1K1and P1∩K1is a CAP-subgroup of G.Then

It is easy to see that K1N/N is normal in G/N.Since
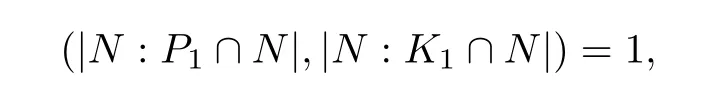
(P1∩N)(K1∩N)=N=N∩G=N∩(P1K1).Now using Lemma 9,we have that

It follows thatis a CAP-subgroup of G/N by Lemma 2.Hence M/N is c#-normal in G/N.Therefore,G/N satis fies the hypotheses of the theorem.The choice of G yields that G/N is p-nilpotent.The uniqueness of N and Φ(G)=1 are obvious.
(2)Op0(G)=1.
Ifthen N≤Op0(G)by(1).By Lemma 1 and 3,G/N satis fies the hypotheses,hence G/N is p-nilpotent.Now the p-nilpotency of G/N implies the p-nilpotency of G,a contradiction.
(3)Op(G)=1 and G is not solvable.
Ifyields N ≤ Op(G)andTherefore,G has a maximal subgroup M such that G=MN and M∩N=1.Since Op(G)∩M is normalized by N and M,Op(G)∩M is normal in G.The uniqueness of N yields N=Op(G).Clearly,P=NMp=N(P∩M).Since P∩M is a group.Then P1M=M or G by maximality of M.If P1M=G,then P=P∩P1M=P1(P∩M)=P1,which is a contradiction.If P1M=M,then P1≤M.Therefore,P1∩N=1 and N is of prime order.Then the p-nilpotency of G/N implies the p-nilpotency of G,a contradiction.Combining this with(2),it is clear that G is not solvable,thus(3)holds. (4)All maximal subgroups of P are s-semipermutable in G. If P has a maximal subgroup P1which is c#-normal in G,then there is a normal subgroup K such that G=P1K and P1∩K is a CAP-subgroup of G.(3)implies P1∩K cannot cover N/1 and P1∩K must avoid N/1,namely,P1∩K∩N=1.Since,N≤K by(1).Then P1∩K∩N=P1∩N=1.It follows that|P∩N|≤p.Then N is p-nilpotent by Lemma 8.Let Np0be the normal p-complement of N and soBy(2)we know Np0=1.So N is a p-group,which is a contradiction with(3). (5)For anyPQ By Lemma 8 we know that P is non-cyclic.Hence we can find two maximal subgroups P1,P2of P such that P=P1P2.By the hypotheses PiQ is a group,i=1,2,where Q∈Sylq(G).Hence PQ is a group.By(3)and the famous paqb-theorem we know that PQ is a proper subgroup of G.Hence PQ is p-nilpotent by the minimality of G. (6)The final contradiction. By(5)we have[P,Q]≤Q for any.Suppose that S1is an arbitrary subgroup of P.Set NG(S1)=N1.Sincewhere(N1)q∈Sylq(N1),S1is centralized by(N1)p0.Thus G is p-nilpotent by the famous Frobenius Theorem([13,10.3.2]).This is the final contradiction and the proof is complete. We study the in fluence of c#-normal and ss-quasinormal subgroups on p-nilpotency of a group. Theorem 2Let P be a Sylow p-subgroup of a group G,where p is a prime divisor of|G|with(|G|,p−1)=1.If every maximal subgroup of P is either c#-normal or ss-quasinormal in G,then G is p-nilpotent. ProofLet H be a maximal subgroup of P.We will prove H is c#-normal in G.If H is ss-quasinormal in G,then there is a subgroup B≤G such that G=HB and HX=XH for all X∈Syl(B).From G=HB,we obtain|B:H∩B|p=|G:H|p=p,and hence H∩B is of index p in Bp,a Sylow p-subgroup of B containing H ∩B.Thus S 6⊆ H for all S ∈ Sylp(B)and HS=SH is a Sylow p-subgroup of G.In the light of|P:H|=p and by comparison of orders,S∩H=B∩H,for all S∈Sylp(B).So We claim that B has a Hall p0-subgroup.Asor 1,it follows thator 1.Since(|G|:p−1)=1,then B/Op(B)is p-nilpotent by Lemma 8,and so B is p-solvable.Thus B has a Hall p0-subgroup.It follows that the claim holds.Now,let K be a p0-subgroup of B,π(K)={p2,...,ps}and Pi∈ Sylpi(K).By the condition,H permutes with every Piand so H permutes with the subgroup hP2,...,Psi=K.Hence,HK≤G.Clearly,K is a Hall p0-subgroup of G and HK is a subgroup of index p in G.Let M=HK and soby Lemma 8.It follows that H is normally embedded in G,and so H is a CAP-subgroup of G by Lemma 6,in particular,c#-normal in G.Since every maximal subgroup of P is c#-normal in G,we have that G is p-nilpotent by Lemma 7. If we remove the hypothesis that p is the smallest prime,then we have the following theorem: Theorem 3Let P be a Sylow p-subgroup of a group G,where p is an odd prime divisor of|G|.If NG(P)is p-nilpotent and every maximal subgroups of P is either subnormally embedded or ss-quasinormal in G,then G is p-nilpotent. ProofAssume that the theorem is not true and let G be a counter-example of minimal order.We will derive a contradiction in following steps. (1)Op0(G)=1. Ifwe consider factor group G/Op0(G).By Lemma 4 and Lemma 5,it is easy to see that every maximal subgroup of POp0(G)/Op0(G)is either subnormally embedded or ss-quasinormal in G/Op0(G).Since is p-nilpotent,G/Op0(G)satis fies all the hypotheses of theorem.The minimality of G yields that G/Op0(G)is p-nilpotent,and so G is p-nilpotent,a contradiction. (2)If M is a proper subgroup of G with P≤M It is easy to seeand so NM(P)is p-nilpotent.From Lemma 4 and Lemma 5,we immediately get that M satis fies the hypotheses of theorem.Now,by the minimality of G,M is p-nilpotent. (3)G=PQ is solvable,where Q is a Sylow q-subgroup of G with Since G is not p-nilpotent,by a result of Thompson[14,Corollary],there exists a non-trivial characteristic subgroup T of P such that NG(T)is not p-nilpotent.Choose T such that the order of T is as large as possible.Since NG(P)is p-nilpotent,we have NG(K)is p-nilpotent for any characteristic subgroup K of P satisfying T (4)G has a unique minimal normal subgroup N such that G/N is p-nilpotent.Furthermore Φ(G)=1. By(3),G is solvable.Let N be a minimal subgroup of G.Then N≤Op(G)by(1).Consider factor group G/N.It is easy to see that every maximal subgroup of P/N is either subnormally embedded or ss-quasinormal in G/N.Sinceis p-nilpotent,we have G/N satis fies the hypothesis of the theorem.The choice of G yields that G/N is p-nilpotent.Consequently,the uniqueness of N and the fact that Φ(G)=1 are obvious. (5)If a maximal subgroup P0of P is subnormally embedded in G,then P0is normally embedded in G. If P0is not normally embedded in G,then there is a subnormal subgroup L of G such that P0∈Sylp(L).It follows that there exists normal subgroup L1of G such thatandHence,|L1|p>|P0|and so|L1|p=|P|and P≤L1 (6)The final contradiction. By step(4),there exists a maximal subgroup M of G such that G=MN and M∩N=1.Since N is elementary abelian p-group,N≤CG(N)and.By the uniqueness of N,we have CG(N)∩M=1 and N=CG(N).But N≤Op(G)≤F(G)≤CG(N),hence N=Op(G)=CG(N).If|N|=p,then Aut(N)is a cyclic group of order p−1.If q>p,then NQ is p-nilpotent and therefore Q≤CG(N)=N,a contradiction. On the other side,if q is isomorphic to a subgroup of Aut(N)and therefore M,and,in particular,Q is cyclic.Since Q is a cyclic group and q From Theorem 3 the following corollary is obtained. Corollary 1Let p be an odd prime dividing the order of a group G and H a normal subgroup of G such that G/H is p-nilpotent.If NG(P)is p-nilpotent and there exists a Sylow p-subgroup P of H such that every maximal subgroup of P is either subnormally embedded or ss-quasinormal in G,then G is p-nilpotent. ProofBy Lemma 4 and Lemma 5,every maximal subgroup of P is either subnormally embedded or ss-quasinormal in H.By Theorem 3,H is p-nilpotent.Now,let Hp0be the normal p-complement of H.ThenIf,then we consider G/Hp0.It is easy to see that G/Hp0satis fies all the hypotheses of the Corollary for the normal subgroup H/Hp0of G/Hp0by Lemma 4 and Lemma 5.Now by induction,we see that G/Hp0is p-nilpotent and so G is p-nilpotent.Hence we assume Hp0=1 and therefore H=P is a p-group.In the case,by our hypotheses,NG(P)=G is p-nilpotent. AcknowledgementsThe authors would like to thank the referee for helpful suggestions. [1]BALLESTER-BOLINCHES A,PEDRAZA-AGUILERA M C.Sufficient condition for supersolvability of if nite groups[J].J.Pure Appl.Algebra,1998:113-118. [2]DOERK H,HAWKES T.Finite Solvable Groups[M].Berlin-New York,1992. [3]GORENSTEIN D.Finite Groups[M].Chelsea,New York,1968. [4]Huang Q.Subnormally embedded subgroups and p-nilpotency of Finite Groups[J].Guangxi Science,2011,18:325-328. [5]HUPPERT B.Endliche Gruppen I[M].Springer-verlag,Berlin,New York,1967. [6]KEGEL O H.Sylow-gruppen und subnormalteiler endlicher gruppen[J].Math.Z.,1962,78:205-221. [7]LI S,SHEN Z,LIU J,et al.The in fluence of ss-quasinormallity of some subgroups on the structure of finite groups[J].J.Algebra,2008,319:4275-2487. [8]LIU J,LI S,SHEN Z.Finite groups with some CAP-Subgroups[J].Indian J.Pure Appl.Math.,2011,42:145-156. [9]LI Y,WANG Y,WEI H.On p-nilpotency of finite groups with some subgroups π-quasinormally embedded.Acta Math.Hungar.,2005,108:283-298. [10]WANG Y.C-normality of groups and its properties.J.Algebra,1996,180:954-965. [11]WEI H,WANG Y.On c∗-normality and its properties[J].J.Group Theory,2007,10:211-223. [12]WEI H,WANG Y.C#-normality of groups and its properties[J].Algebr.Represent Theor.,2013,16:193-204. [13]ROBINSON D J S.A Course in the Theory of Groups[M].Berlin-New York,1993. [14]THOMPSON J G.Normal p-complementsfor finite groups[J].J.Algebra,1964,1:43-46. [15]ZHANG Q,WANG L.The in fluence of s-semipermutable subgroups on the structure of a finite group[J].Acta Math.Sinica,2005,48:81-88.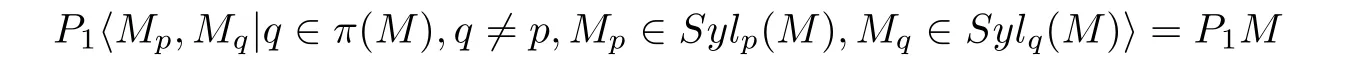


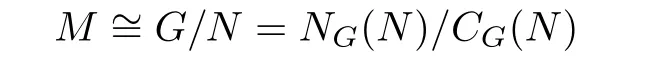
杂志排行
Chinese Quarterly Journal of Mathematics的其它文章
- Common Fixed Point Results for Quasi-contractions Involving Altering Distance Functions in Ordered Cone Metric Spaces
- The Growth Order of Solutions of Systems Complex Di ff erence Equations
- The{P,k+1}-re flexive Solution to System of Matrix Equations AX=C,XB=D
- The Credibility Estimators under MLINEX Loss Function
- Implicative Pseudo Valuations on Hoops
- An Elliptic Gradient Estimate for A Non-homogeneous Heat Equation on Complete Noncompact Manifolds
