一类微分多项式的零点的不等式估计
2017-10-13戴瑞芳徐俊峰
戴瑞芳,徐俊峰
一类微分多项式的零点的不等式估计
戴瑞芳,徐俊峰
(五邑大学 数学与计算科学学院,广东 江门 529020)
本文利用精简计数函数给出了微分多项式的定量估计不等式,设为超越亚纯函数,为正整数,其中为的小函数满足.
亚纯函数;微分多项式;小函数;值分布
1 引言与定理
1995年,Bergweiler和Eremenko[2]证明了当时定理成立.
1993年,Chung等[3]猜测为超越亚纯函数,为正整数,取到任意非零有限值无限多次.
1998年,Zhang等[4]证明了以下结果:
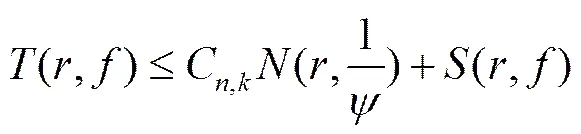
Alotaibi[6]将上述定量的计数函数改为更精确的精简计数函数,得到下述定量结果:

,
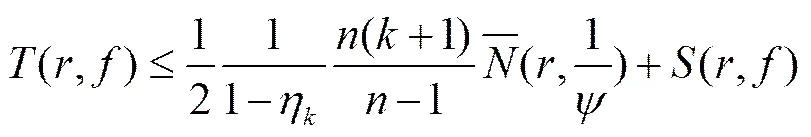
其中,
.
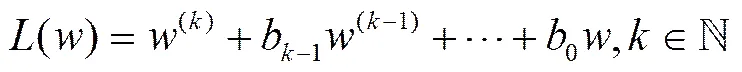
, (2)
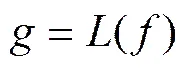
. (4)
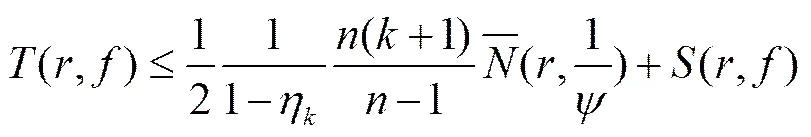
.
注2:最近Jiang[8]利用定理4的方法证明了如下结果:设为超越亚纯函数,均为的整数,为非零常数,我们可得:
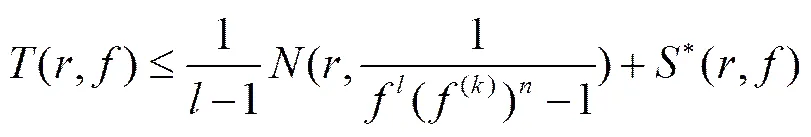
2 引理
本文定理6的证明需要用到以下引理
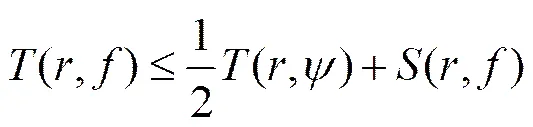
.
由式(1),(3)和(4)得
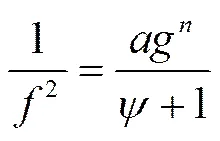
因此
所以

据此完成引理1的证明.
3 定理6的证明
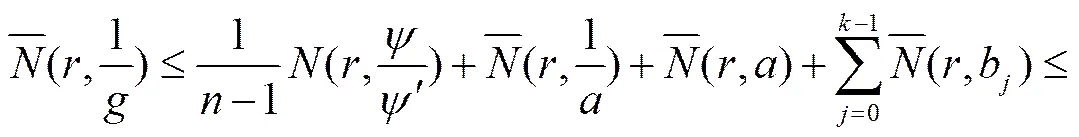
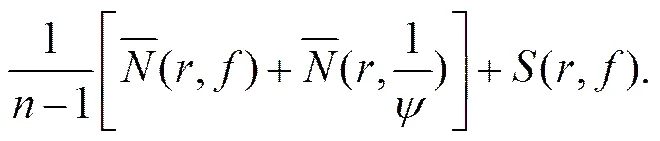
.
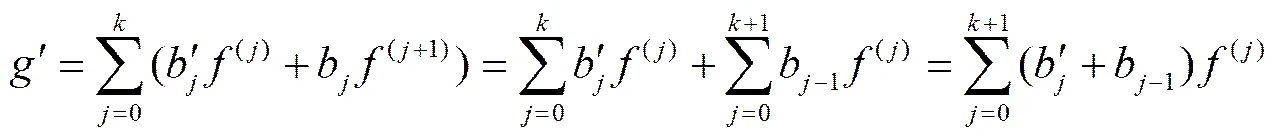
, (6)
其中
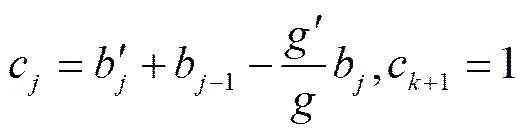
设
. (8)
对式(6)使用莱布尼兹法则得

, (9)

由式(7)得
,
及
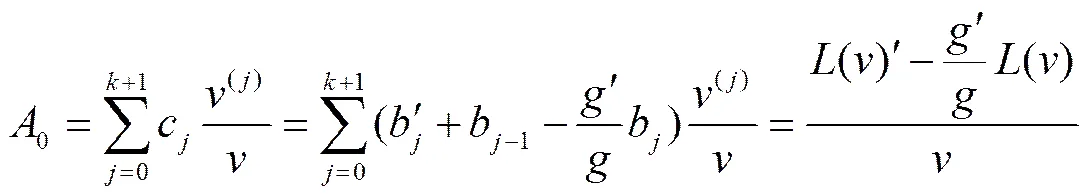
. (12)
我们分两种情形讨论:
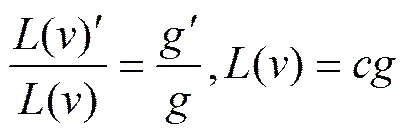
,.
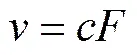
,.

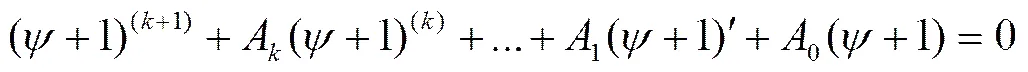
,
,
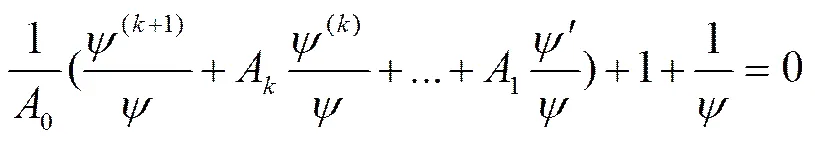
因此
由式(10)得
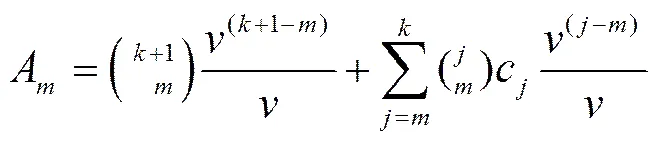
由式(5)得
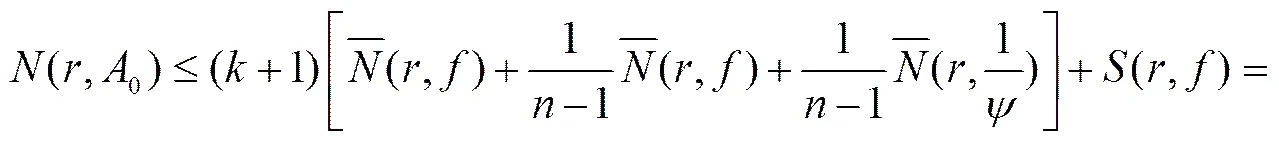
(14)
由式(1),(3)和(4)得
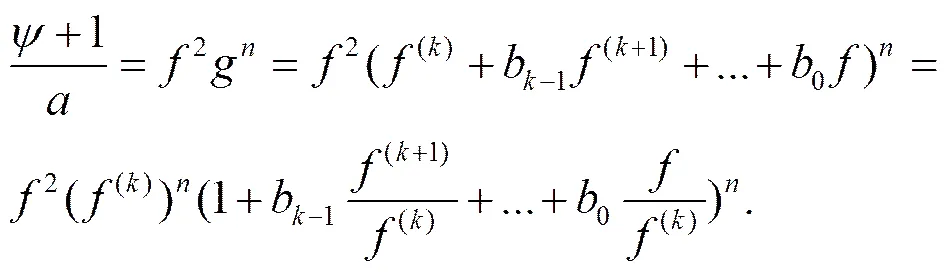
(15)
由式(14)和(15)得
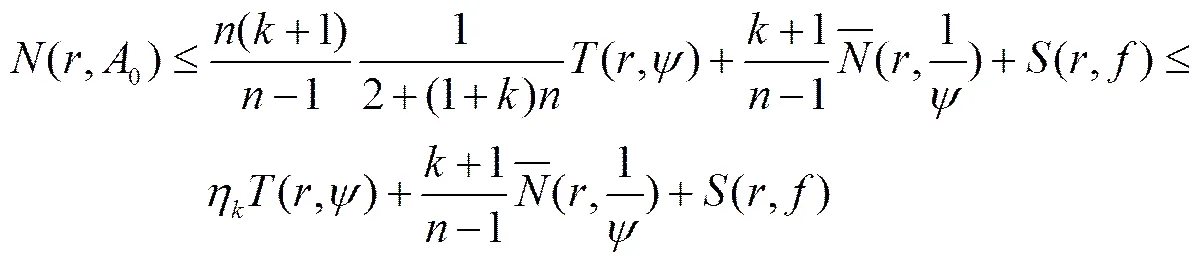
由式(10)和(13)得
, (17)
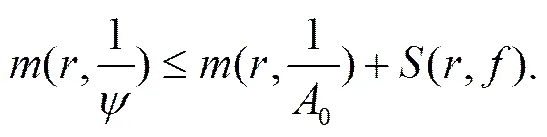
由式(16),(17)和(18)得
所以
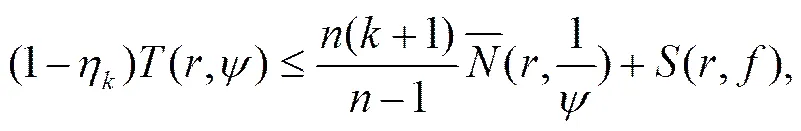
由引理1得
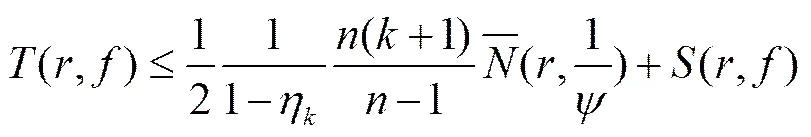
我们完成定理6的证明.
4 例子
[1] HAYMAN W. Picard values of meromorphic function and their derivati-ves [J]. Ann of Math, 1959, 70(2): 9-42.
[2] BERGWEILERAND W, EREMENKO A. On the singularities of the inverse to a meromorphic function of finite order [J]. Rev Mat Iberoamericana, 1995, 11: 355-373.
[3] YANG Chungchun, YANG Le, WANG Yuefei. On the zeros of[J]. Chinese Science Bulletin, 1993, 39(8): 2215-2218.
[4] ZHANG Zhongfa, SONG Cuodong. On the zeros of[J]. Chinese Ann Math Ser A, 1998, 19(2): 275-282.
[5] LI Ping, YANG Chungchun. On the value distribution of a certain type of differrential polynomials [J]. Mh Math, 1998, 125: 15-24.
[6] ALOTAIBI A. On the zeros offor[J]. Computational Methods and Function Theory, 2004, 4(1): 227-235.
[7] DOERINGER W. Exceptional values of differential polynomials[J]. Pacific J Math, 1982, 98(1): 1363-1364.
[8] JIANG Yan. A note on the value distribution offor[J]. Bull Korean Math Soc, 2016, 53(2): 365-371.
[9] XU Junfeng, YI Hongxun, ZHANG Zhanling. Some inequalities of differential polynomials II [J]. Mathematical Inequalities & Applications, 2011, 14(1): 93-100.
[10] HAYMAN W, MILES J. On the growth of a meromorphic function and its derivatives [J]. Complex Variables Theory Appl, 1989, 12(1-4): 245-260.
[责任编辑:韦 韬]
An Inequality Estimate of Differential Polynomials on the Zeros
DAIRui-fang, XUJun-feng
(School of Mathematics and Computational Science, Wuyi University, Jiangmen 529020, China)
In this paper, a quantitative estimate of the value distribution of the differential polynomialsis obtained by the reduced counting function, whereare positive integer,is the small function ofwhich satisfies.
meromorphic functions; differential polynomials; small functions; value distributions
1006-7302(2017)03-0001-07
0174.52
A
2017-03-09
广东省自然科学基金资助项目(2016A030313002);广东高校优秀青年教师培养对象资助项目(Yq2013159)
戴瑞芳(1992—),女,广东新会人,在读硕士生,研究方向为复分析;徐俊峰,教授,博士,硕士生导师,通信作者,研究方向为复分析.
