带有p-Laplacian算子的分数阶微分方程多点边值问题正解的存在性
2017-07-31马德香
张 艳,马德香
(华北电力大学数理学院 北京 102206)
带有p-Laplacian算子的分数阶微分方程多点边值问题正解的存在性
张 艳,马德香
(华北电力大学数理学院 北京 102206)
在以下带有p-Laplacian算子的分数阶微分方程多点边值问题中:
其中Dα,Dβ是Riemann-Liouville分数阶导数,f∶[0,+∞)→[0,+∞)是连续函数,文章的新奇之处在于运用Guo-Krasnoselskii不动点定理来研究了一类含参量的带有p-Laplacian多点边值问题正解的存在性及不存在性.
分数阶微分方程;p-Laplacian算子;多点边值问题;不动点定理
0引言
分数阶微分方程边值问题近年来得到了很多学者的关注,具体见参考文献[1-7],然而直到近几年带有p-Laplacian算子的分数阶微分方程边值问题的研究才开始兴起,这是因为由其建立的模型在多孔介质中的湍流现象,在流变学、材料科学、粘塑性力学等领域有着广泛应用,研究带有p-Laplacian算子的分数阶微分方程边值问题具有较大的研究前景.近年来有一些作者开始研究带有p-Laplacian算子的分数阶微分方程边值问题,例如在文献[8]中,作者陈泰永运用重合度理论研究了以下带有p-Laplacian算子的分数阶微分方程两点边值问题:

在文献[9]中,作者韩振来通过运用Guo-Krasnoselskii不动点定理研究了以下带有p-Laplacian算子的分数阶微分方程两点边值问题:

受以上文献启发,本文将运用Guo-Krasnoselskii不动点定理来研究以下边值问题
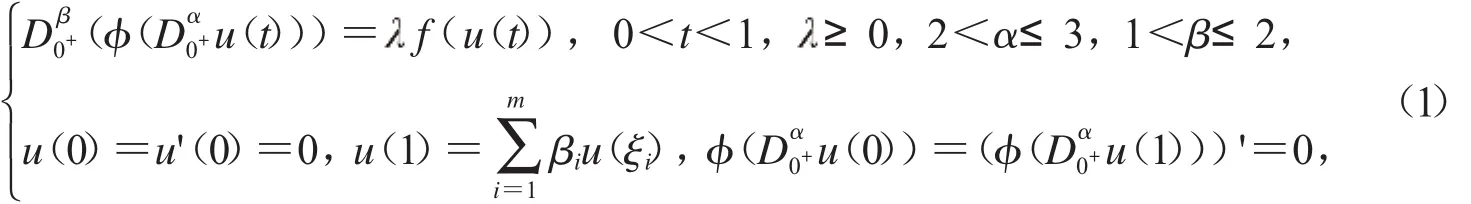
在本文中,假设以下是成立的:

(H2)f∶[0,+∞)→[0,+∞)是连续的;
(H3)φ∶R→R为非减奇函数,且存在非减同胚映射Ψ1,Ψ2,满足
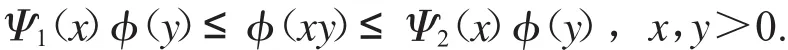
1 预备知识和引理
1.1 预备知识
1.1.1 α>0,定义函数y∶(0,∞)→R的α阶Riemann-Liouville分数阶积分为

其中Γ(·)是欧拉积分,等式右端在(0,∞)上逐点定义.
1.1.2 α>0,定义函数y∶(0,∞)→R的α阶Riemann-Liouville分数阶导数为
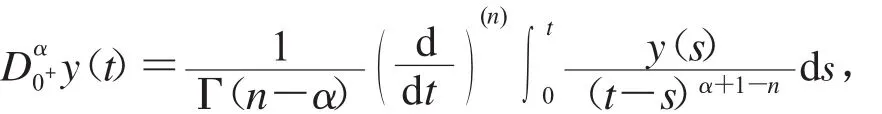
其中n=[α]+1,等式右端在(0,∞)上逐点定义.
1.2 引理
1.2.1 假设α>0,若u∈C(0,1)∩L(0,1)有α阶Riemann-Liouville导数,则

其中N为大于或等于α的最小整数.
1.2.2[10]设x∈C+[0,1]:={x x∈C[0,1],x(t)≥0,t∈[0,1]}.下列边值问题:


其中

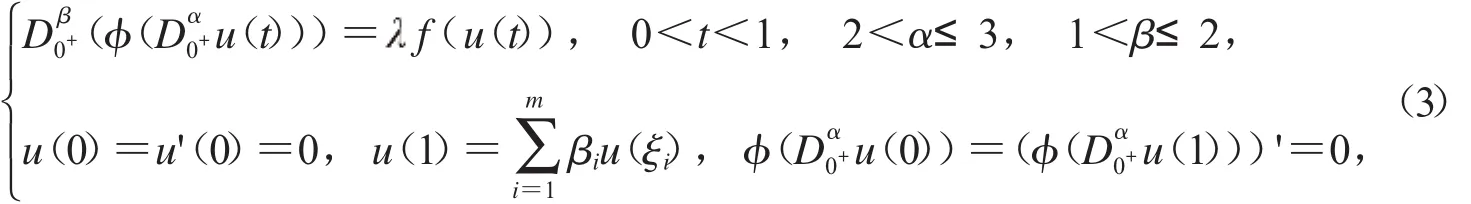
有唯一解
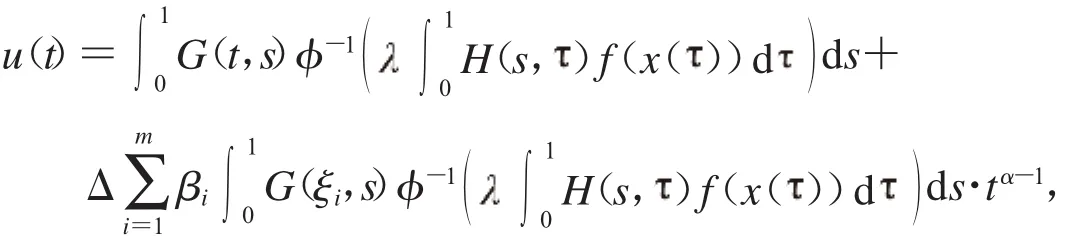
其中G(t,s)见式(2),而证明:

根据引理1.2.1和1<β≤2,得

由式(3),有

即
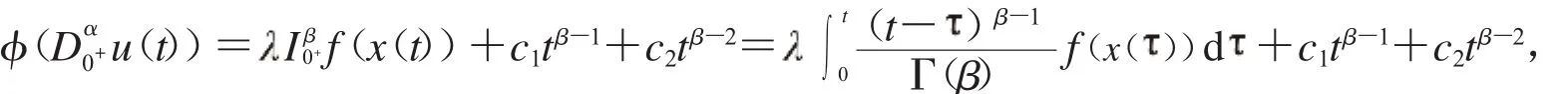


因此:


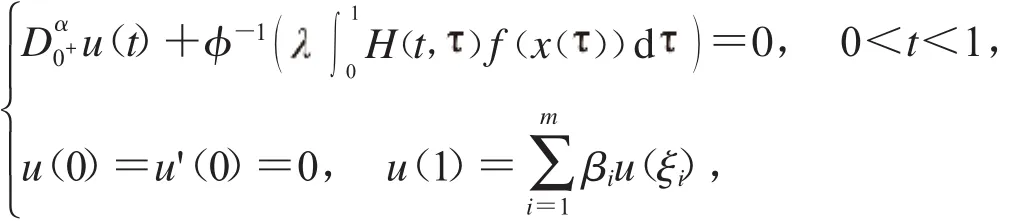
那么根据引理1.2.2,可知式(3)有唯一解

证明完成.
1.2.4[11]G(t,s)有下列性质:
(1)对于任意的(t,s)∈[0,1]×[0,1],G(t,s)≥0.(2)给定s∈[0,1],则对于任意的t∈[0,1],

(3)给定s∈[0,1],则对于任意的t∈[0,1],有G(t,s)≥ρ(t)G(t0,s),其中

1.2.5[12]H(s,)有以下性质:
1.2.7[14](Guo-Krasnoselskii不动点定理) E为Banach空间,P⊂E是一个锥,设Ω1,Ω2是E中的两个有界开子集,且θ∈Ω1⊂Ω1⊂Ω2,有T∶P∩(Ω2Ω1)→P为全连续算子,且满足
(1)Tu≤u,u∈P∩∂Ω1,Tu≥u,u∈P∩∂Ω2,或者
(2)Tu≥u,u∈P∩∂Ω1,Tu≤u,u∈P∩∂Ω2,
则算子T在P∩(Ω2Ω1)中存在不动点.
定义算子T∶P→P如下:

1.2.8 T∶P→P是全连续算子.
证明:由引理1.2.4,得

下面证明T的紧性,设Ω⊂P是有界的,则存在正常数M>0,对于所有的u∈Ω,有u≤M.令k=max+1.当u∈Ω,t∈[0,1]时,有

因此,T(Ω)是一致有界的.
另一方面,因为G(t,s)在[0,1]×[0,1]上是连续的,在[0,1]×[0,1]是一致连续的,因此对于任意的ε>0,存在δ>0,当t1,t2∈[0,1],当t1-t2<δ,有

对于所有的u∈Ω,

因此T(Ω)是等度连续的.再由Arzela-Ascoli定理,可知T是紧的,从而得到T∶P→P是全连续算子.证明完成.
2 正解的存在性与不存在性
为方便起见,引入以下符号,

2.1 正解存在性定理的证明

分数阶微分方程(1)至少有一个正解.(当f∞=+∞,F0=0时,记f-∞1=0,F0-1=+∞.)
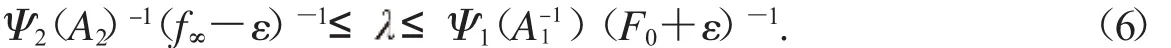
以下证明分为两部分.
第一步:由F0的定义可知,r1>0,

对于u∈P且u =r1,

取Ω1={u∈E<r1},有

第二步:由f∞的定义可知,r3>0
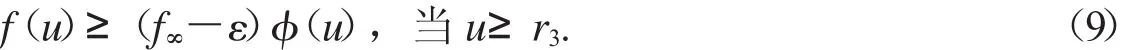
取r2> r3,如果u∈P且u =r2=max{2r1,r3},由式(6)和(9)可得

故Ω2={u∈E<r3}取,得

根据式(8)、(10)以及定理1.2.7可得,T中有一个不动点u∈P∩(Ω2Ω1)且满足r1≤u ≤r2,显然u就是分数阶微分方程边值问题(1)的正解.证明完成.

f0Ψ(1A-11)>F∞Ψ(2A-21)成立.那么对于则分数阶微分方程(1)至少有一个正解(.当f0=+∞,F∞=0,有f-01=0,F-∞1=+∞.)证明:当满足式(11),可知 ε>0,满足
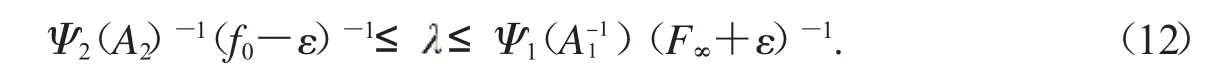
以下证明分为两部分.
第一步:由f0的定义可知,r1>0,

对于u∈P且u =r1,类似于定理2.1.1第二部分的证明方法,可取Ω1={u∈Eu <r1},有

第二步:选取R1>0
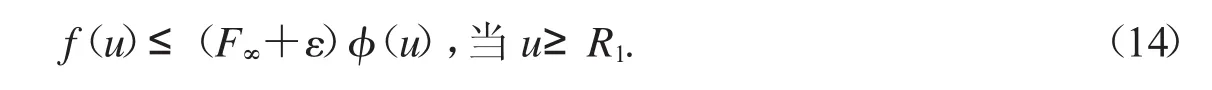
接下来,考虑两种情况:
情况一:假设f是有界的.N>0,有
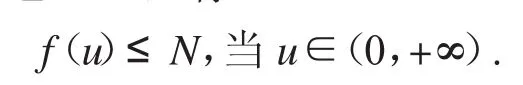
取r3=max{2r1,φ-(1N)A1},对u∈P且u=r3,有


情况二:假设f是无界的.则存在r4>max{2r1,R1}使得
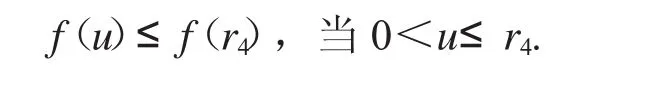
对于u∈P且u =r4,

取Ω4={u∈Eu<r4}有T u≤u ,当u∈P∩∂Ω4.
再由情况一和情况二可知,当Ω2={u∈Eu <r2=max{r3,r4}},有

根据定理1.2.7和式(13)、(15)可知,T中有一个不动点u∈P∩(Ω2Ω)1且满足r1≤u≤r2,显然u就是分数阶微分方程边值问题(1)的正解.证明完成.
2.1.3 假设r2>r1>0,有
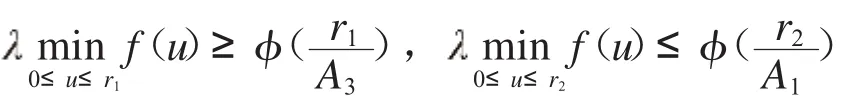
则分数阶微分方程(1)边值问题有一个正解u∈P且r1≤u ≤r2.
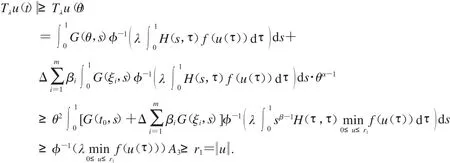
取Ω2={u∈Eu <r2}.当u∈P∩∂Ω2,有

因此,由定理1.2.7知,分数阶微分方程(1)的边值问题有一个正解u∈P且r1≤u ≤r2.证明完成.
2.1.4 假设f0=+∞,f∞=+∞.那么对于∈(0,1),则分数阶微分方程(1)边值问题至少有两个正解,其中
证明:令x(r)=由f的连续性可知,x(r)∶(0,+∞)→(0,+∞)是连续的.
一方面,根据f0=+∞,f∞=+∞,可得limx(r)=limx(r)=0.故存在r0∈(0,+∞),使得x(r0)=supx(r)=1.对于∈(0,1),存在常数a1,a(20<a1<r0<a2<+∞)有

则,

另一方面,根据f0=+∞,f∞=+∞,存在常数b1,b(20<b1<a1<r0<a2<b2<+∞)有
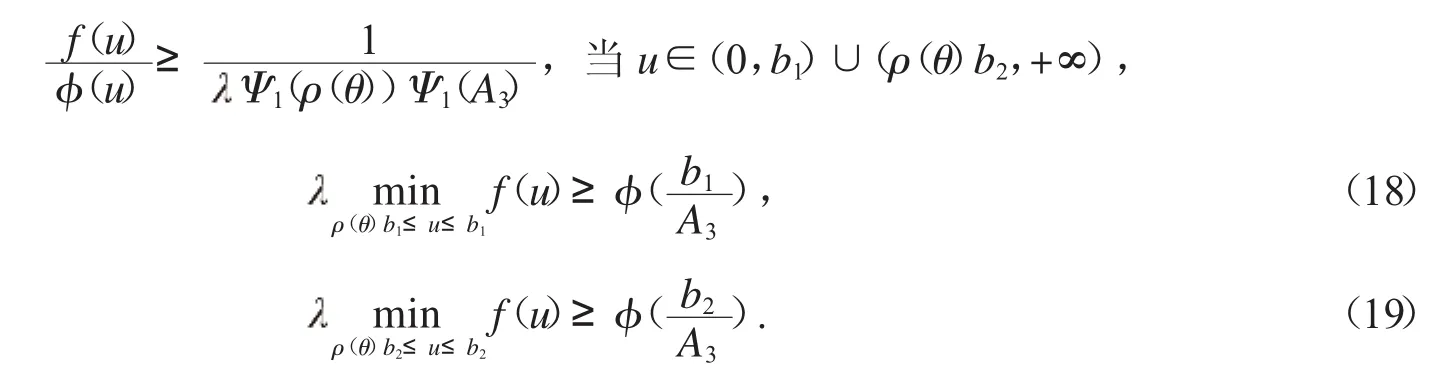
再由式(16)-(19)并根据定理2.1.3和定理1.2.7可得,当∈(0,1)时,分数阶微分方程边值问题(1)至少有两个正解.
2.2 正解不存在性定理的证明
2.2.1 假设F0<+∞,F∞<+∞.那么存在0>0,0<<0,分数阶微分方程边值问题(1)无正解.
证明:根据F0<+∞和F∞<+∞可知,存在正常数M1,M2,r1,r2,使得当r1<r2时,满足
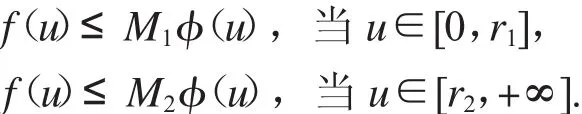

假设v(t)是分数阶微分方程边值问题(1)的正解.当0<<0:=M-01Ψ(1A-11),对于t∈[0,1],有T v(t)=v(t),因此有

与T v(t)=v(t)相矛盾,因此分数阶微分方程边值问题(1)无正解.证明完成.
2.2.2 假设f0>0,f∞>0.对于0>0,>0,分数阶微分方程边值问题(1)无正解.证明:根据f0>0和f∞>0,存在正常数m1,m2,r3,r4,使得当r3<r4,



与T v(t)=v(t)相矛盾,分数阶微分方程边值问题(1)无正解.证明完成.
3 例子
3.1 考虑边值问题:


容易验证:f∞Ψ(1A-11)>F0Ψ(2A-21).因此,由定理2.1.1知,当∈(3 843.197 5,4 960.3)边值问题(20)存在正解.
3.2 考虑以下边值问题:


3.3 考虑以下边值问题:
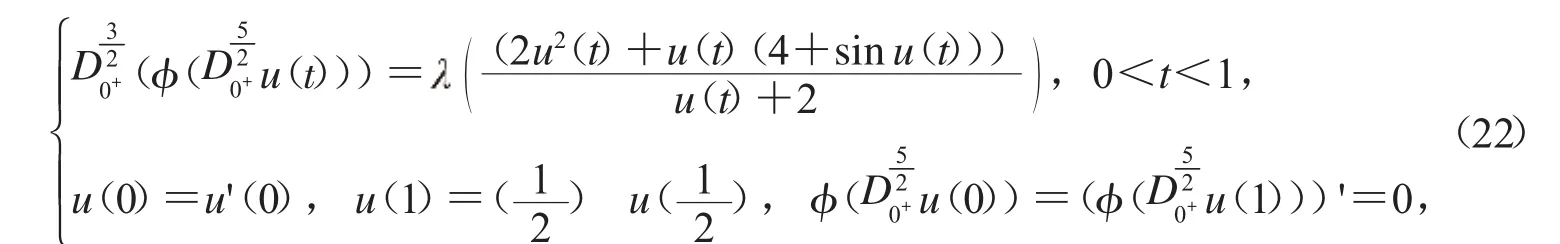
A1≈0.201 6,A2≈0.000 2,F0=f0=2,F∞=10,f∞=6并且u<f(u)<10u,
[1]MILLER K S,ROSS B.An introduction to the fractional calculus and fractional differential equation[M]. New York:Wiley-Interscience,1993.
[2]ROSS B.The fractional calculus and its applications,in:lecture notes in mathematics[M]//Lecture Notes in Mathematics.Berlin-New York:Springer-Verlag,1975.
[3]SAMKOSG,KILBASAA,MARICHEV O I.Fractional integrals and derivatives:theory and applications [M].Yverdon:Gordon and Breach,1993.
[4]KRASONSEL'SKIIMA.Positive solutionsofoperaterequations[M].Gronigen:PNoordhoff Ltd,1964.
[5]OLDHAMKB,SPANIERJ.Thefractionalcalculus[J].MathematicalGazette,1974,56(247):396-400.
[6]BAI Z,LÜ H.Positive solution for boundary value problem of nonlinear differential equation[J].Journal of Mathematical Analysis&Applications,2005,311(2):495-505.
[7]ZHAO Y,SUN S,HAN Z,et al.The existence of multiple positive solutions for boundary value problems ofnonlinearfractionaldifferentialequations[J].CommunicationsinNonlinearScience&Numerical Simulation,2011,16(4):2086-2097.
[8]CHEN T,LIU W,HU Z.A boundary value problem for fractional differential equation with p p math container loading mathjax-Laplacian operator at resonance[J].Nonlinear Analysis Theory Methods& Applications,2012,75(6):3210-3217.
[9]HAN Z,LU H,ZHANG C.Positive solutions for eigenvalue problems of fractional differential equation with generalized p-Laplacian[J].Applied Mathematics&Computation,2015,257:526-536.
[10]NYAMORADIN.Existenceofsolutions for multi-point boundary value problems for fractional differential equations[J].Arab J Math Sci,2012,18(2):165-175.
[11]MA D X.Positive solutions of multi-point boundary value problem of fractional differential equation[J]. Arab Journal of Mathematical Sciences,2015,21(2):225-236.
[12]EL-SHAHEDM.Positivesolutionsforboundaryvalueproblemof nonlinear fractional differential equation [J].Abs Appl Anal,2007,2007:1-8.DOI:10.1155/2007/10368.
[13]WANG H.On the number of positive solutions of nonlinear systems[J].Journal of Mathematical Analysis &Applications,2003,281(1):287-306.
[14]GUO D J,LAKSHMIKANTHAM V.Nonlinear problem in abstract cones[M].San Diego:AcademicPress,1988.
[15]SUN Y,ZHAO M.Positive solutionsfora classoffractionaldifferentialequations with integral boundary conditions[J].Applied Mathematics Letters,2014,34(1):17-21.
[16]ZHAOY,SUNS,HANZ,etal.Theexistenceofmultiplepositivesolutionsforboundaryvalueproblems ofnonlinearfractionaldifferentialequations[J].Communications in Nonlinear Science&Numerical Simulation,2011,16(4):2086-2097.
[17]XU X,JIANG D,YUAN C.Multiple positive solutions for the boundary value problem of a nonlinear fractional differential equation[J].Nonlinear Analysis Theory Methods&Applications,2009,71(10):4676-4688.
[18]AVERY R,HENDERSON J.Two positive fixed points of nonlinear operator on ordered Banach spacea [J].Comm Appl Nonlinear Anal,2001,8(1):27-36.
[19]BAIL,DAIB.Three solutionsfora p-Laplacian boundaryvalue problem with impulsive effects[J].Appl Math Comput,2011,217(24):9895-9904.
[20]FENG M.JI D,GE W.Positive solutions for a class of boundary value problem with integral boundary conditions in Banach space[J].J Comput Appl Math,2008,222(2):351-363.
[21]MA R,ZHANG J,FU S.The method of lower and upper solutions for fourth-order two-point boundary value problems[J].J Math Anal Appl,1997,215(2):415-422.
Existence of Positive Solution for
Multi-Point Boundary Value Problems of FractionalDifferential Equations with p-Laplacian
ZHANG Yan,MA Dexiang
(College of Mathematics and Physics,North China Universityof Electrical Power,Beijing102206,China)
In the following multi-point boundary value problem of fractional differential equation with p-Laplacian:
fractional differential equation;p-Laplacian operator;multi-point boundary value problem;fixed point theorem

O175.1;O175.8
A

1001-4217(2017)03-0029-13
2016-09-21
张 艳(1991—),女,硕士研究生.研究方向:分数阶微分方程边值问题研究. E-mail:zhuanzhufadai@sohu.com
