负二项分布概率最大值的性质

丁　勇

(南京医科大学 康达学院 数学与计算机教研室　江苏 连云港 222000)



负二项分布概率最大值的性质

丁勇

(南京医科大学 康达学院 数学与计算机教研室江苏 连云港 222000)

负二项分布概率的最大值是每次试验成功的概率p和首次试验成功次数r的函数.对确定的r，该函数是p的单调上升的连续函数，仅当(r-1)/p是整数时不可导；对确定的p，该函数是r的单调下降函数.

负二项分布； 概率最大值； 单调性

0　引言

在伯努利实验的家族中，作为几何分布的一种延伸，负二项分布是重要的离散型分布之一，在排队论、可靠性以及群团型生态格局分布等方面有着重要的应用[1-3]，有关该分布的理论和应用研究已取得很多成果[4-10].本文对负二项分布概率最大值的性质进行讨论，使我们对负二项分布有更深入的认识和理解.

1　负二项分布的最大值

在独立重复贝努里试验中，第r次成功的试验次数X是个随机变量，其一切可能值是r，r+1，……，X的概率分布称为负二项分布，且有[10〗

(1)

这里p为每次试验成功的概率.

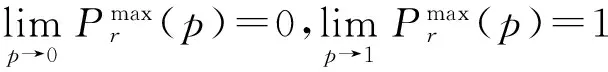
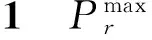
图1　负二项分布的图形(p=0.6，r=5)Fig.1　Graphs of the negative binomial distribution(p=0.6，r=5)

图2　负二项分布最大值(p) 的图形(p=0.001,0.002，…，0.999)Fig.2　Graphs of the maximumvalue (p) of negative binomial distribution(p=0.001,0.002，…，0.999)

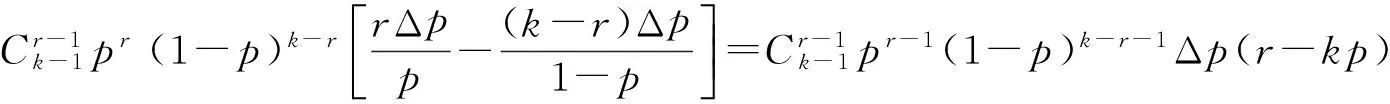
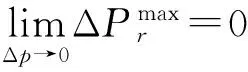
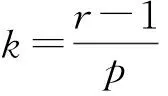
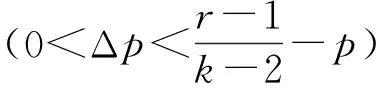
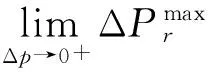
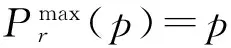
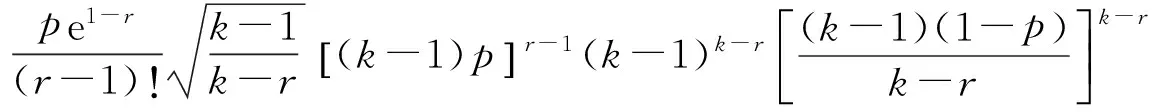
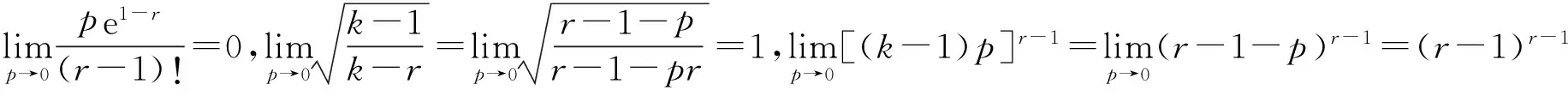
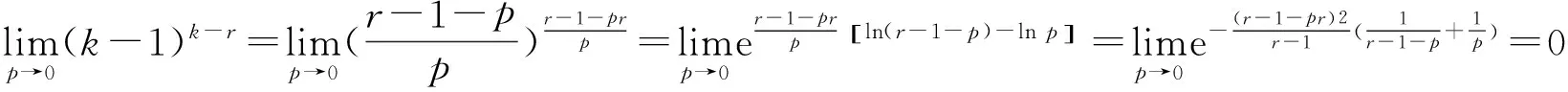
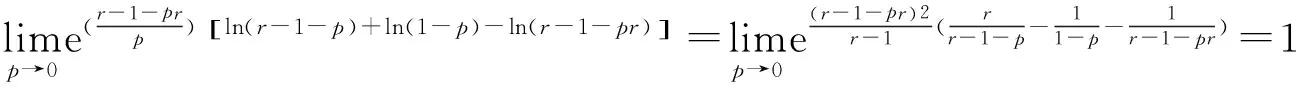
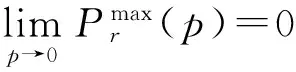
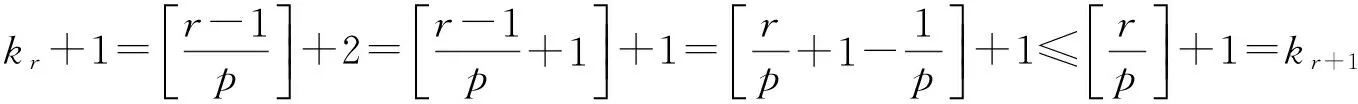
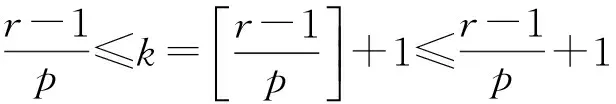
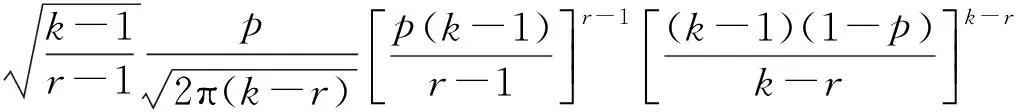
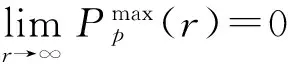
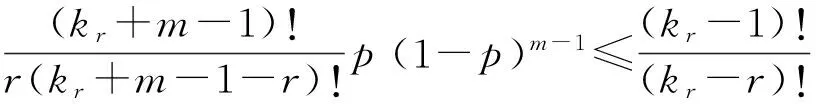
(2)

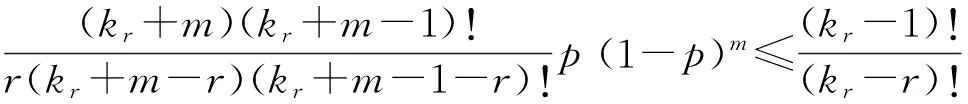
4　讨论

图3　负二项分布概率最大值Pmax分布图 (p=0.02,0.04,…,0.98;r=1,2,…,49)Fig.3　Graphs of the maximum value Pmax of the negative binomial distribution(p=0.02,0.04,…,0.98;r=1,2,…,49)

图4　相邻两个最大值之差(p)-的图形(r=50)Fig.4　The difference between the adjacent two maximum (p)-(p) of the negative binomial distributionmaximum(r=50)

[1]蒋仁言，左明健著．可靠性模型与应用[M]．北京：机械工业出版社，1999．

[2]田乃硕．休假随机服务系统[M]．北京：北京大学出版社，2001．

[3]陈峰，杨树勤．论负二项分布的应用条件[J]．中国卫生统计， 1995，4：21-22．

[4]周源泉，李宝盛. 预定成功数的负二项分布预测[J].质量与可靠性，2013 (1)：1-8．

[5]何春. 负二项分布概率的最大值点[J]. 生物数学学报,2011，26(1)：160-162

[6]GUPTA R C，ONG S H．A new generalization of the negative binomial distribution[J] ．Computational statistics and data analysis,2003， 45(2)：287-300．

[7]牛燕影，王增富，田乃硕．负二项分布类的条件概率封闭性[J]．数学理论与应用，2005，25(3)：101-103.

[8]熊加兵，陈光曙. 负二项分布随机变量的分解定理[J]. 大学数学， 2008，24(1)：108-110.

[9]王新利，陈光曙. 几何分布和负二项分布高阶矩的递推公式[J]. 高等数学研究，2011，14(2)：15-16.

[10]康殿统.负二项分布的两个不同定义[J].河西学院学报.2014，30(5)：22-30.

(责任编辑：方惠敏)

The Characters of the Probability Maximum Value for Negative Binomial Distribution

DING Yong

(DepartmentofMathematicsandComputerScience,KangDaCollegeofNanjingMedicalUniversity,Lianyungang222000,China)

The character of probability maximum value for negative binomial distribution was explored. The probability maximum value for negative binomial distribution was a function ofpandr, wherepwas the probability of success for each test, andrwas the number of the first successful test.It was a monotonically increasing continuous function ofpwhenrwas given，only (r-1)/pwas a integer, its derivative did not exist， and a monotone decreasing function ofrwhenpwas given.

negative binomial distribution; maximum probability; monotonicity

2016-03-06

丁勇(1956—)，男，江苏淮安人，教授，主要从事生物统计研究，E-mail:yding@njmu.edu.cn.

0211.1

A

1671-6841(2016)03-0047-04

10.13705/j.issn.1671-6841.2016050

引用本文：丁勇.负二项分布概率最大值的性质[J].郑州大学学报(理学版)，2016，48(3)：47-50.