有色环境噪音对空间异质种群动态同步性的影响
2016-05-07刘志广张丰盘
刘志广,张丰盘
河南大学,数学与统计学院,应用数学研究所,开封 475004
有色环境噪音对空间异质种群动态同步性的影响
刘志广*,张丰盘
河南大学,数学与统计学院,应用数学研究所,开封475004
摘要:随着种群动态和空间结构研究兴趣的增加,激发了大量的有关空间同步性的理论和实验的研究工作。空间种群的同步波动现象在自然界广泛存在,它的影响和原因引起了很多生态学家的兴趣。Moran定理是一个非常重要的解释。但以往的研究大多假设环境变化为空间相关的白噪音。越来越多的研究表明很多环境变化的时间序列具有正的时间自相关性,也就是说用红噪音来描述更加合理。因此,推广经典的Moran效应来处理空间相关红噪音的情形很有必要。利用线性的二阶自回归过程的种群模型,推导了两种群空间同步性与种群动态异质性和环境变化的时间相关性(即环境噪音的颜色)之间的关系。深入分析了种群异质性和噪音颜色对空间同步性的影响。结果表明种群动态异质性不利于空间同步性,但详细的关系比较复杂。而红色噪音的同步能力体现在两方面:一方面,本身的相关性对同步性有贡献;另一方面,环境变化时间相关性可以通过改变种群密度依赖来影响同步性,但对同步性的影响并无一致性的结论,依赖于种群的平均动态等因素。这些结果对理解同步性的机理、利用同步机理来制定物种保护策略和害虫防治都有重要的意义。
关键词:Moran效应;异质性动态;有色噪音;空间同步性;二阶自回归过程
刘志广,张丰盘.有色环境噪音对空间异质种群动态同步性的影响.生态学报,2016,36(2): 360-368.
Liu Z G,Zhang F P.Effects of colored environmental noise on the spatial synchrony of heterogeneous population dynamics.Acta Ecologica Sinica,2016,36(2): 360-368.
在不同空间栖息地种群波动成一致性,称之为空间同步性。研究报道在各种各样物种的种群动态中观察到了这种现象,如哺乳动物[1]、鸟类[2]、鱼类[3]、昆虫[4]、树类[5]、原生生物[6]和病毒[7]。揭示集合种群同步性的机理尤为重要,而且已经成为集合种群生态学的中心课题。因为这不仅可以帮助理解这些同步现象,而且空间同步性增加了集合种群的全局灭绝风险[8]。自然保护区的设置和濒危物种有效保护的很多方面都与种群动态的同步性水平挂钩,生物控制[9]和害虫防治[7]也都与种群动态的同步性有关。
在生态文献中,对空间同步性的解释主要有3种[10]: Moran效应、扩散和游荡的捕食者。在这里主要讨论分析第一种。早在1953年,Moran首先提出了如果两个种群有相同的线性依赖结构,则种群的空间相关性(即同步性)等于环境噪音的空间相关性,并且认为空间相关的环境噪音是导致种群同步性波动的原因[11]。然而,这一工作直到1992年才引起了生态学家的重视,并被称作“Moran定理”,而把不严格满足定理条件的其他情形下环境波动的同步作用称为“Moran效应”[12]。此后,有大量的理论和实验工作来验证“Moran效应”的效用,并且用它来解释在各种各样的生物系统中观察到的同步性现象。
“Moran定理”定理要求有4个条件:(1)线性密度依赖结构,(2)种群间有相同的密度依赖结构,(3)没有扩散和其他结构化耦合,(4)相关的白色环境噪音。最近的理论研究大多以“Moran定理”为基础,讨论更加符合现实情况的情形,如非线性的密度依赖[13-14]、加入扩散到线性动态[15]或二者兼而有之[16],还有异质性的种群结构[17]等等,主要探索这些因素如何影响空间同步性和环境噪音间的关系。越来越多的研究发现很多气候或环境变量被认为具有或多或少的正的时间自相关性[18]。这种性质可以用自回归噪音的一阶自回归系数来描述[19]。最近的研究表明环境变化的时间相关性(即环境噪音的颜色)对种群的同步性也有着重要的影响[20-21]。有色环境噪音的同步作用为同步性的研究提供了新的契机。前期的工作[21]涉及异质性的种群结构和有色的环境噪音对空间同步性的影响,但利用的是相对简单一阶自回归模型AR1。二阶自回归过程AR2经常用来模拟种群密度(经过对数变化)的时间动态。因为它可以产生更加实际的包括周期波动在内的种群动态波动曲线[12]。值得注意的是,Moran在阐述Moran定理的时候也是采用的这个模型。故将以往的研究推广到二阶自回归过程的情形更具实际意义。
1 模型
假设两个种群可以用二阶自回归过程AR2来描述:

式中,Xt,Yt表示种群X和Y在第t代数量的log值,φi(或ψi)表示种群X(或Y)的第i阶密度依赖强度(记成AR(i)系数),当其趋于零时,密度依赖调控强度增加[12]。ξt和ζt分别表示在t时刻空间相关的有色环境噪音,并设它们是非密度依赖的,均值为0和方差为常数。环境噪音时间序列可以用一阶自回归过程AR1来描述[19]:

式中,α(0≤α<1)是自回归参数,反应环境变化时间序列的时间自相关性,即环境噪音的颜色。当α= 0,时间序列是白色噪音;当0<α<1,时间序列是红色噪音,α值增加表示颜色变红。εt和ωt是均值为0方差为σ的标准白噪音过程。易知Corr(ξt,ζt)= Corr(εt,ωt)。
2 结果
对系统(1)两边运用算子(1-αB)(其中B是后退时间算子: But+1= ut)有:

系统(3)是一个三阶自回归过程,假设它是平稳的。利用Yule-Walker方程(附录A),经过计算可以得到X和Y之间的相关性公式(附录B):


式中,称DC为空间同步性的统计部分,则种群的空间同步性等于统计部分和环境噪音的空间相关性的乘积。容易知道,如果φ1=ψ1,φ1=ψ1,α= 0,则有Corr(X,Y)= Corr(ξ,ζ),也就是Moran定理。而当φ1=ψ1,φ1= ψ1,0<α<1,则Corr(X,Y)= Corr(ξ,ζ)。也就是说,具有相同结构的两种群,在有色噪音的情况下,Moran定理依然成立。但当两个种群的密度依赖结构不相同时,即具有异质性的种群动态时,空间同步性不再仅依赖于环境噪音的相关性,同时还依赖于两种群的动态参数和环境噪音的颜色值。下面分析DC如何依赖于种群动态参数φ1,φ2,ψ1,ψ2和噪音颜色参数α。因此,在参数空间构造3个新的组合参数:(1)两种群间的欧式距离D,D2=(φ1-ψ1)2+(φ2-ψ2)2;(2)重心B(两种群的平均动态),B =[(φ1+ψ1)/2,(φ2+ψ2)/2];(3)连接两种群间的直线与AR(1)轴的夹角A,cos(A)=(ψ1-φ1)/D。
固定α= 0.2,D = 0.275。如果种群只在AR(1)参数上不同(A = 0),DC呈现出离心的模式(图1a):在参数空间中,随着两种群的重心接近定义AR2过程平稳性的三角形的边缘,两个种群不稳定性的乘积增加[17],但空间同步性降低。如果种群只在AR(2)参数上不同(A =π),具有相似的模式。当种群在AR(1)和AR(2)参数都不同时,观察到一个不同的模式。DC的最高值不再位于(0,0)附近,而是向左转移(0<A<π/2,图1b)或者向右转移(π/2<A<π,图1c)。这些结果与Hugueny得到的结果类似[17],而他采用的是相关的白噪音。也就说,环境噪音时间相关性较小时,A和B对同步性影响的规律基本不变。但是当α值较大时,这些模式也要发生变化,观察不到清晰的模式(这里没有作图)。

图1 两个二阶自回归种群动态的空间同步性的统计部分(DC)值的等高线图形Fig.1 Level plot of the demographic component of spatial synchrony(DC)between populations governed by second order autoregressive dynamics一阶和二阶自回归系数所构成的参数平面上(AR(1)—AR(2))的点表示两种群的平均动态,其它参数值固定,D=0.275

图2 空间同步性的统计部分(DC)值的等高线图,以环境噪音颜α和两种群动态参数的欧式距离D为函数Fig.2 Contour of the demographic component of spatial synchrony,as a function of the environmental noise color and the Euclidean distance between populations in parameter plane
下面让重心B和夹角A固定,从而研究DC与环境噪音颜色α和欧式距离D的关系。图2和3表明随着D的增加,同步性减小。图2a表明如果两个种群仅在一阶自回归系数上有差别(A = 0),随着环境噪音颜色系数的增加,同步性下降。但是如果两个种群仅在二阶自回归系数上有差别(A =π/2),随着噪音颜色的增加,同步性增加(图2b,e)。当种群在AR(1)和AR(2)参数都不同时,当0<A<π/2,观察到的模式与A = 0时类似(图2c,f)。当π/2<A<π,观察到的模式与A =π/2时类似。而当重心的位置发生改变时,环境噪音的颜色对同步性的影响规律也相应的发生了变化(图3)。总之,环境噪音颜色对同步性的影响没有一致性的结论,它依赖于两种群参数间的夹角A和重心B的值。
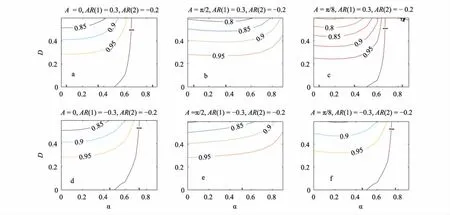
图3 在参数平面上具有不同的平均种群动态Fig.3 Different average location of populations in parameter plane
3结论与讨论
通常认为迁移在局域尺度上起作用,而Moran效应在更大的空间尺度上起作用[22]。根据Moran定理,空间同步性是环境相关性的一个反映。因此,支持Moran效应的一种令人信服的方法是观察到的同步性随地理距离的消散方式应与环境因子相关性随地理距离的变化方式相似。例如,Koenig报道了北美松雀种群的同步性和距离间的关系与降水与距离间的关系匹配良好[23-24]。但大多种群同步性随距离的下降速度比环境相关性随距离的下降速度要快的多,例如Peltonen等对森林昆虫的研究[25]。这与Moran定理不一致。这种不一致的原因可能是对实际驱动环境因子的错误识别,或不完全符合Moran定理的条件。当相同的密度依赖结构不能满足时,如在估计一个大的地理区域或者包含不同类型栖息地区域的种群动态同步性时,考虑异质性就非常重要。种群动态的地理变化是普遍存在,如加拿大的舞毒蛾[25]和猞猁[26]、英国的松尺蠖蛾[27]、欧洲和日本的田鼠[28-29]。其对同步性的影响,已经进行了一些实验、模拟和理论分析的工作。受Hugueny工作[17]的启发,本文分析了具有异质性种群动态和受有色环境噪音影响的AR2种群的同步性,得到了一个分析解。这种方法更加的灵活、有力。结果表明同步性可以分成统计部分和环境空间相关性的乘积。当两种群具有相同的密度依赖时,统计部分等于环境的空间相关性,即Moran定理在红噪音的时候依然成立。而种群具有不同的密度依赖结构时(即AR系数不同),统计部分小于1,甚至可以是0。也就是说即使环境的空间相关性很高,也有可能具有非常小的种群同步性。当然,空间同步性的统计部分与密度依赖参数间并无清晰且简单的关系。甚至在较弱的密度依赖的种群间也能观察到强的空间同步性。
空间同步性的统计部分同时也受环境噪音颜色的影响。已有一些研究注意到了环境噪音颜色对同步性的影响[20-21,30]。结果表明有色噪音对同步性的影响依赖于它进入种群模型的方式、扩散方式和非线性密度依赖的程度。Vasseur[20]和Liu等[21]分别采用不同的空间相关有色噪音的构造方法(1/f和AR1),利用线性一阶自回归过程的种群模型,得到了红噪音加强异质种群同步性的结果。与之比较,本研究发现,即使对线性模型,红噪音既有加强又有减弱异质种群同步性的作用。环境噪音的颜色对异质种群同步性具有非常重要的影响,这一点毋庸置疑。但是噪音颜色对异质种群同步性有什么作用并没有一致的结论。
本文的结果对可以用线性自回归过程描述的种群动态成立。如果种群动态具有非线性的密度依赖结构,尤其出现混沌动态时,将出现更加复杂的空间模式。然而,即使自然种群动态为非线性的,简单的线性模型可以近似很多种群的动态,尤其当种群大小的变化系数很小的时候[22,31]。Engen和Sæther[32]推导了种群动态参数存在差异的一类非线性一阶模型的空间同步性的表达式。但对线性模型,参数值的空间异质性导致同步性的降低。假设空间种群没有迁移且可用线性自相关平稳过程来描述,当已知环境自相关系数和种群密度依赖参数时,空间同步性的统计部分可计算出来的。当然这是一种理想情况,自然种群很少能符合这些假设。但是强调种群异质性减弱空间同步性和环境变化的时间自相关对同步性影响的时候,这个方法还是非常有用的。
Ylikarjula利用单物种模型研究了不同迁移方式和斑块数目对同步性的影响[33]。利用两斑块得到的结果推广到多斑块的情形时可能不一样。因为不同斑块间可能有些相互的抵消。迁移是另一个非常重要的同步因子。有些情况下迁移因素是容易排除的,如苏格兰群岛的索艾羊种群[13],岛屿之间被海洋隔断,迁移不可能发生。但大多数情况下,迁移是不可以忽略的因素。因此迁移和有色噪音都存在的空间种群同步性问题的研究也同样重要。此时二者对所产生同步性的贡献及二者间的相互作用也是众多学者关心的问题[15]。
参考文献(References):
[1]Elton C,Nicholson M.The ten year cycle in numbers of the lynx in Canada.Journal of Animal Ecology,1942,11(2): 215-244.
[2]Moran P A P.The statistical analysis of game-bird record.Journal of Animal Ecology,1952,21(1): 154-158.
[3]Ranta E,Kaitala V,Lindström J,Lindén H.Synchrony in population dynamics.Proceedings of the Royal Society of London Series B: Biological Sciences,1995,262(1364): 113-118.
[4]Den Boer P J.On the survival of populations in a heterogeneous and variable environment.Oecologia,1981,50(1): 39-53.
[5]Koenig W D,Knops J M H.Scale of mast-seeding and tree-ring growth.Nature,1998,396(6708): 225-226.
[6]Holyoak M,Lawler S P.Persistence of an extinction-prone predator-prey interaction through metapopulation dynamics.Ecology,1996,77(6): 1867-1879.
[7]Bolker B M,Grenfell B T.Impact of vaccination on the spatial correlation and persistence of measles dynamics.Proceedings of the National Academy of Sciences of the United States of America,1996,93(22): 12648-12653.
[8]Heino M,Kaitala V,Ranta E,Lindström J.Synchronous dynamics and rates of extinction in spatially structured populations.Proceedings of the Royal Society B: Biological Sciences,1997,264(1381): 481-486.
[9]Cavalieri L F,Kocak H.Chaos: a potential problem in the biological control of insect pests.Mathematical Biosciences,1995,127(1): 1-17.
[10]Liebhold A,Koenig W D,Bjørnstad O N.Spatial synchrony in population dynamics.Annual Review of Ecology,Evolution and Systematics,2004,35: 467-490.
[11]Moran P A P.The statistical analysis of the Canadian lynx cycle.II.Synchronization and meteorology.Australian Journal of Zoology,1953,1(2): 291-298.
[12]Royama T.Analytical Population Dynamics.Netherlands: Springer,1992.
[13]Grenfell B T,Wilson K,Finkenstädt B F,Coulson T N,Murray S,Albon S D,Pemberton J M,Clutton-Brock T H,Crawley M J.Noise and determinism in synchronized sheep dynamics.Nature,1998,394(6694): 674-677.
[14]Royama T.Moran effect on nonlinear population processes.Ecological Monographs,2005,75(2): 277-293.
[15]Kendall B E,Bjørnstad O N,Bascompte J,Bascompte J,Keitt T H,Fagan W F.Dispersal,environmental correlation,and spatial synchrony in population dynamics.American Naturalist,2000,155(5): 628-636.
[16]Hanski I,Woiwod I P.Spatial synchrony in the dynamics of moth and aphid populations.Journal of Animal Ecology,1993,62(4): 656-668.
[17]Hugueny B.Spatial synchrony in population fluctuations: extending the Moran theorem to cope with spatially heterogeneous dynamics.Oikos,2006,115(1): 3-14.
[18]Vasseur D A,Yodzis P.The color of environmental noise.Ecology,2004,85(4): 1146-1152.
[19]Ripa J,Lundberg P.Noise colour and the risk of population extinction.Proceedings of the Royal Society B: Biological Sciences,1996,263: 1751-1753.
[20]Vasseur D A.Environmental colour intensifies the Moran effect when population dynamics are spatially heterogeneous.Oikos,2007,116(10): 1726-1736.
[21]Liu Z G,Gao M,Li Z Z,Zhu G F.Synchrony of spatial populations: heterogeneous population dynamics and reddened environmental noise.Population Ecology,2009,51(1): 221-226.
[22]Lande R,Engen S,Sæther B E.Spatial scale of population synchrony: environmental correlation versus dispersal and density regulation.American Naturalist,1999,154(3): 271-281.
[23]Koenig W D.Spatial autocorrelation and local disappearances in wintering north American birds.Ecology,2001,82(9): 2636-2644.
[24]Koenig W D.Global patterns of environmental synchrony and the Moran effect.Ecography,2002,25(3): 283-288.
[25]Peltonen M,Liebhold A M,Bjørnstad O N,Williams D W.Spatial synchrony in forest insect outbreaks: roles of regional stochasticity and dispersal.Ecology,2002,83(11): 3120-3129.
[26]Stenseth N C,Chan K S,Tong H,Boonstra R,Boutin S,Krebs C J,Post E,Donoghue M O,Yoccoz N G,Forchhammer M C,Hurrell J W.Common dynamics structure of Canada lynx populations within three climatic regions.Science,1999,285(5430): 1071-1073.
[27]Broekhuizen N,Evans H P,Hassell M P.Site characteristics and the population dynamics of the pine looper moth.Journal of Animal Ecology,1993,62(3): 511-518.
[28]Turchin P.Complex Population Dynamics: a Theoretical Empirical Synthesis.Princeton NJ: Princeton University Press,2003.
[29]Saitoh T,Stenseth N C,Bjørnstad O N.The population dynamics of the vole Clethrionomys rufocanus: modeling geographic gradients in populations dynamics.Researches on Population Ecology,1998,40: 85-95.
[30]Heino M.Noise colour,synchrony and extinctions in spatially structured populations.Oikos,1998,83(2): 368-375.
[31]Ives A R,Dennis B,Cottingham K L,Carpenter S R.Estimating community stability and ecological interactions from time-series data.Ecological Monograph,2003,73(2): 301-330.
[32]Engen S,Sæther B E.Generalizations of the Moran effect explaining spatial synchrony in population fluctuations.American Naturalist,2005,166(5): 603-612.
[33]Ylikarjula J,Alaja S,Laakso J,Tesar D.Effects of patch number dispersal patterns on population dynamics and synchrony.Journal of Theoretical Biology,2000,207(3): 377-387.
附录A
两个AR(3)过程的空间同步性
考虑两个用AR(3)过程来描述的种群:
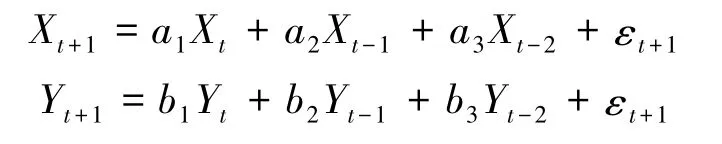
如果系统是3阶平稳多变量时间序列,它应该满足Yule-Walker方程:

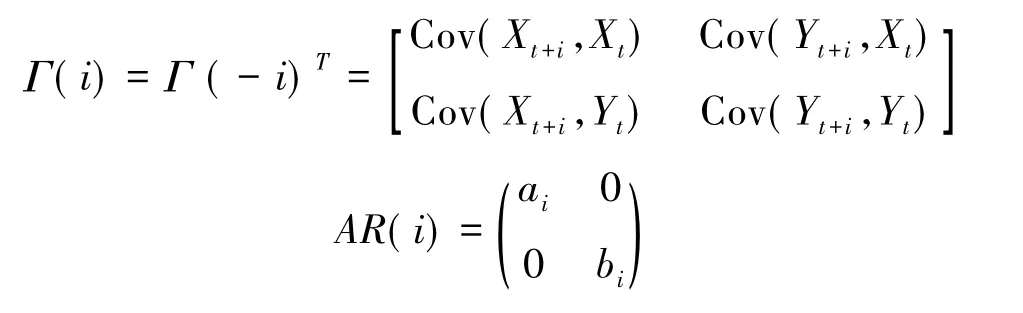
由A2有;

由A1有:

由A6,A7有:
其中,

由A8有:
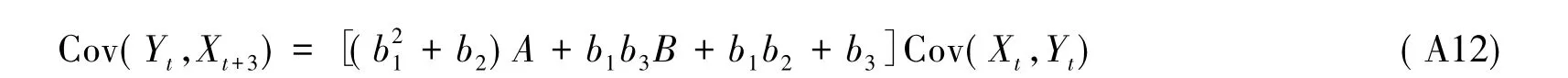
因而容易计算得:

另外,容易计算AR(3)过程的方差,具体细节略去。


最后,

其中,

附录B
有色噪音影响的两个AR(2)过程的空间同步性
对于有色噪音ξt+1=αξt+1+βεt(其中εt独立同分布)。运用算子(1-αB)(其中B是向后时间算子,即But+1= ut)到方程1,且利用关系式(1-αB)ξt+1=εt,则有:

而B1,B2是AR(3)过程,根据附录A和Corr(ξt,ζt)= Corr(εt,ωt),有:
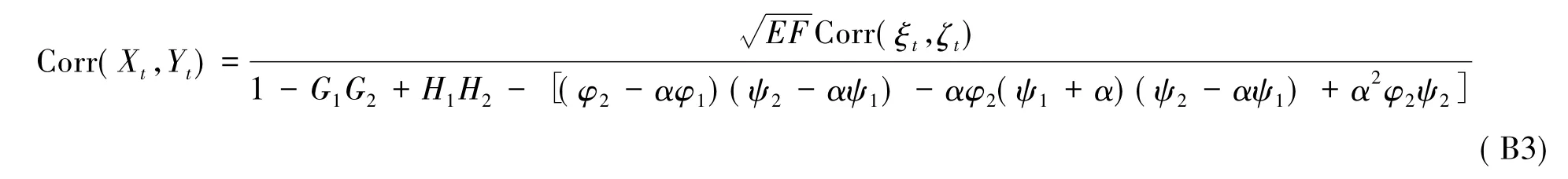
其中,
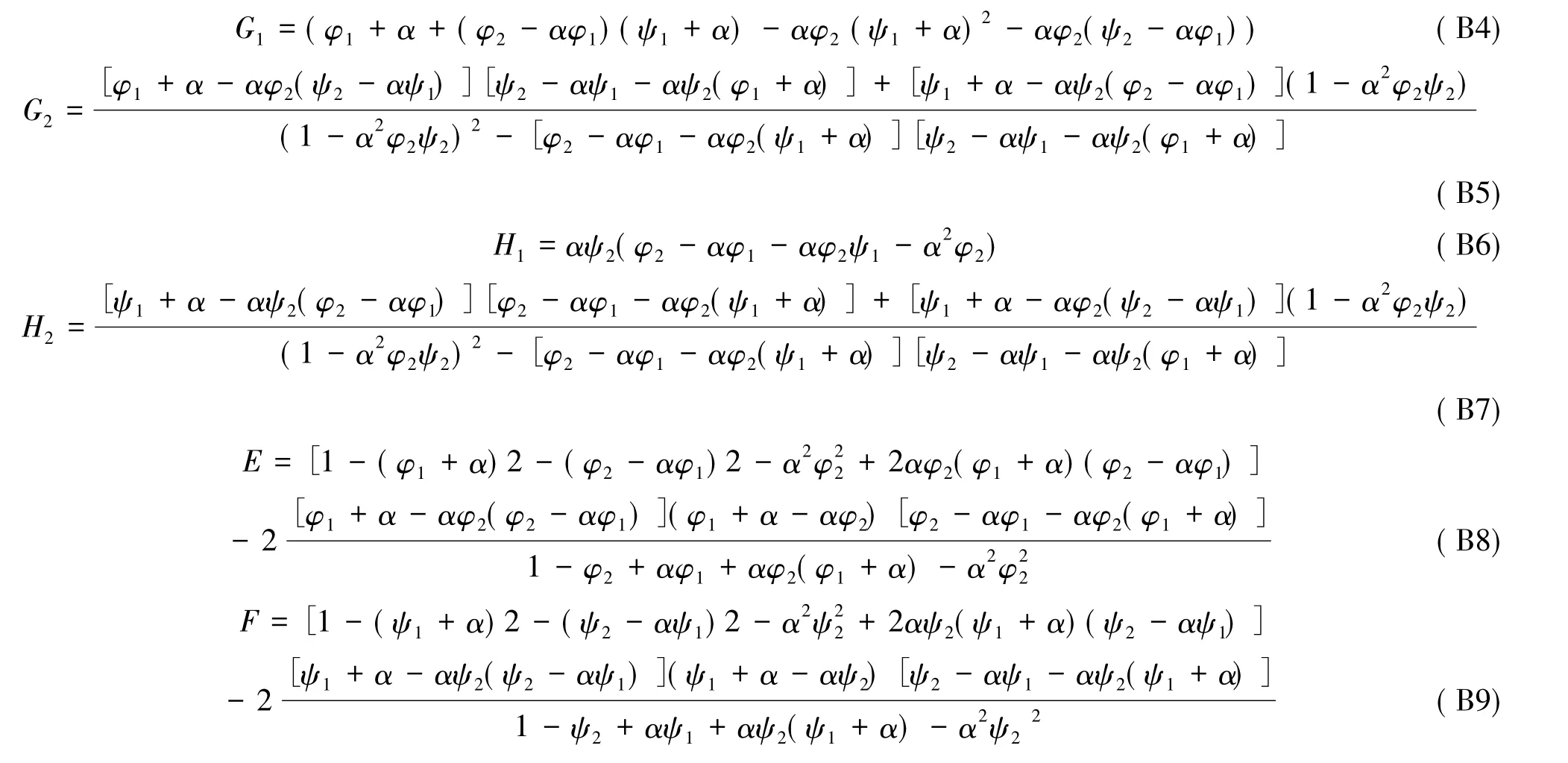
Effects of colored environmental noise on the spatial synchrony of heterogeneous population dynamics
LIU Zhiguang*,ZHANG Fengpan
Institute of Applied Mathematics,School of Mathematics and Information Sciences,Henan University,Kaifeng 475004,China
Abstract:Spatial synchrony of oscillating populations has been observed in various ecological systems,and identifying its causes has attracted the interest of ecologists.The synchrony of a spatial population has been shown to be detrimental to its persistence because all local populations may go extinct simultaneously.Previous studies have shown that three main hypotheses can explain this phenomenon.First,it may be due to synchronous environmental forcing—the so-called Moran effect or Moran theorem.Second,migration or dispersal of individuals is liable to cause population synchrony,and third,nomadic predators have been proposed as a synchronizing mechanism.In this paper,we focus on the first explanation.
Moran's theorem suggests that if two(or more)populations sharing a common linear density-dependence in the renewal process are disturbed with correlated noise,they will become synchronized with a correlation that matches the noise correlation.Four conditions are needed for the Moran theorem to be applicable: linear density-dependence structure,identical density dependence structure,no dynamical coupling,and spatially correlated white environmental noise.However,there is mounting evidence that population dynamics may differ geographically within a given species.Moreover,various climatic variables in nature are known to demonstrate positive temporal autocorrelation.These violate the assumptions that the dynamics of the populations are identical and environmental noise is white.Therefore,the classical Moran theoremneeds to be extended to cope with these situations.
In this paper,we make the assumption that population dynamics can be described by linear and stationary autoregressive processes,and that they are affected by spatially correlated colored environmental noise.The noise color refers to the temporal correlation in the time series data of the environmental noise and is expressed as the degree of(firstorder)autocorrelation for autoregressive noise.The level of synchrony can be measured as the correlation between two populations.We show that(1)the observed spatial synchrony between two populations can be split into two multiplicative components: the demographic component that depends on the values of the autoregressive coefficients and the environmental noise color,and the correlation of the environmental noise.The Moran theorem still holds in spatial synchrony accounted for by the correlated red noise between homogeneous populations described by linear processes.(2)Spatial variability in population dynamics may substantially contribute to the spatial variability of population synchrony.However,it is complex.No obvious connection is found between the values of the autoregressive coefficients and the demographic component of spatial synchrony.(3)The synchronizing potential of correlated red noise has two characteristics: the correlation between red noises can contribute to the spatial synchrony,and the coefficient of noise color can contribute to the spatial synchrony by affecting the density dependent structure of population dynamics.However,we cannot obtain a discernible pattern between the demographic component of spatial synchrony and the environmental noise color.Environmental noise color intensifies or diminishes the Moran effect when population dynamics are spatially heterogeneous,and this effect depends strongly on the values of the three new combined parameters that we consider in this paper.These results should improve our understanding of the mechanism underlying population synchrony.They should also help develop conservation management plans and improve the control of pest species.
Key Words:moran effect; heterogeneous dynamics; colored noise; spatial synchrony; second-order autoregressive process
*通讯作者
Corresponding author.E-mail: liuzhiguang@ henu.edu.cn
收稿日期:2013-06-07;网络出版日期: 2015-06-10
基金项目:国家自然科学基金项目(31200312);河南省教育厅基金项目(2011A180003)
DOI:10.5846/stxb201306071388
