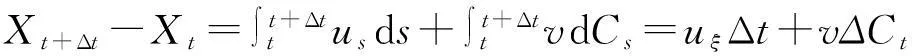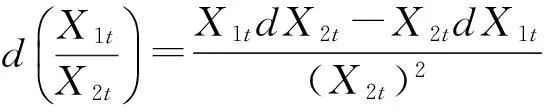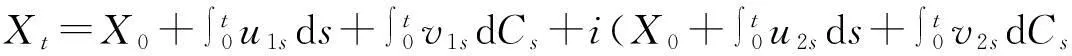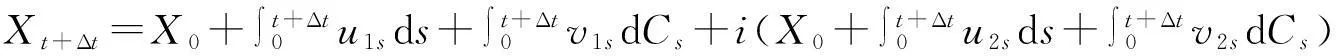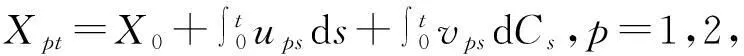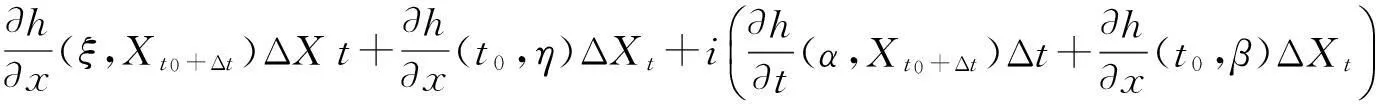模糊微分的性质

尤翠莲，王伟卿

(河北大学 数学与信息科学学院，河北保定　071002)

模糊微分的性质

尤翠莲，王伟卿

(河北大学 数学与信息科学学院，河北保定071002)

摘要：在求解由刘过程驱动的模糊微分方程时，需要研究一类新的模糊微分(刘微分)的性质. 给出了模糊微分的2个中值定理和4个模糊微分公式， 并且将这些结论推广到复模糊微分的情形. 这些工作为模糊微分方程理论的完善和应用奠定了基础.

关键词：模糊过程；模糊微分； 中值定理； 复模糊过程

DOI：10.3969/j.issn.1000-1565.2015.02.001

中图分类号：O159

文献标志码：志码：A

文章编号：编号：1000-1565(2015)02-0113-05

Abstract:To solve fuzzy differential equation driven by Liu process， some properties of a new kind of fuzzy differential (Liu differential) are needed. Two mean value theorems of fuzzy differential and four fuzzy differential formulas are given in this paper. Furthermore， these conclusions are extended to the case of complex fuzzy process. The studies above lay a theoretical foundation for calculation and application of the fuzzy differential equation.

收稿日期:2014-10-08

基金项目：国家自然科学基金资助项目(11201110；61374184；11101115)； 河北省高等学校优秀青年基金资助项目(Y2012021)

Properties of fuzzy differential

YOU Cuilian， WANG Weiqing

(College of Mathematics and Information Science， Hebei University， Baoding 071002， China)

Key words: fuzzy process； fuzzy differential； mean value theorem； complex fuzzy process

MSC 2010: 34A07

第一作者:尤翠莲(1977-)，女，河北唐山人，河北大学副教授，博士，主要从事不确定理论与模糊微分方程的研究.

E-mail：yycclian@163.com

在实际生活中， 随机事件通常用概率来描述.1965年Zadeh[1]给出了模糊事件的定义. 为了度量模糊事件， Zadeh[2]在1978年引入了可能性测度. 后来Nahmias[3]， Yanger[4]， De Cooman[5]， Dubois等[6]做了进一步的研究. 然而可能性测度没有自对偶性， 因此在2002年Liu 等[7]引入了可信性测度的概念， 并且Li等[8]给出了可信性测度的一个充分必要条件， Liu[9]提出了可信性理论.

为了处理动态随机现象， 随机过程已经被广泛应用. 要处理动态模糊现象， 同样也需要相应的计算，因此在2008年Liu[10]提出了模糊过程、微分公式和模糊积分， 之后被更名为刘过程、 刘公式和刘积分. 后来，You等[11]将刘过程、 刘公式和刘积分推广到多维的情形. Qin[12]对复刘过程、复刘公式和复刘积分进行了研究. 尤翠莲等[13]研究了刘积分的性质以及积分存在的条件.刘过程的更多内容可参见文献[14-19].

不管求解模糊微分方程， 还是用模糊微分方程解决实际问题， 前提都是要研究模糊微分的性质. 本文旨在对模糊微分及复模糊微分的性质进行研究.

1预备知识

设Θ为一个非空集合，P为Θ的非空幂集，Cr为可信性测度，A为P中的事件，Cr{A}用来衡量事件A发生的可能性大小，为了保证Cr{A}的数学性质，可信性测度Cr需满足如下条件：

1)(正则性)Cr{Θ}=1;

2)(单调性)当A⊂B时，Cr{A}≤Cr{B}；

3)(自对偶性)当A⊂P时，Cr{A}+Cr{Ac}=1；

4)(极大性)对于任意的Ai⊂P， 如果supiCr{Ai}<0.5， 那么Cr{∪iAi}=supiCr{Ai}.

Liu[9]称三元组(Θ，P，Cr)为可信性空间. 从可信性空间到实数集的函数就是模糊变量.

定义1[10]刘过程Ct是指满足下面3个条件的模糊过程：

1)C0=0；

2)Ct具有独立增、稳态增性质；

3) 对于固定的时刻t，每一个增量Cs+t-Cs是一个具有期望值et和方差σ2t2的正态模糊变量，其隶属函数为

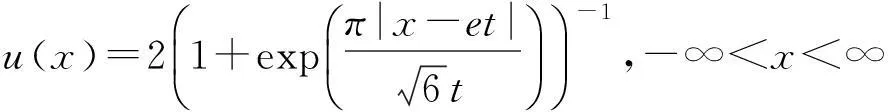
特别地，当e=0，σ=1时，称这样的刘过程为标准刘过程.

定理1(刘公式)[10]假设Xt是一个由dXt=utdt+vtdCt给出的模糊过程，Ct是一个标准刘过程，其中ut，vt分别是绝对可积和刘可积的模糊过程，h(t,x)是一个连续可微的函数， 那么Yt=h(t,Xt)也是一个模糊过程， 并且

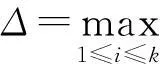
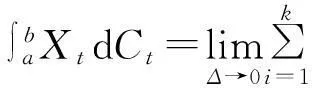
定义3[12]假设T是一个指标集，则一个复模糊过程是一个从T×(Θ,P,Cr)到复数集的函数.

定理2[12]一个模糊过程是复模糊过程的充分必要条件是存在2个实模糊过程X1t，X2t使得Xt=X1t+iX2t， 其中i是虚数单位.

定理3[11]设(C1t,C2t,…,Cmt)T是一个m维标准刘过程，h(t,x1,x2,…,xn)是一个多变量连续可微的函数，n维模糊过程(X1t,X2t,…,xnt)由

给出， 其中upt，vpqt分别是绝对可积和刘可积的模糊过程， 则模糊过程Yt=h(t,X1t,X2t,…,Xnt)的微分为

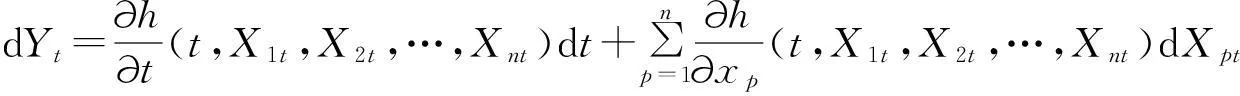
2模糊微分的性质

Xt+Δt-Xt=uξΔt+vΔCt.

由黎曼积分的中值定理可知，存在时刻ξ(t<ξ

定理5(第2中值定理)设Ct是一个标准的刘过程，h(t,x)是连续可微的函数，dXt=utdt+vtdCt，其中us和vs是绝对可积和刘可积的模糊过程，则Yt=h(t,Xt)也是一个模糊过程， 且

证明：由刘公式可知Yt=h(t,Xt)是一个模糊过程.所以根据微分中值定理可知，存在ξ，η，其中ξ=t0+θ1(t-t0)，η=Xt0+θ2(Xt-Xt0)，0<θ1，θ2<1使得

h(t0+Δt,Xt0+Δt)-h(t0,Xt0)=[h(t0+Δt,Xt0+Δt)-h(t0,Xt0+Δt)]+[h(t0,Xt0+Δt)-h(t0,Xt0)]=

定理6若X1t和X2t是模糊过程，则

1)d(X1t±X2t)=dX1t±dX2t.

证明：取定理3中n=2，h(t,x1,x2)=x1±x2,可得出结论.

2)d(X1tX2t)=X2tdX1t+X1tdX2t.

证明：取定理3中n=2，h(t,x1,x2)=x1x2,可得出结论.

3) 对任意常数c，d(cX1t)=cdX1t.

证明：由刘公式可得出结论.

3复模糊微分的性质

Xt+Δt-Xt=uξΔt+vηΔCt+i(uαΔt+vβΔCt).

其中t<ξ,α,β,η

证明：由于h(t,x)是连续可微的复函数，因此Yt=h(t,Xt)是一个复模糊过程. 所以

h(t0+Δt,Xt0+Δt)-h(t0,Xt0)=[h(t0+Δt,Xt0+Δt)-h(t0,Xt0+Δt)]+[h(t0,Xt0+Δt)-h(t0,Xt0)]=

其中ξ=t0+θ1(t-t0)，η=Xt0+θ2(Xt-Xt0)，α=t0+θ3(t-t0)，β=Xt0+θ4(Xt-Xt0) ，0<θ1,θ2,θ3,θ4<1.

定理9若X1t，X2t，Y1t，Y2t是模糊过程，Xt=X1t+iX2t，Yt=Y1t+iY2t， 则

证明：由定理2和定理6可得出结论.

4结论

本文进一步研究了模糊微分(刘微分)的性质， 给出了模糊微分的2个中值定理，4个模糊微分公式， 以及2个复模糊中值定理和4个复模糊微分公式. 这些性质可简化求解模糊微分方程的过程.

参考文献:

[1]ZADEH L A. Fuzzy sets as a basis for a theory of possibility[J]. Fuzzy Sets and Systems， 1987， 1(1): 3-28.

[2]ZADEH L A. Fuzzy sets[J]. Information and Control， 1965， 8(3):338-353.

[3]NAHMIAS S. Fuzzy variables[J]. Fuzzy Sets and Systems， 1987， 1(2):97-110.

[4]YAGER R. A foundation for a theory of possibility[J]. Journal of Cybernetics， 1980， 10(1-3):177-204.

[5]COOMAN De G. Possibility theory I-III[J]. International Journal of General Systems， 1997， 25(4):291-371.

[6]DUBOIS D， PRADE H. Possibility theory: an approach to computerized processing of uncertainty[M]. New York：Plenum，1988.

[7]LIU Baoding， LIU Yankui. Expected value of fuzzy variable and fuzzy expected value models[J]. IEEE Transaction on Fuzzy Systems， 2002， 10(4):445-450.

[8]LI Xiang， LIU Baoding. Chance measure for hybrid events with fuzziness and randomness[J]. Soft Computing， 2009， 13(2):105-115.

[9]LIU Baoding. Uncertainty theory: an introduction to its axiomatic foundations[M]. Berlin：Springer-verlag， 2004.

[10]LIU Baoding. Fuzzy process， hybrid process and uncertain process[J]. Journal of Uncertain Systems， 2008，2(1):3-16.

[11]YOU Cuilian，HUO Huae， WANG Weiqing. Multi-dimensional Liu process，differential and integral[J]. Journal of East Asian Mathematical， 2013，29(1):13-22.

[12]QIN Zhongfeng. On analytic function of complex Liu process.[EB/OL].(2008-04-12) [2013-10-02]. http://orsc.edu.cn/process/071026.pdf，2007.

[13]尤翠莲， 王根森. 一类新的模糊积分的性质[J]. 河北大学学报：自然科学出版社， 2011， 31(4):337-340.

YOU Cuilian， WANG Gensen.Properties of a new kind of fuzzy integral[J].Journal of Hebei University：Natural Science Edition， 2011， 31(4):337-340.

[14]ZHU Yuanguo. Fuzzy control theory[EB/OL]. ( 2008-07-05) [ 2013-10-02]. http://orsc.edu.cn/~yhzhu/fct.pdf.

[15]ZHU Yuanguo. Fuzzy optimal control with application to portfolio selection[EB/OL]. ( 2008-01-17) [2013-10-02]. http://orsc.edu.cn/process/080117.pdf.

[16]LIU Linzhong， LI Yinzhen. The fuzzy quadratic assignment problem with penality: new models and genetic algorithm[J]. Applied Mathematics and Computation， 2006， 174(2):1229-1224.

[17]LIU Yankui， LIU Baoding. Random fuzzy programming with chance measures defined by fuzzy integrals[J]. Mathematical and Computer Modelling， 2002，36(4-5):509-524.

[18]LIU Baoding， LIU Yankui. Expected value of fuzzy variable and fuzzy expected value models[J]. IEEE Transactions on Fuzzy Systems， 2002，10(4):445-450.

[19]QIN Zhongfeng， LI Xiang. Option pricing formula for fuzzy financial market[J]. Journal of Uncertain Systems， 2008， 2(1): 17-21.

(责任编辑：王兰英)