A Note on Gap Phenomena of K¨ahler Manifolds with Nonnegative Curvature

JIAO Zhen-hua

(School of Science,Hangzhou Dianzi University,Hangzhou 310018,China)

A Note on Gap Phenomena of K¨ahler Manifolds with Nonnegative Curvature

JIAO Zhen-hua

(School of Science,Hangzhou Dianzi University,Hangzhou 310018,China)

In this paper,we study the complex structure and curvature decay of K¨ahler manifolds with nonnegative curvature.Using a recent result obtained by Ni-Shi-Tam,we get a gap theorem of Ricci curvature on K¨ahler manifold.

gap phenomena;K¨ahler manifolds;nonnegative curvature

§1.Introduction

The relation between the curvature and the geometric structure of manifolds has been studied extensively.In this article we are interested in the complex structure and curvature decay of K¨ahler manifolds with nonnegative curvature.The structure theorem of Cheeger-Gromoll-Meyer[12]states that a complete non-compact n-dimensional Riemannian manifold with positive sectional curvature is di ff eomorphic toℝn.Based on this result,Greene-Wu[3]formulated the following conjecture concerning the complex structure of K¨ahler manifolds.

ConjectureA complex n-dimensional complete non-compact K¨ahler manifold with positive sectional curvature is biholomorphic toℂn.

In[4],Mok gave a partial affirmative answer to the conjecture for complex 2-dimensional manifolds with additional assumption of maximal volume growth and 0＜R(x)where R(x)and d(x0,x)denote respectively the scalar curvature and geodesic distance from x to x0,C0is some positive constant.Up to now,the best result about the above conjecture is due to Chau and Tam[5].Speci fi cally,they proved that a complete noncompact K¨ahler manifoldwith nonnegative and bounded holomorphic bisectional curvature and maximal volume growth is biholomorphic to complex Euclidean spaceℂn.

For the complex structure of K¨ahler manifolds,Greene and Wu[6,Theorem10]238proved that a complete non-compact K¨ahler manifold with positive sectional curvature is a Stein manifold.In [4],it was proved that M is Stein under the assumptions that M has positive Ricci curvature and nonnegative holomorphic bisectional curvature,provided M has maximal volume growth and the scalar curvature decays likes r−2.With even weaker curvature assumptions,Chen and Zhu[7]get the Steinness of K¨ahler manifolds.Particularly,they proved that a complete noncompact K¨ahler manifold with nonnegative holomorphic bisectonal curvature and with maximal volume growth is Stein if the scalar curvature decays like r−1−εfor some ε＞0.

In the investigation of complex structure of K¨ahler manifolds,the curvature decay conditions play an important role.This motivates us to take interest in the topic of curvature decay of manifolds.The classical Bonnet-Myers theorem states that the Ricci curvature of a complete non-compact manifold can not be uniformly bounded from blow by a positive constant.In [8],Chen-Zhu showed the following theorem concerning decay estimate of scalar curvature of K¨ahler manifold.

Theorem 1.1Let M be a complete non-compact K¨ahler manifold with positive holomorphic bisectional curvature.Then for any x0∈M,there exists a positive constant˜C such that

where Vol(B(x0,r))denotes the volume of the geodesic ball centered at x0∈M with radius r, R(x)is the scalar curvature of M.

This theorem tells us that the curvature can not be too much at inf i nity.In the other direction,is there limit on how less the curvature can have?The answer is affirmative for manifolds of complex dimension n≥2.For example,In[9],Mok,Siu and Yau obtained the following theorem.

Theorem 1.2Let Mnbe a complete non-compact K¨ahler manifold of complex dimension n≥2.Suppose M is a Stein manifold and the holomorphic bisectional curvature is nonnegative. Moreover,assume

Where B(x0,r)and d(x0,x)denote respectively geodesic balls and geodesic distances,c＞0,C′≥0 and ε is an arbitrarily small positive constant.Then M is isometrically biholomorphic toℂnwith the f l at metric.

Theorem 1.2 can be interpreted as a gap phenomenon of bisectional curvature on K¨ahler manifolds.Later Greene and Wu[10]generalized this gap phenomenon to Riemannian manifolds. Recently,Chen and Zhu[11]discovered a gap theorem on locally conformally f l at Riemannian manifolds by developing the technique of Ricci f l ow and Yamabe f l ow.More recently,Ni[12]showed that a noncompact complete K¨ahler manifold of nonnegative holomorphic bisectional curvature must be f l at,if the average scalar curvature decays faster than quadratically.The main result of this note is the following gap theorem of Ricci curvature on K¨ahler manifold.

Theorem 1.3Let M be a complex n-dimensional complete non-compact K¨ahler manifold with nonnegative holomorphic bisectional curvature.Suppose the Ricci curvature is nonnegative and for some positive constant C∗it holds that

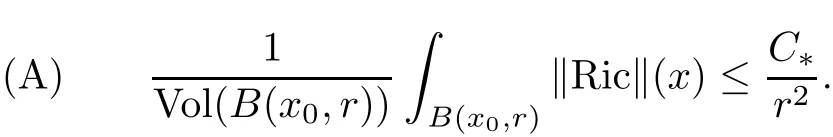
If the scalar curvature decay satisf i es

for some constant C∗＞0 and the volume growth of M is slow,i.e.,

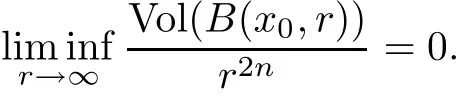
Then the Ricci curvature of M will assume zero everywhere.

§2.Proof of Theorem 1.3

In this section we will complete the proof of Theorem 1.3.As preparation,we repeat the following lemma which has been proved by Ni-Shi-Tam[13].

Lemma 2.1Let M be a complete non-compact K¨ahler manifold with nonnegative holomorphic bisectional curvature.Let ρ be a real closed(1,1)-form with trace f.Suppose ρ≥0 and ρ＞0 at some point x0.We also suppose ρ satisf i es

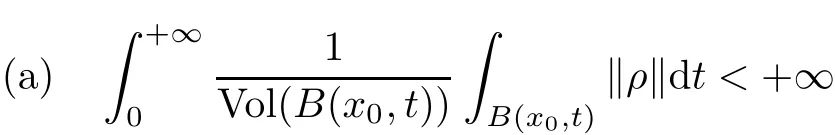
and

Then there exist positive constants C1,C2and C3independent of r such that

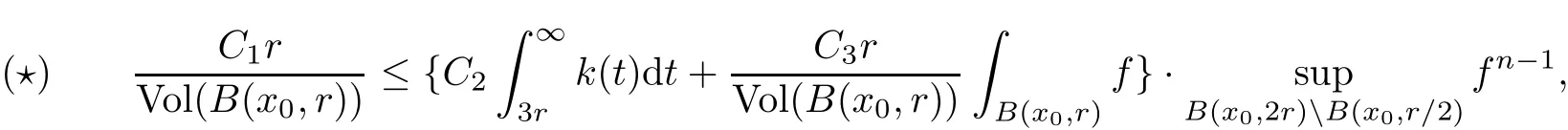
where k(t)=1/Vol(B(x0,t))R B(x0,t)f.

With this lemma,we give the proof of Theorem 1.3 now.

Proof of Theorem 1.3We will argue by contradiction.Suppose the conclusion of Theorem 1.3 is not true.We assume the Ricci form Ric＞0 at some point x0.Obviously, Ric satisf i es conditions(a)and(b).Hence the inequality(⋆)in Lemma 2.1 holds,where thefunction f replaced by the scalar curvature R(x),which is the trace of the Ricci form.By assumptions(A)and(B),we have

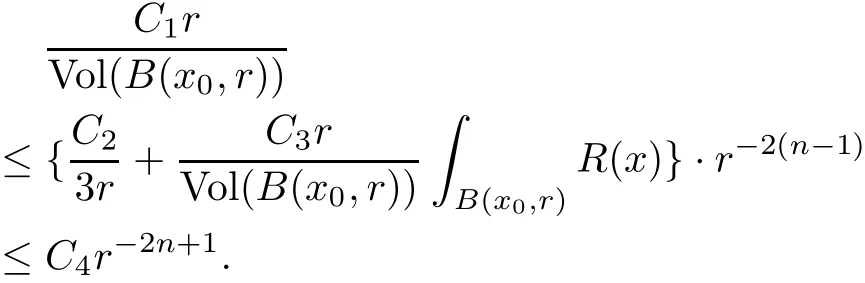
Then one can get

M has maximal volume growth.This contradicts to the condition that the volume growth of M is slow.Hence Ric＞0 does not hold at arbitrary point.Theorem 1.3 is true.

[1]CHEEGER J,GROMOLL D.On the structure of complete manifolds of nonnegative curvature[J].Ann of Math,1972,96(2):413-443.

[2]GROMOLL D,MEYER W.On complete open manifolds of positive curvature[J].Ann of Math,1969,90(2): 75-90.

[3]GREENE R E,WU Hung-hsi.Analysis on noncompact K¨ahler manifolds[J].Proc Sympos Pure Math,Part II,Amer Math Soc,1977,30:69-100.

[4]MOK N.An embedding theorem of complete K¨ahler manifolds of positive bisectional curvature onto affine algebraic varieties[J].Bull Soc Math France,1984,112(2):197-250.

[5]CHAU A,TAM L F.On the complex structure of K¨ahler manifolds with nonnegative curvature[J].J Dif f Geom,2006,73(3):491-530.

[6]GREENE R E,WU Hung-hsi.C∞convex functions and manifolds of positive curvature[J].Acta Math, 1976,137:209-245.

[7]CHEN Bing-long,ZHU Xi-ping.On complete noncompact K¨ahler manifolds with positive bisectional curvature[J].Math Ann,2003,327(1):1-23.

[8]CHEN Bing-long,ZHU Xi-ping.Volume growth and curvature decay of positively curved K¨ahler manifolds[J].Quart J of Pure and Appl Math,2005,1(1):68-108.

[9]MOK N,SIU Yum-tong,YAU Shing-tung.The Poincar´e-Lelong equation on complete K¨ahler manifolds[J]. Compositio Math,1981,44(2):183-218.

[10]GREENE R E,WU Hung-hsi.Gap theorems for noncompact Riemannian manifolds[J].Duke Math J,1982, 49(3):731-756.

[11]CHEN Bing-long,ZHU Xi-ping.A gap theorem for complete noncompact manifolds with nonnegative Ricci curvature[J].Comm Anal Geom,2002,10(1):217-239.

[12]NI Lei.An optimal gap theorem[J].Invent Math,2012,189(3):737-761.

[13]NI Lei,SHI Yu-guang,TAM L F.Poisson equation,Poincar´e-Lelong equation and curvature decay on complete K¨ahler manifolds[J].J Dif fGeom,2001,57(2):339-388.

tion:53C20,53C21

CLC number:O189.3Document code:A

1002–0462(2014)02–0253–04

date:2012-10-19

Supported by the Natural Science Foundation of Zhejiang Province(LY13A010018)

Biography:JIAO Zhen-hua(1977-),male,native of Jining,Shandong,an associate professor of Hangzhou Dianzi University,engages in dif f erential geometry.