A New Nonlinear Integrable Couplings of Yang Equations Hierarchy and Its Hamiltonian Structure
2014-07-31WEIHanyuXIATiecheng
WEI Han-yu,XIA Tie-cheng
(1.College of Mathematics and Statistics,Zhoukou Normal University,Zhoukou 466001,China;2.Department of Mathematics,Shanghai University,Shanghai 200444,China)
A New Nonlinear Integrable Couplings of Yang Equations Hierarchy and Its Hamiltonian Structure
WEI Han-yu1,2,XIA Tie-cheng2
(1.College of Mathematics and Statistics,Zhoukou Normal University,Zhoukou 466001,China;2.Department of Mathematics,Shanghai University,Shanghai 200444,China)
Based on a kind of non-semisimple Lie algebras,we establish a way to construct nonlinear continuous integrable couplings.Variational identities over the associated loop algebras are used to furnish Hamiltonian structures of the resulting continuous couplings. As an illustrative example of the scheme is given nonlinear continuous integrable couplings of the Yang hierarchy.
zero curvature equations;integrable couplings;variational identities
§1.Introduction
Integrable couplings[12]are coupled systems of integrable equations,which has been introduced when we study of Virasoro symmetric algebras.It is an important problems to look for integrable couplings because integrable couplings have much richer mathematical structures and physical meanings.In recent years,many methods of searching for integrable couplingshave been developed[39],for example,the spectral matrices as follows

where the sub-spectral matrix U is associated with the given integrable equation ut=K(u). However,researcher f i nd out that obtained integrable couplings is relative simple.So in order to get better integrable couplings of the known integrable system,we need to introduce an enlarged relatively complex spectral matrix[10-11]

From a zero curvature representation

where
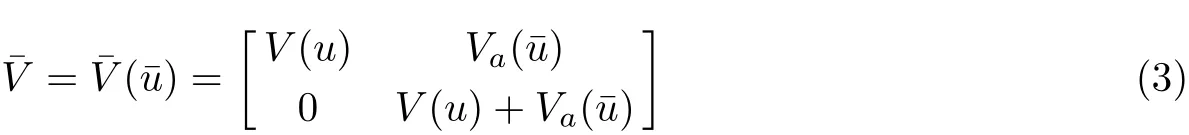
and¯u consist of u and v,we can obtain

This is an integrable couplings of Eq(1),and it is a nonlinear integrable coupling because the commutator[Ua,Va]can generate nonlinear terms.
Let us take a solution¯W to the enlarged stationary zero curvature equation

Then,we use the variational identity[6-7]

to search for the Hamiltonian structures of the integrable couplings[7].In the variational identity(6),〈.,.〉is non-degenerate,symmetric and ad-invariant bilinear form over the Lie algebra

In what follows,we will make an application of this general scheme to the Yang hierarchy.
§2.The Integrable Couplings of the Yang Equations Hierarchy
2.1Yang Hierarchy
From the spectral problem[12]

Setting

The stationary zero curvature equation Vx=[U,V]yields that

Choose the initial data
then we have

From the compatibility conditions of the following problems


The Hamiltonian operator J,the hereditary recursion operator L and the Hamiltonian functions Hnas follows

(1.8)
2.2Integrable Couplings
Let us begin with an enlarged spectral matrix

with the help of the corresponding enlarged stationary zero curvature equation¯Vx=[¯U,¯V],we have

which generates

Setting

Then,the Eq(1.11)can be transformed into

We choose the initial data

then we have

By using the zero curvature equation,


Then,we have the following results
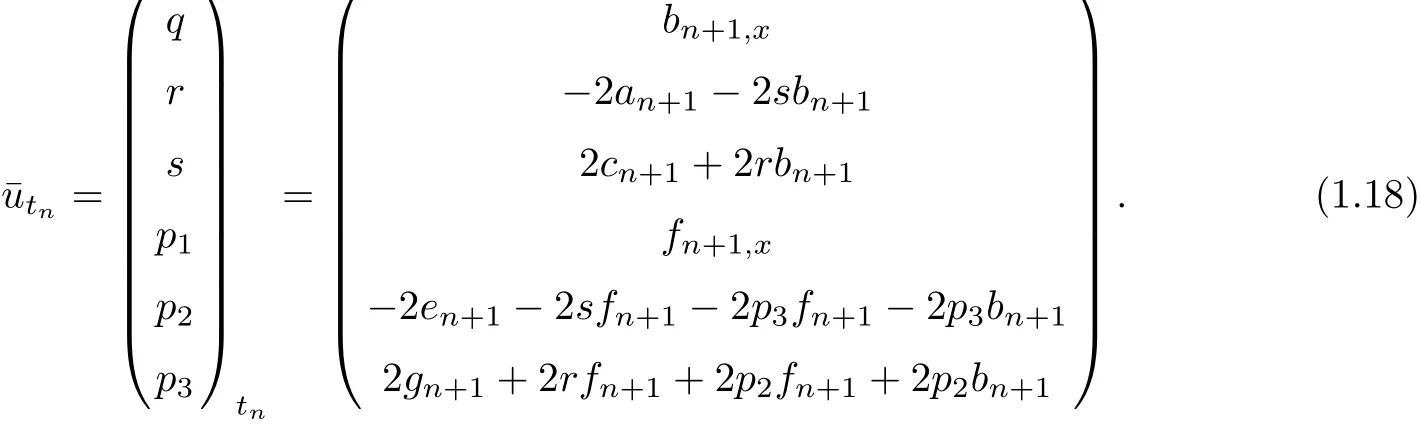
Obviously,when p1=p2=p3=0 in Eq(1.18),the above results become Eq(1.7).So we can say Eq(1.18)is the integrable couplings of Yang hierarchy.When n=2,the Eq(1.18)can reduced to b

where

So,we can say that the system in(1.18)with n≥2 provide nonlinear integrable couplings of the Yang hierarchy.
§3.Hamiltonian Structures of the Integrable Couplings of the Yang Equations Hierarchy
To construct Hamiltonian structures of the integrable couplings obtained,we need to compute non-degenerate,symmetric and invariant bilinear forms on the following Lie algebra

For computations convenience,we transform this Lie algebra¯g into a vector from through the mapping

The mapping δ induces a Lie algebraic structure on R6,isomorphic to the matrix Lie algebra ¯g above.It is easy to see that the corresponding commutator[.,.]on R6is given by

where
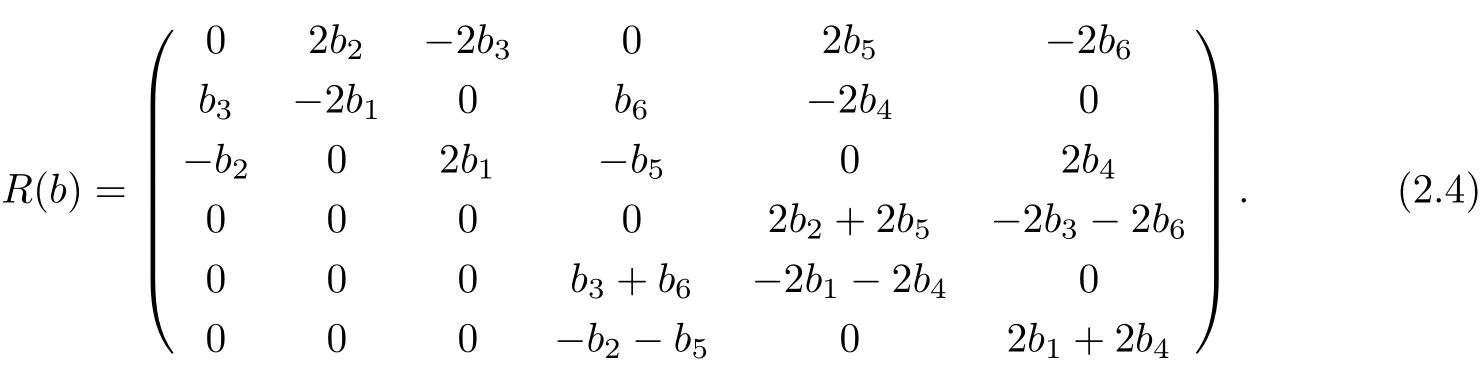
Def i ne a bilinear form on R6as follows

where F is a constant matrix,which is main idea by Zhang and Guo presented in 2005[5].
Then the symmetric property〈a,b〉=〈b,a〉and the ad-invariance property under the Lie product

requires that FT=F and

So we can obtain

where η1and η2are arbitrary constants.Therefore,a bilinear form on the underlying Lie algebra¯g is def i ned by
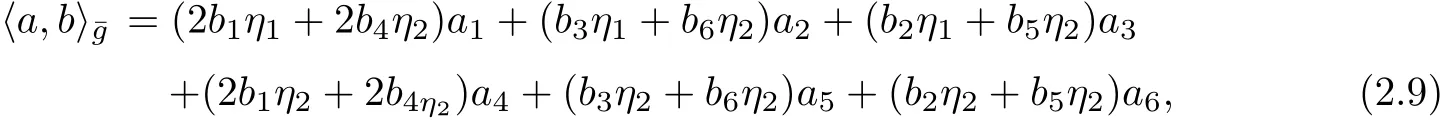
where

It is non-degenerate if and only if

Based on(2.12)and Eqs(1.9)∼(1.10),we can easily compute that

By applying the operator Γn+2to both sides of variational identity(6)

So we obtain that equation hierarchy(1.18)possess the Hamiltonian structures

where the Hamiltonian operator¯J and the Hamiltonian functional Hnare given by

and

With the help of(1.16),we can see a recursion relation

with

where L is given by(1.8)and

Up to now,we have already obtained Hamiltonian structure for integrable couplings of the Yang hierarchy.We must point out that by changing the nonlinear coupling terms of equations, more nonlinear integrable couplings with physical meaning can be obtained.So,with the help of this method,more meaningful results of other integrable hierarchies can be generated.
[References]
[1]MA Wen-xiu,FUCHSSTEINER B.Integrable theory of the perturbation equationa[J].Chaos,Solitons and Fractals,1996,7(8):1227-1250.
[2]MA Wen-xiu.Enlarging spectral problems to construct integrable couplings of soliton equations[J].Physics Letters A,2003,316(1-2):72-76.
[3]XIA Tie-cheng,ZHANG Gai-lian,FAN En-gui.New integrable couplings of generalized Kaup-Newell hierarchy and its Hamiltonian structures[J].Communications in Theoretical Physics,2011,56(1):1-4.
[4]LIU Yu-qing,CHEN Deng-yuan.The exact solutions to a Ragnisco-Tu hierarchy with self-consistent sources[J].Nonlinear Analysis,2011,74(16):5223-5237.
[5]MA Wen-Xiu,XU Xi-Xiang,ZHANG Yu-feng.Semi-direct sums of Lie algebras and discrete integrable couplings[J].Physics Letters A,2006,351(3):125-130.
[6]GUO Fu-kui,ZHANG Yu-feng.The quadratic-form identity for constructing the Hamiltonian structure of integrable systems[J].Journal of Physics A:Mathematical and General,2005,38(40):8537-8548.
[7]MA Wen-xiu.A Hamiltonian structure associated with a matrix spectral problem of arbitrary-order[J]. Physics Letters A,2007,367(6):473-477.
[8]GUO Fu-kui,ZHANG Yu-feng.The integrable coupling of the AKNS hierarchy and its Hamiltonian structure[J].Chaos,Solitons and Fractals,2007,32(5):1898-1902.
[9]MA Wen-xiu,GAO Liang.Coupling integrable couplings[J].Modern Physics Letters B,2009,23(15):1847-1860.
[10]MA Wen-xiu,ZHU Zuo-nong,Constructing nonlinear discrete integrable Hamiltonian couplings[J].Computers and Mathematics with Application,2010,60(9):2601-2608.
[11]MA Wen-xiu.Nonlinear conitnuous integrable Hamiltonian couplings[J].Applied Mathematics and Computation,2011,217(17):7238-7244.
[12]TU Gui-zhang.On liouville integrability of zero-curvature equations and the Yang hierarchy[J].1989,22(13): 2375-2392.
tion:35Q51
CLC number:O175.9Document code:A
1002–0462(2014)02–0180–09
date:2012-04-16
Supported by the Natural Science Foundation of China(11271008,61072147,11071159); Supported by the First-class Discipline of Universities in Shanghai;Supported by the Shanghai University Leading Academic Discipline Project(A13-0101-12-004)
Biography:WEI Han-yu(1982-),male,native of Zhoukou,Henan,a lecturer of Zhoukou Normal University, Ph.D.,engages in solitons and integrable systems.
杂志排行
Chinese Quarterly Journal of Mathematics的其它文章
- Group Twisted Tensor Biproducts over Hopf Group Coalgebras
- A Class of Solvable Lie Algebras and Their Hom-Lie Algebra Structures
- Positive Solutions for Fourth-order Delay Dif f erential Equation of Boundary Value Problem with p-Laplacian
- Cyclic Codes overF2+uF2+vF2
- The Existence and Uniqueness of the Solution for Periodical Boundary Value Problems of 2kth Order Dif f erential Equations with Resonance
- On the Characterization of Cyclic Codes over RingF2+uF2+vF2
