关于n阶可微函数的加权Ostrowski型不等式
2014-07-06时统业韦晓萍
时统业, 吴 涵, 韦晓萍
(海军指挥学院 浦口分院, 南京 211800)
关于n阶可微函数的加权Ostrowski型不等式
时统业, 吴 涵, 韦晓萍
(海军指挥学院 浦口分院, 南京 211800)
针对n阶可微函数, 利用积分恒等式和积分性质建立了两个带有权函数的Ostrowski不等式.
Ostrowski型不等式;n阶可微函数; 权函数
引言
在1938年, Ostrowski对具有一阶有界导函数的可微函数, 建立了下面的不等式.
定理A[1]设:[,]fa b→R是(a,b)上的可微函数, 且其导函数f′在(a,b)上有界, 则对任意x∈[a,b], 有
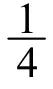
近些年来, 已有许多文献给出Ostrowski不等式的各种改进和推广, 比如文[1~12].本文考虑n阶可微函数, 给出一些新的Ostrowski型不等式.
为此, 需要引入函数k(t):
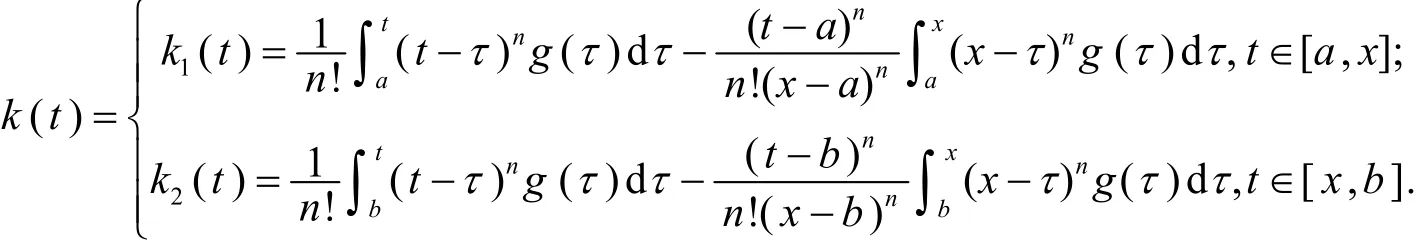
并考虑其简单的性态, 其中g:[a,b]→R是正的可积函数, 且定义函数

引理1函数k1(t)和k2(t)可分别表示为
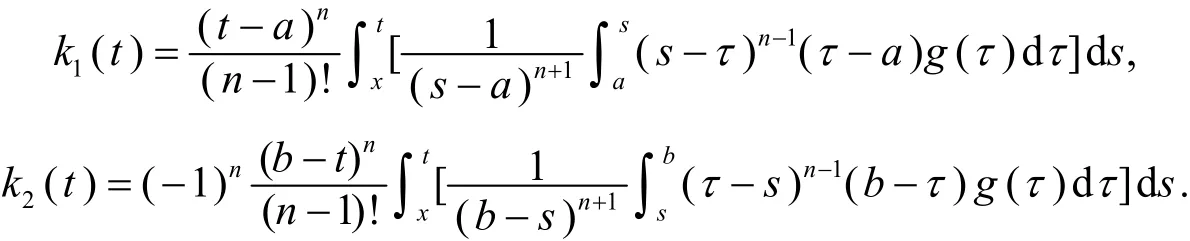
由此可见k1(t)≤0,t∈[a,x]; (−1)nk2(t)≥0,t∈[x,b].
证明我们有

类似可得k2(t)的另一表达式.
为方便起见, 记
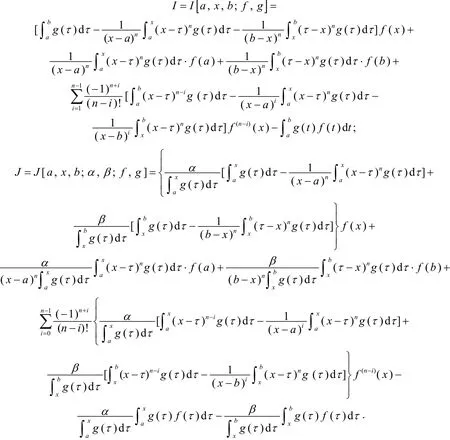
引理2设f:[a,b]→R是(a,b)上的n+1阶可微函数, 且f(n+1)∈L1[a,b], 则有

证明由分部积分法, 得

由k1(t)和k2(t)的定义, 对i=0,1,…,n, 容易得到
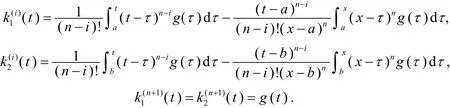
于是

式(2)和(3)相加得式(1).
引理3设f:[a,b]→R是(a,b)上的n+1阶可微函数, 且f(n+1)∈L1[a,b], α, β∈(0,1), α+β=1,则有

证明

主要结果
定理1设f:[a,b]→R是(a,b)上的n+1阶可微函数, 且f(n+1)∈L1[a,b]. 若||f(n+1)||∞= sup|f(n+1)(t)|<∞, 则有

证明由引理2, 得

类似可得

由式(6)、(7)、(8)得式(5).
注1在定理1中, 分别取n=0,1,2, 则有
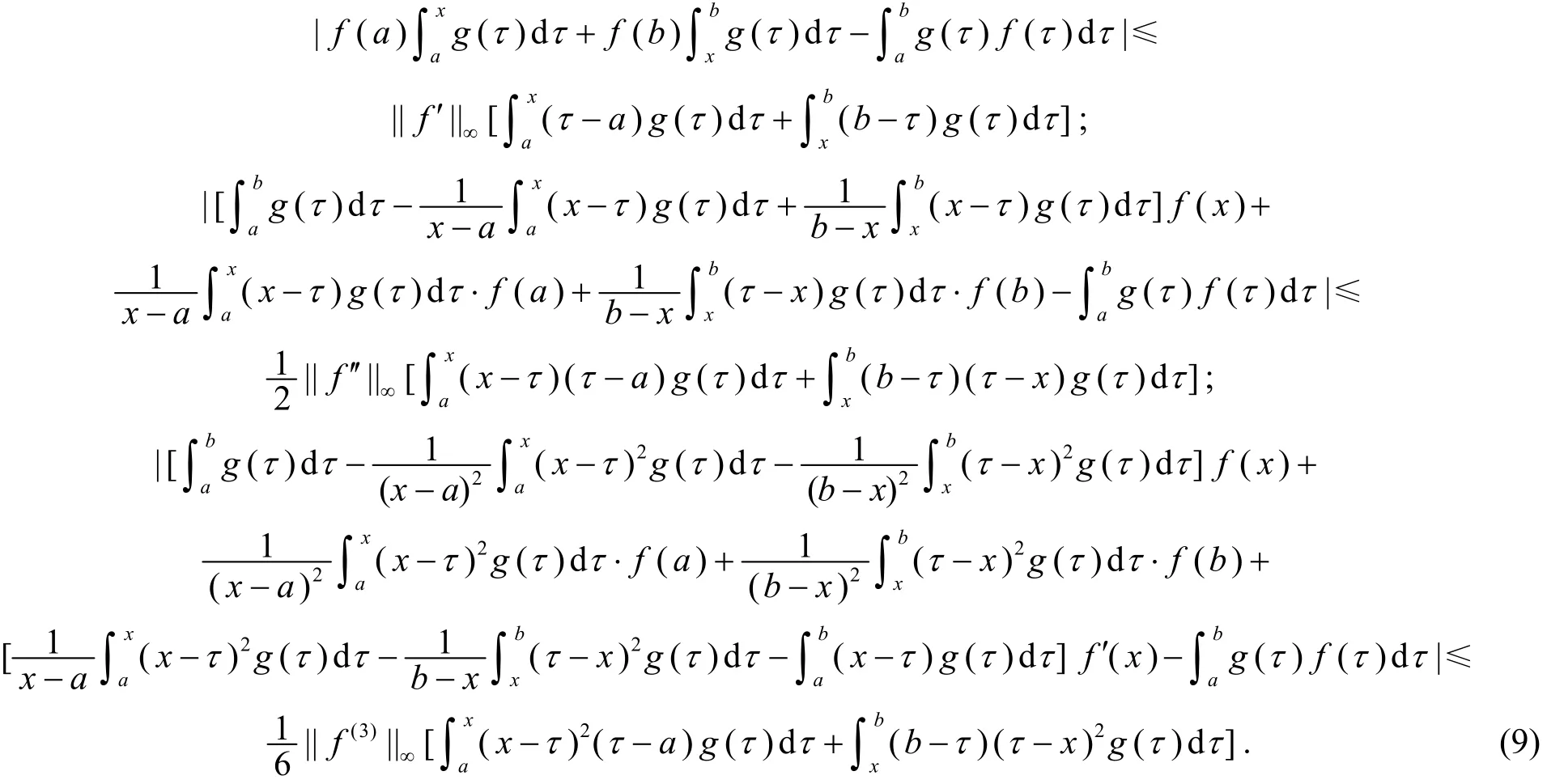
若g关于对称, 在式(9)中取则有

注2在定理1中, 取g≡1, 则有
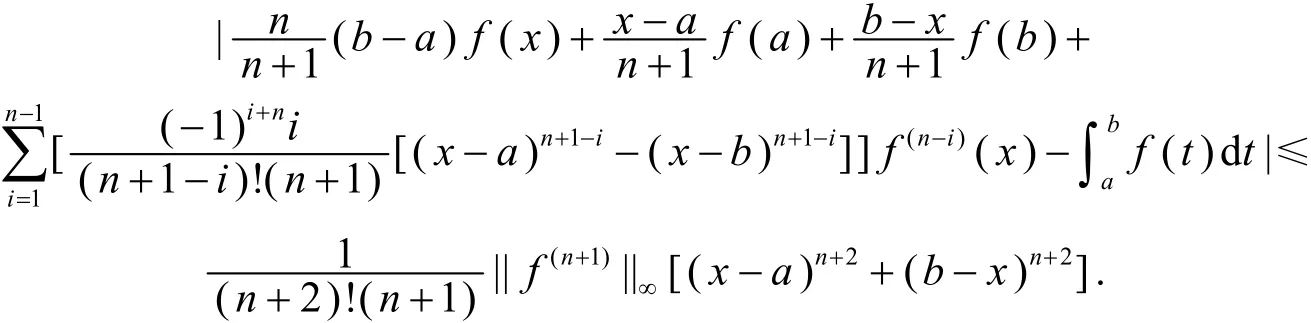
特别地, 取n=2, 则有
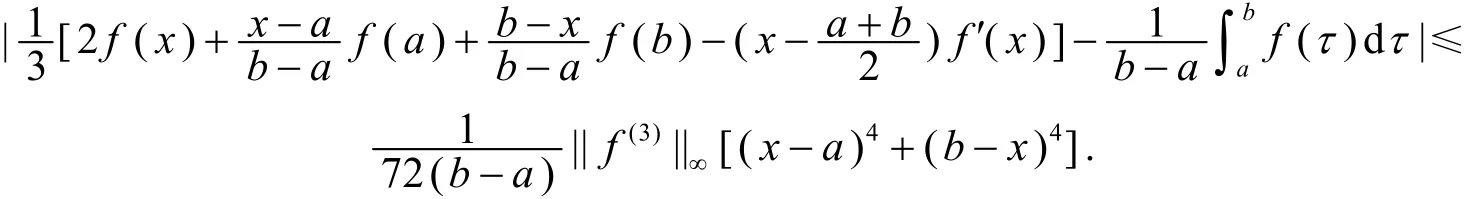

定理2设f:[a,b]→R是(a,b)上的n+1阶可微函数, 且f(n+1)∈L1[a,b], α,β∈(0,1), α+β=1, 则有
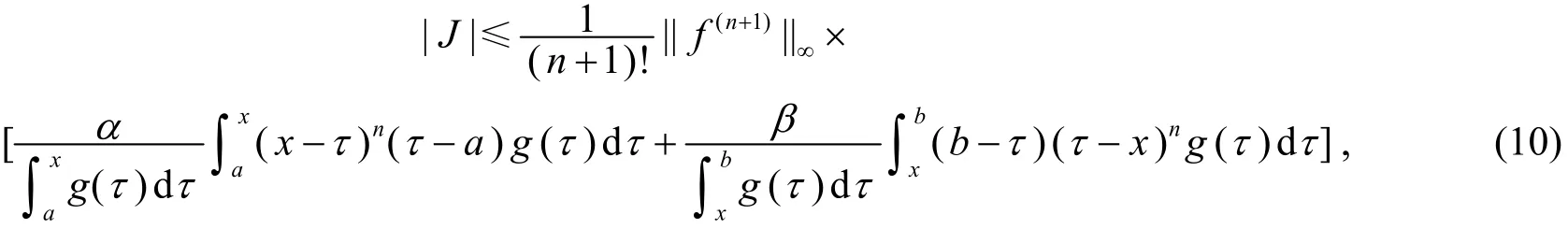
证明由引理3, 得
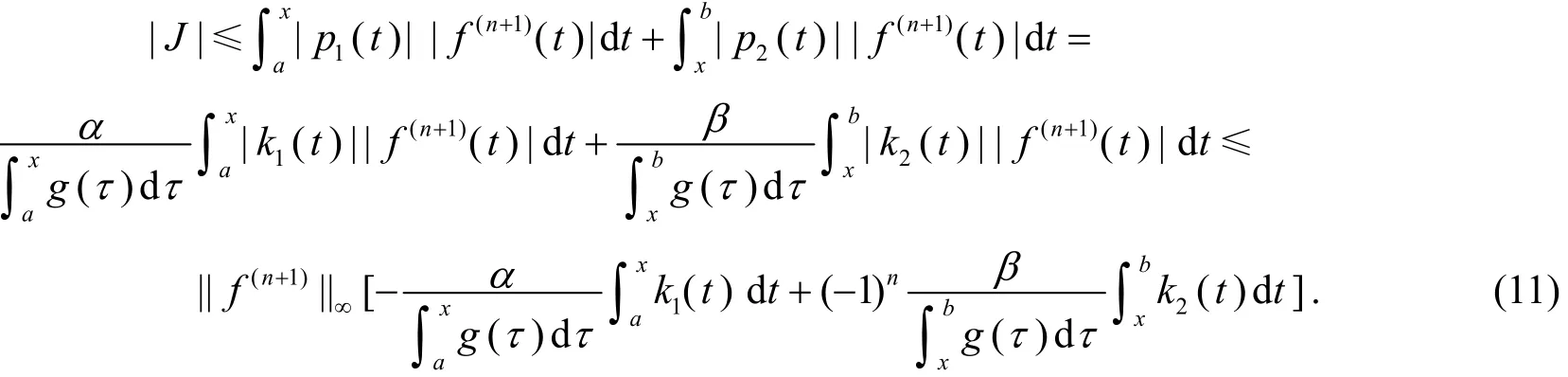
将式(7)、(8)带入式(11), 得到式(10).
注3在定理3中, 取g≡1, 则有


注4在定理3中, 取n=1, 则有

在上式中取g≡1, 则有
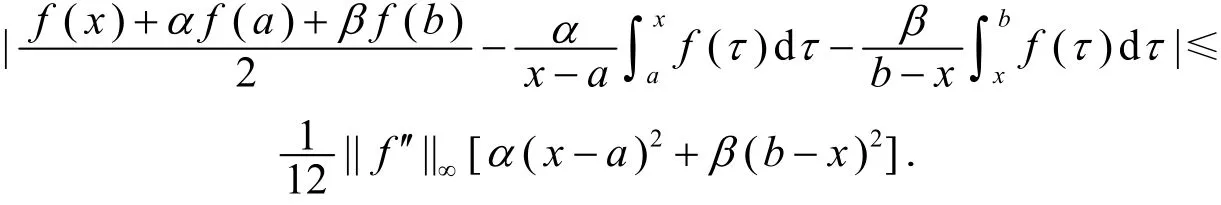

定理3设f:[a,b]→R是(a,b)上的n+1阶可微函数, 且f(n+1)∈Lp[a,b], 则有

其中B表示Belta函数.
证明由引理2和Hölder不等式, 得

因为g≤A, 故由引理1, 得

于是

类似地, 有

由式(13)、(14)、(15)得式(12).
注5在定理3中, 取g≡1, 则有
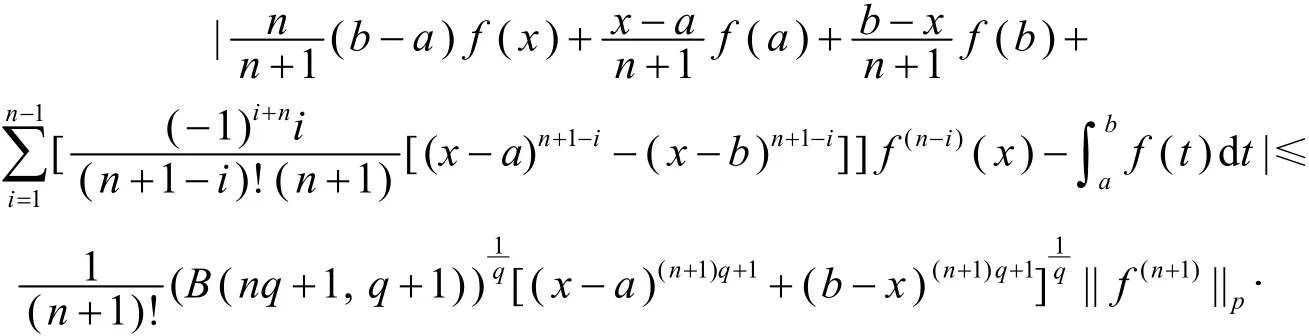
定理4设f:[a,b]→R是(a,b)上的n+1阶可微函数, 且f(n+1)∈Lp[a,b], α,β∈(0,1), α+β=1, 则有
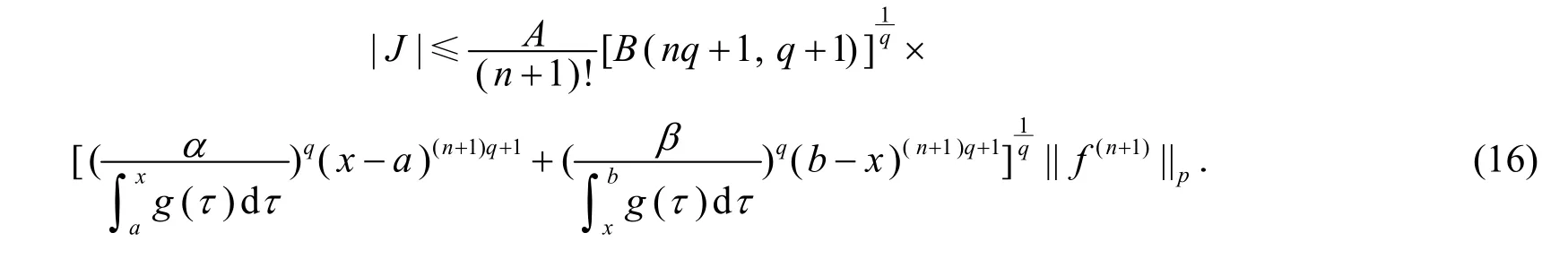
证明由引理3和Hölder不等式, 得

由式(14)、(15)、(17)得式(16).
注6在定理4中, 取g≡1, 则有

在上式中, 取n=1, 则有

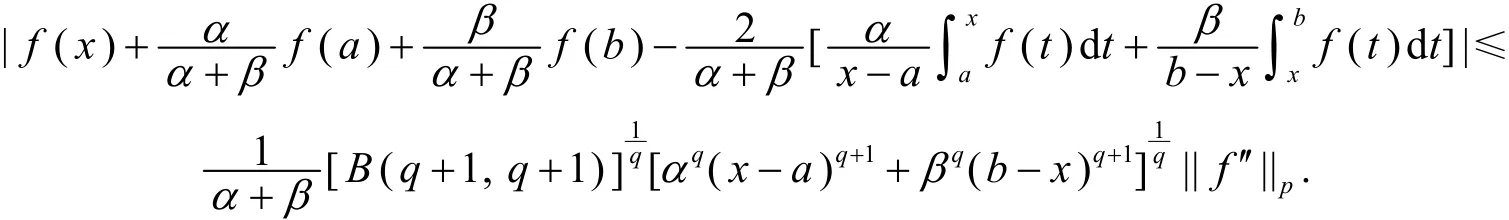
[1] Ostrowski, A.Über die Absolutabweichung einer differentiierbaren Funktion von ihrem Integralmittelwert[J]. Comment. Math. Helv. 1938, 10(1): 226~227
[2] Cerone P. Dragomir S S, Roumeliotis J.An inequality of Ostrowski type for mappings whose second derivatives are bounded and applications[J]. RGMIA Research Report Collection 1(2) (1998).
[3] Cerone P.A new Ostrowski type inequality involving integral means over end intervals[J]. Tamkang J. Math.2002, 33(2): 109~118
[4] Sofo A, Dragomir S S.An inequality of Ostrowski type for twice differentiable mappings in term of the Lpnorm and applications[J]. Soochow J. Math. 2001, 27(1): 97~111
[5] Cerone P, Dragomir S S, Roumeliotis J.Some Ostrowski type inequalities for n-time differentiable mappings and applications[J]. RGMIA Research Report Collection 1(1) (1998).
[6] Barnett N S, Dragomir S S.An identity for n-time differentiable functions and applications for Ostrowski type inequalities[J].RGMIA Research Report Collection 6(2) Article7, (2003).
[7] Rafiq A, Mir N A, Ahmad F.Weighted Ostrowski type inequality for differentiable mappings whose first derivatives belong toLp(a,b)[J]. General mathematics, 2006, 14(3): 91~102
[8] Barnett N S, Dragomir S S.On the weighted Ostrowski inequality[J]. JIMPA, 2007,8(4). Article 96
[9] Liu wei J, Rüzgar H, Tuna A.Some weighted Ostrowski type inequalities on time scales involving combination of weighted Delta-integral means[J/OL]. arXiv preprint. arXiv: 1207. 4243, 2012
[10] Aljinović A A, Pečarić.On some Ostrowski type inequalities via montgomery identity and Taylor′s formula[J]. Tamkang Journal of Mathematics, 2005, 36(3): 199~218
[11] Liu Z.An inequality of Simpson type[J]. Proc. R. Soc.A, 2005(461): 2155~2158
[12] Sarikaya M Z, Set E.On new Ostrowski type integral inequalities[J]. Thai Journal of Mathematics, 2014(12): 145~154
Some Weighted Ostrowski Type Inequalities for n-Time Differentiable Functions
SHI Tong-ye, WU Han, WEI Xiao-ping
(Pukou Institute, Naval Command College, Nanjing 211800, China)
Two weighted Ostrowski type inequalities forn-time differentiable functions are obtained by using the identity and the integral property.
Ostrowski type inequality;n-time differentiable function; weight function
O178
A
1672-5298(2014)03-0007-07
2014-05-18
时统业(1963−), 男, 河北张家口人, 硕士, 海军指挥学院浦口分院副教授. 主要研究方向: 基础数学
